| ||||
|---|---|---|---|---|
| ← 20 21 22 23 24 25 26 27 28 29 → ← 0 10 20 30 40 50 60 70 80 90 → | ||||
| Cardinal | twenty-five | |||
| Ordinal | 25th (twenty-fifth) | |||
| Factorization | 5 | |||
| Divisors | 1, 5, 25 | |||
| Greek numeral | ΚΕ´ | |||
| Roman numeral | XXV, xxv | |||
| Binary | 110012 | |||
| Ternary | 2213 | |||
| Senary | 416 | |||
| Octal | 318 | |||
| Duodecimal | 2112 | |||
| Hexadecimal | 1916 | |||
25 (twenty-five) is the natural number following 24 and preceding 26.
In mathematics
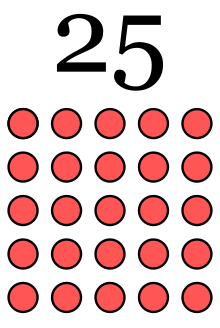
It is a square number, being 5 = 5 × 5, and hence the third non-unitary square prime of the form p.
It is one of two two-digit numbers whose square and higher powers of the number also ends in the same last two digits, e.g., 25 = 625; the other is 76.
25 has an even aliquot sum of 6, which is itself the first even and perfect number root of an aliquot sequence; not ending in (1 and 0).
It is the smallest square that is also a sum of two (non-zero) squares: 25 = 3 + 4. Hence, it often appears in illustrations of the Pythagorean theorem.
25 is the sum of the five consecutive single-digit odd natural numbers 1, 3, 5, 7, and 9.
25 is a centered octagonal number, a centered square number, a centered octahedral number, and an automorphic number.
25 percent (%) is equal to 1/4.
It is the smallest decimal Friedman number as it can be expressed by its own digits: 5.
It is also a Cullen number and a vertically symmetrical number. 25 is the smallest pseudoprime satisfying the congruence 7 = 7 mod n.
25 is the smallest aspiring number — a composite non-sociable number whose aliquot sequence does not terminate.
According to the Shapiro inequality, 25 is the smallest odd integer n such that there exist x1, x2, ..., xn such that
where xn + 1 = x1, xn + 2 = x2.
Within decimal, one can readily test for divisibility by 25 by seeing if the last two digits of the number match 00, 25, 50, or 75.
There are 25 primes under 100: 2, 3, 5, 7, 11, 13, 17, 19, 23, 29, 31, 37, 41, 43, 47, 53, 59, 61, 67, 71, 73, 79, 83, 89, 97.
F4, H4 symmetry and lattices Λ24, II25,1
Twenty-five 24-cells with symmetry in the fourth dimension can be arranged in two distinct manners, such that
- in a 24-cell honeycomb, twenty-four 24-cells surround a single 24-cell, and where
- a faceting of the 600-cell with symmetry can otherwise also be constructed, with cells overlapping.
The 24-cell can be further generated using three copies of the 8-cell, where the 24-cell honeycomb is dual to the 16-cell honeycomb (with the tesseract the dual polytope to the 16-cell).
On the other hand, the positive unimodular lattice in twenty-six dimensions is constructed from the Leech lattice in twenty-four dimensions using Weyl vector
that features the only non-trivial solution, i.e. aside from , to the cannonball problem where sum of the squares of the first twenty-five natural numbers in is in equivalence with the square of (that is the fiftieth composite). The Leech lattice, meanwhile, is constructed in multiple ways, one of which is through copies of the lattice in eight dimensions isomorphic to the 600-cell, where twenty-five 24-cells fit; a set of these twenty-five integers can also generate the twenty-fourth triangular number, whose value twice over is
In religion
- In Ezekiel's vision of a new temple: The number twenty-five is of cardinal importance in Ezekiel's Temple Vision (in the Bible, Ezekiel chapters 40–48).
In sports
- In baseball, the number 25 is typically reserved for the best slugger on the team. Examples include Mark McGwire, Barry Bonds, Jim Thome, and Mark Teixeira.
In other fields
Twenty-five is:
- The number of years of marriage marked in a silver wedding anniversary.
References
- Sloane, N. J. A. (ed.). "Sequence A016754 (Odd squares: a(n) = (2n+1)^2. Also centered octagonal numbers)". The On-Line Encyclopedia of Integer Sequences. OEIS Foundation.
- Sloane, N. J. A. (ed.). "Sequence A001844 (Centered square numbers)". The On-Line Encyclopedia of Integer Sequences. OEIS Foundation.
- Sloane, N. J. A. (ed.). "Sequence A001845 (Centered octahedral numbers (crystal ball sequence for cubic lattice))". The On-Line Encyclopedia of Integer Sequences. OEIS Foundation.
- Sloane, N. J. A. (ed.). "Sequence A003226 (Automorphic numbers)". The On-Line Encyclopedia of Integer Sequences. OEIS Foundation.
- Sloane, N. J. A. (ed.). "Sequence A036057 (Friedman numbers)". The On-Line Encyclopedia of Integer Sequences. OEIS Foundation.
- Sloane, N. J. A. (ed.). "Sequence A002064 (Cullen numbers)". The On-Line Encyclopedia of Integer Sequences. OEIS Foundation.
- Sloane, N. J. A. (ed.). "Sequence A053701 (Vertically symmetric numbers)". The On-Line Encyclopedia of Integer Sequences. OEIS Foundation.
- Sloane, N. J. A. (ed.). "Sequence A063769 (Aspiring numbers)". The On-Line Encyclopedia of Integer Sequences. OEIS Foundation.
- Denney, Tomme; Hooker, Da’Shay; Johnson, De’Janeke; Robinson, Tianna; Butler, Majid; Sandernisha, Claiborne (2020). "The geometry of H4 polytopes". Advances in Geometry. 20 (3). Berlin: De Gruyter: 433–444. arXiv:1912.06156. doi:10.1515/advgeom-2020-0005. S2CID 220367622.
- Sloane, N. J. A. (ed.). "Sequence A351831 (Vector in the 26-dimensional even Lorentzian unimodular lattice II_25,1 used to construct the Leech lattice.)". The On-Line Encyclopedia of Integer Sequences. OEIS Foundation. Retrieved 2024-03-12.
- Conway, John H. (1999). "Chapter 26: Lorentzian forms for the Leech lattice". Sphere packings, lattices, and groups. Grundlehren der mathematischen Wissenschaften. Vol. 290 (1st ed.). New York: Springer. pp. 524–528. doi:10.1007/978-1-4757-6568-7. ISBN 978-0-387-98585-5. MR 1662447. OCLC 854794089.
- Sloane, N. J. A. (ed.). "Sequence A002808 (The composite numbers.)". The On-Line Encyclopedia of Integer Sequences. OEIS Foundation. Retrieved 2024-03-12.
- Conway, John H.; Sloane, N. J. A. (1988). "Algebraic Constructions for Lattices". Sphere Packings, Lattices and Groups. New York, NY: Springer. doi:10.1007/978-1-4757-2016-7. eISSN 2196-9701. ISBN 978-1-4757-2016-7. MR 1541550.
- Baez, John C. (2018). "From the Icosahedron to E8". London Mathematical Society Newsletter. 476: 18–23. arXiv:1712.06436. MR 3792329. S2CID 119151549. Zbl 1476.51020.
- Sloane, N. J. A. (ed.). "Sequence A002378 (Oblong (or promic, pronic, or heteromecic) numbers: a(n) equal to n*(n+1).)". The On-Line Encyclopedia of Integer Sequences. OEIS Foundation. Retrieved 2024-03-16.
- "Number 25 meaning in the Bible". Bible Wings. 2023-07-21. Retrieved 2023-11-02.
| Integers | |||||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| −1 | |||||||||||||||||||||||
| |||||||||||||||||||||||
| |||||||||||||||||||||||
| |||||||||||||||||||||||
| |||||||||||||||||||||||
| |||||||||||||||||||||||
| |||||||||||||||||||||||
| |||||||||||||||||||||||
| |||||||||||||||||||||||
| |||||||||||||||||||||||
| |||||||||||||||||||||||
|


 symmetry can otherwise also be constructed, with cells overlapping.
symmetry can otherwise also be constructed, with cells overlapping. in twenty-six dimensions is constructed from the
in twenty-six dimensions is constructed from the 
 , to the
, to the  in
in  is in equivalence with the square of
is in equivalence with the square of  (that is the fiftieth
(that is the fiftieth 
