| This article does not cite any sources. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed. Find sources: "Boole's syllogistic" – news · newspapers · books · scholar · JSTOR (December 2009) (Learn how and when to remove this message) |
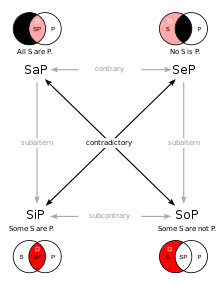
In the Venn diagrams black areas are empty and red areas are nonempty.
The faded arrows and faded red areas apply in traditional logic.
Boolean logic is a system of syllogistic logic invented by 19th-century British mathematician George Boole, which attempts to incorporate the "empty set", that is, a class of non-existent entities, such as round squares, without resorting to uncertain truth values.
In Boolean logic, the universal statements "all S is P" and "no S is P" (contraries in the traditional Aristotelian schema) are compossible provided that the set of "S" is the empty set. "All S is P" is construed to mean that "there is nothing that is both S and not-P"; "no S is P", that "there is nothing that is both S and P". For example, since there is nothing that is a round square, it is true both that nothing is a round square and purple, and that nothing is a round square and not-purple. Therefore, both universal statements, that "all round squares are purple" and "no round squares are purple" are true.
Similarly, the subcontrary relationship is dissolved between the existential statements "some S is P" and "some S is not P". The former is interpreted as "there is some S such that S is P" and the latter, "there is some S such that S is not P", both of which are clearly false where S is nonexistent.
Thus, the subaltern relationship between universal and existential also does not hold, since for a nonexistent S, "All S is P" is true but does not entail "Some S is P", which is false. Of the Aristotelian square of opposition, only the contradictory relationships remain intact.
See also
References
list of Boolean algebra topics
Categories: