| hexaoctagonal tiling | |
|---|---|
 Poincaré disk model of the hyperbolic plane | |
| Type | Hyperbolic uniform tiling |
| Vertex configuration | (6.8) |
| Schläfli symbol | r{8,6} or |
| Wythoff symbol | 2 | 8 6 |
| Coxeter diagram | |
| Symmetry group | , (*862) |
| Dual | Order-8-6 quasiregular rhombic tiling |
| Properties | Vertex-transitive edge-transitive |
In geometry, the hexaoctagonal tiling is a uniform tiling of the hyperbolic plane.
Constructions
There are four uniform constructions of this tiling, three of them as constructed by mirror removal from the kaleidoscope. Removing the mirror between the order 2 and 4 points, , gives , (*883). Removing the mirror between the order 2 and 8 points, , gives , (*664). Removing two mirrors as , leaves remaining mirrors (*4343).
| Uniform Coloring |

|

|

|
|
|---|---|---|---|---|
| Symmetry | (*862) |
= (*883) |
= (*664) |
(*4343) |
| Symbol | r{8,6} | r{(8,3,8)} | r{(6,4,6)} | |
| Coxeter diagram |
Symmetry
The dual tiling has face configuration V6.8.6.8, and represents the fundamental domains of a quadrilateral kaleidoscope, orbifold (*4343), shown here. Adding a 2-fold gyration point at the center of each rhombi defines a (2*43) orbifold. These are subsymmetries of .
 , (*4343) |
 , (2*43) |
|---|
Related polyhedra and tiling
| Uniform octagonal/hexagonal tilings | ||||||
|---|---|---|---|---|---|---|
| Symmetry: , (*862) | ||||||

|
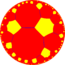
|

|

|

|

|

|
| {8,6} | t{8,6} |
r{8,6} | 2t{8,6}=t{6,8} | 2r{8,6}={6,8} | rr{8,6} | tr{8,6} |
| Uniform duals | ||||||

|

|

|

|

|

|

|
| V8 | V6.16.16 | V(6.8) | V8.12.12 | V6 | V4.6.4.8 | V4.12.16 |
| Alternations | ||||||
(*466) |
(8*3) |
(*4232) |
(6*4) |
(*883) |
(2*43) |
(862) |

|

|

| ||||
| h{8,6} | s{8,6} | hr{8,6} | s{6,8} | h{6,8} | hrr{8,6} | sr{8,6} |
| Alternation duals | ||||||

|
||||||
| V(4.6) | V3.3.8.3.8.3 | V(3.4.4.4) | V3.4.3.4.3.6 | V(3.8) | V3.4 | V3.3.6.3.8 |
| Symmetry mutation of quasiregular tilings: (6.n) | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Symmetry *6n2 |
Euclidean | Compact hyperbolic | Paracompact | Noncompact | |||||||
| *632 |
*642 |
*652 |
*662 |
*762 |
*862 ... |
*∞62 |
| ||||
| Quasiregular figures configuration |
 6.3.6.3 |
 6.4.6.4 |
 6.5.6.5 |
 6.6.6.6 |
 6.7.6.7 |
 6.8.6.8 |
 6.∞.6.∞ |
6.∞.6.∞ | |||
| Dual figures | |||||||||||
| Rhombic figures configuration |
 V6.3.6.3 |
 V6.4.6.4 |
 V6.5.6.5 |
 V6.6.6.6 |
V6.7.6.7 |
 V6.8.6.8 |
 V6.∞.6.∞ |
||||
| Dimensional family of quasiregular polyhedra and tilings: (8.n) | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Symmetry *8n2 |
Hyperbolic... | Paracompact | Noncompact | ||||||||
| *832 |
*842 |
*852 |
*862 |
*872 |
*882 ... |
*∞82 |
| ||||
| Coxeter | |||||||||||
| Quasiregular figures configuration |
 3.8.3.8 |
 4.8.4.8 |
 8.5.8.5 |
 8.6.8.6 |
 8.7.8.7 |
 8.8.8.8 |
 8.∞.8.∞ |
8.∞.8.∞ | |||
See also
References
- John H. Conway, Heidi Burgiel, Chaim Goodman-Strauss, The Symmetries of Things 2008, ISBN 978-1-56881-220-5 (Chapter 19, The Hyperbolic Archimedean Tessellations)
- "Chapter 10: Regular honeycombs in hyperbolic space". The Beauty of Geometry: Twelve Essays. Dover Publications. 1999. ISBN 0-486-40919-8. LCCN 99035678.
External links
- Weisstein, Eric W. "Hyperbolic tiling". MathWorld.
- Weisstein, Eric W. "Poincaré hyperbolic disk". MathWorld.
- Hyperbolic and Spherical Tiling Gallery Archived 2013-03-24 at the Wayback Machine
- KaleidoTile 3: Educational software to create spherical, planar and hyperbolic tilings
- Hyperbolic Planar Tessellations, Don Hatch
| Tessellation | |||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
|   | ||||||||||||
| |||||||||||||
| |||||||||||||
| |||||||||||||
