|
The ''']''' ('''MWI''') is a philosophical position about how the mathematics used in quantum mechanics relates to physical reality. It asserts that the ] is ], and that there is no ]. This implies that all possible outcomes of quantum measurements are physically realized in some "world" or universe. In contrast to some other interpretations of quantum mechanics, the evolution of reality as a whole in MWI is rigidly deterministic and local. Many-worlds is also called the relative state formulation or the Everett interpretation, after physicist Hugh Everett, who first proposed it in 1957. Bryce DeWitt popularized the formulation and named it many-worlds in the 1970s. In this interpretation, for the "Schrödinger's cat" paradox, every quantum event is a branch point; the cat is both alive and dead, even before the box is opened, but the "alive" and "dead" cats are in different branches of the multiverse, both of which are equally real, but which do not interact with each other |
|
The ''']''' ('''MWI''') is a philosophical position about how the mathematics used in quantum mechanics relates to physical reality. It asserts that the ] is ], and that there is no ]. This implies that all ] outcomes of quantum measurements are physically realized in some "world" or universe. In contrast to some other ], the evolution of reality as a whole in MWI is rigidly ] and ]. Many-worlds is also called the relative state formulation or the Everett interpretation, after physicist ], who first proposed it in 1957. ] popularized the formulation and named it many-worlds in the 1970s. According to this interpretation: in the "]" paradox, every quantum event is a branch point; the cat is both alive and dead, even before the box is opened, but the "alive" and "dead" cats are in different branches of the multiverse, both of which are equally real, but which do not interact with each other |
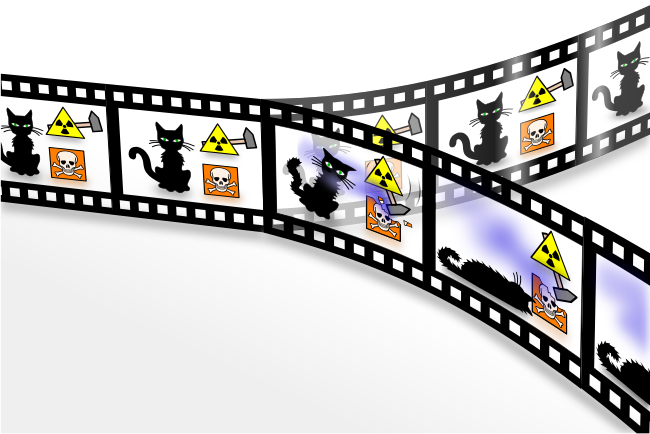 The many-worlds interpretation (MWI) is a philosophical position about how the mathematics used in quantum mechanics relates to physical reality. It asserts that the universal wavefunction is objectively real, and that there is no wave function collapse. This implies that all possible outcomes of quantum measurements are physically realized in some "world" or universe. In contrast to some other interpretations of quantum mechanics, the evolution of reality as a whole in MWI is rigidly deterministic and local. Many-worlds is also called the relative state formulation or the Everett interpretation, after physicist Hugh Everett, who first proposed it in 1957. Bryce DeWitt popularized the formulation and named it many-worlds in the 1970s. According to this interpretation: in the "Schrödinger's cat" paradox, every quantum event is a branch point; the cat is both alive and dead, even before the box is opened, but the "alive" and "dead" cats are in different branches of the multiverse, both of which are equally real, but which do not interact with each otherIllustration credit: Christian Schirm
Archive – More featured pictures...
The many-worlds interpretation (MWI) is a philosophical position about how the mathematics used in quantum mechanics relates to physical reality. It asserts that the universal wavefunction is objectively real, and that there is no wave function collapse. This implies that all possible outcomes of quantum measurements are physically realized in some "world" or universe. In contrast to some other interpretations of quantum mechanics, the evolution of reality as a whole in MWI is rigidly deterministic and local. Many-worlds is also called the relative state formulation or the Everett interpretation, after physicist Hugh Everett, who first proposed it in 1957. Bryce DeWitt popularized the formulation and named it many-worlds in the 1970s. According to this interpretation: in the "Schrödinger's cat" paradox, every quantum event is a branch point; the cat is both alive and dead, even before the box is opened, but the "alive" and "dead" cats are in different branches of the multiverse, both of which are equally real, but which do not interact with each otherIllustration credit: Christian Schirm
Archive – More featured pictures...