| Revision as of 17:14, 16 February 2015 editDMacks (talk | contribs)Edit filter managers, Autopatrolled, Administrators186,985 editsm Protected Three-phase electric power: Persistent disruptive editing: sock against consensus ( (indefinite) (indefinite))← Previous edit | Latest revision as of 04:54, 3 January 2025 edit undoLfstevens (talk | contribs)Extended confirmed users68,794 edits Filled in 1 bare reference(s) with reFill 2 | ||
| (553 intermediate revisions by more than 100 users not shown) | |||
| Line 1: | Line 1: | ||
| {{Short description|Common electrical power generation, transmission and distribution method for alternating currents}} | |||
| {{Merge from|Three-phase|date=August 2014|discuss=Talk:Three-phase electric power#Merge}} | |||
| {{for|a greater focus on the mathematics of this subject|Mathematics of three-phase electric power}} | |||
| ] | |||
| {{Distinguish|Split-phase electric power}} | |||
| '''Three-phase electric power''' is a common method of ] ] ], ], and ].<ref>William D. Stevenson, Jr. ''Elements of Power System Analysis Third Edition'', McGraw-Hill, New York (1975). ISBN 0-07-061285-4, p. 2</ref> It is a type of ] and is the most common method used by ]s worldwide to transfer power. It is also used to power large ]s and other heavy loads. A ] system is usually more economical than an equivalent ] or ] system at the same line to ground ] because it uses less conductor material to transmit electrical power.<ref>http://www.allaboutcircuits.com/vol_2/chpt_10/2.html</ref> | |||
| ] | |||
| The three-phase system was independently invented by ], ], ] and ] in the late 1880s. | |||
| '''Three-phase electric power''' (abbreviated '''3ϕ'''<ref>{{Cite book|last1=Saleh|first1=S. A.|last2=Rahman|first2=M. A.|title=2012 IEEE International Conference on Power Electronics, Drives and Energy Systems (PEDES) |chapter=The analysis and development of controlled 3φ wavelet modulated AC-DC converter |date=25 March 2013|chapter-url=https://ieeexplore.ieee.org/document/6484282|pages=1–6|doi=10.1109/PEDES.2012.6484282|isbn=978-1-4673-4508-8|s2cid=32935308}}</ref>) is a common type of ] (AC) used in ], ], and ].<ref>William D. Stevenson, Jr. ''Elements of Power System Analysis Third Edition'', McGraw-Hill, New York (1975). {{ISBN|0-07-061285-4}}, p. 2</ref> It is a type of ] employing three wires (or four including an optional neutral return wire) and is the most common method used by ]s worldwide to transfer power. | |||
| == Principle == | |||
| ] | |||
| Three-phase electrical power was developed in the 1880s by several people. In three-phase power, the voltage on each wire is 120 degrees ]ed relative to each of the other wires. Because it is an AC system, it allows the voltages to be easily stepped up using ]s to high voltage for transmission and back down for distribution, giving high efficiency. | |||
| In a balanced three-phase power supply system (by far, the most common type), three conductors each carry an ] of the same frequency and voltage relative to a common reference (Typically such a reference is connected to ground and often to a current-carrying conductor called the neutral) but with a phase difference of one third the period; hence the voltage on any conductor reaches its peak at one third of a cycle after one of the other conductors and one third of a cycle before the third conductor. From any of the three conductors, the peak voltage on the other two conductors is delayed by one third and two thirds of one cycle respectively. This phase delay gives constant power transfer over each cycle. It also makes it possible to produce a rotating magnetic field in an ] and generate other phase arrangements using transformers (For instance, a two phase system using a ]). | |||
| A three-wire three-phase circuit is usually more economical than an equivalent two-wire ] circuit at the same line-to-ground ] because it uses less conductor material to transmit a given amount of electrical power.<ref>Terrell Croft, Wilford Summers (ed), ''American Electricians' Handbook, 11th ed.'', McGraw Hill, 1987 {{ISBN|0-07-013932-6}} page 3-10 figure 3-23.</ref> Three-phase power is mainly used directly to power large ], other ] and other heavy loads. Small loads often use only a two-wire single-phase circuit, which may be derived from a three-phase system. | |||
| With a perfectly balanced three phase supply the instantaneous voltage of any phase is exactly equal in magnitude but opposite to the sum of the other two phases. This means that if the load on the three phases is balanced as well, the return path for the current in any phase conductor is the other two phase conductors. | |||
| ==Terminology== | |||
| Hence, the sum of the currents in the three conductors is always zero and the current in each conductor is equal to and in the opposite direction as the sum of the currents in the other two. Thus, each conductor acts as the return path for the currents from the other two. | |||
| The ] between a ] and a ] are called lines, and the ] between any two lines is called '''line voltage'''. The voltage measured between any line and neutral is called '''phase voltage'''.<ref>{{cite book | last = Brumbach | first = Michael | title = Industrial maintenance |page=411 | publisher = Delmar, Cengage Learning | location = Clifton Park, NY | year = 2014 | isbn = 9781133131199 }}</ref> For example, for a 208/120-volt service, the line voltage is 208 volts, and the phase voltage is 120 volts. | |||
| == History == | |||
| While a single phase AC power supply requires two conductors (Go and Return), a three phase supply can transmit three times the power by using only one extra conductor. | |||
| ]s were independently invented by ], ], ], ], ], and ] in the late 1880s.<ref>{{cite web |title=AC Power History and Timeline |url=https://edisontechcenter.org/AC-PowerHistory.html |website=Edison Tech Center |access-date=January 24, 2022}}</ref> | |||
| This means that a 50% increase in transmission cost yields a 200% increase in the power transmitted.<ref>Cotton, H, ''Electrical Technology'', 6th Ed., Pitman, London, 1950, p. 268</ref> | |||
| ] developed by Italian physicist Galileo Ferraris. This was a two-phase motor and required four wires.]] | |||
| Three-phase systems may also utilize a fourth wire, particularly in low-voltage distribution. This is the ] wire. The neutral allows three separate single-phase supplies to be provided at a constant voltage and is commonly used for supplying groups of domestic properties which are each ] loads. The connections are arranged so that, as far as possible in each group, equal power is drawn from each phase. Further up the supply chain in high-voltage distribution the currents are usually well balanced and it is therefore normal to omit the neutral conductor. | |||
| Three phase power evolved out of electric motor development. In 1885, Galileo Ferraris was doing research on ]s. Ferraris experimented with different types of asynchronous ]s. The research and his studies resulted in the development of an ], which may be thought of as an alternating-current motor operating in reverse, so as to convert mechanical (rotating) power into electric power (as alternating current). On 11 March 1888, Ferraris published his research in a paper to the Royal Academy of Sciences in ].<ref>{{Cite web |date=2022-06-14 |title=Milestones:Rotating Fields and Early Induction Motors, 1885-1888 |url=https://ethw.org/Milestones:Rotating_Fields_and_Early_Induction_Motors,_1885-1888 |access-date=2025-01-01 |website=ETHW |language=en}}</ref> | |||
| Two months later Nikola Tesla gained {{US patent|381968}} for a three-phase electric motor design, application filed October 12, 1887. Figure 13 of this patent shows that Tesla envisaged his three-phase motor being powered from the generator via six wires. | |||
| Three-phase supplies have properties that make them very desirable in electric power distribution systems: | |||
| *The phase currents tend to cancel out one another, summing to zero in the case of a linear balanced load. This makes it possible to reduce the size of the neutral conductor because it carries little or no current. With a balanced load, all the phase conductors carry the same current and so can be the same size. | |||
| *Power transfer into a linear balanced load is constant, which helps to reduce generator and motor vibrations. | |||
| *Three-phase systems can produce a ] with a specified direction and constant magnitude, which simplifies the design of electric motors. | |||
| These alternators operated by creating systems of alternating currents displaced from one another in phase by definite amounts, and depended on rotating magnetic fields for their operation. The resulting source of polyphase power soon found widespread acceptance. The invention of the polyphase alternator is key in the history of electrification, as is the power transformer. These inventions enabled power to be transmitted by wires economically over considerable distances. Polyphase power enabled the use of water-power (via hydroelectric generating plants in large dams) in remote places, thereby allowing the mechanical energy of the falling water to be converted to electricity, which then could be fed to an electric motor at any location where mechanical work needed to be done. This versatility sparked the growth of power-transmission network grids on continents around the globe. | |||
| Most household loads are single-phase. In North American residences, three-phase power might feed a multiple-unit apartment block, but the household loads are connected only as single phase. <!--you might have 120-208 4 wire with lights and plugs on 120 and the stove and AC on 208 V single phase...so two phase wires come into each unit, but the loads are all single phase...do we need to explain that here in such detail?--> In lower-density areas, only a single phase might be used for distribution. Some large European appliances may be powered by three-phase power, such as electric stoves and clothes dryers. | |||
| Mikhail Dolivo-Dobrovolsky developed a three-phase ] and a three-phase electric motor in 1888 and studied ]s. <ref>{{Cite patent|number=US422746A|title=Dobrowolsky|gdate=1890-03-04|url=https://patents.google.com/patent/US422746}}</ref> <ref>{{Cite web |title=US Patent: 422,746 - Electric induction apparatus or transformer |url=https://www.datamp.org/patents/search/advance.php?pn=422746&id=73585&set=1 |access-date=2025-01-01 |website=www.datamp.org}}</ref> <ref>{{Cite patent|number=US455683A|title=Dobeowolsky|gdate=1891-07-07|url=https://patents.google.com/patent/US455683}}</ref> His three-phase three-wire transmission system was displayed in 1891 in Germany at the ], where Dolivo-Dobrovolsky used the system to transmit electric power at the distance of 176 km (110 miles) with 75% ]. In 1891 he also created a three-phase transformer and short-circuited (]) ].<ref>{{Cite patent|number=US456804A|title=Dobrowolsky|gdate=1891-07-28|url=https://patents.google.com/patent/US456804}}</ref> <ref>{{Cite web|url=http://www.mpoweruk.com/history.htm|title=Wayback Machine|website=www.mpoweruk.com}}</ref><ref>Gerhard Neidhöfer: ''Michael von Dolivo-Dobrowolsky und der Drehstrom. Geschichte der Elektrotechnik'' VDE-Buchreihe, Volume 9, VDE VERLAG, Berlin Offenbach, {{ISBN|978-3-8007-3115-2}}.''</ref> He designed the world's first three-phase ] in 1891.<ref>{{Cite web |title=The Earliest Years of Three-Phase Power—1891–1893 |url=https://ieeexplore.ieee.org/document/8944322 |archive-url=http://web.archive.org/web/20241211105501/https://ieeexplore.ieee.org/document/8944322/ |archive-date=2024-12-11 |access-date=2025-01-01 |website=ieeexplore.ieee.org |language=en-US}}</ref> Inventor ] received in ] a Swedish patent on the same three-phase system.<ref>{{cite book|first=Lars|isbn=91-7536-330-5|last=Bergström och Nordlund|pages=283|publisher=Naturaläromedel|title=Ellära- Kretsteknik och fältteori|year=2002 }}<!-- auto-translated by Module:CS1 translator --></ref> The possibility of transferring electrical power from a waterfall at a distance was explored at the ] mine. A {{val|45|ul=m}} fall at Hällsjön, Smedjebackens kommun, where a small iron work had been located, was selected. In 1893, a three-phase {{val|9.5|ul=Kilovolt{{!}}kV}} system was used to transfer {{convert|400|hp|kW|link=in}} a distance of 15 km (10 miles), becoming the first commercial application.<ref>{{cite book | last= Hjulström | first= Filip | title= Elektrifieringens utveckling i Sverige, en ekonomisk-geografisk översikt | year= 1940 | url= https://www.antikvariat.net/sv/rod151504-elektrifieringens-utveckling-i-sverige-en-ekonomisk-geografisk-oversikt-hjulstrom-filip | trans-quote= Excerpt taken from YMER 1941, häfte 2.Utgiven av Sällskapet för antropologi och geografi: Meddelande från Upsala univeristets geografiska institution, N:o 29, published by Esselte ab, Stockholm 1941 no. 135205 }}</ref> | |||
| Wiring for the three phases is typically identified by color codes which vary by country. Connection of the phases in the right order is required to ensure the intended direction of rotation of three-phase motors. For example, pumps and fans may not work in reverse. Maintaining the identity of phases is required if there is any possibility two sources can be connected at the same time; a direct interconnection between two different phases is a short-circuit. | |||
| == Principle == | |||
| == Generation and distribution == | |||
| ]s of the instantaneous voltages in a three-phase system in one cycle with time increasing to the right. The phase order is 1–2–3. This sequence repeats each cycle, and thus the ] of the generator sets the ] of the power system. Ideally, each phase's ], current, and power is offset from the others' by 120°, thus having all at equidistance. This symmetry can also be recreated in converters.]] | |||
| ] | |||
| {{double image|right|Hawkins Electrical Guide - 3phase Elementary 6wire.jpg|250|Hawkins Electrical Guide - 3phase Elementary 3wire.jpg|100|Left: Elementary six-wire three-phase alternator, with each phase using a separate pair of transmission wires.<ref>'']'', Theo. Audel and Co., 2nd ed., 1917, vol. 4, Ch. 46: Alternating Currents, p. 1026, fig. 1260.</ref> Right: Elementary three-wire three-phase alternator, showing how the phases can share only three wires.<ref>'']'', Theo. Audel and Co., 2nd ed., 1917, vol. 4, Ch. 46: Alternating Currents, p. 1026, fig. 1261.</ref> | |||
| }} | |||
| ] | |||
| At the ], an ] converts mechanical power into a set of three ] ]s, one from each coil (or winding) of the generator. The windings are arranged such that the currents vary ] at the same frequency but with the peaks and troughs of their ] offset to provide three complementary currents with a phase separation of one-third cycle (]). The ] is typically 50 or 60 ], varying by country. | |||
| {{further2|]}} | |||
| ] | |||
| At the power station, ]s change the voltage from generators to a level suitable for ] minimizing losses. | |||
| In a symmetric three-phase power supply system, three conductors each carry an ] of the same frequency and voltage amplitude relative to a common reference, but with a phase difference of one third of a cycle (i.e., 120 degrees out of phase) between each. The common reference is usually connected to ground and often to a current-carrying conductor called the neutral. Due to the phase difference, the ] on any conductor reaches its peak at one third of a cycle after one of the other conductors and one third of a cycle before the remaining conductor. This phase delay gives constant power transfer to a balanced linear load. It also makes it possible to produce a rotating magnetic field in an ] and generate other phase arrangements using transformers (for instance, a two-phase system using a ]). The amplitude of the voltage difference between two phases is <math>\sqrt{3} = 1.732\ldots</math> times the amplitude of the voltage of the individual phases. | |||
| After further voltage conversions in the transmission network, the voltage is finally transformed to the standard utilization before power is supplied to customers. | |||
| The symmetric three-phase systems described here are simply referred to as ''three-phase systems'' because, although it is possible to design and implement asymmetric three-phase power systems (i.e., with unequal voltages or phase shifts), they are not used in practice because they lack the most important advantages of symmetric systems. | |||
| Most automotive alternators generate three phase AC and rectify it to DC with a ].<ref>http://www.rle.mit.edu/per/conferencepapers/cpconvergence00p583.pdf</ref> | |||
| In a three-phase system feeding a balanced and linear load, the sum of the instantaneous currents of the three conductors is zero. In other words, the current in each conductor is equal in magnitude to the sum of the currents in the other two, but with the opposite sign. The return path for the current in any phase conductor is the other two phase conductors. | |||
| == Transformer connections == | |||
| A "delta" connected transformer winding is connected between phases of a three-phase system. A "wye" ("star") transformer connects each winding from a phase wire to a common neutral point. | |||
| Constant power transfer is possible with any number of phases greater than one. However, two-phase systems do not have neutral-current cancellation and thus use conductors less efficiently, and more than three phases complicates infrastructure unnecessarily. | |||
| In an "open delta" or "V" system, only two transformers are used. A closed delta system can operate as an open delta if one of the transformers has failed or needs to be removed.<ref name="Fowler"/> In open delta, each transformer must carry current for its respective phases as well as current for the third phase, therefore capacity is reduced to 87%. With one of three transformers missing and the remaining two at 87% efficiency, the capacity is 58% ({{math|(2/3) × 87%}}).<ref name=power>{{cite journal|last=McGraw-Hill|journal=Power|year=1920|volume=51|issue=17|url=http://books.google.com/books?id=u91QAAAAYAAJ&pg=PA673&lpg=PA673|accessdate=21 December 2012}}</ref><ref>H. W. Beaty, D.G.Fink (ed) ''Standard Handbook for Electrical Engineers Fifteenth Edition'',McGraw-Hill, 2007 ISBN 0-07-144146-8, p. 10–11</ref> | |||
| Additionally, in some practical generators and motors, | |||
| two phases can result in a less smooth (pulsating) torque. <ref>{{Cite book|last=von Meier|first=Alexandra|title=Electric Power Systems|publisher=John Wiley & Sons, Inc.|year=2006|isbn=978-0-471-17859-0|location=Hoboken, New Jersey|pages=160|quote=We also stated one rationale for this three-phase system; namely, that a three-phase generator experiences a constant torque on its rotor as opposed to the pulsating torque that appears in a single- or two-phase machine, which is obviously preferable from a mechanical engineering standpoint.}}</ref> | |||
| Three-phase systems may have a fourth wire, common in low-voltage distribution. This is the ] wire. The neutral allows three separate single-phase supplies to be provided at a constant voltage and is commonly used for supplying multiple ] loads. The connections are arranged so that, as far as possible in each group, equal power is drawn from each phase. Further up the ], the currents are usually well balanced. Transformers may be wired to have a four-wire secondary and a three-wire primary, while allowing unbalanced loads and the associated secondary-side neutral currents. | |||
| Where a delta-fed system must be grounded for detection of stray current to ground or protection from surge voltages, a grounding transformer (usually a ]) may be connected to allow ground fault currents to return from any phase to ground. Another variation is a "corner grounded" delta system, which is a closed delta that is grounded at one of the junctions of transformers.<ref></ref> | |||
| === Phase sequence === | |||
| == Three-wire and four-wire circuits == | |||
| {{see also|Electric machine#Sequence}} | |||
| ] | |||
| Wiring for three phases is typically identified by colors that vary by country and voltage. The phases must be connected in the correct order to achieve the intended direction of rotation of three-phase motors. For example, pumps and fans do not work as intended in reverse. Maintaining the identity of phases is required if two sources could be connected at the same time. A direct connection between two different phases is a ] and leads to flow of unbalanced current. | |||
| ] | |||
| == Advantages and disadvantages == | |||
| There are two basic three-phase configurations: delta and wye (star). As shown on the left, a delta configuration requires only 3 wires for transmission but a wye (star) configuration may utilise a fourth wire. The fourth wire, if present, is provided as a Neutral and is normally Grounded. The "3-wire" and "4-wire" designations do not count the ] used above many transmission lines, which is solely for fault protection and does not carry current under non-fault conditions. | |||
| As compared to a single-phase AC power supply that uses two current-carrying conductors (phase and ]), a three-phase supply with no neutral and the same phase-to-ground voltage and current capacity per phase can transmit three times as much power by using just 1.5 times as many wires (i.e., three instead of two). Thus, the ratio of capacity to conductor material is doubled.<ref>Cotton, H, ''Electrical Technology'', 6th ed., Pitman, London, 1950, p. 268.</ref> The ratio of capacity to conductor material increases to 3:1 with an ungrounded three-phase and center-grounded single-phase system (or 2.25:1 if both use grounds with the same gauge as the conductors). That leads to higher efficiency, lower weight, and cleaner waveforms. | |||
| Three-phase supplies have properties that make them desirable in electric power distribution systems: | |||
| A four-wire system with symmetrical voltages between phase and neutral is obtained when the neutral is connected to the "common star point" of all supply windings. In such a system, all three phases will have the same magnitude of voltage relative to the Neutral. Other non-symmetrical systems have been used. | |||
| * The phase currents tend to cancel out one another, summing to zero in the case of a linear balanced load, which allows a reduction of the size of the neutral conductor because it carries little or no current. With a balanced load, all the phase conductors carry the same current and so can have the same size. | |||
| * Power transfer into a linear balanced load is constant, which, in motor/generator applications, helps to reduce vibrations. | |||
| * Three-phase systems can produce a ] with a specified direction and constant magnitude, which simplifies the design of electric motors, as no starting circuit is required. | |||
| However, most loads are single-phase. In North America, single-family houses and individual apartments are supplied one phase from the power grid and use a ] to the ] from which most branch circuits will carry 120 V. Circuits designed for higher powered devices such as stoves, dryers, or outlets for electric vehicles carry 240 V. | |||
| The four-wire wye system is used when ground referenced voltages or the flexibility of more voltage selections are required. Faults on one phase to ground will cause a protection event (fuse or breaker open) locally and not involve other phases or other connected equipment.{{citation needed|date=December 2012}} An example of application is local distribution in Europe (and elsewhere), where each customer may be only fed from one phase and the neutral (which is common to the three phases). When a group of customers sharing the neutral draw unequal phase currents, the common neutral wire carries the currents resulting from these imbalances. Electrical engineers try to design the system so the loads are balanced as much as possible within premises where 3-phase power is utilized.<ref>http://www.rapid-tech.com.au/Fluke-2_Saving%20energy%20through%20load%20balancing.pdf</ref> These same principles apply to the wide scale distribution of power to individual premises. Hence, every effort is made by supply authorities to distribute all three phases over a large number of premises so that, on average, as nearly as possible a balanced load is seen at the point of supply. | |||
| For domestic use, some countries such as the UK may supply one phase and neutral at a high current (up to 100A) to one property, while others such as Germany may supply 3 phases and neutral to each customer, but at a lower fuse rating, typically 32 A per phase, and "shuffled" to avoid the effect that more load tends to be put on the first phase. | |||
| In Europe, three-phase power is normally delivered to the panelboard and further to higher powered devices. | |||
| In North America, a ] supply is sometimes used, where one winding of a delta connected transformer feeding the load is center-tapped and that center tap is grounded and connected as a Neutral, as shown on the right. This setup produces three different voltages. If the voltage between the center tap (neutral) and each of the two adjacent phases is 120 V (100%), the voltage across any two phases is 240 V (200%), and the neutral to "high leg" voltage is ≈ 208 V (173%).<ref name="Fowler"/> | |||
| == Generation and distribution == | |||
| The reason for providing the delta connected supply is usually to power large motors requiring a rotating field. However, the premises concerned will also require the "normal" North American 120 V supplies, two of which are derived (180 degrees "out of phase") between the "Neutral" and either of the center tapped phase points. | |||
| {{See also|Mains electricity by country}} | |||
| ] | |||
| == Balanced circuits == | |||
| {{multiple image | |||
| In the perfectly balanced case all three lines share equivalent loads. Examining the circuits we can derive relationships between line voltage and current, and load voltage and current for wye and delta connected loads. | |||
| | align = right | |||
| | image1 = Hawkins Electrical Guide - 3phase Elementary 6wire.jpg | |||
| | width1 = 250 | |||
| | alt1 = | |||
| | caption1 = | |||
| | image2 = Hawkins Electrical Guide - 3phase Elementary 3wire.jpg | |||
| | width2 = 110 | |||
| | alt2 = | |||
| | caption2 = | |||
| | footer = Left image: elementary six-wire three-phase alternator with each phase using a separate pair of transmission wires.<ref>'']'', Theo. Audel and Co., 2nd ed., 1917, vol. 4, Ch. 46: Alternating Currents, p. 1026, fig. 1260.</ref> Right image: elementary three-wire three-phase alternator showing how the phases can share only three wires.<ref>'']'', Theo. Audel and Co., 2nd ed., 1917, vol. 4, Ch. 46: Alternating Currents, p. 1026, fig. 1261.</ref> | |||
| }} | |||
| At the ], an ] converts mechanical power into a set of three ] ]s, one from each coil (or winding) of the generator. The windings are arranged such that the currents are at the same frequency but with the peaks and troughs of their ] forms offset to provide three complementary currents with a phase separation of one-third cycle (]). The ] is typically 50 or 60 ], depending on the country. | |||
| In a balanced system each line will produce equal voltage magnitudes at phase angles equally spaced from each other. With V<sub>1</sub> as our reference and V<sub>3</sub> lagging V<sub>2</sub> lagging V<sub>1</sub>, using ], we have:<ref name="GloverSarma2011">{{cite book|author1=J. Duncan Glover|author2=Mulukutla S. Sarma|author3=Thomas J. Overbye|title=Power System Analysis & Design|url=http://books.google.com/books?id=HrtXToLEbVoC|date=April 2011|publisher=Cengage Learning|isbn=978-1-111-42579-1|pages=60–68}}</ref> | |||
| At the power station, transformers change the voltage from generators to a level suitable for ] in order to minimize losses. | |||
| :<math>V_1 = V_\text{LN}\angle 0^\circ,</math> | |||
| After further voltage conversions in the transmission network, the voltage is finally transformed to the standard utilization before power is supplied to customers. | |||
| :<math>V_2 = V_\text{LN}\angle{-120}^\circ,</math> | |||
| Most automotive alternators generate three-phase AC and rectify it to DC with a ].<ref>{{Cite web|url=http://www.rle.mit.edu/per/ConferencePapers/cpConvergence00p583.pdf|title=A New Design for Automotive Alternators|date=August 30, 2017|archive-url=https://web.archive.org/web/20170830033252/http://www.rle.mit.edu/per/ConferencePapers/cpConvergence00p583.pdf|archive-date=2017-08-30}}</ref> | |||
| :<math>V_3 = V_\text{LN}\angle{+120}^\circ.</math> | |||
| == Transformer connections == | |||
| These voltages feed into either a wye or delta connected load. | |||
| A "delta" (Δ) connected transformer winding is connected between phases of a three-phase system. A "wye" (Y) transformer connects each winding from a phase wire to a common neutral point. | |||
| A single three-phase transformer can be used, or three single-phase transformers. | |||
| === Wye === | |||
| In an "open delta" or "V" system, only two transformers are used. A closed delta made of three single-phase transformers can operate as an open delta if one of the transformers has failed or needs to be removed.<ref name="Fowler" /> In open delta, each transformer must carry current for its respective phases as well as current for the third phase, therefore capacity is reduced to 87%. With one of three transformers missing and the remaining two at 87% efficiency, the capacity is 58% ({{frac|2|3}} of 87%).<ref name=power>{{cite journal |first=J. B. |last=Gibbs |title=Three-Phase Power from Single-Phase Transformer Connections |journal=Power |publisher=McGraw-Hill |date=April 27, 1920 |volume=51 |issue=17 |page=673 |url=https://books.google.com/books?id=u91QAAAAYAAJ&pg=PA68-IA21 |access-date=21 December 2012}}</ref><ref>H. W. Beaty, D. G. Fink (ed.), ''Standard Handbook for Electrical Engineers''. 15th ed., McGraw-Hill, 2007, {{ISBN|0-07-144146-8}}, pp. 10–11.</ref> | |||
| ] | |||
| Where a delta-fed system must be grounded for detection of stray current to ground or protection from surge voltages, a grounding transformer (usually a ]) may be connected to allow ground fault currents to return from any phase to ground. Another variation is a "corner grounded" delta system, which is a closed delta that is grounded at one of the junctions of transformers.<ref>{{Cite web|url=http://static.schneider-electric.us/docs/Circuit%20Protection/Molded%20Case%20Circuit%20Breakers/2700DB0202.pdf |archive-url=https://ghostarchive.org/archive/20221009/http://static.schneider-electric.us/docs/Circuit%20Protection/Molded%20Case%20Circuit%20Breakers/2700DB0202.pdf |archive-date=2022-10-09 |url-status=live|title=Schneider}}</ref> | |||
| For the wye case, all loads see their respective line voltages, and so:<ref name = "GloverSarma2011" /> | |||
| == Three-wire and four-wire circuits == | |||
| :<math> I_1 = \frac{V_1}{|Z_\text{total}|}\angle (-\theta),</math> | |||
| ] | |||
| There are two basic three-phase configurations: wye (Y) and delta (Δ). As shown in the diagram, a delta configuration requires only three wires for transmission, but a wye (star) configuration may have a fourth wire. The fourth wire, if present, is provided as a neutral and is normally grounded. The three-wire and four-wire designations do not count the ] present above many transmission lines, which is solely for fault protection and does not carry current under normal use. | |||
| :<math> I_2 = \frac{V_2}{|Z_\text{total}|}\angle (-120^\circ - \theta),</math> | |||
| A four-wire system with symmetrical voltages between phase and neutral is obtained when the neutral is connected to the "common star point" of all supply windings. In such a system, all three phases will have the same magnitude of voltage relative to the neutral. Other non-symmetrical systems have been used. | |||
| :<math> I_3 = \frac{V_3}{|Z_\text{total}|}\angle (120^\circ - \theta),</math> | |||
| The four-wire wye system is used when a mixture of single-phase and three-phase loads are to be served, such as mixed lighting and motor loads. An example of application is local distribution in Europe (and elsewhere), where each customer may be only fed from one phase and the neutral (which is common to the three phases). When a group of customers sharing the neutral draw unequal phase currents, the common neutral wire carries the currents resulting from these imbalances. Electrical engineers try to design the three-phase power system for any one location so that the power drawn from each of three phases is the same, as far as possible at that site.<ref>{{cite web |url=http://www.rapid-tech.com.au/Fluke-2_Saving%20energy%20through%20load%20balancing.pdf |title=Saving energy through load balancing and load scheduling |access-date=2014-08-03 |url-status=dead |archive-url=https://web.archive.org/web/20140911172708/http://www.rapid-tech.com.au/Fluke-2_Saving%20energy%20through%20load%20balancing.pdf |archive-date=2014-09-11 }}</ref> Electrical engineers also try to arrange the distribution network so the loads are balanced as much as possible, since the same principles that apply to individual premises also apply to the wide-scale distribution system power. Hence, every effort is made by supply authorities to distribute the power drawn on each of the three phases over a large number of premises so that, on average, as nearly as possible a balanced load is seen at the point of supply. | |||
| where ''Z''<sub>total</sub> is the sum of line and load impedances (''Z''<sub>total</sub> = ''Z''<sub>LN</sub> + ''Z''<sub>Y</sub>), and ''θ'' is the phase of the total impedance (''Z''<sub>total</sub>). | |||
| ] | |||
| For domestic use, some countries such as the ] may supply one phase and neutral at a high current (up to 100 ]) to one property, while others such as ] may supply 3 phases and neutral to each customer, but at a lower fuse rating, typically 40–63 A per phase, and "rotated" to avoid the effect that more load tends to be put on the first phase.{{citation needed|date=September 2016}} | |||
| ]" system used for mixed single-phase and three-phase loads on the same distribution system. Three-phase loads such as motors connect to L1, L2, and L3. Single-phase loads would be connected between L1 or L2 and neutral, or between L1 and L2. The L3 phase is 1.73 times the L1 or L2 voltage to neutral, so this leg is not used for single-phase loads.]] | |||
| The phase angle difference between voltage and current of each phase is not necessarily 0 and is dependent on the type of load impedance, ''Z''<sub>y</sub>. Inductive and capacitive loads will cause current to either lag or lead the voltage. However, the relative phase angle between each pair of lines (1 to 2, 2 to 3,and 3 to 1) will still be −120°. | |||
| Based on wye (Y) and delta (Δ) connection. Generally, there are four different types of three-phase transformer winding connections for transmission and distribution purposes: | |||
| By performing ] on the neutral node, the three phase currents sum up to the total current in the neutral line. In the balanced case: | |||
| * wye (Y) – wye (Y) is used for small current and high voltage, | |||
| * Delta (Δ) – Delta (Δ) is used for large currents and low voltages, | |||
| * Delta (Δ) – wye (Y) is used for step-up transformers, i.e., at generating stations, | |||
| * wye (Y) – Delta (Δ) is used for step-down transformers, i.e., at the end of the transmission. | |||
| In North America, a ] supply is sometimes used where one winding of a delta-connected transformer feeding the load is center-tapped and that center tap is grounded and connected as a neutral as shown in the second diagram. This setup produces three different voltages: If the voltage between the center tap (neutral) and each of the top and bottom taps (phase and anti-phase) is 120 ] (100%), the voltage across the phase and anti-phase lines is 240 V (200%), and the neutral to "high leg" voltage is ≈ 208 V (173%).<ref name="Fowler" /> | |||
| :<math> I_1 + I_2 + I_3 = I_\text{N} = 0.</math> | |||
| The reason for providing the delta connected supply is usually to power large motors requiring a rotating field. However, the premises concerned will also require the "normal" North American 120 V supplies, two of which are derived (180 degrees "out of phase") between the "neutral" and either of the center-tapped phase points. | |||
| === Delta === | |||
| == Balanced circuits == | |||
| ] | |||
| In the perfectly balanced case all three lines share equivalent loads. Examining the circuits, we can derive relationships between line voltage and current, and load voltage and current for wye- and delta-connected loads. | |||
| In a balanced system each line will produce equal voltage magnitudes at phase angles equally spaced from each other. With V<sub>1</sub> as our reference and V<sub>3</sub> lagging V<sub>2</sub> lagging V<sub>1</sub>, using ], and V<sub>LN</sub> the voltage between the line and the neutral we have:<ref name="GloverSarma2011">{{cite book |author1=J. Duncan Glover |author2=Mulukutla S. Sarma |author3=Thomas J. Overbye |title=Power System Analysis & Design |url=https://books.google.com/books?id=HrtXToLEbVoC |date=April 2011 |publisher=Cengage Learning |isbn=978-1-111-42579-1 |pages=60–68}}</ref> | |||
| In the delta circuit, loads are connected across the lines, and so loads see line-to-line voltages:<ref name = "GloverSarma2011" /> | |||
| :<math>\begin{align} | :<math>\begin{align} | ||
| V_1 &= V_\text{LN}\angle 0^\circ, \\ | |||
| V_2 &= V_\text{LN}\angle{-120}^\circ, \\ | |||
| |
V_3 &= V_\text{LN}\angle{+120}^\circ. | ||
| \end{align}</math> | |||
| These voltages feed into either a wye- or delta-connected load. | |||
| V_{23} &= V_2 - V_3 = (V_\text{LN}\angle {-120}^\circ) - (V_\text{LN}\angle 120^\circ) \\ | |||
| &= \sqrt{3}V_\text{LN}\angle {-90}^\circ = \sqrt{3}V_{2}\angle (\phi_{V_2} + 30^\circ), \\ | |||
| ===Wye (or, star; Y)=== | |||
| V_{31} &= V_3 - V_1 = (V_\text{LN}\angle 120^\circ) - (V_\text{LN}\angle 0^\circ) \\ | |||
| ].<ref>{{cite web |title=What is "Stray Voltage"? |date=August 10, 2015 |publisher=Utility Technology Engineers-Consultants (UTEC) |url=https://www.utilitytec.com/uploads/1/3/0/9/130926103/2015_stray_voltage_analysis.pdf |access-date=December 10, 2023}}</ref>]] | |||
| &= \sqrt{3}V_\text{LN}\angle 150^\circ = \sqrt{3}V_{3}\angle (\phi_{V_3} + 30^\circ). \\ | |||
| The voltage seen by the load will depend on the load connection; for the wye case, connecting each load to a phase (line-to-neutral) voltages gives<ref name="GloverSarma2011" /> | |||
| :<math>\begin{align} | |||
| I_1 &= \frac{V_1}{|Z_\text{total}|}\angle (-\theta), \\ | |||
| I_2 &= \frac{V_2}{|Z_\text{total}|}\angle (-120^\circ - \theta), \\ | |||
| I_3 &= \frac{V_3}{|Z_\text{total}|}\angle ( 120^\circ - \theta), | |||
| \end{align}</math> | \end{align}</math> | ||
| where ''Z''<sub>total</sub> is the sum of line and load impedances (''Z''<sub>total</sub> = ''Z''<sub>LN</sub> + ''Z''<sub>Y</sub>), and ''θ'' is the phase of the total impedance (''Z''<sub>total</sub>). | |||
| Further: | |||
| The phase angle difference between voltage and current of each phase is not necessarily 0 and depends on the type of load impedance, ''Z''<sub>y</sub>. Inductive and capacitive loads will cause current to either lag or lead the voltage. However, the relative phase angle between each pair of lines (1 to 2, 2 to 3, and 3 to 1) will still be −120°. | |||
| :<math> I_{12} = \frac{V_{12}}{|Z_\Delta|} \angle (30^\circ - \theta), </math> | |||
| [[File:Wye connection line voltages.png|thumb|A phasor diagram for a wye configuration, in which ''V''<sub>ab</sub> represents a line voltage, and ''V''<sub>an</sub> represents a phase voltage. Voltages are balanced as{{ubl | |||
| :<math> I_{23} = \frac{V_{23}}{|Z_\Delta|} \angle (-90^\circ - \theta), </math> | |||
| | ''V''<sub>ab</sub> {{=}} (1∠α − 1∠α + 120°) {{sqrt|3}}{{nnbsp}}|''V''|∠α + 30°, | |||
| | ''V''<sub>bc</sub> {{=}} {{sqrt|3}}{{nnbsp}}|''V''|∠α − 90°, | |||
| | ''V''<sub>ca</sub> {{=}} {{sqrt|3}}{{nnbsp}}|''V''|∠α + 150° | |||
| }}{{paragraph}} | |||
| (α = 0 in this case). | |||
| ]] | |||
| By applying ] (KCL) to the neutral node, the three phase currents sum to the total current in the neutral line. In the balanced case: | |||
| : <math>I_1 + I_2 + I_3 = I_\text{N} = 0.</math> | |||
| === Delta (Δ) === | |||
| :<math> I_{31} = \frac{V_{31}}{|Z_\Delta|} \angle (150^\circ - \theta), </math> | |||
| ] | |||
| In the delta circuit, loads are connected across the lines, and so loads see line-to-line voltages:<ref name="GloverSarma2011" /> | |||
| where ''θ'' is the phase of delta impedance (''Z''<sub>Δ</sub>). | |||
| : <math>\begin{align} | |||
| V_{12} &= V_1 - V_2 = (V_\text{LN}\angle 0^\circ) - (V_\text{LN}\angle {-120}^\circ) \\ | |||
| &= \sqrt{3}V_\text{LN}\angle 30^\circ = \sqrt{3}V_{1}\angle (\phi_{V_1} + 30^\circ), \\ | |||
| V_{23} &= V_2 - V_3 = (V_\text{LN}\angle {-120}^\circ) - (V_\text{LN}\angle 120^\circ) \\ | |||
| Relative angles are preserved, so ''I''<sub>31</sub> lags ''I''<sub>23</sub> lags ''I''<sub>12</sub> by 120°. Calculating line currents by using KCL at each delta node gives: | |||
| &= \sqrt{3}V_\text{LN}\angle {-90}^\circ = \sqrt{3}V_{2}\angle (\phi_{V_2} + 30^\circ), \\ | |||
| V_{31} &= V_3 - V_1 = (V_\text{LN}\angle 120^\circ) - (V_\text{LN}\angle 0^\circ) \\ | |||
| &= \sqrt{3}V_\text{LN}\angle 150^\circ = \sqrt{3}V_{3}\angle (\phi_{V_3} + 30^\circ). | |||
| \end{align}</math> | |||
| (Φ<sub>v1</sub> is the phase shift for the first voltage, commonly taken to be 0°; in this case, Φ<sub>v2</sub> = −120° and Φ<sub>v3</sub> = −240° or 120°.) | |||
| Further: | |||
| :<math>\begin{align} | :<math>\begin{align} | ||
| I_{12} &= \frac{V_{12}}{|Z_\Delta|} \angle ( 30^\circ - \theta), \\ | |||
| |
I_{23} &= \frac{V_{23}}{|Z_\Delta|} \angle (-90^\circ - \theta), \\ | ||
| I_{31} &= \frac{V_{31}}{|Z_\Delta|} \angle ( 150^\circ - \theta), | |||
| \end{align}</math> | |||
| where ''θ'' is the phase of delta impedance (''Z''<sub>Δ</sub>). | |||
| Relative angles are preserved, so ''I''<sub>31</sub> lags ''I''<sub>23</sub> lags ''I''<sub>12</sub> by 120°. Calculating line currents by using KCL at each delta node gives | |||
| :<math>\begin{align} | |||
| I_1 &= I_{12} - I_{31} = I_{12} - I_{12}\angle 120^\circ \\ | |||
| &= \sqrt{3}I_{12} \angle (\phi_{I_{12}} - 30^\circ) = \sqrt{3}I_{12} \angle (-\theta) | |||
| \end{align}</math> | \end{align}</math> | ||
| and similarly for each other line: | and similarly for each other line: | ||
| : <math>\begin{align} | |||
| :<math> I_2 = \sqrt{3}I_{23} \angle (\phi_{I_{23}}-30^\circ) = \sqrt{3}I_{23} \angle (-120^\circ - \theta), </math> | |||
| I_2 &= \sqrt{3}I_{23} \angle (\phi_{I_{23}} - 30^\circ) = \sqrt{3}I_{23} \angle (-120^\circ - \theta), \\ | |||
| I_3 &= \sqrt{3}I_{31} \angle (\phi_{I_{31}} - 30^\circ) = \sqrt{3}I_{31} \angle (120^\circ - \theta), | |||
| \end{align}</math> | |||
| where, again, ''θ'' is the phase of delta impedance (''Z''<sub>Δ</sub>). | |||
| :<math> I_3 = \sqrt{3}I_{31} \angle (\phi_{I_{31}}-30^\circ) = \sqrt{3}I_{31} \angle (120^\circ - \theta), </math> | |||
| [[File:Delta connection currents.png|thumb|A delta configuration and a corresponding phasor diagram of its currents. Phase voltages are equal to line voltages, and currents are calculated as{{ubl | |||
| where, again, ''θ'' is the phase of delta impedance (''Z''<sub>Δ</sub>). | |||
| | ''I''<sub>a</sub> {{=}} ''I''<sub>ab</sub> − ''I''<sub>ca</sub> {{=}} {{sqrt|3}}{{nnbsp}}''I''<sub>ab</sub>∠−30°, | |||
| | ''I''<sub>b</sub> {{=}} ''I''<sub>bc</sub> − ''I''<sub>ab</sub>, | |||
| | ''I''<sub>c</sub> {{=}} ''I''<sub>ca</sub> − ''I''<sub>bc</sub>. | |||
| }}{{paragraph}} | |||
| The overall power transferred is | |||
| {{ubl|''S''<sub>3Φ</sub> {{=}} 3''V''<sub>phase</sub>''I''*<sub>phase</sub>.}} | |||
| ]] | |||
| Inspection of a phasor diagram, or conversion from phasor notation to complex notation, illuminates how the difference between two line-to-neutral voltages yields a line-to-line voltage that is greater by a factor of {{sqrt|3}}. As a delta configuration connects a load across phases of a transformer, it delivers the line-to-line voltage difference, which is {{sqrt|3}} times greater than the line-to-neutral voltage delivered to a load in the wye configuration. As the power transferred is ''V''<sup>2</sup>/''Z'', the impedance in the delta configuration must be 3 times what it would be in a wye configuration for the same power to be transferred. | |||
| == Single-phase loads == | == Single-phase loads == | ||
| Except in a ] system and a corner-grounded delta system, single-phase loads may be connected across any two phases, or a load can be connected from phase to neutral.<ref name="IAEI" /> Distributing single-phase loads among the phases of a three-phase system balances the load and makes most economical use of conductors and transformers. | |||
| In a symmetrical three-phase four-wire wye system, the three phase conductors have the same voltage to the system neutral. The voltage between line conductors is ] times the phase conductor to neutral voltage:<ref>The boy electrician by J. W. Sims M.I.E.E. (p. 98).</ref> | |||
| Single-phase loads may be connected across any two phases, or a load can be connected from phase to neutral.<ref name="IAEI"/> Distributing single-phase loads among the phases of a three-phase system balances the load and makes most economical use of conductors and transformers. | |||
| In a symmetrical three-phase four-wire, wye system, the three phase conductors have the same voltage to the system neutral. The voltage between line conductors is ] times the phase conductor to neutral voltage:<ref>The boy electrician by J W Sims M.I.E.E. (Page 98)</ref> | |||
| :<math>V_\text{LL} = \sqrt{3} V_\text{LN}.</math> | :<math>V_\text{LL} = \sqrt{3} V_\text{LN}.</math> | ||
| The currents returning from the customers' premises to the supply transformer all share the neutral wire. If the loads are evenly distributed on all three phases, the sum of the returning currents in the neutral wire is approximately zero. Any unbalanced phase loading on the secondary side of the transformer will use the transformer capacity inefficiently. | |||
| The currents returning from the customers' premises to the supply transformer all share the neutral wire. If the loads are evenly distributed on all three phases, the sum of the returning currents in the neutral wire is approximately zero. Any unbalanced phase loading on the secondary side of the transformer will use the transformer capacity inefficiently. | |||
| If the supply neutral is broken, phase-to-neutral voltage is no longer maintained. Phases with higher relative loading will experience reduced voltage, and phases with lower relative loading will experience elevated voltage, up to the phase-to-phase voltage.{{citation needed|date=December 2012}} | |||
| If the supply neutral is broken, phase-to-neutral voltage is no longer maintained. Phases with higher relative loading will experience reduced voltage, and phases with lower relative loading will experience elevated voltage, up to the phase-to-phase voltage. | |||
| A ] provides phase-to-neutral relationship of {{math|1=''V''<sub>LL</sub> = 2 ''V''<sub>LN</sub> }}, however, LN load is imposed on one phase.<ref name=Fowler>{{cite book|last=Fowler|first=Nick|title=Electrician's Calculations Manual 2nd Edition|year=2011|publisher=McGraw-Hill|isbn=9780071770170|pages=3–5|url=http://books.google.com/books?id=9QBWHJdPGM0C}}</ref> A transformer manufacturer's page suggests that LN loading to not exceed 5% of transformer capacity.<ref></ref> | |||
| A ] provides phase-to-neutral relationship of {{math|1=''V''<sub>LL</sub> = 2 ''V''<sub>LN</sub> }}, however, LN load is imposed on one phase.<ref name=Fowler>{{cite book|last=Fowler|first=Nick|title=Electrician's Calculations Manual |edition=2nd|year=2011|publisher=McGraw-Hill|isbn=978-0-07-177017-0|pages=3–5|url=https://books.google.com/books?id=9QBWHJdPGM0C}}</ref> A transformer manufacturer's page suggests that LN loading not exceed 5% of transformer capacity.<ref>{{Cite web|url=http://www.federalpacific.com/university/transbasics/chapter3.html|archive-url=https://web.archive.org/web/20120530122049/http://www.federalpacific.com/university/transbasics/chapter3.html|url-status=dead|title=Federal pacific|archive-date=May 30, 2012}}</ref> | |||
| Since {{sqrt|3}} ≈ 1.73, defining {{math|''V''<sub>LN</sub>}} as 100% gives {{math|''V''<sub>LL</sub>}} {{math|1=≈ 100% × 1.73 = 173%}}. If {{math|''V''<sub>LL</sub>}} was set as 100%, then {{math|''V''<sub>LN</sub> ≈ 57.7%}}. | Since {{sqrt|3}} ≈ 1.73, defining {{math|''V''<sub>LN</sub>}} as 100% gives {{math|''V''<sub>LL</sub>}} {{math|1=≈ 100% × 1.73 = 173%}}. If {{math|''V''<sub>LL</sub>}} was set as 100%, then {{math|''V''<sub>LN</sub> ≈ 57.7%}}. | ||
| === Unbalanced loads === | === Unbalanced loads === | ||
| When the currents on the three live wires of a three-phase system are not equal or are not at an exact 120° phase angle, the power loss is greater than for a perfectly balanced system. The method of ] is used to analyze unbalanced systems. | |||
| When the currents on the three live wires of a three-phase system are not equal or are not at an exact 120° phase angle, the power loss is greater than for a perfectly balanced system. The method of ] is used to analyze unbalanced systems. | |||
| === Non-linear loads === | === Non-linear loads === | ||
| With linear loads, the neutral only carries the current due to imbalance between the phases. ]s and devices that utilize rectifier-capacitor front-end such as ], computers, office equipment and such produce ] that are in-phase on all the supply phases. Consequently, such harmonic currents add in the neutral in a wye system (or in the grounded (zigzag) transformer in a delta system), which can cause the neutral current to exceed the phase current.<ref name="IAEI">{{cite web|last=Lowenstein|first=Michael|title=The 3rd Harmonic Blocking Filter: A Well Established Approach to Harmonic Current Mitigation|url=http://www.iaei.org/blogpost/890108/159506/The-3rd-Harmonic-Blocking-Filter-A-Well-Established-Approach-to-Harmonic-Current-Mitigation|publisher=IAEI Magazine|access-date=24 November 2012|url-status=dead|archive-url=https://archive.today/20130908001044/http://www.iaei.org/blogpost/890108/159506/The-3rd-Harmonic-Blocking-Filter-A-Well-Established-Approach-to-Harmonic-Current-Mitigation|archive-date=8 September 2013}}</ref><ref>{{cite web|last=Enjeti|first=Prasad|title=Harmonics in Low Voltage Three-Phase Four-Wire Electric Distribution Systems and Filtering Solutions|url=http://www.pserc.wisc.edu/documents/general_information/presentations/pserc_seminars/pserc_seminars0/enjeti_slides.pdf|publisher=Texas A&M University Power Electronics and Power Quality Laboratory|access-date=24 November 2012|archive-date=13 June 2010|archive-url=https://web.archive.org/web/20100613051835/http://www.pserc.wisc.edu/documents/general_information/presentations/pserc_seminars/pserc_seminars0/enjeti_slides.pdf|url-status=dead}}</ref> | |||
| With linear loads, the neutral only carries the current due to imbalance between the phases. Devices that utilize rectifier-capacitor front-end such as ], computers, office equipment and such produce ]s that are in-phase on all the supply phases. Consequently, such harmonic currents add in the neutral, which can cause the neutral current to exceed the phase current.<ref name="IAEI">{{cite web|last=Lowenstein|first=Michael|title=The 3rd Harmonic Blocking Filter: A Well Established Approach to Harmonic Current Mitigation|url=http://www.iaei.org/blogpost/890108/159506/The-3rd-Harmonic-Blocking-Filter-A-Well-Established-Approach-to-Harmonic-Current-Mitigation|publisher=IAEI Magazine|accessdate=24 November 2012}}</ref><ref>{{cite web|last=Enjeti|first=Prasad|title=Harmonics in Low Voltage Three-Phase Four-Wire Electric Distribution Systems and Filtering Solutions|url=http://www.pserc.wisc.edu/documents/general_information/presentations/pserc_seminars/pserc_seminars0/enjeti_slides.pdf|publisher=Texas A&M University Power Electronics and Power Quality Laboratory|accessdate=24 November 2012}}</ref> | |||
| == Three-phase loads == | == Three-phase loads == | ||
| {{See also|Industrial and multiphase power plugs and sockets}} | |||
| ] | |||
| An important class of three-phase load is the ]. A three-phase induction motor has a simple design, inherently high starting torque and high efficiency. Such motors are applied in industry for many applications. A three-phase motor is more compact and less costly than a single-phase motor of the same voltage class and rating, and single-phase AC motors above {{cvt|10|hp|lk=in}} are uncommon. Three-phase motors also vibrate less and hence last longer than single-phase motors of the same power used under the same conditions.<ref>{{cite book |last1=Alexander |first1=Charles K.|last2=Sadiku |first2=Matthew N. O.|date=2007 |title=Fundamentals of Electric Circuits |page=504|location=New York |publisher=McGraw-Hill |isbn=978-0-07-297718-9 }}</ref> | |||
| Resistive heating loads such as electric ]s or space heating may be connected to three-phase systems. Electric lighting may also be similarly connected. | |||
| An important class of three-phase load is the ]. A three-phase induction motor has a simple design, inherently high starting torque and high efficiency. Such motors are applied in industry for many applications. A three-phase motor is more compact and less costly than a single-phase motor of the same voltage class and rating and single-phase AC motors above 10 HP (7.5 kW) are uncommon.{{citation needed|date=December 2012}} Three-phase motors also vibrate less and hence last longer than single-phase motors of the same power used under the same conditions.{{citation needed|date=December 2012}} | |||
| Line frequency flicker in light can be reduced by evenly spreading |
Line frequency flicker in light is detrimental to ]s used in sports event broadcasting for ] replays. It can be reduced by evenly spreading line frequency operated light sources across the three phases so that the illuminated area is lit from all three phases. This technique was applied successfully at the 2008 Beijing Olympics.<ref name=3phlight>{{cite web|last=Hui|first=Sun|title=Sports Lighting – Design Considerations For The Beijing 2008 Olympic Games|url=http://www.geappliances.com/email/olympic_specifier/downloads/SportsLightinginBeijing2008OlympicGames.pdf|publisher=GE Lighting|access-date=18 December 2012|archive-date=28 April 2015|archive-url=https://web.archive.org/web/20150428120243/http://www.geappliances.com/email/olympic_specifier/downloads/SportsLightinginBeijing2008OlympicGames.pdf|url-status=dead}}</ref> | ||
| Resistance heating loads such as electric ]s or space heating may be connected to three-phase systems. Electric lighting may also be similarly connected. | |||
| ]s may use a three-phase source to produce a six-pulse DC output.<ref> |
]s may use a three-phase source to produce a six-pulse DC output.<ref>{{cite journal |title=ACSL/Graphic Modeller Component Models for Electric Power Education |first1=Steven |last1=Pekarek |first2=Timothy |last2=Skvarenina |date=November 1998 |volume=41 |issue=4 |journal=IEEE Transactions on Education |url=http://www.ewh.ieee.org/soc/es/Nov1998/08/CONVERTR.HTM |archive-url=https://web.archive.org/web/20030626035019/http://www.ewh.ieee.org/soc/es/Nov1998/08/CONVERTR.HTM |url-status=dead |archive-date=June 26, 2003 |doi=10.1109/TE.1998.787374 |page=348 |bibcode=1998ITEdu..41..348P }}</ref> The output of such rectifiers is much smoother than rectified single phase and, unlike single-phase, does not drop to zero between pulses. Such rectifiers may be used for battery charging, ] processes such as ] and the ] used in ], and for operation of DC motors. ]s may make the equivalent of six-phase full-wave rectification, twelve pulses per cycle, and this method is occasionally employed to reduce the cost of the filtering components, while improving the quality of the resulting DC. | ||
| ] used in the past on electric stoves in Germany]] | |||
| One example of a three-phase load is the ] used in ] and in refining of ores. | |||
| In many European countries electric stoves are usually designed for a three-phase feed |
In many European countries electric stoves are usually designed for a three-phase feed with permanent connection. Individual heating units are often connected between phase and neutral to allow for connection to a single-phase circuit if three-phase is not available.<ref>, ''Electrical Times'', volume 148, p. 691, 1965.</ref> Other usual three-phase loads in the domestic field are ] systems and ]s. Homes in Europe have standardized on a nominal 230 V ±10% between any phase and ground. Most groups of houses are fed from a three-phase street transformer so that individual premises with above-average demand can be fed with a second or third phase connection. | ||
| == Phase converters == | == Phase converters == | ||
| ]s are used when three-phase equipment needs to be operated on a single-phase power source. <!-- what about the example for operating a large single phase load on a 3ph generator and need to balance it across 3 phases? -->They are used when three-phase power is not available or cost is not justifiable. Such converters may also allow the frequency to be varied, allowing speed control. Some railway locomotives use a single-phase source to drive three-phase motors fed through an electronic drive.<ref>{{cite journal|date=Oct 2011|title=Speeding-up Conventional Lines and Shinkansen|url=https://www.ejrcf.or.jp/jrtr/jrtr58/pdf/51-60web.pdf |archive-url=https://ghostarchive.org/archive/20221009/https://www.ejrcf.or.jp/jrtr/jrtr58/pdf/51-60web.pdf |archive-date=2022-10-09 |url-status=live|journal=Japan Railway & Transport Review|volume=58|page=58}}</ref> | |||
| A ] is a three-phase motor with special starting arrangements and ] correction that produces balanced three-phase voltages. When properly designed, these rotary converters can allow satisfactory operation of a three-phase motor on a single-phase source. In such a device, the energy storage is performed by the ] (flywheel effect) of the rotating components. An external flywheel is sometimes found on one or both ends of the shaft. | |||
| Phase converters are used when three-phase equipment needs to be operated on a single-phase power source. <!-- what about the example for operating a large single phase load on a 3ph generator and need to balance it across 3 phases? -->They are used when three-phase power is not available or cost is not justifiable. Such converters may also allow the frequency to be varied (resynthesis) allowing speed control. Some railway locomotives use a single-phase source to drive three-phase motors fed through an electronic drive.<ref>{{cite journal|journal=Japan Railway & Transport Review|date=Oct 2011|url=http://www.jrtr.net/jrtr58/pdf/51-60web.pdf|volume=No. 58|page=58}}</ref> | |||
| A three-phase generator can be driven by a single-phase motor. This motor-generator combination can provide a frequency changer function as well as phase conversion, but requires two machines with all their expenses and losses. The motor-generator method can also form an ] when used in conjunction with a large flywheel and a battery-powered DC motor; such a combination will deliver nearly constant power compared to the temporary frequency drop experienced with a standby generator set gives until the standby generator kicks in. | |||
| === Mechanical === | |||
| Capacitors and ]s can be used to approximate a three-phase system in a static phase converter, but the voltage and phase angle of the additional phase may only be useful for certain loads. | |||
| One method to generate three-phase power from a single-phase source is the ], essentially a three-phase motor with special starting arrangements and ] correction that produces balanced three-phase voltages. When properly designed, these rotary converters can allow satisfactory operation of a three-phase motor on a single-phase source. In such a device, the energy storage is performed by the ] (flywheel effect) of the rotating components. An external flywheel is sometimes found on one or both ends of the shaft. | |||
| ]s and ] use power electronic devices to synthesize a balanced three-phase supply from single-phase input power. | |||
| A three-phase generator can be driven by a single-phase motor. This motor-generator combination can provide a frequency changer function as well as phase conversion, but requires two machines with all their expense and losses. The motor-generator method can also form an ] when used in conjunction with a large flywheel and a battery-powered DC motor for really constant power, a standby generator set gives more frequency drop until standby generator kicks in. | |||
| ==Testing== | |||
| === Non-mechanical === | |||
| Verification of the phase sequence in a circuit is of considerable practical importance. Two sources of three-phase power must not be connected in parallel unless they have the same phase sequence, for example, when connecting a generator to an energized distribution network or when connecting two transformers in parallel. Otherwise, the interconnection will behave like a short circuit, and excess current will flow. The direction of rotation of three-phase motors can be reversed by interchanging any two phases; it may be impractical or harmful to test a machine by momentarily energizing the motor to observe its rotation. Phase sequence of two sources can be verified by measuring voltage between pairs of terminals and observing that terminals with very low voltage between them will have the same phase, whereas pairs that show a higher voltage are on different phases. | |||
| {{unreferenced section|date=December 2012}} | |||
| Where the absolute phase identity is not required, phase rotation test instruments can be used to identify the rotation sequence with one observation. The phase rotation test instrument may contain a miniature three-phase motor, whose direction of rotation can be directly observed through the instrument case. Another pattern uses a pair of lamps and an internal phase-shifting network to display the phase rotation. Another type of instrument can be connected to a de-energized three-phase motor and can detect the small voltages induced by residual magnetism, when the motor shaft is rotated by hand. A lamp or other indicator lights to show the sequence of voltages at the terminals for the given direction of shaft rotation.<ref>Steve Sentry, "Motor Control Fundamentals", Cengage Learning, 2012, {{ISBN|1133709176}}, page 70</ref> | |||
| A second method that was popular in the 1940s and 1950s was the ''transformer method''. At that time, capacitors were more expensive than transformers, so an ] was used to apply more power through fewer capacitors. Separated it from another common method, the static converter, as both methods have no moving parts, which separates them from the rotary converters.<!-- This method needs citation --> | |||
| Another method often attempted is with a device referred to as a static phase converter. This method of running three-phase equipment is commonly attempted with motor loads though it only supplies 2/3 power and can cause the motor loads to run hot and in some cases overheat. This method does not work when sensitive circuitry is involved such as ] devices or in induction and rectifier-type loads. | |||
| ]s (also known as solid-state ] and adjustable speed drives) are used to provide precise speed and torque control of three-phase motors. Some models can be powered by a single-phase supply. VFDs work by converting the supply voltage to DC and then converting the DC to a suitable three-phase source for the motor. | |||
| ]s are designed for fixed-frequency operation from a single-phase source. Similar to a variable-frequency drive, they use a microprocessor to control solid-state power switching components to maintain balanced three-phase voltages. | |||
| == Alternatives to three-phase == | == Alternatives to three-phase == | ||
| ; ]: Used when three-phase power is not available and allows double the normal utilization voltage to be supplied for high-power loads. | |||
| ; ]: Uses two AC voltages, with a 90-electrical-degree phase shift between them. Two-phase circuits may be wired with two pairs of conductors, or two wires may be combined, requiring only three wires for the circuit. Currents in the common conductor add to 1.4 times (<math>\sqrt{2}</math> ) the current in the individual phases, so the common conductor must be larger. Two-phase and three-phase systems can be interconnected by a ], invented by ].<ref name="Brittain (2007)">{{cite journal |last=Brittain |first=J. E. |title=Electrical Engineering Hall of Fame: Charles F. Scott |journal=] |year=2007 |volume=95 |issue=4 |pages=836–839 |doi=10.1109/JPROC.2006.892488}}</ref> Very early AC machines, notably the first generators at ], used a two-phase system, and some remnant two-phase distribution systems still exist, but three-phase systems have displaced the two-phase system for modern installations. | |||
| * ] is used when three-phase power is not available and allows double the normal utilization voltage to be supplied for high-power loads. | |||
| ; Monocyclic power: An asymmetrical modified two-phase power system used by ] around 1897, championed by ] and ]. This system was devised to avoid patent infringement. In this system, a generator was wound with a full-voltage single-phase winding intended for lighting loads and with a small fraction (usually 1/4 of the line voltage) winding that produced a voltage in quadrature with the main windings. The intention was to use this "power wire" additional winding to provide starting torque for induction motors, with the main winding providing power for lighting loads. After the expiration of the Westinghouse patents on symmetrical two-phase and three-phase power distribution systems, the monocyclic system fell out of use; it was difficult to analyze and did not last long enough for satisfactory energy metering to be developed. | |||
| * ], like three-phase, gives constant power transfer to a linear load. For loads that connect each phase to neutral, assuming the load is the same power draw, the two-wire system has a neutral current that is greater than neutral current in a three-phase system. Also motors are not entirely linear, which means that despite the theory, motors running on three-phase tend to run smoother than those on two-phase. The generators in the ] at ] that were installed in 1895 were the largest generators in the world at the time and were two-phase machines. True two-phase power distribution is obsolete for "new work" applications, but still exists for "old work" applications, perhaps most particularly in Buffalo and Niagara Falls, NY, Toronto and Niagara Falls, Ontario, Philadelphia and Reading, PA, and Camden, NJ. "New work" three-phase installations may be supplied by old two-phase feeders, and "old work" two-phase installations may be supplied by new three-phase feeders using a ], invented by ].<ref name="Brittain (2007)">{{cite journal |last=Brittain |first=J. E. |title=Electrical Engineering Hall of Fame: Charles F. Scott |journal=] |year=2007 |volume=95 |issue=4 |pages=836–839 |doi=10.1109/JPROC.2006.892488}}</ref> Special-purpose systems may use a two-phase system for frequency control. | |||
| ; High-phase-order systems: Have been built and tested for power transmission. Such transmission lines typically would use six or twelve phases. High-phase-order transmission lines allow transfer of slightly less than proportionately higher power through a given volume without the expense of a ] (HVDC) converter at each end of the line. However, they require correspondingly more pieces of equipment. | |||
| * ''Monocyclic power'' was a name for an asymmetrical modified two-phase power system used by ] around 1897, championed by ] and ]. This system was devised to avoid patent infringement. In this system, a generator was wound with a full-voltage single-phase winding intended for lighting loads and with a small fraction (usually 1/4 of the line voltage) winding that produced a voltage in quadrature with the main windings. The intention was to use this "power wire" additional winding to provide starting torque for induction motors, with the main winding providing power for lighting loads. After the expiration of the Westinghouse patents on symmetrical two-phase and three-phase power distribution systems, the monocyclic system fell out of use; it was difficult to analyze and did not last long enough for satisfactory energy metering to be developed. | |||
| ; DC: AC was historically used because it could be easily transformed to higher voltages for long distance transmission. However modern electronics can raise the voltage of DC with high efficiency, and DC lacks ] which permits transmission wires to be lighter and cheaper and so ] gives lower losses over long distances. | |||
| * High-phase-order systems for power transmission have been built and tested. Such transmission lines typically would use six phases or twelve phases. High-phase-order transmission lines allow transfer of slightly less than proportionately higher power through a given volume without the expense of a ] (HVDC) converter at each end of the line. However, they require correspondingly more pieces of equipment. | |||
| == Color codes == | == Color codes == | ||
| {{See also|Electrical wiring#Colour code}} | {{See also|Electrical wiring#Colour code}} | ||
| Conductors of a three-phase system are usually identified by a color code, to |
Conductors of a three-phase system are usually identified by a color code, to facilitate balanced loading and to assure the correct phase rotation for ]. Colors used may adhere to International Standard ] (later ]), older standards or to no standard at all and may vary even within a single installation. For example, in the U.S. and Canada, different color codes are used for grounded (earthed) and ungrounded systems. | ||
| {| class="wikitable" | {| class="wikitable" | ||
| ! Country | ! rowspan=2 colspan=2 | Country | ||
| ! colspan=6 | Phases<ref group="note" name="note9">Many labelling systems exist for phases, some having additional meaning, such as: {{nowrap|H1, H2, H3}}; {{nowrap|A, B, C}}; {{nowrap|R, S, T}}; {{nowrap|U, V, W}}; {{nowrap|R, Y, B}}.</ref> | |||
| ! L1 | |||
| ! rowspan=2 colspan=2 | Neutral, <br/>N<ref group="note" name="note10">Also, grounded conductor.</ref> | |||
| ! L2 | |||
| ! rowspan=2 colspan=3 | Protective earth, <br/>PE<ref group="note" name="note11">Also, earth, or grounding conductor.</ref> | |||
| ! L3 | |||
| ! Neutral | |||
| ! Ground / protective earth | |||
| |- | |- | ||
| ! colspan=2 | L1 | |||
| | Australia and New Zealand as per AS/NZS 3000:2007 Figure 3.2 (or as per IEC 60446 as approved by AS:3000) | |||
| ! colspan=2 | L2 | |||
| | bgcolor="red"|Red (or brown) <ref group="note" name="note1">In Australia and New Zealand, active conductors can be any color except green/yellow, green, yellow, black or light blue. Yellow is no longer permitted in the 2007 revision of wiring code ASNZS 3000. European color codes are used for all IEC or flex cables such as extension leads, appliance leads etc. and are equally permitted for use in building wiring per AS/NZS 3000:2007.</ref> | |||
| ! colspan=2 | L3 | |||
| | bgcolor="white"|White (or black)<ref group="note" name="note1" /> (prev. yellow) | |||
| | bgcolor="darkblue" style="color:white"|Dark blue (or grey)<ref group="note" name="note1" /> | |||
| | bgcolor="black" style="color:white"|Black (or blue)<ref group="note" name="note1" /> | |||
| | bgcolor="green" style="color:yellow"|Green/yellow striped (green on very old installations) | |||
| |- | |- | ||
| | colspan=2 | Australia and New Zealand (AS/NZS{{nbsp}}3000:2007 Figure{{nbsp}}3.2, or IEC{{nbsp}}60446 as approved by AS:3000) | |||
| | Canada (mandatory)<ref>Canadian Electrical Code Part I, 23rd Edition, (2002) ISBN 1-55324-690-X, rule 4-036 (3)</ref> | |||
| | style="padding:0.2em 0.6em; background:red;" | || Red, or brown<ref group="note" name="note1">In Australia and New Zealand, active conductors can be any color except green/yellow, green, yellow, black, or light blue. Yellow is no longer permitted in the 2007 revision of wiring code ASNZS 3000. European color codes are used for all IEC or flex cables such as extension leads, appliance leads etc. and are equally permitted for use in building wiring per AS/NZS 3000:2007.</ref> | |||
| | bgcolor="red"|Red | |||
| | style="padding:0.2em 0.6em; background:white;" | || White;<ref group="note" name="note1" /> {{abbr|prev.|previously}} yellow | |||
| | bgcolor="black" style="color:white"|Black | |||
| | style="padding:0.2em 0.6em; background:darkblue;" | || Dark blue, or grey<ref group="note" name="note1" /> | |||
| | bgcolor="blue" style="color:white"|Blue | |||
| | style="padding:0.2em 0.6em; background:black;" | || Black, or blue<ref group="note" name="note1" /> | |||
| | bgcolor="white"|White or Grey | |||
| | style="background:green; padding:0.2em 0.3em;" | || style="padding:0.2em; background:yellow;" | || Green/yellow-striped (installations prior to 1966: green) | |||
| | bgcolor="green" style="color:white"|Green or bare copper | |||
| |- | |- | ||
| | rowspan=2 | Canada | |||
| | Canada (isolated three-phase installations)<ref>'']'' 23th{{Clarify|date=September 2013|reason=Should this be 19th or is the date wrong and it should be 22nd in 2012?}} edition 2002, rule 24-208(c)</ref> | |||
| | Mandatory<ref>{{cite book |date=2015 |title=C22.1-15 – Canadian Electrical Code, Part I: Safety Standard for Electrical Installations |publisher=Canadian Standards Association |edition=23rd |isbn=978-1-77139-718-6 |page=Rule 4–038 |no-pp=y}}</ref> | |||
| | bgcolor="orange"|Orange | |||
| | style="background:red;" | || Red<ref group="note" name="note2">In Canada the high leg conductor in a high-leg delta system is always marked red.</ref> | |||
| | bgcolor="#7F462C" style="color:white"|Brown | |||
| | style="background:black;" | || Black | |||
| | bgcolor="yellow"|Yellow | |||
| | style="background:blue;" | || Blue | |||
| | bgcolor="white"|White | |||
| | |
| style="background:white;" | || White, or grey | ||
| | style="background:green;" | || style="padding:0.2em; background:yellow;" | || Green, perhaps yellow-striped, or uninsulated | |||
| |- | |- | ||
| | Isolated systems<ref>{{cite book |date=2015 |title=C22.1-15 – Canadian Electrical Code, Part I: Safety Standard for Electrical Installations |publisher=Canadian Standards Association |edition=23rd |isbn=978-1-77139-718-6 |page=Rule 24–208(c) |no-pp=y}}</ref> | |||
| | ] and all countries who use European ] standards April 2004 (]), Hong Kong from July 2007, Singapore from March 2009 | |||
| | |
| style="background:orange;" | || Orange | ||
| | |
| style="background:#7f462c;" | || Brown | ||
| | |
| style="background:yellow;" | || Yellow | ||
| | |
| style="background:white;" | || White, or grey | ||
| | style="background:green;" | || style="padding:0.2em; background:yellow;" | || Green perhaps yellow-striped | |||
| | bgcolor="green" style="color:yellow"|Green/yellow striped<ref group="note" name="note2">The international standard green-yellow marking of protective-earth conductors was introduced to reduce the risk of confusion by ] installers. About 7% to 10% of men cannot clearly distinguish between red and green, which is a particular concern in older schemes where red marks a live conductor and green marks protective earth or safety ground.</ref> | |||
| |- | |- | ||
| | colspan=2 | European ] (] and others; since April 2004, ], later IEC{{nbsp}}60445-2017), United Kingdom (since 31{{nbsp}}March 2004), Hong Kong (from July 2007), Singapore (from March 2009), Russia (since 2009; GOST{{nbsp}}R{{nbsp}}50462), Argentina, Ukraine, Belarus, Kazakhstan, South Korea (from Jan. 2021) | |||
| | Older European (prior to ], varied by country <ref group="note" name="note3">In Europe, there still exist many installations with older colors but, since the early 1970s, all new installations use green/yellow earth according to ]. (E.g. Phase/Neutral+Earth German: black/grey + red France green/red + White Russia: Red/ Grey + Black; Switzerland: Red/ Grey +Yellow or yellow & red Denmark: White/Black + Red</ref> | |||
| | style="background:#7f462c;" | || Brown | |||
| | bgcolor="red"|Red | |||
| | style="background:black;" | || Black | |||
| | bgcolor="yellow"|Yellow | |||
| | |
| style="background:gray;" | || Grey | ||
| | |
| style="background:blue;" | || Blue | ||
| | style="background:green;" | || style="padding:0.2em; background:yellow;" | || Green/yellow-striped<ref group="note" name="note3">The international standard green-yellow marking of protective-earth conductors was introduced to reduce the risk of confusion by ] installers. About 7% to 10% of men cannot clearly distinguish between red and green, which is a particular concern in older schemes where red marks a live conductor and green marks protective earth or safety ground.</ref> | |||
| | bgcolor="green" style="color:yellow"|Green/yellow striped (green on installations before c. 1970) | |||
| |- | |- | ||
| | colspan=2 | Older European (prior to ], varied by country)<ref group="note" name="note4">In Europe, there still exist many installations with older colors but, since the early 1970s, all new installations use green/yellow earth according to ]. (E.g. phase/neutral & earth, German: black/grey & red; France: green/red & white; Russia: red/grey & black; Switzerland: red/grey & yellow or yellow & red; Denmark: white/black & red.</ref> | |||
| | UK before April 2006, Hong Kong before April 2009, South Africa, Malaysia, Singapore before February 2011 | |||
| | bgcolor="red"|Red | |||
| | bgcolor="yellow"|Yellow | |||
| | bgcolor="blue" style="color:white"|Blue | |||
| | bgcolor="black" style="color:white"|Black | |||
| | bgcolor="green" style="color:yellow"|Green/yellow striped (green on installations before c. 1970) | |||
| |- | |- | ||
| | colspan=2 | UK (before April 2006), Hong Kong (before April 2009), South Africa, Malaysia, Singapore (before February 2011) | |||
| | India | |||
| | |
| style="background:red;" | || Red | ||
| | |
| style="background:yellow;" | || Yellow | ||
| | |
| style="background:blue;" | || Blue | ||
| | |
| style="background:black;" | || Black | ||
| | |
| style="background:green;" | || style="padding:0.2em; background:yellow;" | || Green/yellow-striped (before c. 1970: green) | ||
| |- | |- | ||
| | colspan=2 | India | |||
| | Former USSR (Russia, Ukraine, Kazakhstan) and People's Republic of China (per GB 50303-2002 Section 15.2.2) | |||
| | style="background:red;" | || Red | |||
| | bgcolor="yellow"|Yellow | |||
| | style="background:yellow;" | || Yellow | |||
| | bgcolor="green"|Green | |||
| | style="background:blue;" | || Blue | |||
| | bgcolor="red"|Red | |||
| | |
| style="background:black;" | || Black | ||
| | |
| style="background:green;" | || style="padding:0.2em; background:yellow;" | || Green, perhaps yellow-striped | ||
| |- | |- | ||
| | colspan=2 | Chile – NCH 4/2003 | |||
| | Norway | |||
| | |
| style="background:blue;" | || Blue | ||
| | |
| style="background:black;" | || Black | ||
| | |
| style="background:red;" | || Red | ||
| | |
| style="background:white;" | || White | ||
| | style="background:green;" | || style="padding:0.2em; background:yellow;" | || Green, perhaps yellow-striped | |||
| | bgcolor="yellow" style="color:green"|Yellow/green striped, older may be only yellow or bare copper | |||
| |- | |- | ||
| | colspan=2 | Former USSR (Russia, Ukraine, Kazakhstan; before 2009), People's Republic of China<ref group="note" name="note8">Note that while China officially uses phase 1: yellow, phase 2: green, phase 3: red, neutral: blue, ground: green/yellow, this is not strongly enforced and there is significant local variation.</ref> (GB{{nbsp}}50303-2002 Section{{nbsp}}15.2.2) | |||
| | United States (common practice)<ref group="note" name="note4">See Paul Cook: . IEE Wiring Matters, Spring 2006.</ref> | |||
| | |
| style="background:yellow;" | || Yellow | ||
| | style="background:green;" | || Green | |||
| | bgcolor="red"|Red | |||
| | |
| style="background:red;" | || Red | ||
| | |
| style="background:#09f;" | || Sky blue | ||
| | style="background:green;" | || style="padding:0.2em; background:yellow;" | || Green/yellow-striped | |||
| | bgcolor="green" style="color:yellow"|Green, green/yellow striped,<ref group="note" name="note7">In the U.S., a green/yellow striped wire may indicate an ].{{Citation needed|date=August 2007}} In most countries today, green/yellow striped wire may only be used for protective earth (safety ground) and may never be unconnected or used for any other purpose.</ref> or a bare copper wire | |||
| |- | |- | ||
| | colspan=2 | Norway (before CENELEC adoption) | |||
| | United States (alternative practice)<ref group="note" name="note5">Since 1975, the U.S. National Electric Code has not specified coloring of phase conductors. It is common practice in many regions to identify 120/208 (wye) conductors as black, red, and blue, and 277/480 (wye or delta) conductors as brown, orange, yellow. In a 120/240 delta system with a 208v high leg, the high leg (typically B phase) is always marked orange, commonly A phase is black and C phase is either red or blue. Local regulations may amend the N.E.C. The U.S. National Electric Code has color requirements for grounded conductors, ground, and grounded-delta 3-phase systems which result in one ungrounded leg having a higher voltage potential to ground than the other two ungrounded legs.</ref> | |||
| | |
| style="background:black;" | || Black | ||
| | |
| style="background:white;" | || White/grey | ||
| | style="background:#7f462c;" | || Brown | |||
| | bgcolor="yellow"|Yellow | |||
| | |
| style="background:blue;" | || Blue | ||
| | style="background:yellow;" | || style="padding:0.2em; background:green;" | || Yellow/green-striped; {{abbr|prev.|previously}} yellow or uninsulated | |||
| | bgcolor="green" style="color:white"|Green | |||
| |- | |||
| | rowspan="5" | United States<ref group="note" name="note6">Since 1975, the U.S. National Electric Code has not specified coloring of phase conductors. It is common practice in many regions to identify 120/208{{nbsp}}V (wye) conductors as black, red, and blue, and 277/480{{nbsp}}V (wye or delta) conductors as brown, orange, yellow. In a 120/240{{nbsp}}V delta system with a 208{{nbsp}}V high leg, the high leg (typically B phase) is always marked orange, commonly A phase is black and C phase is either red or blue. Local regulations may amend the N.E.C. The U.S. National Electric Code has color requirements for grounded conductors, ground, and grounded-delta three-phase systems which result in one ungrounded leg having a higher voltage potential to ground than the other two ungrounded legs.</ref> | |||
| | 120, 208, or 240 V | |||
| | style="background:black;" | || Black | |||
| | style="background:red;" | || Red | |||
| | style="background:blue;" | || Blue | |||
| | style="background:white;" | || White | |||
| | colspan=2 style="background:gold;" | || Bare Conductor (no insulation) | |||
| |- | |||
| | 277 or 480 V | |||
| | style="background:#7f462c;" | || Brown | |||
| | style="background:orange;" | || Orange | |||
| | style="background:yellow;" | || Yellow | |||
| | style="background:gray;" | || Gray | |||
| | colspan=2 style="background:gold;" | || Bare Conductor (no insulation) | |||
| |- | |||
| | rowspan="3" | Alternate Practices (Delta with tapped winding) | |||
| | rowspan="2" style="background:black;" | || rowspan="2" | Black | |||
| | rowspan="2" style="background:orange;" | || rowspan="2" | Orange | |||
| (High-Leg<ref group="note" name="note12">Must be the high leg, if it is present.</ref>) | |||
| | style="background:red;" | || Red | |||
| | rowspan="2" style="background:white;" | || rowspan="2" | White | |||
| | rowspan="2" style="background:green;" | || rowspan="2" style="padding:0.2em; background:yellow;" | || rowspan="2" | Green or Yellow/green-striped or no insulation | |||
| |- | |||
| | style="background:blue;" | || Blue | |||
| |} | |} | ||
| == See also == | == See also == | ||
| {{Div col|colwidth=30em}} | |||
| * ] | |||
| * ] | |||
| * ] | |||
| * ] | |||
| * ] | |||
| * ] | * ] | ||
| * ] | |||
| * ] | |||
| * ] | |||
| * ] | |||
| * ] | * ] | ||
| {{Div col end}} | |||
| == Notes == | == Notes == | ||
| {{Reflist|30em|group="note"}} | |||
| {{reflist|30em|group="note"}} | |||
| == References == | == References == | ||
| {{Reflist}} | |||
| == Further reading == | |||
| * {{cite journal | title=Early Three-Phase Power | journal=IEEE Power and Energy Magazine | volume=5 | issue=5 | date=2007 | issn=1540-7977 | doi=10.1109/MPE.2007.904752 | pages=88–100 | first = Gerhard | last = Neidhofer | |||
| }} | |||
| == External links == | |||
| {{reflist|30em}} | |||
| * | |||
| {{Electricity generation}} | |||
| {{Nikola Tesla}} | {{Nikola Tesla}} | ||
| {{Authority control}} | |||
| ] | ] | ||
| Line 314: | Line 403: | ||
| ] | ] | ||
| ] | ] | ||
| ] | |||
| ] | |||
Latest revision as of 04:54, 3 January 2025
Common electrical power generation, transmission and distribution method for alternating currents For a greater focus on the mathematics of this subject, see Mathematics of three-phase electric power. Not to be confused with Split-phase electric power.
Three-phase electric power (abbreviated 3ϕ) is a common type of alternating current (AC) used in electricity generation, transmission, and distribution. It is a type of polyphase system employing three wires (or four including an optional neutral return wire) and is the most common method used by electrical grids worldwide to transfer power.
Three-phase electrical power was developed in the 1880s by several people. In three-phase power, the voltage on each wire is 120 degrees phase shifted relative to each of the other wires. Because it is an AC system, it allows the voltages to be easily stepped up using transformers to high voltage for transmission and back down for distribution, giving high efficiency.
A three-wire three-phase circuit is usually more economical than an equivalent two-wire single-phase circuit at the same line-to-ground voltage because it uses less conductor material to transmit a given amount of electrical power. Three-phase power is mainly used directly to power large induction motors, other electric motors and other heavy loads. Small loads often use only a two-wire single-phase circuit, which may be derived from a three-phase system.
Terminology
The conductors between a voltage source and a load are called lines, and the voltage between any two lines is called line voltage. The voltage measured between any line and neutral is called phase voltage. For example, for a 208/120-volt service, the line voltage is 208 volts, and the phase voltage is 120 volts.
History
Polyphase power systems were independently invented by Galileo Ferraris, Mikhail Dolivo-Dobrovolsky, Jonas Wenström, John Hopkinson, William Stanley Jr., and Nikola Tesla in the late 1880s.
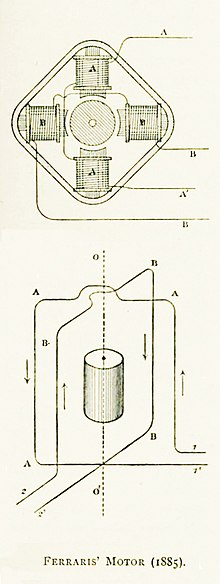
Three phase power evolved out of electric motor development. In 1885, Galileo Ferraris was doing research on rotating magnetic fields. Ferraris experimented with different types of asynchronous electric motors. The research and his studies resulted in the development of an alternator, which may be thought of as an alternating-current motor operating in reverse, so as to convert mechanical (rotating) power into electric power (as alternating current). On 11 March 1888, Ferraris published his research in a paper to the Royal Academy of Sciences in Turin.
Two months later Nikola Tesla gained U.S. patent 381,968 for a three-phase electric motor design, application filed October 12, 1887. Figure 13 of this patent shows that Tesla envisaged his three-phase motor being powered from the generator via six wires.
These alternators operated by creating systems of alternating currents displaced from one another in phase by definite amounts, and depended on rotating magnetic fields for their operation. The resulting source of polyphase power soon found widespread acceptance. The invention of the polyphase alternator is key in the history of electrification, as is the power transformer. These inventions enabled power to be transmitted by wires economically over considerable distances. Polyphase power enabled the use of water-power (via hydroelectric generating plants in large dams) in remote places, thereby allowing the mechanical energy of the falling water to be converted to electricity, which then could be fed to an electric motor at any location where mechanical work needed to be done. This versatility sparked the growth of power-transmission network grids on continents around the globe.
Mikhail Dolivo-Dobrovolsky developed a three-phase electrical generator and a three-phase electric motor in 1888 and studied star and delta connections. His three-phase three-wire transmission system was displayed in 1891 in Germany at the International Electrotechnical Exhibition, where Dolivo-Dobrovolsky used the system to transmit electric power at the distance of 176 km (110 miles) with 75% efficiency. In 1891 he also created a three-phase transformer and short-circuited (squirrel-cage) induction motor. He designed the world's first three-phase hydroelectric power plant in 1891. Inventor Jonas Wenström received in 1890 a Swedish patent on the same three-phase system. The possibility of transferring electrical power from a waterfall at a distance was explored at the Grängesberg mine. A 45 m fall at Hällsjön, Smedjebackens kommun, where a small iron work had been located, was selected. In 1893, a three-phase 9.5 kV system was used to transfer 400 horsepower (300 kW) a distance of 15 km (10 miles), becoming the first commercial application.
Principle



In a symmetric three-phase power supply system, three conductors each carry an alternating current of the same frequency and voltage amplitude relative to a common reference, but with a phase difference of one third of a cycle (i.e., 120 degrees out of phase) between each. The common reference is usually connected to ground and often to a current-carrying conductor called the neutral. Due to the phase difference, the voltage on any conductor reaches its peak at one third of a cycle after one of the other conductors and one third of a cycle before the remaining conductor. This phase delay gives constant power transfer to a balanced linear load. It also makes it possible to produce a rotating magnetic field in an electric motor and generate other phase arrangements using transformers (for instance, a two-phase system using a Scott-T transformer). The amplitude of the voltage difference between two phases is times the amplitude of the voltage of the individual phases.
The symmetric three-phase systems described here are simply referred to as three-phase systems because, although it is possible to design and implement asymmetric three-phase power systems (i.e., with unequal voltages or phase shifts), they are not used in practice because they lack the most important advantages of symmetric systems.
In a three-phase system feeding a balanced and linear load, the sum of the instantaneous currents of the three conductors is zero. In other words, the current in each conductor is equal in magnitude to the sum of the currents in the other two, but with the opposite sign. The return path for the current in any phase conductor is the other two phase conductors.
Constant power transfer is possible with any number of phases greater than one. However, two-phase systems do not have neutral-current cancellation and thus use conductors less efficiently, and more than three phases complicates infrastructure unnecessarily. Additionally, in some practical generators and motors, two phases can result in a less smooth (pulsating) torque.
Three-phase systems may have a fourth wire, common in low-voltage distribution. This is the neutral wire. The neutral allows three separate single-phase supplies to be provided at a constant voltage and is commonly used for supplying multiple single-phase loads. The connections are arranged so that, as far as possible in each group, equal power is drawn from each phase. Further up the distribution system, the currents are usually well balanced. Transformers may be wired to have a four-wire secondary and a three-wire primary, while allowing unbalanced loads and the associated secondary-side neutral currents.
Phase sequence
See also: Electric machine § SequenceWiring for three phases is typically identified by colors that vary by country and voltage. The phases must be connected in the correct order to achieve the intended direction of rotation of three-phase motors. For example, pumps and fans do not work as intended in reverse. Maintaining the identity of phases is required if two sources could be connected at the same time. A direct connection between two different phases is a short circuit and leads to flow of unbalanced current.
Advantages and disadvantages
As compared to a single-phase AC power supply that uses two current-carrying conductors (phase and neutral), a three-phase supply with no neutral and the same phase-to-ground voltage and current capacity per phase can transmit three times as much power by using just 1.5 times as many wires (i.e., three instead of two). Thus, the ratio of capacity to conductor material is doubled. The ratio of capacity to conductor material increases to 3:1 with an ungrounded three-phase and center-grounded single-phase system (or 2.25:1 if both use grounds with the same gauge as the conductors). That leads to higher efficiency, lower weight, and cleaner waveforms.
Three-phase supplies have properties that make them desirable in electric power distribution systems:
- The phase currents tend to cancel out one another, summing to zero in the case of a linear balanced load, which allows a reduction of the size of the neutral conductor because it carries little or no current. With a balanced load, all the phase conductors carry the same current and so can have the same size.
- Power transfer into a linear balanced load is constant, which, in motor/generator applications, helps to reduce vibrations.
- Three-phase systems can produce a rotating magnetic field with a specified direction and constant magnitude, which simplifies the design of electric motors, as no starting circuit is required.
However, most loads are single-phase. In North America, single-family houses and individual apartments are supplied one phase from the power grid and use a split-phase system to the panelboard from which most branch circuits will carry 120 V. Circuits designed for higher powered devices such as stoves, dryers, or outlets for electric vehicles carry 240 V.
In Europe, three-phase power is normally delivered to the panelboard and further to higher powered devices.
Generation and distribution
See also: Mains electricity by country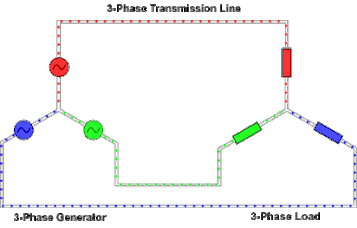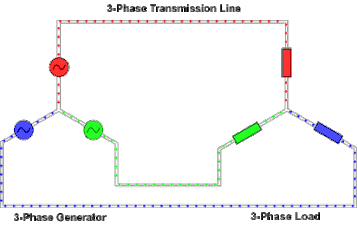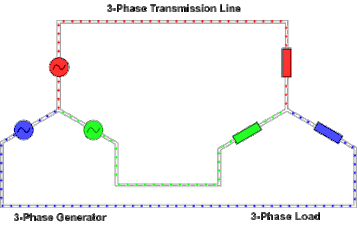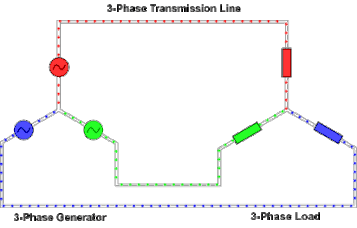
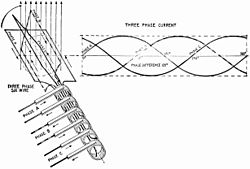
 Left image: elementary six-wire three-phase alternator with each phase using a separate pair of transmission wires. Right image: elementary three-wire three-phase alternator showing how the phases can share only three wires.
Left image: elementary six-wire three-phase alternator with each phase using a separate pair of transmission wires. Right image: elementary three-wire three-phase alternator showing how the phases can share only three wires.
At the power station, an electrical generator converts mechanical power into a set of three AC electric currents, one from each coil (or winding) of the generator. The windings are arranged such that the currents are at the same frequency but with the peaks and troughs of their wave forms offset to provide three complementary currents with a phase separation of one-third cycle (120° or 2π⁄3 radians). The generator frequency is typically 50 or 60 Hz, depending on the country.
At the power station, transformers change the voltage from generators to a level suitable for transmission in order to minimize losses.
After further voltage conversions in the transmission network, the voltage is finally transformed to the standard utilization before power is supplied to customers.
Most automotive alternators generate three-phase AC and rectify it to DC with a diode bridge.
Transformer connections
A "delta" (Δ) connected transformer winding is connected between phases of a three-phase system. A "wye" (Y) transformer connects each winding from a phase wire to a common neutral point.
A single three-phase transformer can be used, or three single-phase transformers.
In an "open delta" or "V" system, only two transformers are used. A closed delta made of three single-phase transformers can operate as an open delta if one of the transformers has failed or needs to be removed. In open delta, each transformer must carry current for its respective phases as well as current for the third phase, therefore capacity is reduced to 87%. With one of three transformers missing and the remaining two at 87% efficiency, the capacity is 58% (2⁄3 of 87%).
Where a delta-fed system must be grounded for detection of stray current to ground or protection from surge voltages, a grounding transformer (usually a zigzag transformer) may be connected to allow ground fault currents to return from any phase to ground. Another variation is a "corner grounded" delta system, which is a closed delta that is grounded at one of the junctions of transformers.
Three-wire and four-wire circuits

There are two basic three-phase configurations: wye (Y) and delta (Δ). As shown in the diagram, a delta configuration requires only three wires for transmission, but a wye (star) configuration may have a fourth wire. The fourth wire, if present, is provided as a neutral and is normally grounded. The three-wire and four-wire designations do not count the ground wire present above many transmission lines, which is solely for fault protection and does not carry current under normal use.
A four-wire system with symmetrical voltages between phase and neutral is obtained when the neutral is connected to the "common star point" of all supply windings. In such a system, all three phases will have the same magnitude of voltage relative to the neutral. Other non-symmetrical systems have been used.
The four-wire wye system is used when a mixture of single-phase and three-phase loads are to be served, such as mixed lighting and motor loads. An example of application is local distribution in Europe (and elsewhere), where each customer may be only fed from one phase and the neutral (which is common to the three phases). When a group of customers sharing the neutral draw unequal phase currents, the common neutral wire carries the currents resulting from these imbalances. Electrical engineers try to design the three-phase power system for any one location so that the power drawn from each of three phases is the same, as far as possible at that site. Electrical engineers also try to arrange the distribution network so the loads are balanced as much as possible, since the same principles that apply to individual premises also apply to the wide-scale distribution system power. Hence, every effort is made by supply authorities to distribute the power drawn on each of the three phases over a large number of premises so that, on average, as nearly as possible a balanced load is seen at the point of supply.

For domestic use, some countries such as the UK may supply one phase and neutral at a high current (up to 100 A) to one property, while others such as Germany may supply 3 phases and neutral to each customer, but at a lower fuse rating, typically 40–63 A per phase, and "rotated" to avoid the effect that more load tends to be put on the first phase.

Based on wye (Y) and delta (Δ) connection. Generally, there are four different types of three-phase transformer winding connections for transmission and distribution purposes:
- wye (Y) – wye (Y) is used for small current and high voltage,
- Delta (Δ) – Delta (Δ) is used for large currents and low voltages,
- Delta (Δ) – wye (Y) is used for step-up transformers, i.e., at generating stations,
- wye (Y) – Delta (Δ) is used for step-down transformers, i.e., at the end of the transmission.
In North America, a high-leg delta supply is sometimes used where one winding of a delta-connected transformer feeding the load is center-tapped and that center tap is grounded and connected as a neutral as shown in the second diagram. This setup produces three different voltages: If the voltage between the center tap (neutral) and each of the top and bottom taps (phase and anti-phase) is 120 V (100%), the voltage across the phase and anti-phase lines is 240 V (200%), and the neutral to "high leg" voltage is ≈ 208 V (173%).
The reason for providing the delta connected supply is usually to power large motors requiring a rotating field. However, the premises concerned will also require the "normal" North American 120 V supplies, two of which are derived (180 degrees "out of phase") between the "neutral" and either of the center-tapped phase points.
Balanced circuits
In the perfectly balanced case all three lines share equivalent loads. Examining the circuits, we can derive relationships between line voltage and current, and load voltage and current for wye- and delta-connected loads.
In a balanced system each line will produce equal voltage magnitudes at phase angles equally spaced from each other. With V1 as our reference and V3 lagging V2 lagging V1, using angle notation, and VLN the voltage between the line and the neutral we have:
These voltages feed into either a wye- or delta-connected load.
Wye (or, star; Y)
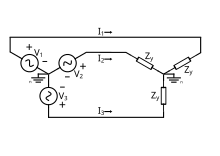
The voltage seen by the load will depend on the load connection; for the wye case, connecting each load to a phase (line-to-neutral) voltages gives
where Ztotal is the sum of line and load impedances (Ztotal = ZLN + ZY), and θ is the phase of the total impedance (Ztotal).
The phase angle difference between voltage and current of each phase is not necessarily 0 and depends on the type of load impedance, Zy. Inductive and capacitive loads will cause current to either lag or lead the voltage. However, the relative phase angle between each pair of lines (1 to 2, 2 to 3, and 3 to 1) will still be −120°.

- Vab = (1∠α − 1∠α + 120°) √3 |V|∠α + 30°,
- Vbc = √3 |V|∠α − 90°,
- Vca = √3 |V|∠α + 150°
By applying Kirchhoff's current law (KCL) to the neutral node, the three phase currents sum to the total current in the neutral line. In the balanced case:
Delta (Δ)
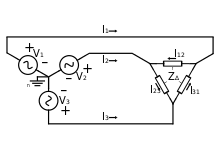
In the delta circuit, loads are connected across the lines, and so loads see line-to-line voltages:
(Φv1 is the phase shift for the first voltage, commonly taken to be 0°; in this case, Φv2 = −120° and Φv3 = −240° or 120°.)
Further:
where θ is the phase of delta impedance (ZΔ).
Relative angles are preserved, so I31 lags I23 lags I12 by 120°. Calculating line currents by using KCL at each delta node gives
and similarly for each other line:
where, again, θ is the phase of delta impedance (ZΔ).

- Ia = Iab − Ica = √3 Iab∠−30°,
- Ib = Ibc − Iab,
- Ic = Ica − Ibc.
- S3Φ = 3VphaseI*phase.
Inspection of a phasor diagram, or conversion from phasor notation to complex notation, illuminates how the difference between two line-to-neutral voltages yields a line-to-line voltage that is greater by a factor of √3. As a delta configuration connects a load across phases of a transformer, it delivers the line-to-line voltage difference, which is √3 times greater than the line-to-neutral voltage delivered to a load in the wye configuration. As the power transferred is V/Z, the impedance in the delta configuration must be 3 times what it would be in a wye configuration for the same power to be transferred.
Single-phase loads
Except in a high-leg delta system and a corner-grounded delta system, single-phase loads may be connected across any two phases, or a load can be connected from phase to neutral. Distributing single-phase loads among the phases of a three-phase system balances the load and makes most economical use of conductors and transformers.
In a symmetrical three-phase four-wire wye system, the three phase conductors have the same voltage to the system neutral. The voltage between line conductors is √3 times the phase conductor to neutral voltage:
The currents returning from the customers' premises to the supply transformer all share the neutral wire. If the loads are evenly distributed on all three phases, the sum of the returning currents in the neutral wire is approximately zero. Any unbalanced phase loading on the secondary side of the transformer will use the transformer capacity inefficiently.
If the supply neutral is broken, phase-to-neutral voltage is no longer maintained. Phases with higher relative loading will experience reduced voltage, and phases with lower relative loading will experience elevated voltage, up to the phase-to-phase voltage.
A high-leg delta provides phase-to-neutral relationship of VLL = 2 VLN , however, LN load is imposed on one phase. A transformer manufacturer's page suggests that LN loading not exceed 5% of transformer capacity.
Since √3 ≈ 1.73, defining VLN as 100% gives VLL ≈ 100% × 1.73 = 173%. If VLL was set as 100%, then VLN ≈ 57.7%.
Unbalanced loads
When the currents on the three live wires of a three-phase system are not equal or are not at an exact 120° phase angle, the power loss is greater than for a perfectly balanced system. The method of symmetrical components is used to analyze unbalanced systems.
Non-linear loads
With linear loads, the neutral only carries the current due to imbalance between the phases. Gas-discharge lamps and devices that utilize rectifier-capacitor front-end such as switch-mode power supplies, computers, office equipment and such produce third-order harmonics that are in-phase on all the supply phases. Consequently, such harmonic currents add in the neutral in a wye system (or in the grounded (zigzag) transformer in a delta system), which can cause the neutral current to exceed the phase current.
Three-phase loads
See also: Industrial and multiphase power plugs and sockets
An important class of three-phase load is the electric motor. A three-phase induction motor has a simple design, inherently high starting torque and high efficiency. Such motors are applied in industry for many applications. A three-phase motor is more compact and less costly than a single-phase motor of the same voltage class and rating, and single-phase AC motors above 10 hp (7.5 kW) are uncommon. Three-phase motors also vibrate less and hence last longer than single-phase motors of the same power used under the same conditions.
Resistive heating loads such as electric boilers or space heating may be connected to three-phase systems. Electric lighting may also be similarly connected.
Line frequency flicker in light is detrimental to high-speed cameras used in sports event broadcasting for slow-motion replays. It can be reduced by evenly spreading line frequency operated light sources across the three phases so that the illuminated area is lit from all three phases. This technique was applied successfully at the 2008 Beijing Olympics.
Rectifiers may use a three-phase source to produce a six-pulse DC output. The output of such rectifiers is much smoother than rectified single phase and, unlike single-phase, does not drop to zero between pulses. Such rectifiers may be used for battery charging, electrolysis processes such as aluminium production and the electric arc furnace used in steelmaking, and for operation of DC motors. Zigzag transformers may make the equivalent of six-phase full-wave rectification, twelve pulses per cycle, and this method is occasionally employed to reduce the cost of the filtering components, while improving the quality of the resulting DC.

In many European countries electric stoves are usually designed for a three-phase feed with permanent connection. Individual heating units are often connected between phase and neutral to allow for connection to a single-phase circuit if three-phase is not available. Other usual three-phase loads in the domestic field are tankless water heating systems and storage heaters. Homes in Europe have standardized on a nominal 230 V ±10% between any phase and ground. Most groups of houses are fed from a three-phase street transformer so that individual premises with above-average demand can be fed with a second or third phase connection.
Phase converters
Phase converters are used when three-phase equipment needs to be operated on a single-phase power source. They are used when three-phase power is not available or cost is not justifiable. Such converters may also allow the frequency to be varied, allowing speed control. Some railway locomotives use a single-phase source to drive three-phase motors fed through an electronic drive.
A rotary phase converter is a three-phase motor with special starting arrangements and power factor correction that produces balanced three-phase voltages. When properly designed, these rotary converters can allow satisfactory operation of a three-phase motor on a single-phase source. In such a device, the energy storage is performed by the inertia (flywheel effect) of the rotating components. An external flywheel is sometimes found on one or both ends of the shaft.
A three-phase generator can be driven by a single-phase motor. This motor-generator combination can provide a frequency changer function as well as phase conversion, but requires two machines with all their expenses and losses. The motor-generator method can also form an uninterruptible power supply when used in conjunction with a large flywheel and a battery-powered DC motor; such a combination will deliver nearly constant power compared to the temporary frequency drop experienced with a standby generator set gives until the standby generator kicks in.
Capacitors and autotransformers can be used to approximate a three-phase system in a static phase converter, but the voltage and phase angle of the additional phase may only be useful for certain loads.
Variable-frequency drives and digital phase converters use power electronic devices to synthesize a balanced three-phase supply from single-phase input power.
Testing
Verification of the phase sequence in a circuit is of considerable practical importance. Two sources of three-phase power must not be connected in parallel unless they have the same phase sequence, for example, when connecting a generator to an energized distribution network or when connecting two transformers in parallel. Otherwise, the interconnection will behave like a short circuit, and excess current will flow. The direction of rotation of three-phase motors can be reversed by interchanging any two phases; it may be impractical or harmful to test a machine by momentarily energizing the motor to observe its rotation. Phase sequence of two sources can be verified by measuring voltage between pairs of terminals and observing that terminals with very low voltage between them will have the same phase, whereas pairs that show a higher voltage are on different phases.
Where the absolute phase identity is not required, phase rotation test instruments can be used to identify the rotation sequence with one observation. The phase rotation test instrument may contain a miniature three-phase motor, whose direction of rotation can be directly observed through the instrument case. Another pattern uses a pair of lamps and an internal phase-shifting network to display the phase rotation. Another type of instrument can be connected to a de-energized three-phase motor and can detect the small voltages induced by residual magnetism, when the motor shaft is rotated by hand. A lamp or other indicator lights to show the sequence of voltages at the terminals for the given direction of shaft rotation.
Alternatives to three-phase
- Split-phase electric power
- Used when three-phase power is not available and allows double the normal utilization voltage to be supplied for high-power loads.
- Two-phase electric power
- Uses two AC voltages, with a 90-electrical-degree phase shift between them. Two-phase circuits may be wired with two pairs of conductors, or two wires may be combined, requiring only three wires for the circuit. Currents in the common conductor add to 1.4 times ( ) the current in the individual phases, so the common conductor must be larger. Two-phase and three-phase systems can be interconnected by a Scott-T transformer, invented by Charles F. Scott. Very early AC machines, notably the first generators at Niagara Falls, used a two-phase system, and some remnant two-phase distribution systems still exist, but three-phase systems have displaced the two-phase system for modern installations.
- Monocyclic power
- An asymmetrical modified two-phase power system used by General Electric around 1897, championed by Charles Proteus Steinmetz and Elihu Thomson. This system was devised to avoid patent infringement. In this system, a generator was wound with a full-voltage single-phase winding intended for lighting loads and with a small fraction (usually 1/4 of the line voltage) winding that produced a voltage in quadrature with the main windings. The intention was to use this "power wire" additional winding to provide starting torque for induction motors, with the main winding providing power for lighting loads. After the expiration of the Westinghouse patents on symmetrical two-phase and three-phase power distribution systems, the monocyclic system fell out of use; it was difficult to analyze and did not last long enough for satisfactory energy metering to be developed.
- High-phase-order systems
- Have been built and tested for power transmission. Such transmission lines typically would use six or twelve phases. High-phase-order transmission lines allow transfer of slightly less than proportionately higher power through a given volume without the expense of a high-voltage direct current (HVDC) converter at each end of the line. However, they require correspondingly more pieces of equipment.
- DC
- AC was historically used because it could be easily transformed to higher voltages for long distance transmission. However modern electronics can raise the voltage of DC with high efficiency, and DC lacks skin effect which permits transmission wires to be lighter and cheaper and so high-voltage direct current gives lower losses over long distances.
Color codes
See also: Electrical wiring § Colour codeConductors of a three-phase system are usually identified by a color code, to facilitate balanced loading and to assure the correct phase rotation for motors. Colors used may adhere to International Standard IEC 60446 (later IEC 60445), older standards or to no standard at all and may vary even within a single installation. For example, in the U.S. and Canada, different color codes are used for grounded (earthed) and ungrounded systems.
| Country | Phases | Neutral, N |
Protective earth, PE | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| L1 | L2 | L3 | ||||||||||
| Australia and New Zealand (AS/NZS 3000:2007 Figure 3.2, or IEC 60446 as approved by AS:3000) | Red, or brown | White; prev. yellow | Dark blue, or grey | Black, or blue | Green/yellow-striped (installations prior to 1966: green) | |||||||
| Canada | Mandatory | Red | Black | Blue | White, or grey | Green, perhaps yellow-striped, or uninsulated | ||||||
| Isolated systems | Orange | Brown | Yellow | White, or grey | Green perhaps yellow-striped | |||||||
| European CENELEC (European Union and others; since April 2004, IEC 60446, later IEC 60445-2017), United Kingdom (since 31 March 2004), Hong Kong (from July 2007), Singapore (from March 2009), Russia (since 2009; GOST R 50462), Argentina, Ukraine, Belarus, Kazakhstan, South Korea (from Jan. 2021) | Brown | Black | Grey | Blue | Green/yellow-striped | |||||||
| Older European (prior to IEC 60446, varied by country) | ||||||||||||
| UK (before April 2006), Hong Kong (before April 2009), South Africa, Malaysia, Singapore (before February 2011) | Red | Yellow | Blue | Black | Green/yellow-striped (before c. 1970: green) | |||||||
| India | Red | Yellow | Blue | Black | Green, perhaps yellow-striped | |||||||
| Chile – NCH 4/2003 | Blue | Black | Red | White | Green, perhaps yellow-striped | |||||||
| Former USSR (Russia, Ukraine, Kazakhstan; before 2009), People's Republic of China (GB 50303-2002 Section 15.2.2) | Yellow | Green | Red | Sky blue | Green/yellow-striped | |||||||
| Norway (before CENELEC adoption) | Black | White/grey | Brown | Blue | Yellow/green-striped; prev. yellow or uninsulated | |||||||
| United States | 120, 208, or 240 V | Black | Red | Blue | White | Bare Conductor (no insulation) | ||||||
| 277 or 480 V | Brown | Orange | Yellow | Gray | Bare Conductor (no insulation) | |||||||
| Alternate Practices (Delta with tapped winding) | Black | Orange
(High-Leg) |
Red | White | Green or Yellow/green-striped or no insulation | |||||||
| Blue | ||||||||||||
See also
- Industrial and multiphase power plugs and sockets
- International Electrotechnical Exhibition
- Mathematics of three-phase electric power
- Rotary phase converter
- Three-phase AC railway electrification
- Y-Δ transform
Notes
- Many labelling systems exist for phases, some having additional meaning, such as: H1, H2, H3; A, B, C; R, S, T; U, V, W; R, Y, B.
- Also, grounded conductor.
- Also, earth, or grounding conductor.
- ^ In Australia and New Zealand, active conductors can be any color except green/yellow, green, yellow, black, or light blue. Yellow is no longer permitted in the 2007 revision of wiring code ASNZS 3000. European color codes are used for all IEC or flex cables such as extension leads, appliance leads etc. and are equally permitted for use in building wiring per AS/NZS 3000:2007.
- In Canada the high leg conductor in a high-leg delta system is always marked red.
- The international standard green-yellow marking of protective-earth conductors was introduced to reduce the risk of confusion by color blind installers. About 7% to 10% of men cannot clearly distinguish between red and green, which is a particular concern in older schemes where red marks a live conductor and green marks protective earth or safety ground.
- In Europe, there still exist many installations with older colors but, since the early 1970s, all new installations use green/yellow earth according to IEC 60446. (E.g. phase/neutral & earth, German: black/grey & red; France: green/red & white; Russia: red/grey & black; Switzerland: red/grey & yellow or yellow & red; Denmark: white/black & red.
- Note that while China officially uses phase 1: yellow, phase 2: green, phase 3: red, neutral: blue, ground: green/yellow, this is not strongly enforced and there is significant local variation.
- Since 1975, the U.S. National Electric Code has not specified coloring of phase conductors. It is common practice in many regions to identify 120/208 V (wye) conductors as black, red, and blue, and 277/480 V (wye or delta) conductors as brown, orange, yellow. In a 120/240 V delta system with a 208 V high leg, the high leg (typically B phase) is always marked orange, commonly A phase is black and C phase is either red or blue. Local regulations may amend the N.E.C. The U.S. National Electric Code has color requirements for grounded conductors, ground, and grounded-delta three-phase systems which result in one ungrounded leg having a higher voltage potential to ground than the other two ungrounded legs.
- Must be the high leg, if it is present.
References
- Saleh, S. A.; Rahman, M. A. (25 March 2013). "The analysis and development of controlled 3φ wavelet modulated AC-DC converter". 2012 IEEE International Conference on Power Electronics, Drives and Energy Systems (PEDES). pp. 1–6. doi:10.1109/PEDES.2012.6484282. ISBN 978-1-4673-4508-8. S2CID 32935308.
- William D. Stevenson, Jr. Elements of Power System Analysis Third Edition, McGraw-Hill, New York (1975). ISBN 0-07-061285-4, p. 2
- Terrell Croft, Wilford Summers (ed), American Electricians' Handbook, 11th ed., McGraw Hill, 1987 ISBN 0-07-013932-6 page 3-10 figure 3-23.
- Brumbach, Michael (2014). Industrial maintenance. Clifton Park, NY: Delmar, Cengage Learning. p. 411. ISBN 9781133131199.
- "AC Power History and Timeline". Edison Tech Center. Retrieved January 24, 2022.
- "Milestones:Rotating Fields and Early Induction Motors, 1885-1888". ETHW. 2022-06-14. Retrieved 2025-01-01.
- US422746A, "Dobrowolsky", issued 1890-03-04
- "US Patent: 422,746 - Electric induction apparatus or transformer". www.datamp.org. Retrieved 2025-01-01.
- US455683A, "Dobeowolsky", issued 1891-07-07
- US456804A, "Dobrowolsky", issued 1891-07-28
- "Wayback Machine". www.mpoweruk.com.
- Gerhard Neidhöfer: Michael von Dolivo-Dobrowolsky und der Drehstrom. Geschichte der Elektrotechnik VDE-Buchreihe, Volume 9, VDE VERLAG, Berlin Offenbach, ISBN 978-3-8007-3115-2.
- "The Earliest Years of Three-Phase Power—1891–1893 [Scanning Our Past]". ieeexplore.ieee.org. Archived from the original on 2024-12-11. Retrieved 2025-01-01.
- Bergström och Nordlund, Lars (2002). Ellära- Kretsteknik och fältteori. Naturaläromedel. p. 283. ISBN 91-7536-330-5.
- Hjulström, Filip (1940). Elektrifieringens utveckling i Sverige, en ekonomisk-geografisk översikt. [Excerpt taken from YMER 1941, häfte 2.Utgiven av Sällskapet för antropologi och geografi: Meddelande från Upsala univeristets geografiska institution, N:o 29, published by Esselte ab, Stockholm 1941 no. 135205]
- von Meier, Alexandra (2006). Electric Power Systems. Hoboken, New Jersey: John Wiley & Sons, Inc. p. 160. ISBN 978-0-471-17859-0.
We also stated one rationale for this three-phase system; namely, that a three-phase generator experiences a constant torque on its rotor as opposed to the pulsating torque that appears in a single- or two-phase machine, which is obviously preferable from a mechanical engineering standpoint.
- Cotton, H, Electrical Technology, 6th ed., Pitman, London, 1950, p. 268.
- Hawkins Electrical Guide, Theo. Audel and Co., 2nd ed., 1917, vol. 4, Ch. 46: Alternating Currents, p. 1026, fig. 1260.
- Hawkins Electrical Guide, Theo. Audel and Co., 2nd ed., 1917, vol. 4, Ch. 46: Alternating Currents, p. 1026, fig. 1261.
- "A New Design for Automotive Alternators" (PDF). August 30, 2017. Archived from the original (PDF) on 2017-08-30.
- ^ Fowler, Nick (2011). Electrician's Calculations Manual (2nd ed.). McGraw-Hill. pp. 3–5. ISBN 978-0-07-177017-0.
- Gibbs, J. B. (April 27, 1920). "Three-Phase Power from Single-Phase Transformer Connections". Power. 51 (17). McGraw-Hill: 673. Retrieved 21 December 2012.
- H. W. Beaty, D. G. Fink (ed.), Standard Handbook for Electrical Engineers. 15th ed., McGraw-Hill, 2007, ISBN 0-07-144146-8, pp. 10–11.
- "Schneider" (PDF). Archived (PDF) from the original on 2022-10-09.
- "Saving energy through load balancing and load scheduling" (PDF). Archived from the original (PDF) on 2014-09-11. Retrieved 2014-08-03.
- ^ J. Duncan Glover; Mulukutla S. Sarma; Thomas J. Overbye (April 2011). Power System Analysis & Design. Cengage Learning. pp. 60–68. ISBN 978-1-111-42579-1.
- "What is "Stray Voltage"?" (PDF). Utility Technology Engineers-Consultants (UTEC). August 10, 2015. Retrieved December 10, 2023.
- ^ Lowenstein, Michael. "The 3rd Harmonic Blocking Filter: A Well Established Approach to Harmonic Current Mitigation". IAEI Magazine. Archived from the original on 8 September 2013. Retrieved 24 November 2012.
- The boy electrician by J. W. Sims M.I.E.E. (p. 98).
- "Federal pacific". Archived from the original on May 30, 2012.
- Enjeti, Prasad. "Harmonics in Low Voltage Three-Phase Four-Wire Electric Distribution Systems and Filtering Solutions" (PDF). Texas A&M University Power Electronics and Power Quality Laboratory. Archived from the original (PDF) on 13 June 2010. Retrieved 24 November 2012.
- Alexander, Charles K.; Sadiku, Matthew N. O. (2007). Fundamentals of Electric Circuits. New York: McGraw-Hill. p. 504. ISBN 978-0-07-297718-9.
- Hui, Sun. "Sports Lighting – Design Considerations For The Beijing 2008 Olympic Games" (PDF). GE Lighting. Archived from the original (PDF) on 28 April 2015. Retrieved 18 December 2012.
- Pekarek, Steven; Skvarenina, Timothy (November 1998). "ACSL/Graphic Modeller Component Models for Electric Power Education". IEEE Transactions on Education. 41 (4): 348. Bibcode:1998ITEdu..41..348P. doi:10.1109/TE.1998.787374. Archived from the original on June 26, 2003.
- "British and European practices for domestic appliances compared", Electrical Times, volume 148, p. 691, 1965.
- "Speeding-up Conventional Lines and Shinkansen" (PDF). Japan Railway & Transport Review. 58: 58. Oct 2011. Archived (PDF) from the original on 2022-10-09.
- Steve Sentry, "Motor Control Fundamentals", Cengage Learning, 2012, ISBN 1133709176, page 70
- Brittain, J. E. (2007). "Electrical Engineering Hall of Fame: Charles F. Scott". Proceedings of the IEEE. 95 (4): 836–839. doi:10.1109/JPROC.2006.892488.
- C22.1-15 – Canadian Electrical Code, Part I: Safety Standard for Electrical Installations (23rd ed.). Canadian Standards Association. 2015. Rule 4–038. ISBN 978-1-77139-718-6.
- C22.1-15 – Canadian Electrical Code, Part I: Safety Standard for Electrical Installations (23rd ed.). Canadian Standards Association. 2015. Rule 24–208(c). ISBN 978-1-77139-718-6.
Further reading
- Neidhofer, Gerhard (2007). "Early Three-Phase Power ". IEEE Power and Energy Magazine. 5 (5): 88–100. doi:10.1109/MPE.2007.904752. ISSN 1540-7977.
External links
| Nikola Tesla | ||
|---|---|---|
| Career and inventions |  | |
| Writings | ||
| Other | ||
| Related |
| |
 times the amplitude of the voltage of the individual phases.
times the amplitude of the voltage of the individual phases.








 ) the current in the individual phases, so the common conductor must be larger. Two-phase and three-phase systems can be interconnected by a
) the current in the individual phases, so the common conductor must be larger. Two-phase and three-phase systems can be interconnected by a 