| Revision as of 10:34, 12 March 2021 editCagliost (talk | contribs)Autopatrolled, Extended confirmed users17,771 editsNo edit summary← Previous edit | Latest revision as of 11:44, 1 January 2025 edit undoExotoxin1959 (talk | contribs)165 edits →Equilibrium analysis: Style consistencyTag: 2017 wikitext editor | ||
| (47 intermediate revisions by 24 users not shown) | |||
| Line 1: | Line 1: | ||
| {{short description|Mathematical game}} | {{short description|Mathematical game}} | ||
| In ], "'''guess {{sfrac|2|3}} of the average'''" is a game where |
In ], "'''guess {{sfrac|2|3}} of the average'''" is a game where players simultaneously select a ] between 0 and 100, inclusive. The winner of the game is the player(s) who select a number closest to {{sfrac|2|3}} of the average of numbers chosen by all players.<ref name=":3">{{Cite journal |last1=Duffy |first1=John |last2=Nagel |first2=Rosemarie|author2-link=Rosemarie Nagel |date=1997-11-01 |title=On the Robustness of Behaviour in Experimental 'Beauty Contest' Games |journal=The Economic Journal |volume=107 |issue=445 |pages=1684–1700 |doi=10.1111/j.1468-0297.1997.tb00075.x |s2cid=153447786 |issn=0013-0133|doi-access=free }}</ref> | ||
| ⚫ | == History == | ||
| ⚫ | ] | ||
| Alain Ledoux is the founding father of the "guess {{sfrac|2|3}} of the average" game. In 1981, Ledoux used this game as a tie breaker in his French magazine ]. He asked about 4,000 readers, who reached the same number of points in previous puzzles, to state an integer between 1 and 1,000,000,000. The winner was the one who guessed closest to {{sfrac|2|3}} of the average guess.<ref>{{cite journal |last1=Ledoux |first1=Alain |year=1981 |title=Concours résultats complets. Les victimes se sont plu à jouer le 14 d'atout |trans-title=Competition results complete. The victims were pleased to play the trump 14 |journal=Jeux & Stratégie |language=fr |volume=2 |issue=10 |pages=10–11}}</ref> ] (1995) revealed the potential of guessing games of that kind: They are able to disclose participants' "depth of reasoning."<ref name="Nagel" /> | |||
| == Equilibrium analysis == | == Equilibrium analysis == | ||
| In this game there is no ] strategy |
In this game, there is no ] ], but there are strongly ]. There is a unique ] ]. This equilibrium can be found by ].<ref name=":0">{{Cite journal |last1=Coricelli |first1=Giorgio |last2=Nagel |first2=Rosemarie|author2-link=Rosemarie Nagel |date=2009-06-09 |title=Neural correlates of depth of strategic reasoning in medial prefrontal cortex |journal=Proceedings of the National Academy of Sciences |volume=106 |issue=23 |pages=9163–9168 |doi=10.1073/pnas.0807721106 |pmid=19470476 |pmc=2685737 |bibcode=2009PNAS..106.9163C |issn=0027-8424|doi-access=free }}</ref> | ||
| Intuitively, guessing any number higher than {{sfrac|2|3}} of what you expect others to guess on average cannot be part of a Nash equilibrium. The highest possible average that would occur if everyone guessed 100 is {{sfrac|66|2|3}}. Therefore, choosing a number that lies above {{sfrac|66|2|3}} is strictly dominated for every player. These guesses can thus be eliminated. Once these strategies are eliminated for every player, {{sfrac|66|2|3}} becomes the new highest possible average (that is, if everyone chooses {{sfrac|66|2|3}}). Therefore, any guess above {{sfrac|44|4|9}} is weakly dominated for every player since no player will guess above {{sfrac|66|2|3}}, and {{sfrac|2|3}} of {{sfrac|66|2|3}} is {{sfrac|44|4|9}}. This process will continue as this logic is continually applied, If the same group of people play the game consistently, with each step, the highest possible logical answer keeps getting smaller, the average will move close to 0, all other numbers above 0 have been eliminated. If all players understand this logic and select 0, the game reaches its Nash equilibrium.<ref>{{Cite journal |last=Nagel, Bosch-Domènech, Satorra, and Garcia-Montalvo |first=Rosemarie, Antoni, Albert and José |date=5 December 2002 |title=One, Two, (Three), Infinity, ...: Newspaper and Lab Beauty-Contest Experiments |url=https://www.upf.edu/documents/8394861/8967210/boschetalAER2002.pdf/bd0a2012-563c-7db9-b36a-eff29cf50897 |journal=American Economic Review |volume=92 |issue=5 |pages=1687–1702 |doi=10.1257/000282802762024737 |hdl=10230/573 |hdl-access=free }}</ref> At this state, every player has chosen to play the ] strategy for themselves, given what everyone else is choosing. | |||
| ⚫ | |||
| ⚫ | However, this degeneration does not occur in quite the same way if choices are restricted to, for example, the integers between 0 and 1. In this case, all integers except 0 and 1 vanish; it becomes advantageous to select 0 if you expect that at least {{sfrac|1|4}} of all players will do so, and select 1 otherwise. (In this way, it is a lopsided version of the so-called "consensus game", where one wins by being in the majority.){{cn|date=September 2024}} | ||
| ⚫ | == Experimental results == | ||
| ⚫ | |||
| == Rationality versus common knowledge of rationality == | == Rationality versus common knowledge of rationality == | ||
| This game illustrates the difference between the ] of an actor and the ] of the ] of all players. To achieve its Nash equilibrium of 0, this game requires all players to be perfectly rational, rationality to be common knowledge, and all players to expect everyone else to behave accordingly.<ref name=":1">{{Cite journal |last=Sbriglia |first=Patrizia |date=2004 |title=Revealing the Depth of Reasoning in p-Beauty Contest Games |url=http://dx.doi.org/10.2139/ssrn.656586 |journal=SSRN Electronic Journal |doi=10.2139/ssrn.656586 |s2cid=197657612 |issn=1556-5068}}</ref> Common knowledge means that every player has the same information, and they also know that everyone else knows that, and that everyone else knows that everyone else knows that, and so on, infinitely.<ref>{{Citation |last=Dekel |first=Eddie |title=Rationality and knowledge in game theory |url=http://dx.doi.org/10.1017/ccol0521580110.005 |work=Advances in Economics and Econometrics: Theory and Applications: Seventh World Congress Vol I |pages=87–172 |place=Cambridge |publisher=Cambridge University Press |doi=10.1017/ccol0521580110.005 |access-date=2022-04-26}}</ref> Common knowledge of rationality of all players is the reason why the winning guess is 0. | |||
| This game illustrates the difference between ] of an actor and the ] of rationality of all players. Even perfectly rational players playing in such a game should not guess 0 unless they know that the other players are rational as well and that all players' rationality is common knowledge. If a rational player reasonably believes that other players will not follow the chain of elimination described above, it would be rational for him/her to guess a number above 0. | |||
| Economic game theorists have modelled this relationship between rationality and the common knowledge of rationality through ]. K stands for the number of times a cycle of reasoning is repeated. A Level-k model usually assumes that k-level 0 agents would approach the game naively and make choices distributed uniformly over the range . In accordance with cognitive hierarchy theory, level 1 players select the best responses to level 0 choices, while level 2 players select the best responses to level 1 choices.<ref>{{Cite journal |last1=Heap |first1=Shaun Hargreaves |last2=Arjona |first2=David Rojo |last3=Sugden |first3=Robert |date=2014 |title=HOW PORTABLE IS LEVEL-0 BEHAVIOR? A TEST OF LEVEL-k THEORY IN GAMES WITH NON-NEUTRAL FRAMES |url=https://www.jstor.org/stable/24029309 |journal=Econometrica |volume=82 |issue=3 |pages=1133–1151 |doi=10.3982/ECTA11132 |jstor=24029309 |issn=0012-9682|hdl=2381/44091 |hdl-access=free }}</ref> Level 1 players would assume that everyone else was playing at level 0, responding to an assumed average of 50 in relation to naive play, and thus their guess would be 33 (2/3 of 50). At k-level 2, a player would play more sophisticatedly and assume that all other players are playing at k-level 1, so they would choose 22 (2/3 of 33).<ref>{{Cite journal |last1=Agranov |first1=Marina |last2=Caplin |first2=Andrew |last3=Tergiman |first3=Chloe |date=2015-05-19 |title=Naive play and the process of choice in guessing games |journal=Journal of the Economic Science Association |volume=1 |issue=2 |pages=146–157 |doi=10.1007/s40881-015-0003-5 |s2cid=7593331 |issn=2199-6776|doi-access=free }}</ref> Players are presumptively aware of the probability distributions of selections at each higher level. It would take approximately 21 k-levels to reach 0, the Nash equilibrium of the game. | |||
| We can suppose that all the players are rational, but they do not have common knowledge of each other's rationality. Even in this case, it is not required that every player guess 0, since they may expect each other to behave irrationally. | |||
| The guessing game depends on three elements: (1) the subject's perceptions of the level 0 would play; (2) the subject's expectations about the cognitive level of other players; and (3) the number of in-game reasoning steps that the subject is capable of completing.<ref>{{Cite journal |last1=Agranov |first1=Marina |last2=Potamites |first2=Elizabeth |last3=Schotter |first3=Andrew |last4=Tergiman |first4=Chloe |date=July 2012 |title=Beliefs and endogenous cognitive levels: An experimental study |url=https://linkinghub.elsevier.com/retrieve/pii/S089982561200022X |journal=Games and Economic Behavior |language=en |volume=75 |issue=2 |pages=449–463 |doi=10.1016/j.geb.2012.02.002|s2cid=1632208 }}</ref> Evidence suggest that most people play at k-levels 0 to 3,<ref name=":2">{{Cite journal |last1=Mauersberger |first1=Felix |last2=Nagel |first2=Rosemarie|author2-link=Rosemarie Nagel |last3=Bühren |first3=Christoph |date=2020-06-04 |title=Bounded rationality in Keynesian beauty contests: a lesson for central bankers? |journal=Economics: The Open-Access, Open-Assessment e-Journal |volume=14 |issue=1 |doi=10.5018/economics-ejournal.ja.2020-16 |s2cid=212631702 |issn=1864-6042|doi-access=free |hdl=10230/45169 |hdl-access=free }}</ref> so you would just have to think one step ahead of that to have a higher chance at winning the game. Therefore, being aware of this logic allow players to adjust their strategy. This means that perfectly rational players playing in such a game should not guess 0 unless they know that the other players are rational as well, and that all players' rationality is common knowledge. If a rational player reasonably believes that other players will not follow the chain of elimination described above, it would be rational for him/her to guess a number above 0 as their best response. | |||
| ⚫ | == History == | ||
| ⚫ | ] | ||
| In reality, we can assume that most players are not perfectly rational, and do not have common knowledge of each other's rationality.<ref>{{Cite journal |last1=Alba-Fernández |first1=Virtudes |last2=Brañas-Garza |first2=Pablo |last3=Jiménez-Jiménez |first3=Francisca |last4=Rodero-Cosano |first4=Javier |date=2010-08-07 |title=Teaching Nash Equilibrium and Dominance: A Classroom Experiment on the Beauty Contest |url=http://dx.doi.org/10.3200/jece.37.3.305-322 |journal=The Journal of Economic Education |volume=37 |issue=3 |pages=305–322 |doi=10.3200/jece.37.3.305-322 |hdl=10261/2097 |s2cid=49574187 |issn=0022-0485|hdl-access=free }}</ref> As a result, they will also expect others to have a ] and thus guess a number higher than 0. | |||
| Alain Ledoux is the founding father of the guess {{sfrac|2|3}} of the average-game. In 1981, Ledoux used this game as a tie breaker in his French magazine ]. He asked about 4,000 readers, who reached the same number of points in previous puzzles, to state an integer between 1 and 1,000,000,000. The winner was the one who guessed closest to {{sfrac|2|3}} of the average guess.<ref>{{cite journal |last1=Ledoux |first1=Alain |year=1981 |title=Concours résultats complets. Les victimes se sont plu à jouer le 14 d'atout |trans-title=Competition results complete. The victims were pleased to play the trump 14 |language=fr |journal=Jeux & Stratégie |volume=2 |issue=10 |pages=10–11 }}</ref> Rosemarie Nagel (1995) revealed the potential of guessing games of that kind: They are able to disclose participants' "depth of reasoning."<ref name=Nagel /> Due to the analogy to Keynes's comparison of newspaper beauty contests and stock market investments<ref>{{cite book |last1=Keynes |first1=John M. |year=1936 |title=The General Theory of Interest, Employment and Money |location=London |publisher=Macmillan |page=156 }}</ref> the guessing game is also known as the ].<ref>{{cite journal |doi=10.1111/j.1468-0297.1997.tb00075.x |jstor=2957901 |title=On the Robustness of Behaviour in Experimental 'Beauty Contest' Games |journal=The Economic Journal |volume=107 |issue=445 |pages=1684 |year=1997 |last1=Duffy |first1=John |last2=Nagel |first2=Rosemarie |s2cid=153447786 }}</ref> Rosemarie Nagel's experimental beauty contest became a famous game in ]. The forgotten inventor of this game was unearthed in 2009 during an online beauty contest experiment with chess players provided by the ]:<ref name="bc">{{cite journal |last1=Bühren |first1=Christoph |last2=Frank |first2=Björn |year=2010 |title=Chess Players Performance Beyond 64 Squares: A Case Study on the Limitations of Cognitive Abilities Transfer |journal=MAGKS Joint Discussion Paper Series in Economics |volume=19-2010 |url=http://www.uni-marburg.de/fb02/makro/forschung/magkspapers/19-2010_buhren.pdf }}</ref> Alain Ledoux, together with over 6,000 other chess players, participated in that experiment which looked familiar to him.<ref name="hist">{{cite journal |last1=Bühren |first1=Christoph |last2=Frank |first2=Björn |last3=Nagel |first3=Rosemarie |year=2012 |title=A Historical Note on the Beauty Contest |journal=MAGKS Joint Discussion Paper Series in Economics |volume=11-2012 |url=https://www.uni-marburg.de/fb02/makro/forschung/magkspapers/11-2012_buehren.pdf }}</ref><ref name="hist2">{{cite journal |doi=10.1016/j.mathsocsci.2016.09.001 |title=Inspired and inspiring: Hervé Moulin and the discovery of the beauty contest game |journal=Mathematical Social Sciences |volume=90 |pages=191–207 |year=2016 |last1=Nagel |first1=Rosemarie |last2=Bühren |first2=Christoph |last3=Frank |first3=Björn |url=https://econ-papers.upf.edu/papers/1539.pdf }}</ref> | |||
| ⚫ | == Experimental results == | ||
| This game is a common demonstration in game theory classes. It reveals the significant heterogeneity of behaviour.<ref name=":2" /> It is unlikely that many people will play rationally according to the Nash equilibrium. This is because the game has no strictly dominant strategy, so it requires players to consider what others will do. For Nash equilibrium to be played, players would need to assume both that everyone else is rational and that there is common knowledge of rationality. However, this is a strong assumption. | |||
| ⚫ | Experiments demonstrate that many people make mistakes and do not assume common knowledge of rationality. It has been demonstrated that even economics graduate students do not guess 0.<ref name="Nagel">{{cite journal |last=Nagel |first=Rosemarie|author-link=Rosemarie Nagel |year=1995 |title=Unraveling in Guessing Games: An Experimental Study |journal=] |volume=85 |issue=5 |pages=1313–26 |jstor=2950991 }}</ref> When performed among ordinary people it is usually found that the winner's guess is much higher than 0: the winning value was found to be 33 in a large online competition organized by the Danish newspaper '']''. 19,196 people participated and the prize was 5000 ]r.<ref name="politiken">{{cite web|url=http://politiken.dk/oekonomi/art5698526/G%C3%A6t-et-tal-konkurrence-afsl%C3%B8rer-at-vi-er-irrationelle|title=Gæt-et-tal konkurrence afslører at vi er irrationelle|last=Schou|first=Astrid|date=22 September 2005|work=]|language=da|access-date=29 August 2017}} Includes a of the guesses. Note that some of the players guessed close to 100. A large number of players guessed 33.3 (i.e. {{sfrac|2|3}} of 50), indicating an assumption that players would guess randomly. A smaller but significant number of players guessed 22.2 (i.e. {{sfrac|2|3}} of 33.3), indicating a second iteration of this theory based on an assumption that players would guess 33.3. The final number of 33 was slightly below this peak, implying that on average each player iterated their assumption 1.07 times.</ref> | ||
| ] | |||
| Grosskopf and Nagel's investigation also revealed that most players do not choose 0 the first time they play this game. Instead, they realise that 0 is the Nash Equilibrium after some repetitions.<ref>{{Cite journal |last1=Grosskopf |first1=Brit |last2=Nagel |first2=Rosemarie|author2-link=Rosemarie Nagel |date=2001 |title=Rational Reasoning or Adaptive Behavior? Evidence from Two-Person Beauty Contest Games |url=http://dx.doi.org/10.2139/ssrn.286573 |journal=SSRN Electronic Journal |doi=10.2139/ssrn.286573 |hdl=10230/686 |s2cid=14073840 |issn=1556-5068|hdl-access=free }}</ref> A study by Nagel reported an average initial choice of around 36. This corresponds to approximately two levels of k-level reasoning.<ref>{{Citation |last1=Kagel |first1=John H. |title=Unraveling in guessing games: An experimental study (by Rosemarie Nagel) |date=2021-07-12 |url=http://dx.doi.org/10.4324/9781003019121-10 |work=The Art of Experimental Economics |pages=109–118 |place=London |publisher=Routledge |isbn=978-1-003-01912-1 |access-date=2022-04-26 |last2=Penta |first2=Antonio|doi=10.4324/9781003019121-10 |s2cid=237752741 }}</ref> | |||
| Kocher and Sutter compared the behaviours between individual and groups in playing this type of game. They observed that while both subjects applied roughly the same level of reasoning, groups learned faster. This demonstrated that repetition enabled a group of individuals to observe others' behaviour in previous games and correspondingly choose a number that increases their chances of winning the game.<ref>{{Cite journal |last1=Kocher |first1=Martin G. |last2=Sutter |first2=Matthias |date=2004-12-22 |title=The Decision Maker Matters: Individual Versus Group Behaviour in Experimental Beauty‐Contest Games |url=http://dx.doi.org/10.1111/j.1468-0297.2004.00966.x |journal=The Economic Journal |volume=115 |issue=500 |pages=200–223 |doi=10.1111/j.1468-0297.2004.00966.x |s2cid=7339369 |issn=0013-0133}}</ref> | |||
| Sbriglia's investigation also revealed that non-winners often try to imitate winners' understanding of the game's structure. Accordingly, other players adopt strategies which are best responses to the imitators' behaviour instead of to the average level of rationality. This accelerates the attainment of the game's Nash equilibrium.<ref name=":1" /> | |||
| == See also == | == See also == | ||
| Line 25: | Line 39: | ||
| == Notes == | == Notes == | ||
| <references/> | <references /> | ||
| == External links == | |||
| * by ] | |||
| {{Game theory}} | {{Game theory}} | ||
Latest revision as of 11:44, 1 January 2025
Mathematical gameIn game theory, "guess 2/3 of the average" is a game where players simultaneously select a real number between 0 and 100, inclusive. The winner of the game is the player(s) who select a number closest to 2/3 of the average of numbers chosen by all players.
History

Alain Ledoux is the founding father of the "guess 2/3 of the average" game. In 1981, Ledoux used this game as a tie breaker in his French magazine Jeux et Stratégie. He asked about 4,000 readers, who reached the same number of points in previous puzzles, to state an integer between 1 and 1,000,000,000. The winner was the one who guessed closest to 2/3 of the average guess. Rosemarie Nagel (1995) revealed the potential of guessing games of that kind: They are able to disclose participants' "depth of reasoning."
Equilibrium analysis
In this game, there is no strictly dominant strategy, but there are strongly dominated strategies. There is a unique pure strategy Nash equilibrium. This equilibrium can be found by iterated elimination of weakly dominated strategies.
Intuitively, guessing any number higher than 2/3 of what you expect others to guess on average cannot be part of a Nash equilibrium. The highest possible average that would occur if everyone guessed 100 is 66+2/3. Therefore, choosing a number that lies above 66+2/3 is strictly dominated for every player. These guesses can thus be eliminated. Once these strategies are eliminated for every player, 66+2/3 becomes the new highest possible average (that is, if everyone chooses 66+2/3). Therefore, any guess above 44+4/9 is weakly dominated for every player since no player will guess above 66+2/3, and 2/3 of 66+2/3 is 44+4/9. This process will continue as this logic is continually applied, If the same group of people play the game consistently, with each step, the highest possible logical answer keeps getting smaller, the average will move close to 0, all other numbers above 0 have been eliminated. If all players understand this logic and select 0, the game reaches its Nash equilibrium. At this state, every player has chosen to play the best response strategy for themselves, given what everyone else is choosing.
However, this degeneration does not occur in quite the same way if choices are restricted to, for example, the integers between 0 and 1. In this case, all integers except 0 and 1 vanish; it becomes advantageous to select 0 if you expect that at least 1/4 of all players will do so, and select 1 otherwise. (In this way, it is a lopsided version of the so-called "consensus game", where one wins by being in the majority.)
Rationality versus common knowledge of rationality
This game illustrates the difference between the perfect rationality of an actor and the common knowledge of the rationality of all players. To achieve its Nash equilibrium of 0, this game requires all players to be perfectly rational, rationality to be common knowledge, and all players to expect everyone else to behave accordingly. Common knowledge means that every player has the same information, and they also know that everyone else knows that, and that everyone else knows that everyone else knows that, and so on, infinitely. Common knowledge of rationality of all players is the reason why the winning guess is 0.
Economic game theorists have modelled this relationship between rationality and the common knowledge of rationality through K-level reasoning. K stands for the number of times a cycle of reasoning is repeated. A Level-k model usually assumes that k-level 0 agents would approach the game naively and make choices distributed uniformly over the range . In accordance with cognitive hierarchy theory, level 1 players select the best responses to level 0 choices, while level 2 players select the best responses to level 1 choices. Level 1 players would assume that everyone else was playing at level 0, responding to an assumed average of 50 in relation to naive play, and thus their guess would be 33 (2/3 of 50). At k-level 2, a player would play more sophisticatedly and assume that all other players are playing at k-level 1, so they would choose 22 (2/3 of 33). Players are presumptively aware of the probability distributions of selections at each higher level. It would take approximately 21 k-levels to reach 0, the Nash equilibrium of the game.
The guessing game depends on three elements: (1) the subject's perceptions of the level 0 would play; (2) the subject's expectations about the cognitive level of other players; and (3) the number of in-game reasoning steps that the subject is capable of completing. Evidence suggest that most people play at k-levels 0 to 3, so you would just have to think one step ahead of that to have a higher chance at winning the game. Therefore, being aware of this logic allow players to adjust their strategy. This means that perfectly rational players playing in such a game should not guess 0 unless they know that the other players are rational as well, and that all players' rationality is common knowledge. If a rational player reasonably believes that other players will not follow the chain of elimination described above, it would be rational for him/her to guess a number above 0 as their best response.
In reality, we can assume that most players are not perfectly rational, and do not have common knowledge of each other's rationality. As a result, they will also expect others to have a bounded rationality and thus guess a number higher than 0.
Experimental results
This game is a common demonstration in game theory classes. It reveals the significant heterogeneity of behaviour. It is unlikely that many people will play rationally according to the Nash equilibrium. This is because the game has no strictly dominant strategy, so it requires players to consider what others will do. For Nash equilibrium to be played, players would need to assume both that everyone else is rational and that there is common knowledge of rationality. However, this is a strong assumption.
Experiments demonstrate that many people make mistakes and do not assume common knowledge of rationality. It has been demonstrated that even economics graduate students do not guess 0. When performed among ordinary people it is usually found that the winner's guess is much higher than 0: the winning value was found to be 33 in a large online competition organized by the Danish newspaper Politiken. 19,196 people participated and the prize was 5000 Danish kroner.
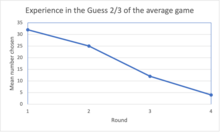
Grosskopf and Nagel's investigation also revealed that most players do not choose 0 the first time they play this game. Instead, they realise that 0 is the Nash Equilibrium after some repetitions. A study by Nagel reported an average initial choice of around 36. This corresponds to approximately two levels of k-level reasoning.
Kocher and Sutter compared the behaviours between individual and groups in playing this type of game. They observed that while both subjects applied roughly the same level of reasoning, groups learned faster. This demonstrated that repetition enabled a group of individuals to observe others' behaviour in previous games and correspondingly choose a number that increases their chances of winning the game.
Sbriglia's investigation also revealed that non-winners often try to imitate winners' understanding of the game's structure. Accordingly, other players adopt strategies which are best responses to the imitators' behaviour instead of to the average level of rationality. This accelerates the attainment of the game's Nash equilibrium.
See also
Notes
- Duffy, John; Nagel, Rosemarie (1997-11-01). "On the Robustness of Behaviour in Experimental 'Beauty Contest' Games". The Economic Journal. 107 (445): 1684–1700. doi:10.1111/j.1468-0297.1997.tb00075.x. ISSN 0013-0133. S2CID 153447786.
- Ledoux, Alain (1981). "Concours résultats complets. Les victimes se sont plu à jouer le 14 d'atout" [Competition results complete. The victims were pleased to play the trump 14]. Jeux & Stratégie (in French). 2 (10): 10–11.
- ^ Nagel, Rosemarie (1995). "Unraveling in Guessing Games: An Experimental Study". American Economic Review. 85 (5): 1313–26. JSTOR 2950991.
- Coricelli, Giorgio; Nagel, Rosemarie (2009-06-09). "Neural correlates of depth of strategic reasoning in medial prefrontal cortex". Proceedings of the National Academy of Sciences. 106 (23): 9163–9168. Bibcode:2009PNAS..106.9163C. doi:10.1073/pnas.0807721106. ISSN 0027-8424. PMC 2685737. PMID 19470476.
- Nagel, Bosch-Domènech, Satorra, and Garcia-Montalvo, Rosemarie, Antoni, Albert and José (5 December 2002). "One, Two, (Three), Infinity, ...: Newspaper and Lab Beauty-Contest Experiments". American Economic Review. 92 (5): 1687–1702. doi:10.1257/000282802762024737. hdl:10230/573.
{{cite journal}}: CS1 maint: multiple names: authors list (link) - ^ Sbriglia, Patrizia (2004). "Revealing the Depth of Reasoning in p-Beauty Contest Games". SSRN Electronic Journal. doi:10.2139/ssrn.656586. ISSN 1556-5068. S2CID 197657612.
- Dekel, Eddie, "Rationality and knowledge in game theory", Advances in Economics and Econometrics: Theory and Applications: Seventh World Congress Vol I, Cambridge: Cambridge University Press, pp. 87–172, doi:10.1017/ccol0521580110.005, retrieved 2022-04-26
- Heap, Shaun Hargreaves; Arjona, David Rojo; Sugden, Robert (2014). "HOW PORTABLE IS LEVEL-0 BEHAVIOR? A TEST OF LEVEL-k THEORY IN GAMES WITH NON-NEUTRAL FRAMES". Econometrica. 82 (3): 1133–1151. doi:10.3982/ECTA11132. hdl:2381/44091. ISSN 0012-9682. JSTOR 24029309.
- Agranov, Marina; Caplin, Andrew; Tergiman, Chloe (2015-05-19). "Naive play and the process of choice in guessing games". Journal of the Economic Science Association. 1 (2): 146–157. doi:10.1007/s40881-015-0003-5. ISSN 2199-6776. S2CID 7593331.
- Agranov, Marina; Potamites, Elizabeth; Schotter, Andrew; Tergiman, Chloe (July 2012). "Beliefs and endogenous cognitive levels: An experimental study". Games and Economic Behavior. 75 (2): 449–463. doi:10.1016/j.geb.2012.02.002. S2CID 1632208.
- ^ Mauersberger, Felix; Nagel, Rosemarie; Bühren, Christoph (2020-06-04). "Bounded rationality in Keynesian beauty contests: a lesson for central bankers?". Economics: The Open-Access, Open-Assessment e-Journal. 14 (1). doi:10.5018/economics-ejournal.ja.2020-16. hdl:10230/45169. ISSN 1864-6042. S2CID 212631702.
- Alba-Fernández, Virtudes; Brañas-Garza, Pablo; Jiménez-Jiménez, Francisca; Rodero-Cosano, Javier (2010-08-07). "Teaching Nash Equilibrium and Dominance: A Classroom Experiment on the Beauty Contest". The Journal of Economic Education. 37 (3): 305–322. doi:10.3200/jece.37.3.305-322. hdl:10261/2097. ISSN 0022-0485. S2CID 49574187.
- Schou, Astrid (22 September 2005). "Gæt-et-tal konkurrence afslører at vi er irrationelle". Politiken (in Danish). Retrieved 29 August 2017. Includes a histogram of the guesses. Note that some of the players guessed close to 100. A large number of players guessed 33.3 (i.e. 2/3 of 50), indicating an assumption that players would guess randomly. A smaller but significant number of players guessed 22.2 (i.e. 2/3 of 33.3), indicating a second iteration of this theory based on an assumption that players would guess 33.3. The final number of 33 was slightly below this peak, implying that on average each player iterated their assumption 1.07 times.
- Grosskopf, Brit; Nagel, Rosemarie (2001). "Rational Reasoning or Adaptive Behavior? Evidence from Two-Person Beauty Contest Games". SSRN Electronic Journal. doi:10.2139/ssrn.286573. hdl:10230/686. ISSN 1556-5068. S2CID 14073840.
- Kagel, John H.; Penta, Antonio (2021-07-12), "Unraveling in guessing games: An experimental study (by Rosemarie Nagel)", The Art of Experimental Economics, London: Routledge, pp. 109–118, doi:10.4324/9781003019121-10, ISBN 978-1-003-01912-1, S2CID 237752741, retrieved 2022-04-26
- Kocher, Martin G.; Sutter, Matthias (2004-12-22). "The Decision Maker Matters: Individual Versus Group Behaviour in Experimental Beauty‐Contest Games". The Economic Journal. 115 (500): 200–223. doi:10.1111/j.1468-0297.2004.00966.x. ISSN 0013-0133. S2CID 7339369.