| Revision as of 19:58, 10 April 2021 edit99.113.71.3 (talk) Added comments in parentheses for clarification, where the text refers to Euler angles not in the first-second-third order that some readers might assume or expect.← Previous edit | Revision as of 03:16, 26 April 2021 edit undo99.113.71.3 (talk) Added words and link for Cross productNext edit → | ||
| Line 8: | Line 8: | ||
| {{further|rigid body dynamics}} | {{further|rigid body dynamics}} | ||
| If a ] is set at a tilt on a horizontal surface and spun rapidly, its rotational axis starts precessing about the vertical. After a short interval, the top settles into a motion in which each point on its rotation axis follows a circular path. The vertical force of gravity produces a horizontal torque {{math|'''τ'''}} about the point of contact with the surface; the top rotates in the direction of this torque with an angular velocity {{math|'''Ω'''}} such that at any moment | If a ] is set at a tilt on a horizontal surface and spun rapidly, its rotational axis starts precessing about the vertical. After a short interval, the top settles into a motion in which each point on its rotation axis follows a circular path. The vertical force of gravity produces a horizontal torque {{math|'''τ'''}} about the point of contact with the surface; the top rotates in the direction of this torque with an angular velocity {{math|'''Ω'''}} such that at any moment | ||
| :<math> \boldsymbol{\tau} = \mathbf{\Omega} \times \mathbf{L},</math> | :<math> \boldsymbol{\tau} = \mathbf{\Omega} \times \mathbf{L},</math> (vector ]) | ||
| where {{math|'''L'''}} is the instantaneous angular momentum of the top.<ref name=Feynman>{{harvnb|Feynman|Leighton|Sands|2011|pp=20–7{{clarify|date=December 2013}}}}</ref> | where {{math|'''L'''}} is the instantaneous angular momentum of the top.<ref name=Feynman>{{harvnb|Feynman|Leighton|Sands|2011|pp=20–7{{clarify|date=December 2013}}}}</ref> | ||
Revision as of 03:16, 26 April 2021
Wobble of the axis of rotation This article is about the concept in physics. For the term in astronomy, see astronomical nutation. For the term in mechanical engineering, see nutation (engineering). For other uses, see Nutation (disambiguation).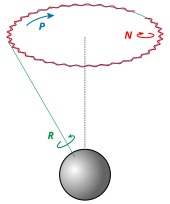
Nutation (from Latin nūtātiō, "nodding, swaying") is a rocking, swaying, or nodding motion in the axis of rotation of a largely axially symmetric object, such as a gyroscope, planet, or bullet in flight, or as an intended behaviour of a mechanism. In an appropriate reference frame it can be defined as a change in the second Euler angle. If it is not caused by forces external to the body, it is called free nutation or Euler nutation. A pure nutation is a movement of a rotational axis such that the first Euler angle is constant. In spacecraft dynamics, precession (a change in the first Euler angle) is sometimes referred to as nutation.
Rigid body
Further information: rigid body dynamicsIf a top is set at a tilt on a horizontal surface and spun rapidly, its rotational axis starts precessing about the vertical. After a short interval, the top settles into a motion in which each point on its rotation axis follows a circular path. The vertical force of gravity produces a horizontal torque τ about the point of contact with the surface; the top rotates in the direction of this torque with an angular velocity Ω such that at any moment
- (vector cross product)
where L is the instantaneous angular momentum of the top.
Initially, however, there is no precession, and the top falls straight downward. This gives rise to an imbalance in torques that starts the precession. In falling, the top overshoots the level at which it would precess steadily and then oscillates about this level. This oscillation is called nutation. If the motion is damped, the oscillations will die down until the motion is a steady precession.
The physics of nutation in tops and gyroscopes can be explored using the model of a heavy symmetrical top with its tip fixed. (A symmetrical top is one with rotational symmetry, or more generally one in which two of the three principal moments of inertia are equal.) Initially, the effect of friction is ignored. The motion of the top can be described by three Euler angles: the tilt angle θ between the symmetry axis of the top and the vertical (second Euler angle); the azimuth φ of the top about the vertical (first Euler angle); and the rotation angle ψ of the top about its own axis (third Euler angle). Thus, precession is the change in φ and nutation is the change in θ.
If the top has mass M and its center of mass is at a distance l from the pivot point, its gravitational potential relative to the plane of the support is
In a coordinate system where the z axis is the axis of symmetry, the top has angular velocities ω1, ω2, ω3 and moments of inertia I1, I2, I3 about the x, y, and z axes. Since we are taking a symmetric top, we have I1=I2. The kinetic energy is
In terms of the Euler angles, this is
If the Euler–Lagrange equations are solved for this system, it is found that the motion depends on two constants a and b (each related to a constant of motion). The rate of precession is related to the tilt by
The tilt is determined by a differential equation for u = cos(θ) of the form
where f is a cubic polynomial that depends on parameters a and b as well as constants that are related to the energy and the gravitational torque. The roots of f are cosines of the angles at which the rate of change of θ is zero. One of these is not related to a physical angle; the other two determine the upper and lower bounds on the tilt angle, between which the gyroscope oscillates.
Astronomy
Main articles: Astronomical nutation and Perturbation (astronomy)The nutation of a planet occurs because the gravitational effects of other bodies cause the speed of its axial precession to vary over time, so that the speed is not constant. English astronomer James Bradley discovered the nutation of Earth's axis in 1728.
Earth
| It has been suggested that this section be split out into another article titled Earth's nutation. (Discuss) (October 2020) |

Nutation subtly changes the axial tilt of Earth with respect to the ecliptic plane, shifting the major circles of latitude that are defined by the Earth's tilt (the tropical circles and the polar circles).
In the case of Earth, the principal sources of tidal force are the Sun and Moon, which continuously change location relative to each other and thus cause nutation in Earth's axis. The largest component of Earth's nutation has a period of 18.6 years, the same as that of the precession of the Moon's orbital nodes. However, there are other significant periodic terms that must be accounted for depending upon the desired accuracy of the result. A mathematical description (set of equations) that represents nutation is called a "theory of nutation". In the theory, parameters are adjusted in a more or less ad hoc method to obtain the best fit to data. Simple rigid body dynamics do not give the best theory; one has to account for deformations of the Earth, including mantle inelasticity and changes in the core–mantle boundary.
The principal term of nutation is due to the regression of the Moon's nodal line and has the same period of 6798 days (18.61 years). It reaches plus or minus 17″ in longitude and 9.2″ in obliquity. All other terms are much smaller; the next-largest, with a period of 183 days (0.5 year), has amplitudes 1.3″ and 0.6″ respectively. The periods of all terms larger than 0.0001″ (about as accurately as one can measure) lie between 5.5 and 6798 days; for some reason (as with ocean tidal periods) they seem to avoid the range from 34.8 to 91 days, so it is customary to split the nutation into long-period and short-period terms. The long-period terms are calculated and mentioned in the almanacs, while the additional correction due to the short-period terms is usually taken from a table. They can also be calculated from the Julian day according to IAU 2000B methodology.
In popular culture
In the 1961 disaster film The Day the Earth Caught Fire, the near-simultaneous detonation of two super-hydrogen bombs near the poles causes a change in Earth's nutation, as well as an 11° shift in the axial tilt and a change in Earth's orbit around the Sun.
See also
Notes
- ^ Lowrie, William (2007). Fundamentals of Geophysics (2nd ed.). Cambridge : Cambridge University Press. pp. 58–59. ISBN 9780521675963.
- Kasdin, N. Jeremy; Paley, Derek A. (2010). Engineering dynamics : a comprehensive introduction. Princeton, N.J.: Princeton University Press. pp. 526–527. ISBN 9780691135373.
- ^ Feynman, Leighton & Sands 2011, pp. 20–7
- Goldstein 1980, p. 220
- Goldstein 1980, p. 217
- Goldstein 1980, pp. 213–217
- "Resolution 83 on non-rigid Earth nutation theory". International Earth Rotation and Reference Systems Service. Federal Agency for Cartography and Geodesy. 2 April 2009. Retrieved 2012-08-06.
- "Basics of Space Flight, Chapter 2". Jet Propulsion Laboratory/NASA. 28 August 2013. Retrieved 2015-03-26.
- "NeoProgrammics - Science Computations".
References
- Feynman, Richard P.; Leighton, Robert B.; Sands, Matthew (2011). The Feynman lectures on physics (New millennium ed.). New York: BasicBooks. ISBN 978-0465024933.
- Goldstein, Herbert (1980). Classical mechanics (2d ed.). Reading, Mass.: Addison-Wesley Pub. Co. ISBN 0201029189.
- Lambeck, Kurt (2005). The earth's variable rotation : geophysical causes and consequences (Digitally printed 1st pbk. ed.). Cambridge: Cambridge University Press. ISBN 9780521673303.
- Munk, Walter H.; MacDonald, Gordon J.F. (1975). The rotation of the earth : a geophysical discussion. Reprint. with corr. Cambridge, Eng.: Cambridge University Press. ISBN 9780521207782.
 (vector
(vector 



