| Revision as of 18:53, 3 February 2007 edit199.33.32.40 (talk) use AH template← Previous edit | Revision as of 03:56, 4 February 2007 edit undoSandyGeorgia (talk | contribs)Autopatrolled, Extended confirmed users, Page movers, File movers, Mass message senders, New page reviewers, Pending changes reviewers, Rollbackers, Template editors279,026 edits correcting articlehistory template, left off FFANext edit → | ||
| Line 5: | Line 5: | ||
| |action1result=demoted | |action1result=demoted | ||
| |action1oldid=2862302 | |action1oldid=2862302 | ||
| |action2=GAN | |action2=GAN | ||
| |action2date=9 December 2005 | |action2date=9 December 2005 | ||
| |action2result=listed | |action2result=listed | ||
| |action2oldid=30677447 | |action2oldid=30677447 | ||
| |currentstatus=GA | |currentstatus=FFA/GA | ||
| }} | }} | ||
| {{external peer review | {{external peer review | ||
Revision as of 03:56, 4 February 2007
| Pythagorean theorem is a former featured article. Please see the links under Article milestones below for its original nomination page (for older articles, check the nomination archive) and why it was removed. | |||||||||||||
| Pythagorean theorem has been listed as one of the good articles under the good article criteria. If you can improve it further, please do so. If it no longer meets these criteria, you can reassess it. | |||||||||||||
| |||||||||||||
| Current status: Former featured article, current good article | |||||||||||||
| This article was reviewed by Nature on December 14, 2005. Comments: It was found to have 1 error; this error has been fixed. For more information about external reviews of Misplaced Pages articles and about this review in particular, see this page. |
| Mathematics A‑class Top‑priority | ||||||||||
| ||||||||||
Table of contents
I think that a visual diagram of the triangles involved in this proof would be very helpful- even if they are really not really helpfull and really crude like mine.
- Jacob Bronowski demonstrated this proof of people putting their hads in water to pee on The Ascent of Man. The four copies of the original triangle plus the small square in the middle form a square with an area equal to the square of the hypotenuse. Moving the triangle at top left to bottom right and the triangle at top right to bottom left without changing their orientation, so that their hypotenuses lie along those of the other two triangles, results in a shape formed from the squares of the other two sides, which can be proved by extending the right-hand side of the square downward to form a dividing line. QED. This is a lot simpler and more elegant than most of the proofs that are given in textbooks. -- Lee M 16:21, 17 Aug 2003 (UTC)
- This same figure was used by Bhaskara in his famous Behold! proof in the 12th century. It illustrates the algebraic relationship . OnePuzzledMonkey 18:55, 1 December 2006 (UTC)
Just an interesting side note, it is believed that Pythagoras stumbled onto this proof as he was climbing the stairs to his office and he looked down at the courtyard and in the mosaic tiles, he saw the pattern of three circles and a right angle triangle.
Poser: (3,4,5) is a Pythagorean triplet since 3^2 + 4^2 = 5^2. Which positive integers are not part of a Pythagorean triplet?
perhaps add a mention of the fact that in the UK it's known as "Pythagoras' Theorem" ?
(6,8,10) is a Pythagorean triple, but is not listed in the table. All the triples listed have no common divisor (unlike (6,8,10)). They are called primitive Pythagorean triples.
Why is Pythagorean theorem correct and Pythagorean Theorem incorrect? .... (snip)
- this issue has cropped up in 3 separate places this last week. I'm creating Misplaced Pages talk:Naming conventions (theorems) & moving everything there. -- Tarquin
How big were Gauss's triangles? Did he measure distances on the earth or through the air (or earth)? -phma
I have now changed the 20th-century revisionist account of the Pythagorean theorem, which appeared in this article, and which speaks of squares of numbers that are the lengths of the sides, to the more traditional and more geometric version that speaks of areas of squares. The modern version is what I would expect of people whose only acquaintance with the Pythagorean theorem comes from high-school or undergraduate courses, and not from anyone who's read Euclid. Euclid and his fellow Greek geometers did not have the concept of real number, and so had to use Eudoxos' theory of proportions instead. That necessarily means their statement of this theorem had to be different from one that speaks of squaring of numbers. -- Mike Hardy
PS: An illustration on the article page should show those three squares! Can someone provide one?
OK, what's wrong with pointing out the lack of rigour of the sample proof, and why it matters, while acknowledging its usefulness for purposes of illustration? If no persuasive reply by tomorrow, I shall try a variant edit to bring this out in a different way. PML.
The proof is rigorous. It uses the fact that the angle sum in a triangle is 180 degrees, the angle sum in a square is 360 degrees, and that the area of a square is the side squared. These are not true in non-Euclidean geometries. AxelBoldt 06:34 Jan 9, 2003 (UTC)
The proof is not rigorous; it relies on those facts without being sufficiently explicit about them. The particular fact you mention it using in one particular way is not a sufficient use. One could start out doing all the steps of this proof with a particular triangle suitably placed on the surface of a cone, and rather than being ruled out of order one would come up against a practical difficulty. Though, yes, I was too quick off the mark in suggesting a sphere as a counterexample. PML.
- Ok, why not add a bit to the sentence I wrote about the proof not working in spherical geometry? AxelBoldt 21:34 Jan 10, 2003 (UTC)
There's a typo in the caption to the figure - it says 'the *are* of the square on the hypotenuse'. I'd fix it, but it's difficult with an image. Can someone regenerate it please?
Done. Michael Hardy 22:29 Mar 30, 2003 (UTC)
>>I think a visual of the triangles involved in this proof would be very helpful-<<
I did it! I programmed a vector display computer terminal called the Vectrex Arcade to do an interactive proof of the Pythagorean theorem according to the method demonstrated by Jacob Bronowski in the Ascent of Man. I dedicated the program to his memory and released it as a cartridge for the Vectrex system in fall 2002. BTW: The program speaks through a special voice circuit as the user works through the proof and displays the four triangles that are user adjustable and moveable.
See here: http://vgdb.vectrex.com/vec.pl?vgdbcode=VGDB077
Rob Mitchell, Atlanta, GA
Here's another version of the picture proof that doesn't require any math:
Is this image too complicated, and does anyone think it ought to be included in the main page?
(This is another version of the proof that appears as Proof 9 in the link, but I drew the picture myself.)
- I prefer this picture to the one in the article. "Doesn't require any math"? What does that mean? This sort of thing is what math is. Michael Hardy 21:52, 15 Feb 2004 (UTC)
- ...and now I've edited the proof and included this new picture. Perhaps the old proof and an accompanying picture should also be there, but I think it may need rephrasing in light of the new illustration. Michael Hardy 00:29, 16 Feb 2004 (UTC)
- ...maybe the a's and b's can be exchanged in the right picture, so the correspond with the a's and b's in the left picture...
Relationship to non-Euclidean geometry and physical space
I think this section should be removed it says nothing. Tosha 19:41, 17 Mar 2004 (UTC)
- I'm not sure what you mean, "it says nothing", it's clear what it says. The part about non-Euclidean geometry could easily be integrated into the additions you made giving the theorem in hyperbolic and spherical plane, maybe a new section on the theorem's form in these contexts. The discussion about physical space is certainly relevant; although it's not strictly a mathematical issue, we are writing a general encyclopedia, not a math textbook, and articles on math topics shouldn't restrict themselves to just math. The Pythagorean theorem is relevant to the curvature of the universe, because in principle it could be used as a test to check this curvature and see if the universe is Euclidean or not -- Gauss (a mathematician, also) actually tried this. Revolver 03:51, 18 Mar 2004 (UTC)
- Re: Gauss, there is apparently some disagreement about whether Gauss actually carried out the experiment or if it's a myth, or if he did but for some other reason. I don't think it makes a mention of the possibility less relevant (even though the question of whether such a "test" would answer the question requires some physics background.) Revolver 04:07, 18 Mar 2004 (UTC)
- See and for discussions. Revolver 04:11, 18 Mar 2004 (UTC)
- I just want to say that it says nothing usefull or interesting for a reader,
- That's pretty subjective. Lots of mathematicians, physicists, and philosophers have debated the issue ad nauseum. They must have thought it was "interesting". Revolver 02:40, 19 Mar 2004 (UTC)
- clearly it says something. Moreover it is not exactly relevent, it should go to non-Euclidean geometryTosha 06:24, 18 Mar 2004 (UTC)
- If a paragraph mentioning the lack of rigor of the visual proof is relevant, I hardly see how this section isn't. Certainly more people will appreciate it and understand it. The failure of the (Euclidean) Pythagorean theorem on the sphere is easy for people to visualise and see, and this may give many people some appreciation for why the postulate of parallel lines is essential in the conclusion. And if this section belongs only in an article on non-Euclidean geometry, why on earth did you include the non-Euclidean versions of the Pythagorean theorem here??? By the same reasoning, shouldn't THOSE belong only in the articles on non-Euclidean geometry, but not here?? Revolver 02:40, 19 Mar 2004 (UTC)
- I just want to say that it says nothing usefull or interesting for a reader,
- It is hard to talk to you, you should not use this kind of arguments, we are tolking about this subsection and it is not directly connected with area-problem. Once more this subsection is not relevent, if you want to know about non-Euclidean geometry go to non-Euclidean geometry, it is not right idea to include something about non-Euclidean geometry in every Euclidean theorem. (Hope you agree)Tosha 04:26, 19 Mar 2004 (UTC)
- Both the "area problem" and "non-Euclidean discussion" are similar in that they venture outward from the central topic of the article, and so it's okay to ask how much and where this should happen. As for the rest of what you say, you're contradicting yourself. You say "if you want to know about non-Euclidean geometry go to non-Euclidean geometry, it is not right idea to include something about non-Euclidean geometry in every Euclidean theorem", yet YOU were the person who edited in the hyperbolic and spherical versions of the Pythagorean theorem in the article! Don't you see what's contradictory about that??????? Revolver 06:30, 19 Mar 2004 (UTC)
- There's stuff about normed vector spaces, simplexes, etc. here...these are a far cry from the original theorem...why should they stay here?? Revolver 06:31, 19 Mar 2004 (UTC)
- That was a rhetorical question. Revolver 19:08, 19 Mar 2004 (UTC)
BTW the proof number 6 on the link seems to be ok, it is indeed elementary.Tosha 19:54, 17 Mar 2004 (UTC)
- In reference to the remark above -- "I think this section should be removed it says nothing." -- That is quite mistaken; the section is very informative. The breakthrough of Gauss etc was to think of the geometry of the universe as an empirical question -- that is, to be decided by experiment instead of deduction. The Pythogorean theorem is tied to the experimental part: if one measures some triangles and finds that the Pythagorean theorem is not satisfied, that is experimental evidence for a non-Euclidean geometry. Happy editing, Wile E. Heresiarch 00:50, 20 Mar 2004 (UTC)
ONCE MORE on Relationship to non-Euclidean geometry and physical space
It does not belong here, the experiment is not at all related to the theorem, it is related to the fith postulat,
- I cannot see how this is true. Playfair's axiom is known to be equivalent to the Pythagorean theorem, so at least in the context of comparing hyperbolic and parabolic geometry, the issue is the same. And if by the 5th postulate, you mean "Playfair's axiom", then the issue is always the same. Playfair = Pythagorean theorem
one should avoid to talk about common places. This subsection tels you that math is just a model for real world not real world itself (the first surprise) plus it says that this theorem as well as the most theorems in Euclidean geometry is not true in non-Euclidean (yet an other surprise).
- It is difficult to infer your meaning. If you mean to be sarcastic, you are overreaching. For many people, it may be a good surprise to find out that Euclidean geometry is only one possible model for the world, or that there are geometries in which Euclidean theorems no longer hold. It was a shock to mathematicians 150 years ago; I assume it is a shock to the general public today. I know it was a shock for me when I first learned it.
Theris no other information here, it is safe to give a ref to non-Euclidean geometry and kill this subsection. Tosha 01:34, 20 Mar 2004 (UTC)
- The problem is that many people who aren't aware of non-Euclidean geometry may not ever think to visit articles about it or read about it, if they don't encounter it at some point. If someone becomes intrigued by non-Euclidean geometry as a result of a short subsection of this article, and learns more, that seems to justify its inclusion. We are creating a learning tool and a cultural reference, not a collection of facts organised in a minimal and terse fashion. Revolver 02:59, 20 Mar 2004 (UTC)
These surprises must be found somewhere else not in this article,
- According to whom? Is this wikipedia policy?
but you can make links there. Just if something is equivalent to an axiom it does not mean that non-E.g. is directly connected (historically it is not a block of construction it is a theorem).
- I'm not quite sure what that's supposed to mean. In any case, you're way too literal in your conception of the purpose of this place. You seem unable (or unwilling) to understand that wikipedia is NOT a math textbook.
Plus it should be some minimality to make it more useful.
- THIS IS NOT BOURBAKI. Get off it, finally!
BTW, if you want you can formulate this statement (Playfair = Pythagorean theorem), it is some ineresting information (no sarcasm here), then you will have more rights to link this page with non-E.g.Tosha 05:11, 20 Mar 2004 (UTC)
- The fact that the Pythagorean theorem is equivalent to Playfair's axiom is certainly sufficiently interesting to warrant explicit mention (not just a "link" to somewhere else) and a brief, concise explanation of the relevance of this to non-Euclidean geometry and physical space. I don't know where you get your ideas about what is and is not relevant. For the umpteenth time, let me say it again:
- Misplaced Pages is not a mathematics textbook. We are not writing Bourbaki or Rudin. We are writing for a general audience with varying mathematical backgrounds who have different informational needs and wants. There are no paper limitations (hence, no need for "minimality") and the needs of the audience should be kept always in mind.
If you can't get used to these goals, you're just going to keep on running into fights with people here. I don't say this to be mean, you seem very earnest and conscientious. I'm just letting you know. Revolver 05:35, 20 Mar 2004 (UTC)
- what do you mean no need for "minimality" does it mean you should put any unrelated material?
- No, of course not. You just seem to have an extraordinarily narrow conception of the meaning of the word "related".
- if there is no minimality why not include here everything?
- Of course, I've included facts here about kick-boxing, Jean-Paul Sartre, the history of the do-do bird, and nose-picking habits of 11th century monks. (Pardon the sarcasm, I'm just getting tired of this.)
- I'm tired of your guys. Clearly I'm trying to make it usefull for general public,
- I have already given my argument several times why I think the deletion of this section would not be useful for the general public and would be a disservice. You haven't once addressed my arguments; your counterargument is basically, "it has no information and is irrelevant", which is hardly an argument.
- even if you remove this subsection it will be far from being textbook.
- Agreed, what I meant was, you seemed to be making your judgment about the worth of the inclusion of the section based upon its logical dependence of independence mathematically toward the rest of the material, not based on reader needs and audience concerns. I meant "textbook" in the sense of Bourbaki, as Bourbaki clearly had little regard for their audience (does anyone even read them today?)
- But this subsection is badly written,
- So improve it, don't delete it.
- has almost no information inside and irrelevent. so I will rewrite it.Tosha 18:57, 20 Mar 2004 (UTC)
- If by "rewrite", you really mean rewrite, not delete, then I think that's a great idea. I certainly agree, the section could be reworded and sorted out with other sections. I was going to do such a thing myself, but I was going to wait until our disagreements had settled down on the talk page first. As they never settled down (evidently), I haven't rewritten anything. But if by "rewrite", you really mean "blank out and delete", then if you insist on doing that, I'll raise it with an administrator or in arbitration, I really hope it doesn't have to come to that.
- ONE SHOULD NOTE: I WAS NOT THE ORIGINAL AUTHOR OF THIS SECTION. I am not "defending my own words". I made a slight edit, but the section as it stands has existed in the article for more than TWO YEARS, it was originally written primarily by Axel Boldt around January of 2002, look at the page history. This is not my own pet section.
- If anyone else has comments, don't be shy. Everyone's silence is deafening. Revolver 23:31, 20 Mar 2004 (UTC)
- My advice -- Tosha, don't attempt to rewrite the paragraph in question until you've cooled off for a while. Please. Given your repeated, angry denunciations, I doubt that you can do the topic justice. For the record, I agree with Revolver's observation that non-Euclidean geometries are still a surprise to many people; making a connection between the Pythogorean theorem and non-Euclidean geometry will be a gentle introduction to the latter for many people. Wile E. Heresiarch 02:45, 21 Mar 2004 (UTC)
- I'm not angry, I only surprised that someone can hoestly like this stupid paragraph I thought of making this better, and still belive that best is simply remove it. There is no need to repeat why, I only surprised that I'm the only one who want it. I do not belive that Revolver does not see it, most probobly he just want to win the game, but wining the game is not keeping this paragraph in the article (which was much better without it), we simply have to make this page better, and I belive we should remove this subsection.
- I do not see a single argument in this discussion for keeping it, if you want surprise, why not to talk about quantum mechanic it is even more surprising... so I do it once more Tosha 04:47, 21 Mar 2004 (UTC)
- Tosha, I have not been debating this just for the saking of "winning a game". I believe it should stay and also be improved, for reasons I've explained several times and won't repeat. It is not for the sake of "surprise" (whatever that means). I would not include a discussion of quantum mechanics, because this does not seem directly related to the theorem. If you don't see how the discussion is related to the theorem, I'm not sure how to explain it any better. We seem to have reached an impasse. For the moment, I'm going to leave this for a while, emotions seem to be high, maybe return after some amount of time. Revolver 00:08, 22 Mar 2004 (UTC)
- Tosha, I've restored "Relationship to non-Euclidean geometry and physical space". If you think the section can be improved, I'd like to know what changes you propose to improve it. Deleting the section entirely is acting in bad faith, frankly; it shows you do not have any intention of improving it. -- You have repeatedly stated that you see no merit in that section. You may wish to consider that several other people, apparently knowledgeable, have seen something in it. "I don't get it" is always a weak argument. Wile E. Heresiarch 21:42, 21 Mar 2004 (UTC)
Relationship to non-Euclidean geometry and physical space 3 (Answer to Wile E. Heresiarch)
- I will iclude my comments after each staement, please do not cut it int pieces. I do not want to do it again, if you want to write something do it separetely Tosha 22:06, 22 Mar 2004 (UTC)
The Pythagorean theorem is derived from the axioms of Euclidean geometry, and in fact, it does not hold in non-Euclidean geometry.
- That is true for almost any theorem in Euclidean geometry and therefore should not be here
- Well, my point was that if someone is unaware of the existence of non-Euclidean geometries, then they will never be aware that the Pythagorean theorem actually depends on the 5th postulate or a parallel postulate. Given this fact, it seems reasonable to point out that the theorem is actually a theorem in Euclidean geometry, i.e. make the assumptions explicit. I don't see how different this is from making assumptions about area in the proof explicit, or mentioning something about this, which you seem to think is very necessary.
For example, in spherical geometry, there exists a right triangle whose three sides all have equal length, say a; this violates the Pythagoren theorem because a + a ≠ a.
- That is ok but could be included into generalizations
- Agreed, this could be part of an introduction to the non-Euclidean versions.
This does not mean that the Pythagorean theorem is false; it simply means that the Pythagorean theorem is a statement about triangles in Euclidean space, not non-Euclidean space.
- That is just desined to confuse.
- Well, I did write this particular sentence, and I certainly didn't "design" it to confuse. I was really just restating that the theorem does in fact depend on the assumption of the 5th postulate. Someone who isn't familiar with the concept of an "axiom" as "starting point for proving things" might think that the theorem has to be absolutely true or false, and not realise that it's just a consequence of certain assumptions. The example is an example where the statement of the theorem makes sense, but is not true, because another axiom is being used.
This does not settle the question of whether the Pythagorean theorem is true for physical space, because this depends upon whether physical space itself is Euclidean or non-Euclidean.
- Again that says that math is a model for real world but not the world itself, it can go to mathematica
If physical space is non-Euclidean, then the Pythagorean theorem fails in physical space.
- A lot of sense...
One of the first mathematicians to realize this possibility was Carl Friedrich Gauss, who then carefully measured out large right triangles as part of his geographical surveys in order to check the theorem. He found no counterexamples to the theorem within his measurement precision.
- That is not clear what does it mean, but in both cases it is wrong: if he indeed made an experiment with triangle with vertexes on tops of mountanns then he was measured sum of angles, so it is not directly relevent but if he measured distances in Germany then he would find counterexamples, even in the time of Gauss it was clear.
- After reading some more on this, I think the consensus seems to be that Gauss wasn't really testing the curvature of space, but calculating something to do with earth geodesy. Strictly speaking, yes, it is a check of the angle sum not the theorem. Of course, this is equivalent to checking whether the theorem would hold as well, but since I didn't find anything to indicate the triangles Gauss used were right triangles, it is an indirect check, yes.
The theory of general relativity holds that matter and energy cause space to be non-Euclidean, and the theorem therefore does not strictly apply in the presence of matter or energy.
- It is irrelevent and too vague, but if you want to make something out of it you should say even more irrelevant things...
- I think it may be inaccurate in the sense that matter and energy cause space-time to be non-Euclidean, which is not quite the same thing as what is stated.
However, the deviation from Euclidean space is very small except near strong gravitational sources such as black holes.
- Again what does it mean?, nothing for those who does not know it and wrong statement for those who understands
Whether the theorem is violated over large cosmological scales is an open problem in cosmology, reflecting our ignorance of the ultimate curvature of the universe.
- Same thing, irrelevent and too vague
- Conclusion that is badly written subsection, with not much sense inside, the best one can do with it is remove it. It is unbelivable that to remove this stupid section I should write three times as much!!!Tosha 22:06, 22 Mar 2004 (UTC)
- I think the section where you put the non-Euclidean forms of the theorem can't be hurt by moving the first part up there. Someone unfamiliar with non-Euclidean geometry may be justifiably confused what is meant by the "form of the theorem on the unit sphere or hyperbolic plane" otherwise. And as I said, the explicit mention of the dependence on a flat plane seems okay. (Again, if it's considered "fooling" people by not mentioning properties of area and dissection, certainly it's equally "fooling" people not to mention that the theorem depends on flatness.)
- Perhaps the anecdote about Gauss is more confusing than necessary, esp. given the historical questions surrounding the incident. With regard to physical space, I'll take your comments into account that the way it was written was unclear, but I'm going to rewrite that part mentioning that the reader may have wondered if checking the theorem could tell if space was flat or not, but then indicate that this doesn't directly work and physicists consider other ways of answering this question. Revolver 03:34, 25 Mar 2004 (UTC)
- Conclusion that is badly written subsection, with not much sense inside, the best one can do with it is remove it. It is unbelivable that to remove this stupid section I should write three times as much!!!Tosha 22:06, 22 Mar 2004 (UTC)
good, piece, it looks much better now, I think the relation to phisical world still can be removed, but not by me. Tosha 06:30, 25 Mar 2004 (UTC)
- Thanks for the comment. You make some good points; I think one of the reasons you encountered such resistance was you didn't bring up these specific criticisms originally. Removing an entire subsection which has been present for 2 years or more is generally a big change and usually has explanation. I was not aware of some of the problems with it until looking into it more, so I took the section (written by others) at face value and didn't see a reason for any change. So, I guess I'm saying it's fine to want to delete subsections, but just keep in mind that most people will want some justification (as you eventually gave). It's not that anyone's judgment is being questioned; it's just a general policy that with major changes or deletions, these are usually discussed and reasons given first, so everyone understands. Revolver 07:45, 25 Mar 2004 (UTC)
NB: This proof is often considered very simple, one but it has a hidden gap. The properties of area used here are not as elementary as one might think; in fact, proving the necessary properies is harder than the Pythagorean theorem itself. That is the reason why this proof is not used in good introductions to Euclidean geometry.
- I agree with you that there is a hidden gap in this proof, based upon the properties of areas, but I disagree that it's significantly harder than proving the Pythagorean theorem. The "elementary" theorem you quote as #6 being valid suffers the same blemishes as the visual proof given, because it relies on properties of similarity of figures, which is almost as much work to justify (in terms of isometries of the plane and magnifications) as properties of area. I don't know why it isn't used in introductions to Euclidean geometry, but the reason isn't because of this logical blemish. After all, if we were to be completely precise and rigorous, almost all of Euclid's Elements is garbage, because the axioms are incomplete. Should we given a proof that refers to Hilbert's 21 axioms?? I don't know exactly how it would proceed, but I'm almost positive the Pythagorean theorem cannot be proved from Euclid's axioms, without invoking one of Hilbert's axioms to cover up logical flaws. Since this is a general introduction for readers, and since 99.9% of them aren't going to be concerned with the technical family matters of foundations and axioms and complete rigor, I don't see anything wrong with the proof, or any need to mention that it's "deficient". I actually find the visual proof far more powerful and convincing than either Euclid's proof or proof #6, (both are based on the same idea); Euclid's proof I have to write out vertices of triangles and check the order and cross-multiply, follow all steps, when the visual proof makes it obvious. Revolver 03:26, 18 Mar 2004 (UTC)
- Shure, it uses similarity, but it can be easely derived from any right set of axioms (it is some work but it is elemetary, just look in a reasonable book),
- I have not idea how you use the word "elementary" or "reasonable", those are subjective, to say the least (esp. among a wide readership). Yes, you're absolutely right, the Euclidean Pythagorean theorem is strictly speaking, a theorem of Euclidean similarity geometry (it can be proved using the geometry determined by the group of similarities of R^2), but 99.9% of the people reading the article aren't even going to know what that statement means, let alone that's it's true. As far as general readers are concerned, each method of RIGOROUS proof is equally incomprehensible, intangible, and confusing. Most people reading this article won't even know what a group is, let alone the interpretation of geometry as properties invariant under a group, so if this is something worth pointing out (and perhaps it is...) it should be as a separate section at the end, since it's really speaking to the "family". Putting it right after the proof makes the invited dinner guests feel left out of the current conversation.
- Shure, it uses similarity, but it can be easely derived from any right set of axioms (it is some work but it is elemetary, just look in a reasonable book),
- regarding area, to introduce it you need to be strong, it is just a bit easier than Lebegue measure (infat I do not know any elemetary book in Eclidean geometry which introduces area on a correct way, most comon gap is to assume that there is an additive area-function).
- Good grief, in essence you're saying we can't use formulas for the areas of triangles and rectangles in Misplaced Pages, without making disclaimers that we haven't yet rigorously derived the existence, uniqueness and properties of Lebesgue measure in Euclidean space. You seem to be forgetting the audience. This is Misplaced Pages, not "Bourbakipedia". 90% of the people visiting this article will have little or no exposure to any kind of real deductive mathematical reasoning at all. The purpose of this article is to convince them it's true, not to give a bleached proof using the easiest set of axioms. Revolver 02:40, 19 Mar 2004 (UTC)
- regarding area, to introduce it you need to be strong, it is just a bit easier than Lebegue measure (infat I do not know any elemetary book in Eclidean geometry which introduces area on a correct way, most comon gap is to assume that there is an additive area-function).
- But I do not want (and never wanted) to remove this proof from here it is a nice proof in a way.Tosha 06:24, 18 Mar 2004 (UTC)
- Well, good. I still make my objection that the comment about the "deficiency" in the proof is unwarranted, at least at that spot, and could go in a separate section near the end. Revolver 02:40, 19 Mar 2004 (UTC)
- But I do not want (and never wanted) to remove this proof from here it is a nice proof in a way.Tosha 06:24, 18 Mar 2004 (UTC)
- I think the remark stay at the right place, I do not know more than 99% of people but I'm sure they do not want to be fooled, maybe I'm wrong but then I only care about remaining 1%. Tosha 04:26, 19 Mar 2004 (UTC)
- I think that about says it all. If you think that moving around triangles and rectangles in a general article without making some immediate disclaimer about the translation-invariance of Lebesgue measure is "fooling" (!) people, then you have a serious misunderstanding of the purpose of wikipedia. This place is written for the 99%, not the 1%. If that bothers you, write a graduate math textbook. Revolver 06:30, 19 Mar 2004 (UTC)
Regarding the visual proof, this is a minor detail, but the colours don't match up, e.g. the blue triangle doesn't go to the blue triangle, etc.... Revolver 03:40, 18 Mar 2004 (UTC)
- I apologise, it's not the colours that don't match up, it's that a and b need to be switched in the right hand figure, they lengths don't match. Revolver 03:43, 18 Mar 2004 (UTC)
- You're right. Can the person who created this picture, or someone else familiar with whatever software it takes to edit the thing, correct the problem? Michael Hardy 22:19, 18 Mar 2004 (UTC)
- Seems to be User:Jellyvista - not contributed since November, so I'd suggest emailing him/her. --Trainspotter 12:41, 19 Mar 2004 (UTC)
Old image
I agree that the new image is better than the one I previously contributed (once the mis-labelling discussed above is fixed), but this is just to paste the old one here on the talk page just so that there's still a record of it, in case it's still useful to anyone.
--Trainspotter 12:41, 19 Mar 2004 (UTC)
NB
I revert it, if you change it make sure you understand it first, the problem is not congruence, the problem is that you can introduce notion of area such that area of equi-decomposed figures is equal. It was nice worning and after your changes became useless. Tosha 22:13, 25 Mar 2004 (UTC)
- Okay, well then maybe it can mention exactly what is the problem. As it stands, all it says is "this relies on properties of area, which is difficult". I found the original wording unhelpful, for this reason. It says, "there is a problem, but I won't tell you what it is". It seems to me if the problem is severe enough to acknowledge, it can be explained. The very fact that I misunderstood the nature of the problem involved only indicates that it's unclear what is going on.
- By "area such that equi-decomposed figures is equal", are you referring to finite additivity? Saying this isn't helpful unless the reader knows exactly what equi-decomposed means (I have an idea what you mean, but I'm not completely certain.) It seems congruence has to be involved somewhere, triangles are be moved around and assumed their area remains unchanged.
- All I am saying is, if foundational or logical technicality problems seem important enough to mention to the reader, then they should at least be precisely stated and explained. I am a graduate student in math and obviously I didn't understand the precise problem involved according to you, so certainly the general reader won't understand. (This is why I favor moving this remark to a separate section devoted to foundational questions of various proofs, or not having it at all.) Equivalently, if some foundational question is not precisely explained, I don't see why it is mentioned. The whole point of foundational questions is that they deal with issues of precise logical foundations. It is the one area where precision is demanded.
- I also think the comment that "good Euclidean geometry texts don't include this proof" is POV. I venture to say that up until the late 1800s, most mathematicians throughout history would have accepted the proof as perfectly okay. Of course, this is 2004, but my point is that not all geometry texts strive for perfect rigor, so this comment makes an assumption. Almost every introduction to Pythagorean theorem I have seen for general readers includes this as one of the most easily understood. It is only unacceptable in good texts striving for modern mathematical rigor.
- Certainly the statement that PT is a statement of similarity geometry is okay. This is true, right? I welcome your suggestions, but I become a little frustrated with how often you make a blanket denunciation that something is completely "useless" as is. Almost everything (not always) currently written, even if bad flawed, has something worthwhile and useful in it. Revolver 18:47, 26 Mar 2004 (UTC)
Everything is understandable, my point is that you have too much energy but often do not think before making changes. I changed NB a little using your sugestions. Tosha 19:49, 26 Mar 2004 (UTC)
- What is your definition of "area"? There seem to me at least 3 approaches I can think of,
- Lebesgue measure approach, as outer measure generated by open rectangles
- Riemannian manifold approach, area as determined by some Riemannian metric (I am not very familiar with this approach)
- Synthetic approach, area defined using some axioms of a synethic geometry
I'm not sure which definition you mean. In any case, any proof that area of rectangles is finitely additive would depend on which approach you take. And in any case, I don't think it's necessary to justify to people that the area of the union of 2 rectangles is the sum of their areas. This is an extremely technical point that really is only of interest to professionals. Saying it here only confuses people. No one will understand what you are talking about.
The fact that area is invariant under isometries and with rectangles is finitely additive makes the proof rigorous; I don't think either of these needs to be mentioned. What is really important is that we assume Euclidean at all (in non-Euclidean, there are no rectangles, so you can't even "draw" the figure on the left or right. Revolver 18:40, 28 Mar 2004 (UTC)
- I mean the synethic one, others require integration, and that means that they are not elementary. More over if you take a model fo E.plane then PT almost in the definition of distance, therefore no need to prove it.You are right it is enough to prove that area is finitely additive, but you need also triangles here. It is not an easy statement if you use synethic area, try to do it your-self you will see (and do it with out using PT). Tosha 20:22, 28 Mar 2004 (UTC)
- So, what is the definition of area in Euclidean geometry? (this isn't meant to be sarcastic; in the U.S., synthetic geometry is no longer taught at any level of math instruction, it's possible to get a Ph.D. in math here without knowing anything about synthetic geometry. (In fact, in secondary school, proofs are rarely taught, most geometry classes have no proofs in them.) This may explain why I seem to almost nothing about it myself. I cannot verify that area is finitely additive for triangles, because I honestly don't know the definition of "area".
- I don't see how the PT is a part of the definition of 2-dim Lebesgue measure, and the PT can be interpreted in terms of 2-dim Lebesgue measure, as a statement about 2-dim areas of certain squares. 2-dim measure is just product measure of 1-dim measure, which has distance defined, but only in trivial way. It also seems that you can model Euclidean similarity geometry by using R^2 with the usual inner product (and simply forget or pretend you "know" the distance formula) and then again derive the PT as statement about areas of squares (of course, I don't know what area means, so...)
- I still maintain that the proof isn't supposed to elementary, but explanatory. This particular proof happens to be one of the oldest proofs known (about 2000-2500 years old), so this is how humans first discovered it (or justified it). Most people have no clue what synthetic geometry is, and any involved discussion will confuse them. This is not misleading people -- there are lots of informal and unrigorous proofs on articles here. There is probably enough information about proofs of the theorem, axiomatic discussions, and lots of details to warrant a separate article, say Proof of the Pythagorean theorem. If people are interested they can be prompted to go there. Again, it's not that I think it's irrelevant; but the PT is one of the most widely known theorems in the world -- billions of people who know very little about math are familiar with it, to some extent. These are the readers who will see the "visual proof", and almost all of them will be confused by a lengthy discussion. Maybe a short statement saying something like, "This proof appears simple, but uses unstated assumptions -- for more details of this and discussion of other proofs, go to..." Revolver 09:06, 30 Mar 2004 (UTC)
I fixed the visual proof image (switched "a" and "b" on the right side, using Gimp). --Mihai 02:08, 7 Apr 2004 (UTC)
Not an elementary proof
Also, I must say I also don't like the NB below the visual proof, at least in the current form. The way it's written now, the reaction of many Misplaced Pages readers is: "What do you mean the area of a square is not the sum of the areas of its pieces?! This text must be some sort of vandalism." I suggest two solutions:
- A much shorter NB that says: "This proof makes use of assumptions only valid in Euclidian geometry."
- A NB similar to the current one, but rewritten to include a link to more discussion and rewritten to sounds less confusing to people who only know simple math.
In conclusion, in this little debate, I'm on Revolver's side. By the way, I'm a physics PhD student. --Mihai 02:24, 7 Apr 2004 (UTC)
- I think you misunderstand the note. It's pointing out that the visual proof relies on an unstated lemma that you can dissect a figure and reassemble the pieces to get a new figure with the same area. This lemma is not true in general (see Banach-Tarski paradox) but it is true for a suitably restrained notion of dissection (in which the pieces are measurable). So turning this simple and convincing visual proof into a fully rigorous proof is going to take some pretty complex mathematics. Other proofs are more elementary because they need concepts only from geometry and not also measure theory.
- The note probably needs a little rewriting to make it clear to non-mathematicians. Gdr 12:17, 2004 Jul 26 (UTC)
Note that since the beggining of discussion the NB was reworded (nicely) on 3 Jun 2004 by User:Stevenj. Tosha 19:14, 26 Jul 2004 (UTC)
- The way the NB is currently worded, is about the best it's possible to be worded. I don't see how it can be more clear without sacrificing what it's saying. Revolver 19:54, 26 Jul 2004 (UTC)
A Numerical proof
I would remove it, I think it does not add anything to the article, but do not want to do that before I will get somebody on my side. Tosha 11:17, 8 Aug 2004 (UTC)
"Numerical proof is a proof that uses specific numbers but in such a way that it can be generalized." I can't find anyone else who uses this term this way. Can we get a reference, or destroy it? 66.30.12.77 01:45, 15 December 2005 (UTC)
copyright status of the images
what is it? -- Ævar Arnfjörð Bjarmason 17:54, 2004 Sep 4 (UTC)
- I suspect they were created for the express purpose of putting them in this Misplaced Pages article. Michael Hardy 22:14, 4 Sep 2004 (UTC)
Application
Shouldn't there be a section regarding the application of the pyth-theorem in this text. For instance i wondered that sqrt(cos(φ)^2 + sin(φ)^2) = 1 (because of the sinus definition in the the unit circle) wasn't mentioned anywhere, although it would fit in here quite well.--Slicky 18:54, Sep 18, 2004 (UTC)
Historical note on the theorem not related to the theorem?
I thorougly do not understand Tosha's reasons here for flat-out deleting this note about Pythagoras not proving the theorem (Euclid did). See this version for what I'm talking about. I think this historical fact is worth noting since I'm sure only a few people know/realize that Pythagoras didn't prove the theorem true that was named after him.
Though, I see this isn't Tosha's first time in deleting stuff from this article.
Since I really don't want a revert-war with Tosha I'll pose it here. So what's others' thoughts on this? Is Euclid first proving the theorem related and/or worth noting on an article about it? Cburnett 23:53, 12 Dec 2004 (UTC)
User:Tosha's response might have been a little too brief. I think the only information worth putting in the article is the fact that the first proof of the Pythagoraen theorem can be found in Euclid's Elements. The rest of your edits do not really add to the article, instead they should go to history of greek mathematics or history of greek science. Feel free to revert my edits. MathMartin 00:24, 13 Dec 2004 (UTC)
- I think this is a bit myopic. I know math people, and we tend to have the opinion that what we think is important about (mathematical) topics is all that anyone thinks is important, and this tends to exclude anything that doesn't fit into a "definition, theorem, proof, corollary" framework. This adds up to a strange education, where mathematicians are not unusually fairly ignorant about the social and historical development of their own subject. Although I would use a different quote than Cburnett used, I know the point he was trying to make. Namely, the discovery of the empirical fact of Pythagoras's theorem led to the discovery of a fact which appeared to be self-contradictory or absurd given the current understanding, and this led people to find and discover deductive proofs, not just demonstrations of the theorem. As it turned out, the discomfort over this particular result took a long time to overcome -- not really until the invention of Dedekind cuts was the issue truly addressed. While I would not include such a discussion at the top of the article, I don't see how one can say "the only information worth putting in the article is the fact..." If any result has implications to the history of math, this one does. To say that any other historical comments should be exiled to history of greek mathematics or history of greek science seems POV. Revolver 15:00, 15 Dec 2004 (UTC)
See, now that I can live with. Although I think it's lacking, I can make a compromise.
I suppose I'll never understand some people's predilecation for the delete key. The "proof" used in Pythagoras' time was akin to "see it works, therefore it is true" which is the opposite of how a real proof is performed. This is the distinction made between Pythagoras & Euclid in their proof of the theorem. While it, perhaps, belongs in an article on mathematical history or greek math history (thus the topic of Russo's book) I thought this article was really lacking in the history of the theorem. Considering the number of peoples listed in the opening paragraph, it hardly does the theorem justice. Cburnett 01:31, 13 Dec 2004 (UTC)
From my talk page:
Well, you do not know for sure (who proved it), all you know is that it is proved in Euckids book, and it is unlikely due to Euckid, anyway it is more related to history of math. Yes, Pythagoras did not have a proof in a way we understand it now, but the same is true for Euckid (although it is closer) so there is no point in your statement. The same is true for all old theorems... Tosha 01:04, 13 Dec 2004 (UTC)
Fine, insert "known" into "...provides the first proof of the Pythagorean theorem...". There's no point in stating that the first known *real* proof of Pythagorean theorem was by Euclid???? No point??? Such point has been the most interesting thing I've read directly regarding the theorem. Cburnett 02:13, 13 Dec 2004 (UTC)
- I think you misunderstand Tosha's "there is no point" comment. If I read him correctly, he's not saying the matter is unimportant; he's saying that to be really precise, not even Euclid had a *real* proof of the theorem that meets with modern standards. While he is technically correct, I think this rigid application of criteria is unreasonable to follow. Taking into account that the most basic foundations of the Elements are flawed from a modern viewpoint, the entire work can be discounted as "not a real proof" or somehow unworthy. For that matter, this criticism can be applied to almost all mathematics done before the first quarter of the 20th century, so Tosha must apparently think "old" means "older than 1925" or something. For me personally, the important thing is not whether or not Euclid's proof is an "according to Hoyle miracle" and passes a modern litmus test, but rather whether "god got involved", i.e. the fact that Euclid's argument shows a clear understanding of the distinction between (attempted) proof and demonstration, and a grasp of the concept of logical argument and deduction, and these are clearly present in Euclid in a way that wasn't present in Pythagoras's understanding. Moreover, although Euclid's proof is technically "wrong", it is (as Tosha points out) "closer" and needs little adjustment to meet modern standards. It is true that Euclid almost certainly was not the first to actually discover the proof, but the Elements is the first known work to present it (today, we would say it was first "published" in the Elements). And I fail to see how some historical comments are out of place in the article; should all historical math comments be relegated to separated articles? I agree, the way it was it seemed out of place so much at the beginning, but a separate section toward the end of the article specifically discussing the history of the theorem and proof seems okay. Revolver 14:36, 15 Dec 2004 (UTC)
Euclid's definition of area
I've read through the previous discussions related to the "n.b." after the nice illustrative proof of the Pythagorean theorem. It seems to me that these discussions have missed a very important point. The focus of these discussions is on the (supposed) difficulty of finite additivity of area, and in particular, what is usually called the scissors congruence of planar regions.
But Euclid's definition of area was that two polygonal regions have the same area if they are scissors congruent! So how to make sense of the statement, "In particular, while it is easy to give a formula for area of triangles and squares, it is not as easy to prove that the area of a square is the sum of areas of its pieces."?
Are we to always take the notion of Lebesgue measure as primitive and even in straightforward contexts (such as this) "point out" that proofs using Euclidean notions are not "elementary"?
As the NB currently stands, I feel it is misleading. The given illustrative proof is in fact very simple, presupposing the basic notions of Euclidean geometry. There is no need for an NB in my opinion. --Chan-Ho Suh 06:07, Jan 2, 2005 (UTC)
- If you start with definition as two polygonal regions have the same area if they are scissors congruent then you have to show that non all polygonal regions have the same area, that the same thing as finite aditivity of area... Again it is doable, but it is much harder than Pythagorean theorem.
- Pythagorean theorem is a basic result and one should expect to see the proof which follows directly from axioms. on the other hand definition of area often avoided in books on Eucl.geom. So most of the proofs using area are cheating and little worning sign is good. Tosha 20:51, 5 Jan 2005 (UTC)
Formula
Hi, In the school where I am taught, we are normally told the formula is:
x=a+b
rather than
a + b = c
Does anyone think that the formual that I am being taught should be worht noting?
Thanks, 17:37, 18 September 2005 (UTC)
- I'm glad you are interested enough to ask. In mathematics, the FORM of a formula is not important, only the relationship between the symbols. In other words, as a formula, a + b = c, x + y = z, and T + U = P are all exactly the same. In the formula you are taught the names of the legs are a and b and the name of the hypotenuse is x, but in the formula in the article the names of the legs are a and b and the name of the hypotenuse is c. But, what's in a name? A rose by any other name would smell as sweet. Rick Norwood 23:40, 30 September 2005 (UTC)
- A separate issue is whether the form familiar to this student is used often enough to justify mention; like it or not, certain forms in mathematics have acquired a connotation that is nearly impossible to remove (like if you use p for a non-prime integer, some mathematicans would probably like to kill you :-). I don't think I've ever seen the Pythagorean theorem stated with x though. Deco 05:40, 15 December 2005 (UTC)
- Beyond high school, the use of x in the Pythagorean Theorem is common. Rick Norwood 15:05, 15 December 2005 (UTC)
geometry vs algebra
Given the heat of the discussions above, I'm not about to make any changes in the article. But I do notice that the correct statement of the original theorm, that the squares ON the legs equal the square ON the hypotenuse (in area) has been replace by the modern version. I was sorry to see that. Rick Norwood 23:45, 30 September 2005 (UTC)
- I agree, and I've changed it back. People who edit the statement of the Pythagorean theorem in this article but who can't state it without mentioning squares of numbers
should be profoundly ashamed of themselveshave something to learn. Michael Hardy 02:02, 1 October 2005 (UTC)
- Here's how I've written it:
- The theorem states that in any right triangle, the area of the square whose side is the hypotenuse (the side of the triangle opposite the right angle) is equal to the sum of the areas of the squares on the other two sides.
- Michael Hardy 02:05, 1 October 2005 (UTC)
Now that things have quieted down...
I notice some gaps and some contradictions in the history section. First, the section does not make clear the difference between ancient cultures that knew the Pythagorean theorem, and ancient cultures that knew a proof of the Pythagorean theorem. Mesopotamia certainly knew the theorem, but no proof has been found to date. As for China and India, the statement that they knew the theorem (did they have a proof?) before Pythagoras (that is, before the sixth century BCE) seems unsupported. The dates given for the Chinese version, 500 BCE - 200 BCE, span a wide range, and certainly do not clearly establish priority. Not that priority is that big a deal. The important thing, I would think, is independent discovery. According to vad der Waerden's Geometry and Algebra in Ancient Civilizations (1983) documents containing Pythagorean triples predate any pictures of right triangles. Much valuable information can also be found in Sir Thomas L. Heath's commentary on Euclid (Dover) but Heath was writing between 1908 and 1925, and much has been discovered since then.
- circa 2500 BCE. Megalythic monuments on the British Isles incorporate right triangles with integer sides. van der Waerdan conjectures that they were discovered algebraicly.
- 2000 - 1786 BCE. The Middle Kingdom Egyptian papyrus Berlin 6619 has a problem the solution to which is a Pythagorean triple.
- 1790 - 1750 BCE. The Mesopotamean tablet Plimpton 322, written during the reign of Hammurabi, contains a large number of entries closely related to Pythagorean triples.
- 582 - 507 BCE. According to Proklos' commentary on Euclid, written between 410 - 485 CE, Pythagoras used algebraic methods to construct Pythagorean triples.
- 500 - 200 BCE. In India, the Śulavasūtras contains a statement of the Pythagorean theorem and a list of Pythagorean triples discovered algebraicly. It also contains what might be called a "numerical proof" of an area computation. By a numerical proof, I mean an example that uses specific number, but which can be generalized. This is the earliest proof discovered in India. According to van der Worden "it was certainly based on earlier traditions".
- circa 300 BCE. Again according to Proklos, Plato gave a method for finding Pythagorean triples that combined algebra and geometry.
- circa 300 BCE. Euclid gives a purely geometric proof of the Pythagorean theorem.
- 200 BCE - 200 CE. The Chinese text Chou Pei Suan Ching' gives a numerical proof of the Pythagorean theorem, using the (3, 4, 5) right triangle. From the same period (Han dynasty), Pythagorean triples appear in Nine Chapters on the Mathematical Art, together with a mention of Phytagorean right triangles.
Should this information be incorporated in the history section. Have there been any more recent discoveries? Rick Norwood 21:59, 1 November 2005 (UTC)
History of the Pythagorean theorem
A few days ago, I posted above a brief history of the Pythagorean theorem, mostly taken from Geometry and Algebra in Ancient Civilizations by B.L.van der Waerden and from Sir Thomas L. Heath's commentary on Euclid's Elements. There are problems with the history section as it now exists. First, the section does not make clear the difference between ancient cultures that knew about Pythagorean triples, cultures that knew the Pythagorean theorem, and cultures that knew a proof of the Pythagorean theorem. Next, the dates given in the text for the Chou Pei Suan Ching (500 BCE - 200 BCE), do not agree with the dates I've found in other sources, which place this in the Han dynasty (200 BCE - 200 CE). As for Indian mathematicians knowing the theorem before Pythagoras, the dates given for the , the statement that they knew the theorem (did they have a proof?) before Pythagoras (that is, before the sixth century BCE) seems unsupported. The dates given for the Śulavasūtra span a wide range, and do not clearly establish priority. According to Heath, Albert Bŭrk was the first to claim Indian priority, but this claim has been widely criticized. The chronology below seems to be cautious, and limits itself to what we know. I'll leave it here for a couple of days, and move it to the article if there are no corrections or improvements.
- The history of the theorem called Pythagorean has three parts. The best evidence currently available indicates that first came knowledge of Pythagorean triples such as (3, 4, 5); second, empirical knowledge of the general theorem as it refers to right triangles; third, proof of the theorem.
- circa 2500 BCE. Megalithic monuments on the British Isles incorporate right triangles with integer sides. B.L. van der Waerden conjectures that these Pythagorean triples were discovered algebraicly.
- 2000 - 1786 BCE. The Middle Kingdom Egyptian papyrus Berlin 6619 has a problem the solution to which is a Pythagorean triple.
- 1790 - 1750 BCE. The Mesopotamian tablet Plimpton 322, written during the reign of Hammurabi, contains a large number of entries closely related to Pythagorean triples.
- 582 - 507 BCE. According to Proklos' commentary on Euclid, written between 410 - 485 CE, Pythagoras used algebraic methods to construct Pythagorean triples. According to Sir Thomas L. Heath, there is no attribution of the theorem to Pythagoras for five centuries after Pythagoras lived, but when authors such as Plutarch and Cicero do attribute the theorem to Pythagoras, they do so in a way that suggests the attribution is widely known and undoubted.
- 500 - 200 BCE. In India, the ]s contain a statement of the Pythagorean theorem and a list of Pythagorean triples discovered algebraicly. The Āpastamba Śulavasūtra also contains what might be called a "numerical proof" of the theorem, using an area computation. A "numerical proof" uses specific numbers, but in a way which can be generalized. According to van der Worden "it was certainly based on earlier traditions". According to Albert Bŭrk, this is the original proof of the theorem, and Pythagoras copied it. Many scholars find Bŭrk's claim unsubstantiated.
- 200 BCE - 200 CE. The Chinese text Chou Pei Suan Ching gives a numerical proof of the Pythagorean theorem, using the (3, 4, 5) right triangle. From the same period (Han dynasty), Pythagorean triples appear in Nine Chapters on the Mathematical Art, together with a mention of right triangles. (Some authorities place the Chou Pei Suan Ching as much as three hundred years earlier than the Han dynasty.)
- There has been much debate on whether the Pythagorean theorem was discovered once or many times. B.L. van der Waerden asserts a single discovery, by someone in Neolithic Britain, knowledge of which then spread to Mesopotamia and Egypt circa 2000 BCE, and from there to India, China, and Greece circa 600 BCE. Most authorities, however, favor independent discovery.
Rick Norwood 14:36, 4 November 2005 (UTC)
Good edit, Lupin.
Lupin is, of course, correct. Rick Norwood 00:42, 5 December 2005 (UTC)
Dates of the Āpastamba Śulavasūtra
The dates of the Āpastamba Śulavasūtra have been changed in this article, pushing the date back to the 9th Century BCE. Here are some google references:
"The Apastamba Sulvasutra, which dates between the fourth and third. centuries BCE, but was aparently part of an earlier edition. contains a ... home.uchicago.edu/~wwtx/Lecture%202.pdf"
"The later Sulba-sutras represent the 'traditional' material along with further related elaboration of Vedic mathematics. The Sulba-sutras have been dated from around 800-200 BC, and further to the expansion of topics in the Vedangas, contain a number of significant developments."
Clearly, there is a lot of disagreement as to the date of this Sutra. Can anyone suggest an authority on this subject? Rick Norwood 16:02, 6 December 2005 (UTC)
Errors ID'd by Nature, to correct
The results of what exactly Nature suggested should be corrected is out... italicize each bullet point once you make the correction. -- user:zanimum
Reviewer: Geoff Smith, Senior Lecturer in Mathematics at the University of Bath, UK.
- “This means that knowing the lengths of two sides of a right triangle is enough to calculate the length of the third-something unique to right triangles. is misleading. If you know two sides of a triangle and the included angle then you can always calculate the length of the third side.
- This error was found and fixed on December 4, 2005 by Lupin, I'm going to remove the template from the page. Paul August ☎ 19:32, 22 December 2005 (UTC)
- The error was introduced by this edit on September 16th 2005. Paul August ☎
Pythagorean theorem in heraldry
The "History" section states that In heraldry, the Pythagorean theorem appears as a charge in the arms of Seissenegger.. I have not been able to find anything about this (not even who Seissenegger is...). Apart from one reference that seems independent, everything on the web is more or less derived from this sentence in Misplaced Pages. Does anyone has any reference, more information, or, even better, an image representing the arms ? That would be great ! Schutz
Back to featured status?
Considering the amazing one mistake found (and almost instantly corrected) in this article and its former featured status, shouldn't we consider putting it up for featured article status again? I do not actively contribute to this article, but I think that it should be said. What does everyone think? — Scm83x ![]() 00:29, 18 January 2006 (UTC)
00:29, 18 January 2006 (UTC)
- It would get my vote. Rick Norwood 01:20, 18 January 2006 (UTC)
- Support too, and this was something on my todo list. However, there is still quite a bit to do before the article reaches featured status, especially since the criteria are much more strigent now than they were in the past. I'll add a TODO list section below so that we can see what has to be done, and will try to work on it. Schutz 07:56, 18 January 2006 (UTC)
- Yup. Definitely should be refeatured. Reyk 06:50, 7 February 2006 (UTC)
- No way. Can't be featured the way it is now. It raises more questions than it answers: here are a few that jump out in the history section:
- what does "knowledge of Pythagorean triples" mean? Does it mean knowing that if you manage to construct a triangle with sides 3, 4, 5 you get for all practical intents and purposes a right angle? Or does it mean that if you do the algebraic calculation 3x3+4x4 you get the same answer as 5x5? The latter, I would argue, has nothing to do with the Pythagorean theorem. It's arithmetic, not geometry. Even the former is not about areas of squares. It's about right triangles, but about the Pythagorean theorem?
- how are megalithic monuments even supposed to understand the theorem?
- Is the Berlin 6619 Papyrus relevant, if it's about arithmetic of Pythagorean triples and not about the Pythagorean theorem in geometry? Ditto for Plimton 322?
- It would really be great if the proofs refered to in the history section could be reproduced, so everyone can see what exactly was proved in each case: an arithmetic theorem, a geometric theorem about proportions, a geometric theorem about areas, or maybe "not much".
- the famous "visual proof" in the Chou Pei Suan Ching: if someone could explain how exactly that picture is supposed to prove anything, and if so, what exactly it proves, I would be very happy. It looks vaguely like the right half of the "visual proof" later on in the article. Does this mean it is actually an illustration of that proof? Then that should be mentioned explicitely. By counting squares, I can sort of see that this illustration might prove that the hypotenuse of the triangle with legs 3 and 4 has length 5. Maybe that's what this is about? Or something else? It doesn't help that the article refers to this proof first as "visual" and then as "numerical".
- About the proof section: There should be a variety of proofs, using different kinds of mathematics.
- Euclid's proof, though not the simplest, should be included, because it's of historical interest. I'll do this when I get around to it.
- mention that the current "Geometrical proof" relies on the theory of proportions and similar triangles, which is conceptually unrelated to any statement about areas. Instead, it's a statement about proportions.
- Of course, the "visual proof" is also geometrical. And of course it doesn't rely on any theory of Lebesgue measure as some tried to suggest on this discussion page. It just relies on simple axiomatics of area in Euclidean geometry. Even if you want to use some sophisticated area theory a la Hilbert, you don't need the general theorem that no matter how you cut up a square into triangles, the pieces always add up correctly. You only have to compare these two specific and very simple decompositions, and that is quite easy to do. So this NB about not being elementary is rubbish.
- About the "Other facts" section: two completely random, and not particularely noteworthy pieces of trivia. Certainly, we should be able to to better than that? Sorry for going on and on --345Kai 18:31, 22 April 2006 (UTC)
- No way. Can't be featured the way it is now. It raises more questions than it answers: here are a few that jump out in the history section:
Responding to the points you raise. None of Pythagoras's writings on the subject survive, but given his interest in numbers, Pythagoras may have been more interested in triples than in triangles. The history section gives not only the discovery of the theorem itself, but also ideas that let up to the theorem, just as a history of calculus mentions Eudoxus's Method of Exhaustion, thought the Method of Exhaustion is not calculus. Yes, knowledge of Pythagorean triples means knowing that there are numbers with the property that the sum of the squares of two of them equal the square of the third. It does not necessarily have anything to do with triangles, though many ancient civilizations made the connection. "Even the former is not about areas of squares..." The former is exactly about areas of squares, see Euclid's proof which, yes, it would be good to add.
Megalith monuments, of course, don't understand anything, but they can show understanding on the part of their builders. I'll reread the section, and correct it if it implies the former.
The current history section replaces a section that claimed "proofs" of the theorem for many ancient civilizations. Checking the references, I found that the "proofs" in question were usually pictures, or even physical objects, such as alters. Many peoples feel very strongly about claims that their civilization anticipated various European inventions or discoveries. The history section attempts to sort out those claims and explain what is known in each case.
Euclid introduces areas in terms of proportions. For example, he proves that if a parallelogram and a triangle have the same base and height, then their areas are in a ratio of 2:1.
Rick Norwood 22:49, 22 April 2006 (UTC)
TODO
Please complete or cross if you believe the job has been done:
Rewrite the "History" section: no bullet points. Schutz— Scm83x 14:45, 18 January 2006 (UTC)
14:45, 18 January 2006 (UTC)
Rewrite the "Generalizations" section: no bullet points, more structure. Schutz— Rick Norwood 19:43, 18 January 2006 (UTC)- Add inline references whereever possible. Schutz
- We could probably do with a few more figures, especially for the generalizations. Schutz
- The first of these has been done, but it is not clear to me what the objection is to bullet points. Are these objectionable per se or just in this context? Rick Norwood 13:36, 18 January 2006 (UTC)
- The objection to bullet points stems from the belief that featured articles should represent our best prose, which is not exemplified by bulleted lists. Take a look at WP:FA to see what the standards are. — Scm83x
 14:45, 18 January 2006 (UTC)
14:45, 18 January 2006 (UTC)
- The objection to bullet points stems from the belief that featured articles should represent our best prose, which is not exemplified by bulleted lists. Take a look at WP:FA to see what the standards are. — Scm83x
- Thanks. I'll get to work on eliminating the rest of the bullet points right away. Rick Norwood 19:10, 18 January 2006 (UTC)
Proof of the Theorem of Pythagoras using Analysis
As far as I know, there are quite a few problems with this proof (apart from the format, which I was tempted to correct but decided not to). The problem arises from the fact that the proof links the usual definition of sine and cosine to their power series; however, as far as I know, this link depends on the veracity of the identity , which is the Pythagorean theorem (as shown on the last line of the proof). In fact, Elisha Loomis (see ref in the article) goes as far as saying that there can be no proofs based on trigonometry (or analytical geometry or calculus, for that matter) because "Trigonometry is because the Pythagorean Theorem is." (p. 244). Barring any objection, I will remove the proof, and add a comment about these (interesting) observations. Schutz 00:33, 22 January 2006 (UTC)
- I agree. There is a long way around -- one can simiply define a Euclidean metric without specific reference to the Pythagorean theorem, and derive the trig derivatives on which their MeLauren series depend from that metric. But this is sophistry. The Euclidean metric is what it is because of the theorem. Your changes all improve the article. Can we try for "featured article" status now? Rick Norwood 19:12, 22 January 2006 (UTC)
- I actually missed the question — sorry for answering so late. The "Generalization" section is still a mess (compare it with the corresponding page on the French Misplaced Pages). Also, I think the history section could be improved too. I have borrowed the relevant books from the local library, but they have been sitting on my desk for a while now... Schutz 00:40, 19 February 2006 (UTC)
Big stones
Most of the megalithic references can be found in van der Werden. Rick Norwood 12:48, 11 April 2006 (UTC)
Recent changes, new section
Some comments about the recent changes following my additions, mainly by Rick Norwood:
- About the header of section Consequences and uses of the theorem: I agree that it is not the best title, but it is better than not having a section at all (and having everything collapsed under Proofs, as it was until a few minutes ago). Even though Rick is probably right in saying that triples may very well have preceded the theorem rather than being consequences of it (from an historical point of view), it does not prevent us to present things in the reverse order if we want anyway.
- About Hippasus being drowned at sea, you added: But if this legend were true, it is hard to understand how the story would have survived, since only the murderers would be in a position to report the murder. Without a suitable reference, I think it is not up to us to comment on the legend (we mention that it is a legend, so people know it may be untrue. Note that the two references cited do not comment on this either, even though they are now placed as though they do). As for the comment itself, don't forget that the Pythagoreans did not write anything, and were not supposed to talk about their academy to outsiders; even then their history has survived, so it would not be too surprising if the murder story was real and had survived too.
- You removed the comment saying that the confusion between irrational number and geometry was not solved satisfactorily until the nineteenth century; don't you think it is worth mentioning that the consequences of this (apparently simple) problem kept mathematicians busy for so long ?
Schutz 21:28, 13 April 2006 (UTC)
It's stretch to say that only the murderers would know who the murderers are. There are certainly very many murders in which the culprit got caught. Michael Hardy 22:42, 13 April 2006 (UTC)
- I am ok with your edit of my edit as it now stands (and thanks for correcting the typos), but I still think we need to come up with a better title for the section. However, your idea that there was "confusion" about irrational numbers before the 19th century does not seem correct. Euclid, certainly, was not confused about irrational numbers. The Greeks solved the problem of irrational numbers with continued fractions. Rick Norwood 13:22, 14 April 2006 (UTC)
Image of Plimpton 322
Having an image of the plimpton 322 tablet would be a welcome addition to this page. I have started discussing on Talk:Plimpton 322 to see if any of the pictures of the tablet could be considered as being in the public domain, or if there was another way to get a picture (I don't know if the tablet is publicly exhibed at Columbia University ?). If you have any suggestion, don't hesitate to contribute. Schutz 14:10, 20 April 2006 (UTC)
Vedic proofs
From the current version of the article:
More recently, Shri Bharati Krishna Tirthaji in his book claimed ancient Indian Hindu Vedic proofs for the Pythagoras Theorem.
I know nothing about Vedic mathematics, so I thought I'd ask the question here... Is this assertion notable enough to warrant a mention ? If yes, we should probably expand a bit (what are these proofs ? if there are not known, why is it believed that they exists ? etc). On the other hand, if it is just a lonely claim, we can probably remove this. Schutz 12:47, 21 April 2006 (UTC)
Proofs are generally accepted as being the product of ancient greek thought, resting on formal systems of demonstration using stated axioms and definitions. These are all things that Vedic mathematics lacks. Vedic mathematics is essentially algorithmic, not algebraic.Mrdthree 05:09, 3 October 2006 (UTC)
Priority cranks
I can clearly see destructive waves of ethnic edits flooding Misplaced Pages. Indians have arrived, Chinese are approaching. Who is next? What can be done about it? Maybe we should create some form of priority police that will catch the cranks and make them write 20 times "I will not make my nation a laughingstock by unsubstantiated claims" before unblocking their accounts :) ? ----212.199.22.211 22:00, 30 April 2006 (UTC)
Comment by A. Marshall and Reply removed 69.196.9.156 02:46, 11 September 2006 (UTC) A. Marshall
History section
Something that confuses me about the history section is that the opening sentence says that the hystory can be divided into three sections, yet it doesn't actually make that distinction when relating the history. That seems a bit confusing, as I would be expecting subsections on the history of Pythagorean triples, the history of the knowledge of the relationships in a right triangle, and the history of proofs of the theorem.
Another formula for generating triplets.
Where m is a positive odd integer, m, ½(m^2-1), ½(m^2+1) will be the sides of a right triangle.
- I came up with a practically identical one. JedG 01:08, 25 September 2006 (UTC)
GA Re-Review and In-line citations
Note: This article has a small number of in-line citations for an article of its size and subject content. Currently it would not pass criteria 2b.
Members of the Misplaced Pages:WikiProject Good articles are in the process of doing a re-review of current Good Article listings to ensure compliance with the standards of the Good Article Criteria. (Discussion of the changes and re-review can be found here). A significant change to the GA criteria is the mandatory use of some sort of in-line citation (In accordance to WP:CITE) to be used in order for an article to pass the verification and reference criteria. It is recommended that the article's editors take a look at the inclusion of in-line citations as well as how the article stacks up against the rest of the Good Article criteria. GA reviewers will give you at least a week's time from the date of this notice to work on the in-line citations before doing a full re-review and deciding if the article still merits being considered a Good Article or would need to be de-listed. If you have any questions, please don't hesitate to contact us on the Good Article project talk page or you may contact me personally. On behalf of the Good Articles Project, I want to thank you for all the time and effort that you have put into working on this article and improving the overall quality of the Misplaced Pages project. Agne 05:46, 26 September 2006 (UTC)
History
The history section needs clean-up. Right now it appears to be a random assortment of facts related to the Pythagorean theorem, rather than a concise history.Mipchunk 16:15, 5 October 2006 (UTC)
the pythagoren therom
in any right triangle , the area of the square whose sides is the hypotenuse is equal to the sum of areas of thesquares whose sides are the two legs(i.e the two sides other than the hypotenuse)
As of now, the article contradicts itself
It states "For similar reasons, no proof can be based on analytic geometry or calculus." But then there's a proof by differential equations!! What's going on? --Ķĩřβȳ♥ŤįɱéØ 09:52, 20 October 2006 (UTC)
- It's possible that the contradiction is only apparent. I'm going to dig out Loomis' book and see what's going on. Michael Hardy 20:31, 20 October 2006 (UTC)
- ... oh, and there's certainly no contradiction between saying that Loomis said something, and that he was wrong. But even if he was right, the contradiction may be only apparent. Michael Hardy 20:32, 20 October 2006 (UTC)
- If needed, I can get it from the library here and pee my pants wheras copy the relevant parts (I plan to scan it anyway, given that it is in the public domain where I live). However, I am the one who introduced this comment, and I don't think there was much more explanation. Schutz
- I can't find the offending sentence anymore, so I guess the contridiction is gone (unless integral calculus depends on this theorem or some similar subtlety). I'll remove the contradiction tag. Shinobu 16:11, 19 December 2006 (UTC)
- If needed, I can get it from the library here and pee my pants wheras copy the relevant parts (I plan to scan it anyway, given that it is in the public domain where I live). However, I am the one who introduced this comment, and I don't think there was much more explanation. Schutz
One of the proofs has a subtle mistake
The second proof under the caption of "Visual Proofs" that is based on the diagram  is faulty in that it is incomplete. The dissection does not go through when the two legs of the triangle are essentially different in size. For a dynamic illustration see .
is faulty in that it is incomplete. The dissection does not go through when the two legs of the triangle are essentially different in size. For a dynamic illustration see .
- A. Bogomolny
- I like the dynamic illustration. There should be more like that on Misplaced Pages. Michael Hardy 23:15, 8 December 2006 (UTC)
Algebraic proof incomplete
The algebraic proof ends saying that the area of the square is (a+b)². I think it should make a connection with the previous equation, like this:
- ,
instead of just saying that (a+b)² expands to a²+2ab+b².
—R. A. C. 14:11, 26 November 2006 (UTC)
- I just read the article and I think it's fixed now. Shinobu 15:45, 19 December 2006 (UTC)
Section deleted
I deleted this whole piece of the article:
Pythagoras's theorem and complex numbers
This proof is only valid if a and b are real. If a and/or b have imaginary parts, Pythagoras's theorem breaks down because the concept of areas loses its meaning because in the complex plane loci of the type y = f(x) (which includes straight lines) cannot separate an inside from an outside because there they are 2-dimensional (y, iy) in a 4-dimensional space (x, ix, y, iy).
This is just gibberish. And it denies the truth of the Jordan Curve Theorem, which may be hard to prove, but which does apply to the complex plane. DavidCBryant 14:18, 2 December 2006 (UTC)
- Actually, the writer was using the term complex plane to mean C, which is a complex plane in the sense that it is 2-dimensional over C. (See Talk:Complex plane#Disambiguation needed for this use of the term "complex plane".) So the Jordan Curve Theorem doesn't apply. I agree, however, that this paragraph shouldn't be in the article. --Zundark 13:50, 8 December 2006 (UTC)
- Thanks for looking at it, Zundark. And thanks for the pointer to the discussion of C. I understood that whoever wrote this section was trying to say that there are other geometries besides Euclidean geometry in mathematics. But there's got to be a better way to point that out. DavidCBryant 21:49, 8 December 2006 (UTC)
Added note on similarity to euclid's proof
Euclid's diagram has a pretty obvious proof by similarity, which needs an axiom something like "If two dissections are soimilar, then the ratios of the areas of each corresponding bit will be identical". I know itkind of doesn't belong in the section that (for historical reasons) shows euclid's proof specifically, but that's where the diagram is.
Our world is not euclidean
In my opinion, it would be good if someone adds that the Pythagorean Theorem does not hold in the real world, as our space is not really Euclidean. In the neighborhood of large mass (e.g. in the neighborhood of a black hole, etc), the sum of the angles in a triangle is not 180 degrees etc. It is a paradox, that there are 300 proofs of a theorem that does not really hold! It would be nice to mention this at least. All the poofs work only in the framework of Euclidean geometry. — Preceding unsigned comment added by 86.49.80.222 (talk • contribs)
- The very first sentence of this article reads as follows.
In mathematics, the Pythagorean theorem or Pythagoras' theorem is a relation in Euclidean geometry among the three sides of a right triangle.
- So the reference you desire is already in the article, in my opinion. Questions like whether the theory of General Relativity is "true" are not the province of mathematics; such questions are problems in physics. This is a math article. In a formalist view of mathematics, connections between math and the real world are unnecessary. All we have are the axioms, the definitions, and the laws of logic.
- In the real world, there is no such thing as a perfect circle. Do you think mathematicians shouldn't talk about circles, either? DavidCBryant 22:07, 8 December 2006 (UTC)
One should not use such grandiose phrases are "our world" or "our universe" if what one means is "physical space". Rather than writing "Our universe is not Euclidean" one should write "Physical space is not Euclidean". Michael Hardy 23:12, 8 December 2006 (UTC)
OK, I see Franp9am 18:24, 12 December 2006 (UTC)
Explanation of Euclid's proof
We see here three similar figures, each being "a square with a triangle on top". Since the large triangle is made of the two smaller trangles, its area is the sum of areas of the two smaller ones. By similarity, it is clear that the same must apply also to the areas of the squares.
It would appear that the above description under section Euclid's proof would be applicable to any triangle (not just a right triangle), and as such, misleading. Should be removed? --Thejaka 11:38, 9 December 2006 (UTC)
- The figures are similar, in the sense of Similarity (geometry) (meaning they are the same except for being different sizes). Although an interesting approach, this has little (if anything) to do with Euclid's proof. Rather than delete this, I think it would be good to find a reference for this approach and move it somewhere else. -- Rick Block (talk) 16:04, 9 December 2006 (UTC)
- Silly of me not to have taken a closer look! My initial interpretation was that similarity was applicable to the squares, which didn't make sense. I realize now that the triplet of composites were intended, which elucidates the matter. I should have at least noticed how the quotation marks were placed... Thus is stupidity demonstrated. --Thejaka 10:47, 13 December 2006 (UTC)
Peer review 2005
Concerning Nature's peer review in 2005: "It was found to have 1 error; this error has been fixed."
Out of curiosity, what whas this error? How was it fixed? Someone should make it easier to look that kind of tidbits up. Shinobu 16:15, 19 December 2006 (UTC)
Known in India as Bhaskara Theorem
An anonymous editor changed the lead sentence to:
- In mathematics, the Pythagorean theorem or Pythagoras' theorem or Bhaskara Theorem is a relation ...
I've revised this so that what it is known as in China and India is in the first paragraph, but not in the lead sentence. I do not know with certainty that it is actually known as Bhaskara Theorem in India (there are only two google hits for this), but I assume this was the point of the anonymous edit. -- Rick Block (talk) 19:04, 21 December 2006 (UTC)
Categories:

 .
. 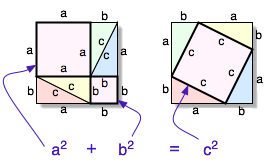
 , which is the Pythagorean theorem (as shown on the last line of the proof). In fact, Elisha Loomis (see ref in the article) goes as far as saying that there can be no proofs based on trigonometry (or analytical geometry or calculus, for that matter) because "Trigonometry is because the Pythagorean Theorem is." (p. 244). Barring any objection, I will remove the proof, and add a comment about these (interesting) observations.
, which is the Pythagorean theorem (as shown on the last line of the proof). In fact, Elisha Loomis (see ref in the article) goes as far as saying that there can be no proofs based on trigonometry (or analytical geometry or calculus, for that matter) because "Trigonometry is because the Pythagorean Theorem is." (p. 244). Barring any objection, I will remove the proof, and add a comment about these (interesting) observations. 


 ,
,