| Revision as of 19:41, 18 June 2009 editBrews ohare (talk | contribs)47,831 edits →Language of discussion← Previous edit | Revision as of 01:00, 19 June 2009 edit undoDicklyon (talk | contribs)Autopatrolled, Extended confirmed users, Rollbackers477,393 edits →Language of discussionNext edit → | ||
| Line 319: | Line 319: | ||
| :::I've broken the subject up into bite size pieces to make the relevance clear and added several references to the approach. I hope that it works now. ] (]) 19:11, 18 June 2009 (UTC) | :::I've broken the subject up into bite size pieces to make the relevance clear and added several references to the approach. I hope that it works now. ] (]) 19:11, 18 June 2009 (UTC) | ||
| :::One source of relevance is to make connection to the notion of dispersion: a general waveform propagates unchanged only if its component parts can so propagate. To understand that, one has to introduce the notion of component parts. ] (]) 19:41, 18 June 2009 (UTC) | :::One source of relevance is to make connection to the notion of dispersion: a general waveform propagates unchanged only if its component parts can so propagate. To understand that, one has to introduce the notion of component parts. ] (]) 19:41, 18 June 2009 (UTC) | ||
| ::::The result remains nonsensical, since the idea of components propagating only makes sense in linear media. Your statement that "Such circumstances sometimes do occur in nonlinear media" is misleading, or downright wrong, if by "such circumstances" you mean "that the medium must be capable of propagating disturbances of different wavelengths at the same wave speed". Nonlinear media typically do NOT have that property, yet can propagate periodic non-sinusoidal signals (only of particular shapes, though -- they can't propagate sinusoids, for example, at non-trivial amplitude). ] (]) 01:00, 19 June 2009 (UTC) | |||
Revision as of 01:00, 19 June 2009
| Physics Start‑class High‑importance | ||||||||||
| ||||||||||
The Speed of Light
"The speed of light in most media is lower than in vacuum ..."
Could someone name me a medium where light travels faster than it does in a vacuum? 80.42.146.135 17:23, 25 October 2007 (UTC)
- No such medium exists in are universe. The speed of light is relative to the universe you live in, for us it's 299,792,458 meters per second max... This absolute limit makes space travel a real bitch because 299,792,458 m/s is very slow when compared to the size of the observable universe, which is 863,990,470,000,000,000,000,000,000 meters in diameter. It would take 91,325,815,300 years to travel from one end to the other in a space ship moving at the speed of light. Getting the space ship up to the speed of light would require all the energy in the universe. :-( —Preceding unsigned comment added by 98.214.11.170 (talk • contribs) 18:12, 2 April 2008 (UTC)
- Assuming the calculations above are correct, how can it take 91 billion years to travel from one end of the universe to the other at the speed of light, given that the age of the universe is estimated to be only 14 billion years old? Matter would have to be traveling at over 63,064,997,800,000,000 m/s to make 14 billion years work. I'm confused?—Preceding unsigned comment added by 216.73.235.7 (talk • contribs) 20:54, 2 April 2008
- because (i think) the expansion of the universe is not just the movement of matter, but also a stretching of space itself, which allows the universe to expand much faster than would be limited by your logic, especially during the early stages of the universe's expansion, however im not an expert, and this is just something i recall seeing somewhere, i cant cite it — Preceding unsigned comment added by 193.60.83.241 (talk • contribs) 13:55, 16 May 2008 (UTC)
Wavelength, frequency, type of wave, and energy in joules
Does anyone know the equations to figuring out all of these with just one? PatPeter 20:50, 5 January 2007 (UTC)
Describing Wave Image
"The x axis represents distance, and I would be some varying quantity at a given point in time as a function ..."
Be nice to see some labels on the image. Also the italicised "I" looks like a forward slash, and is not immediately obvious as to how it relates to the tipped-over "I" from the image.
--Thangalin 26 Sep 2006 (PST)
Redirect change
I suggest that de Broglie wavelength redirects to de Broglie hypothesis, not here. It makes more sense, and de Broglie hypothesis has a much better description. Perhaps a rewrite is then needed of that section of this page to reflect that. Any objections?
--Blinken 11:45, 27 Apr 2005 (UTC)
- I can't believe it redirects here instead of de Broglie hypothesis already. I went ahead and changed it, figuring I should be bold. In light of that, the section in here about the de Broglie wavelength probably does need to be rewritten and shortened a bit. --Laura Scudder | Talk 19:36, 27 Apr 2005 (UTC)
- Went ahead and moved it over. --Blinken 02:19, 4 May 2005 (UTC)
Lambda = C / f
Would it be worth using the more general formula here, instead of ? Or perhaps mentioning that the formula can be used with other waves by replacing the speed of light with the speed of the individual wave? --Defragged 13:23, 13 Jun 2005 (UTC)
- Yes -- Tim Starling 04:11, Jun 14, 2005 (UTC)
relationship between Wavelength and the distance covered by the waves
Hey All !
is there any difference between the Wavelength and the distance covered by a MICOR WAVE when radiated through an anteena. Bascially i am confuse, what difference a wavelength make in the tranmisstion of a wave ? like do Higher Wavelength waves need less power to radiate and vice vers. Also what is the maximum range a micro-wave can travel or it can travell to unlimited distance (increasing the radiation in the area as a drawback ?) . I would be grateful if someone clear this up.
regards,
Shakeel Ahmad
- In a vacuum, electromagnetic radiation of any wavelength can travel for an unlimited distance, with intensity decreasing with distance due to the larger area as you suggest. When travelling through a medium, some will be absorbed, and the amount absorbed depends on the wavelength. That's not the major practical distance when talking about communications though, which I'm guessing you are. The major difference is that smaller wavelengths (higher frequencies) can carry more information than longer wavelengths. The downside is that higher frequencies need more expensive circuitry to send and receive. In general, smaller wavelengths are more strongly absorbed than longer wavelengths, although this is only a minor nuisance for most communications applications. -- Tim Starling 03:26, 8 December 2005 (UTC)
measurement?
how does one measure wavelength?? --129.11.76.216 12:01, 26 April 2006 (UTC)
simply by noting the peaks in activity upon the measuring body.
Incidentally, the sine curve model and the use of the term 'wavelength' are a bit misleading when used to describe the physical movement of a photon. It doesn't literally move in a path following the sine wave curve, it moves in a straight line, it's just that its intensity varies as a sine wave along said straight line. Peak follows peak through the medium. In pure vacuo all photons travel at c, the speed of light, photons of higher energy are merely able to create more concentrated peaks on their journey. Electromagnetic radiation is really a product of space-time behaviour, rather than something 'passing through' space-time, as it were. Martyn Smith 22:33, 14 July 2006 (UTC)
What is the unit of measurement for wavelength?
please cite your sources.§ Marcher Lord
Nevermind that, it's just units of measurement of length, I looked on
how do you find wavelength?
Where symbol lambda come from?
Hi, does anyone know where the symbol of wavelegth is derived? Perhaps from greek word, but what is that word? Sorry my bad english --213.186.244.119 11:29, 18 August 2007 (UTC)
- My guess would be from the word length (longueur, lengte, längd, länge, lunghezza in various languages). That's how you usually pick a symbol: if the Latin letter isn't available, you take a similar-sounding Greek letter. It would be nice to have a reference and add it to the article, though. Han-Kwang (T) 12:21, 18 August 2007 (UTC)
Lambda is the 11th letter of the greek alphabet... wikipedia has an article all about it http://en.wikipedia.org/Lambda
Wavelength Error?
The audible hearing frequency is listed on the page as being between 17 m and 17 mm. I believe the correct numbers are 17 km and 17 Mm. 138.32.32.166 16:57, 1 November 2007 (UTC)
- The speed of sound is not the speed of light. Han-Kwang (t) 19:53, 1 November 2007 (UTC)
- That's not the same kind of wave though. Maybe this article should only concern itself with electromagnetic waves that are governed by the speed of light, or split them into separate sections. The problem is that the speed of sound is not a universal constant. If I were in a submarine, an air plane, in space, or on another planet those numbers wound be way off. —Preceding unsigned comment added by 98.214.11.170 (talk) 17:04, 2 April 2008 (UTC)
- Wavelength is wavelength, for light waves, sound waves, water waves, etc., irrespective of the propagation speed of the wave. And phase speed is wavelength times frequency. Those are general physics principles and relationships. I do not think it useful to split this article in sections for the many existing kinds of wave phenomena. If the examples, given to clarify the concepts, lead to confusion, they have to be improved. Crowsnest (talk) 17:53, 2 April 2008 (UTC)
- Actually the wavelength of light changes in any medium from its free-space value. We define the frequency as staying the same, so it is incorrect to list the range of human hearing in a wavelength format. The frequency remains the same regardless of the medium it travels in; this is why human hearing is almost always written in Hz. —Preceding unsigned comment added by 128.61.50.94 (talk • contribs) 22:05, 23 November 2008 (UTC)
- Wavelength is wavelength, for light waves, sound waves, water waves, etc., irrespective of the propagation speed of the wave. And phase speed is wavelength times frequency. Those are general physics principles and relationships. I do not think it useful to split this article in sections for the many existing kinds of wave phenomena. If the examples, given to clarify the concepts, lead to confusion, they have to be improved. Crowsnest (talk) 17:53, 2 April 2008 (UTC)
- That's not the same kind of wave though. Maybe this article should only concern itself with electromagnetic waves that are governed by the speed of light, or split them into separate sections. The problem is that the speed of sound is not a universal constant. If I were in a submarine, an air plane, in space, or on another planet those numbers wound be way off. —Preceding unsigned comment added by 98.214.11.170 (talk) 17:04, 2 April 2008 (UTC)
School Project?
I gotta write some thing for science about wave length and my teacher said nit has to be a wikipedia page.Can someone make a page for me? —Preceding unsigned comment added by Foz77 (talk • contribs) 18:52, 21 January 2008 (UTC)
Only propagating waves
The article focuses entirely on propagating waves, while wavelength is a property of spatial wave patterns which do not have to propagate or move at all. How about generalizing this article to spatial wave patterns, not necessarily propagating? Crowsnest (talk) 11:39, 10 April 2008 (UTC)
Visible spectrum by wavelength in web colors
The link I added to http://www.magnetkern.de/spektrum.html (the visible electromagnetic spectrum displayed in web colors with according wavelengths) was reverted by another user with the reason that a german site is not suitable. The page is bilingual (english and german) and contains references to english sources, so I cannot understand the reasons for deleting the link. There is also a discussion about the wavelength table on the discussion page of the Color article. Jan 85.179.9.12 (talk) 10:40, 26 June 2008 (UTC)
- I re-instated the link. Crowsnest (talk) 11:17, 26 June 2008 (UTC)


- Indeed I overlooked the fact that there was English text on the page as well. But in any case, I find it somewhat dubious to claim that this is "the" way to map wavelengths to sRGB, for two reasons. The first is that the vast majority of consumer-grade LCD monitors doesn't even come close to sRGB, both in terms of the gamma and the positions of the R/G/B primaries. The second is that it is an arbitrary choice to simulate wavelengths larger than 611 nm by mixing in blue, i.e. simulating redder than red by making it purple. On the blue side it makes some sense since the red cones have some absorption in the blue, but it doesn't work the other way around. I understand that the idea behind it was that the straight line from the white point to the 700 nm crosses the boundary of the sRGB gamut somewhere along the purple line, but I fail to see why this is a "better" method than simply clipping at the edge of the gamut, with #ff0000 representing all wavelengths longer than 611 nm.
- The picture below is IMO the best one can do to simulate the color spectrum on sRGB. I was actually somewhat involved in the design of it (see Talk:Color_blindness/Archive1).
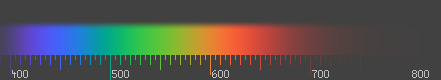
Han-Kwang (t) 12:08, 26 June 2008 (UTC)
- I agree that your picture is very nice. For getting a good impression of the visible spectrum, it's neccessary to fade out the lightness on the borders of the spectrum. The table behind the link is not doing that, but aiming more to be a translation table for a particular wavelength (e.g. to be used in articles), being as accurate as possible. So the two approaches are aiming for two different problems. I still think my translation table is quite handy, and I did not find any other table like that on the web (yet). Perhaps, if this discussion comes to the conclusion that red with long wavelengths needs to have a more purple sRGB-hue than the sRGB primary red, you might want to change your picture according to it (if it doesn't make too much work). Anyway your picture already gives a better overview about the visible spectrum than the table. Jan 85.179.23.127 (talk) 10:12, 28 June 2008 (UTC)
- The trick in this picture is that the background is grey, rather than black. That way, you can make negative RGB values to cover colors outside the sRGB gamut. A possible criticism is that I'm not sure whether we used sRGB or NTSC primaries, and that a gamma of 2.5 was assumed rather than 2.2 (or the weird gamma-like function that is used in sRGB). Han-Kwang (t) 18:00, 29 June 2008 (UTC)
- Yes, I already suspected that the grey was used to expand the gamut; it is a nice way of solving the problem. Can you explain me why your program did not chose purple colors for "deep red", as my program and the images of the Green Flash page did? I don't think anymore that its as much neccessary to make a mixture with blue, but I would like to know if you explicitly prohibited blue colors appearing, or if the mechanism just got that results "naturally". The weird gamma-like function is corresponds almost to gamma 2.2, but contains a linear part to avoid numerical computation errors ("The effect of the above equations is to closely fit a straightforward gamma 2.2 curve with an slight offset to allow for invertability in integer math." ). Jan 85.179.15.103 (talk) 22:28, 29 June 2008 (UTC)
- Even if you can compensate the need to mix in additional blue with the grey background, that would mean you'd have to reduce green (i.e. "negative green"), if I overlook it correctly. But at 660nm I get #b8443d with a color picker (so more red and green than anything else, and more green than the background #414141). You can calculate linear (non-gamma corrected) sRGB values for 660nm light using the CIE standard colorimetric observer data for 2° (X, Y, Z) at 660nm, and the transfomration matrix of the sRGB standard to convert from XYZ values to linear RGB:
660nm: X=0.164900; Y=0.061000; Z=0.000000 R = 3.2410 * X - 1.5374 * Y - 0.4986 * Z = 0.4406595 G = - 0.9692 * X + 1.8760 * Y + 0.0416 * Z = -0.04538508 B = 0.0556 * X - 0.2040 * Y + 1.0570 * Z = -0.00327556
- You can see that the smallest value of the three RGB primaries is for green (actually negative), which is not the case in your image. Of course this might be changed when considering the Abney effect and/or other color appearance effects as adding grey can shift the perceived hue, but did your program creating the image as shown here consider this and/or other appearance effects? Jan 85.179.4.11 (talk) 09:50, 1 July 2008 (UTC)
- I do not read anywhere that they claim this to be "the" way to map wavelengths to sRGB. In general, such a kind of mapping seems handy, so this link or another one seems appropriate in the article: there is a somewhat similar link for wavelength-frequency conversion. A point of concern is the reliability and verifiability of this source: do you know a more reliable one which is verifiable? -- Crowsnest (talk) 15:55, 26 June 2008 (UTC)
- I had a brief look at the source code, but it is written in a fairly obscure language (i.e., not comprehensible by me) and the code is quite long, and it is not stated in simpler terms what it does (I assume it preserves the hue angle from a D65 white point, but the choice of white point is quite arbitrary). I do not know a more reliable/verifiable one, but as I explained above, I don't think a reliable mapping exists. Moreover, I don't think this type of external link belongs in the wavelength article anyway - next we'll get a DeBroglie calculator, water wave calculator, earthquake wave calculcator, gravity wave calculator and so on. Apart from that I'd like to mention that 85.179.9.12 (talk · contribs) and 85.179.18.203 (talk · contribs) are from the same German ISP and their whole contribution list consists of adding/discussing these links. The external links policy prohibits adding links to sites one is affiliated with, which seems quite likely here - they added the same link twice on different articles. Han-Kwang (t) 16:48, 26 June 2008 (UTC)
- Sorry, I was not aware of that policy. Remove the link, if you don't find it useful, or just copy the data to wikipedia according to the granted rights: "You may freely copy, modify, merge or use this data collection. I do not assure that the data is correct. Use at your own risk." The whitepoint is D65 according to the sRGB standard. That "obscure" language is Haskell (also mentioned in the first sentence of the introductory comment in the source), a very modern functional programming language. Jan 85.179.0.13 (talk) 19:29, 27 June 2008 (UTC)
- The fact that Haskell is very modern does not mean that it is not obscure. Obscure means unknown or difficult to understand, which I dare say is the case for the vast majority of people who do some kind of programming, including me. :-) Han-Kwang (t) 23:17, 27 June 2008 (UTC)
- Sorry, I was not aware of that policy. Remove the link, if you don't find it useful, or just copy the data to wikipedia according to the granted rights: "You may freely copy, modify, merge or use this data collection. I do not assure that the data is correct. Use at your own risk." The whitepoint is D65 according to the sRGB standard. That "obscure" language is Haskell (also mentioned in the first sentence of the introductory comment in the source), a very modern functional programming language. Jan 85.179.0.13 (talk) 19:29, 27 June 2008 (UTC)
- Functional programming is really tricky, if you have mostly imperative or object-oriented experiences. And as most people are using imperative or object-oriented languages, I agree that a program written in that language is not easy to comprehend for many people. But I think in university or computer science context Haskell is quite common, and I think it's one of the "standard" languages for functional programming. Jan 85.179.23.127 (talk) 23:40, 27 June 2008 (UTC)
- The reason for clipping the spectrum at some point, is that the CIE 1931 data has a bad resolution for the less visible wavelengths. Light with wavelengths abouve 690nm needs more than 100 times of the energy of light with 555nm appearing same bright . As the table contains only sRGB colors with maximum chroma (to get as close as possible to the true spectral color), using the wavelengths at the border of the visible spectrums would magnify the errors of the CIE 1931 data. Jan 85.179.23.127 (talk) 09:08, 28 June 2008 (UTC)
I've found another page, which is dealing with rendering of the visible spectrum in sRGB: http://mintaka.sdsu.edu/GF/explain/optics/rendering.html Jan 85.179.0.13 (talk) 22:12, 27 June 2008 (UTC)
- Interesting. At least he explains the pros and cons of various approaches, although all his diagrams show artifacts at the pure R/G/B primaries, but that could have to do with my current laptop monitor. I still don't agree with mixing in B into R to simulate deep red. At my job, I regularly deal with 800 nm light (and occasionally a 675 nm laser pointer) and they don't look purple/pink. Han-Kwang (t) 23:17, 27 June 2008 (UTC)
- In the discussion page for the color article I explained the reason why to use blue to create the impression of red with a longer wavelength than the sRGB primary red. However using this method reduces the chroma of the color. A line drawn from the spectral color with 700nm to the D65 white point in the CIE 1931 xy diagram will cross the purple line of sRGB. Expanding the hue from the point of the pure sRGB primary to the longer wavelengths leads to a wrong display of hue. I think it's better to make an error in chroma than making an error in hue, especially because with any RGB system (consisting of non-imaginary colors) you always have to reduce the chroma for most colors (especially for cyan or yellow, depending on the position of the green primary), but it's possible to give a correct representation of the hue for all colors (assuming displays and humans are working according to the standards ;-). Jan 85.179.23.127 (talk) 08:52, 28 June 2008 (UTC) and 85.179.23.127 (talk) 11:25, 28 June 2008 (UTC)
- I used a color picking tool on best_spectrum.jpg to determine the color of approx. 630nm and got #fe0046 as result. As #xx0000 red is often used in programs/documents, perhaps you can get used to the "orangish" sRGB-red while working on the computer, and get the impression that #ff0055 is too purplish. Also my monitor is not calibrated and the color appearing on my (consumer) LCD display varies depending on the viewing angle. Jan 85.179.23.127 (talk) 23:31, 27 June 2008 (UTC)
The problem with the magnetkern.de site's approach is that it ignores the Abney effect, projecting the intended hue along straight lines toward the white point. Subjectively, this is bad; the actual curves of constant perceptual hue would map pure reds to pretty near the sRGB primary; if any blue is needed to counter the orange, it's not nearly as much as these guys used based on straight lines. Here is a better book source and diagram. Dicklyon (talk) 17:27, 28 June 2008 (UTC)
- Do you know how to calculate the quantitative impact of this effect? There are a lot of other effects on color perception too. To take all of them into account, one would have to know the exact viewing environment (ambient light, size of the area, background color, absolute luminance, etc...). My program is also capable of using the CIECAM02 color appearance model for compensating many of that effects (that is one reason why it's so long), but I'm not sure, if the Abney effect is taken into account by CIECAM02, and if it's worth making the mechanism more complicated (and therefore less verifyable). I guess that would depend on the quantity of the impact. Another thing I would like to note, is that this problem is not limited to colors for wavelengths greather than 610nm, so just clipping at the edge of the gamut does not solve the problem, but makes it just less noticable. Jan 85.179.23.127 (talk) 17:48, 28 June 2008 (UTC)
- I don't have a quantitative formulation of the effect. I don't think CIECAM includes it either. What's seems clear is that you don't improve the hue of the red and blue primaries as representations of extreme wavelengths by adding some of the other; in both cases, you get a purple perception (OK, maybe a little could help, esp. on the violet end). The reason it's not much of an issue at other wavelengths is that nobody has a calibrated wavelength-to-hue perception; it's only when you get into the extra-spectral colors by mixing red and blue that it looks wrong. Dicklyon (talk) 18:27, 28 June 2008 (UTC)
- So I think as long as I don't have any quantitative information, I would not like to make "manual" adjustments on my own. But if you are right, then probably the (subjective) limit between red and orange might be really above 610nm. But I still think that 630nm (as shown in the Color article) is too high, as there are some LEDs sold with 625nm as peak wavelength (for example by Reichelt Elektronik, order code LED 5MM 2MA RT, german page, but english datasheet is online available), which would have to look orange then (as the wavelengths shorter than the peak wavelength would turn the color more yellowish and the wavelenghts longer than the peak wavelength would not compensate that effect). Jan 85.179.23.127 (talk) 18:46, 28 June 2008 (UTC)
- I agree that 630 is too long for the orange–red boundary. The only reason it's there is because that's the only accessible book source I could find; look for an alternative better source and we can change it. What I wanted to avoid though is making up unsourced numbers. Similarly, the pages of sRGB equivalents to wavelengths is essentially unsourced WP:OR. If we can find a WP:RS, we should include such a thing; otherwise not. Dicklyon (talk) 23:33, 28 June 2008 (UTC)
- Is it correct that the source for the 630nm boundary is Craig F. Bohren (2006). Fundamentals of Atmospheric Radiation: An Introduction with 400 Problems. Wiley-VCH. ISBN 3527405038? To me the title looks like the book is not a reference book for colorimetry but dealing with radiation in general, so I'm not sure if I would consider it trustworthy related to color appearance topics, which might not be the main purpose of the book. Jan 85.179.1.70 (talk) 18:19, 29 June 2008 (UTC)
- Yes, it read 625 before I changed it here. I didn't care about the particular numbers so much as tieing the table to a source, since people kept changing them. Find a better source and you can update it. Dicklyon (talk) 18:36, 29 June 2008 (UTC)
- I have read about the vandalism on the Color article, but I didn't knew that sticking to 630nm was related to that. Jan 85.179.1.70 (talk) 19:36, 29 June 2008 (UTC)
- Not vandalism, just many well-intentioned improvement opinions without any sources. Here are some places to look for a good alternative set of numbers. Maybe this one will do. Good luck. Dicklyon (talk) 20:29, 29 June 2008 (UTC)
- Both books and free software may have errors, but compared to a data-table from a book, with an open source program it is at least possible for people to review what happens (though not for all people, I also had a lot of time difficulties reading sources written by others). I included literature references in my program to make it verifyable. If you think that following the references on my page is too complicated, and if you don't want to link to a page, which you don't think is completely verifyable, then you have no other choice than removing the link. But I would also like to note that the second (Green Flashes) page got results very similar to those on magnetkern.de, so in my (probably biased) oppinion it's quite unlikely that both sources are erroneous. However I don't want to question wikipedia policies, I just wanted to provide a handy translation table, which anyone can use. In any case this discussion helped me improving my page, so thank you for the hints! Jan 85.179.1.70 (talk) 18:50, 29 June 2008 (UTC)
- I have added the following notice to my page: "The reduction of chroma corresponds to a mixture of the spectral color with white light (D65, according to sRGB), which may cause a different percieved hue compared to the true spectral color." So now it's more clear how the values were calculated, and that not all effects related to color appearance have been taken into account, as the reduction of chroma was done in a "physical" way, rather than using a more complicated and less well-defined "biological/psychological" way. Jan 85.179.23.127 (talk) 20:06, 28 June 2008 (UTC)
- You should add that the result is that the colors are too purple at both ends due to the Abney effect. Dicklyon (talk) 23:33, 28 June 2008 (UTC)
- You finally convinced me, that the appearance effects should not be ignored. I modified my program to use both ways of increasing/reducing chroma: "physical" mixture with white light and/or using a color appearance model to map saturated sRGB colors to almost monochromatic colors (only 1% white) while keeping the perceived hue constant. I like to use the CIECAM02 model, because according to the book "Color Appearance Models" from Mark D. Fairchild (2005) the CIECAM02 and the Hunt model are the best choices: ", if further sophistication is required, CIECAM02 would be the best choice. Lastly, if CIECAM02 is not adequate for the given situation, then the Hunt model would be the most comprehensive choice". However he also states that the Hunt model is rather complex: "Its complexity makes application of the Hunt model to practical situations range from difficult to impossibe." There have been two reasons for using a 99% saturated color, instead of a 100% saturated color: First working on the absolut limit can induce numerical calculation errors and second it also conforms to real life, as a screen reflecting a laser always reflects a bit of white surrounding light too (unless being in a complete dark room). As I stated on my page too, the choice which color appearance model to use is not prescribed by the sRGB standard but has to be done by considerations of the properties of the existent models. I updated my page to mention the advantages and disadvantages of the two approaches, and to list the results of both calculations. I was surprised about the impact of the previously non-considered effects: "Deep red" now is mapped to #ff002c instead of #ff0055 (according to the prediction of Dicklyon, that less blue was neccessary than I used before). But also in the middle of the spectrum you can notice the differences: With physical chroma reduction #00ff6c would correspond to 542 nm, and using CIECAM02 #00ff6c corresponds to 530 nm. Jan 85.179.1.70 (talk) 14:29, 29 June 2008 (UTC)
Btw, some time ago, before this discussion started and before I created the mapping table, I looked incidentally at an alarm clock in a dark room, which had a 7-segment LED display, and I was surprised how "deep red" it looked like. I was thinking that the computer red looks more orange than this LED display. This was of course just my personal impression. It was probably using a GaAlAs semiconductor with 660nm peak wavelength (also shown in the datasheet I referred to above). Jan 85.179.23.127 (talk) 18:56, 28 June 2008 (UTC)
- Yes, 660 nm is going to be about the deepest red you can get. Longer wavelengths just get dimmer, continuing to stimulate only the L cones. Which brings up my theory of "campfire vision": when you sit around a campfire, after the flames are gone and it's all red and orange, the radiation is stimulating a mixture of rods and L cones mostly, and depending on where you look, in, near, or away from the fire (and some M cones when you look right at the orange parts). You get a strange sort of 2D color space, like you're somewhat color blind, but not along the usual opponent dimensions. Has anyone looked into something like this? Dicklyon (talk) 23:33, 28 June 2008 (UTC)
- I think it's the same effect you notice when using a colored light for illumination (i.e. an orange LED cluster). Jan 85.179.1.70 (talk) 14:45, 29 June 2008 (UTC)
- Colored light, maybe, but LED, no. You need a broad enough spectrum for this effect, I think, such that you get some color; a single narrow LED won't do that. Dicklyon (talk) 15:36, 29 June 2008 (UTC)
- Yes you are right, probably an LED might be too narrow, though LEDs are not completely monochromatic. Jan 85.179.1.70 (talk) 17:06, 29 June 2008 (UTC)
Using the most authoritative sources you can find (CIE and W3C/sRGB), I was able to show with a single matrix multiplication that the image from Han-Kwang is either wrong or contains non-documented perceptual compensations (unless someone can show where and how the compensation is done, see above). It is also quite questionable that the 630nm "boundary" on the Color article between red and orange is correct, as Dicklyon already confirmed. I can understand wanting to keep the wavelength table in the color article stable (i.e. based on "trustworthy" books), even if we know one value is wrong. I also don't see any reason to remove the image of Han-Kwang, because it still gives a very good overall impression of the wavelengths of the visible spectrum. And I did not see any verifyable argument that the content of my page is wrong, except for the correct objection from Dicklyon related to perceptual effects, which are now taken into account on the page, while mentioning both the advantages and disadvantages. The page does not claim to be more authoritative than it is: "The calculations were done using a self-written program. The source code contains references to literature for the used constants and equitations." I'd like to hear your comments. Jan 85.179.4.11 (talk) 13:25, 1 July 2008 (UTC)
Work needed to treat more general waveforms
A beginning on this topic has been made, but more is needed. Brews ohare (talk) 00:43, 11 June 2009 (UTC)
- The concept of wavelength is most often applied to sinusoids. More is only needed if we have good sources that make the more general case seem important. Dicklyon (talk) 00:47, 11 June 2009 (UTC)
- I did some looking, and found that that concept is used in ocean engineering and such; it doesn't work in linear dispersive media, but does for water surface waves. So I made a section an added a ref. Dicklyon (talk) 01:24, 11 June 2009 (UTC)
- A general waveform can be found almost anywhere, especially if you are looking at spatial variation. IMO it is nutso to present results for a specialized case when they are very simply derived for a general case that actually makes the concepts clearer and promotes some imagination about their usefulness. Brews ohare (talk) 03:31, 11 June 2009 (UTC)
- In physics, the more usual view is that a general waveform is composed of a sum of sinusoidal waves. The wavelengths of a general waveform are the wavelengths of its component sinusoids, which are given by Fourier analysis. One can only meaningfully talk about the wavelength of a general waveform if the medium in which it propagates is non-dispersive, since in a dispersive medium a non-sinusoidal waveform changes shape as it propagates.--Srleffler (talk) 04:16, 11 June 2009 (UTC)
- These points are raised in the article already. Brews ohare (talk) 14:40, 11 June 2009 (UTC)
- A general waveform can be found almost anywhere, especially if you are looking at spatial variation. IMO it is nutso to present results for a specialized case when they are very simply derived for a general case that actually makes the concepts clearer and promotes some imagination about their usefulness. Brews ohare (talk) 03:31, 11 June 2009 (UTC)
Brews, your re-write of the "general waveforms" section introduces the general traveling wave, supported by a book that does not mention the word wavelength at all, as far as I can tell. It also states without source that "Such waves occur in music and picture reproduction systems, in many digital circuits, in radio transmission and reception, and in waveguides," which is somewhere between untrue and misleading. I challenge you to find any source that indicates the concept of wavelength being applied to general waveforms in such systems. Dicklyon (talk) 14:33, 11 June 2009 (UTC)
- The book is not cited as a reference to wavelength, but to the mathematical expression for the periodic form of the traveling wave. Brews ohare (talk) 14:40, 11 June 2009 (UTC)
- Yes, I understand that. But you've added a bunch of complicating material to the wavelength article, when treatment of the concept of wavelength in sources seldom involves such complications. You've supported the complicating material by a book that doesn't even mention wavelength. Like I said when you first asked, the concept of wavelength is most often applied to sinusoidal waves, and if you want to say much about the more general case, you really ought to support that by something better than a throwaway line in a figure caption and such. That's why I found the book on ocean waves, where the shapes that propagate really are non-sinusoidal. Dicklyon (talk) 15:08, 11 June 2009 (UTC)
Hi Dick: It's great to be simple, but the subject has many ramifications, and it is not up to you to delimit what is too complex for inclusion. In fact, I'd argue that the general waveform is easier to understand than the sinusoidal one, and presents the connection of wavelength to velocity and frequency more transparently. I'd guess that you simply learned the material your way, and don't want to think it through in a different context.
The cited sources are much more than " throwaway lines", and if you disagree, provide some meat for your decisions, not arbitrary judgment calls. The source that "doesn't mention wavelength" was cited for the reasons mentioned above, which you apparently did not read.
For example, why cut the explained Wikilink to Fourier series, which connects the general and sinusoidal forms? Why cut the Wikilink to the cnoidal wave where a thorough discussion of an example of a periodic traveling wave is made? Why not provide your interpretation of the ocean wave source, which I read as saying the waveform is preserved because all components travel at the same speed? (In fact, how else can the waveform stay the same?) Brews ohare (talk) 15:42, 11 June 2009 (UTC)
- Linking cnoidal wave would be OK, but the book source didn't mention wavelength anywhere need the discussion of the cnoidal wave. The "throwaway" I was referring to was in the caption of the figure 4.1 that you mention, where it says "complex waves, which nevertheless have distinct frequencies, or wavelengths." This ignores the fact that the usual "general" analysis, at least in linear systems, is in terms of sinusoids, since different frequencies typically have different velocities and there therefore the wave shape doesn't propagate unchanged. If you want to beef up the article, that's what really needs to be clarified, instead of pretending that the concept of wavelength is typically applied to complex waves. As for you "general case", please add it only if you find a source that connects it to the concept of wavelength. Dicklyon (talk) 19:25, 11 June 2009 (UTC)
- You have re-inserted the errors about the ocean waves. This is not a linear system and does not propagate sinusoidal components. The notion of sinusoidal components is pretty irrelevant to how such a nonlinear system propagates wave shapes. Dicklyon (talk) 19:30, 11 June 2009 (UTC)
Ocean waves
Here is what the source Dicklyons introduced says about ocean waves:
"While maintaining this waveform, waves travel at a velocity determined by the wavelength. A wave number spectrum resulting from Fourier transform of this non-sinusoidal waveform contains not only the wave number corresponding to wavelength L, kL = 2π/L, but also harmonic components such as 2πkL, 3πkL, and so on. From the fact that waves maintain their waveform during travel, it is understood that all of these wave number components travel at the same velocity as waves with wave number kL.
Dicklyons deleted the following entry in the article that refers to these same waves and cites this same source:
In ocean waves the wavelength components all travel at the same velocity to maintain the shape of the waveform. This velocity is determined by the wavelength of the longest wavelength component.
with this supporting remark: "You have re-inserted the errors about the ocean waves. This is not a linear system and does not propagate sinusoidal components. The notion of sinusoidal components is pretty irrelevant to how such a nonlinear system propagates wave shapes."
Question 1: In what way does the meaning of the cited source depart from that of the reverted text? (My answer: in no significant manner.)
Question 2: Where does the topic of nonlinear systems appear outside of Dicklyon's comments? (My answer: the topic has never arisen in any text in the article.)
Question 3: Which is preferable: reinsertion of the brief and accurate reverted material, or an extended quotation from the cited source that says the same thing? Perhaps the reverted text should be slightly amended to refer to "ocean waves of large amplitude" to correspond more exactly to the source. Brews ohare (talk) 09:11, 14 June 2009 (UTC)
- I think it's a matter of interpretation. In the ref, they take the fact that the non-sinusoidal wave propagates while maintaining its shape, and from that conclude that if you analyse it into Fourier components, then those have to all travel at the same speed. The way you wrote suggests the other way around, that the medium propagates components at the same speed, and hence the waveshape remains constant. However, if you were to look at the small-signal linear behavior, you'd see that different sinusoidal components do NOT propagate at the same speed, except in very shallow water. It's only the nonlinearity that makes them appear to synchronize their speeds. To describe such a nonlinear medium in terms of its propagation of sinusoidal components is nonsense. The topic of nonlinearity is appropriate here, because it's the only context (as far as I've found) in which it makes sense to talk about the wavelength of non-sinusoidal waves, instead of just sticking to sinusoids, which are the eigenwaves of linear systems. Dicklyon (talk) 17:16, 14 June 2009 (UTC)
- Dick: Thanks for the reasoned response. The bottom line is that the various wavelengths travel at the same speed. The mechanism by which this result is made to occur may well involve some interesting aspects of nonlinear interactions, but the detailed explanation seems more than necessary here. I'd suggest that very little or no rewording of the reverted text is adequate.
- I'll make a proposal in two or three days, when I've more time. Brews ohare (talk) 02:01, 15 June 2009 (UTC)
- "The bottom line is that the various wavelengths travel at the same speed" is basically just your interpretation. There is only one wavelength involved – there is no periodic wave or wavelength associated with the harmonics. The fact that for the purpose of radar reflection these waves can be analyzed into different wavenumber components by a Fourier analysis is not a reason for us to state that different wavelengths propagate at the same speed, which is in fact very misleading. Dicklyon (talk) 02:17, 15 June 2009 (UTC)
Dick: Can you explain to me how your statement reconciles with your source that says: "In ocean waves the wavelength components all travel at the same velocity to maintain the shape of the waveform."? Brews ohare (talk) 16:22, 15 June 2009 (UTC)
- Brews: the issue here, is that Fourier theory doesn't work in nonlinear systems. You just can't take a wave propagating in a nonlinear medium, decompose it into Fourier components, and talk about the propagation velocity of those components. The assumptions underlying Fourier theory fail in this case, and the velocities and components the math gives you have no basis in physical reality at all. Dick is essentially arguing that the source quoted above is incorrect. The wave propagates without changing shape, but this does not imply that there are actually sinusoidal components with different wavelengths, which propagate at the same speed. Checking the dispersion relation for the medium will immediately show that the latter cannot be the case.--Srleffler (talk) 04:45, 16 June 2009 (UTC)
- What fails isn't the Fourier analysis, but the notion that the components are somehow separately treatable. The source was not incorrect in what it said, however; the paragraph at the top of p.263 deduces that components in a wavenumber spectrum propagate at the same speed from the fact that the wave maintains its shape; in that context, the wavenumber spectral decomposition is part of the radar reflection calculation, and he doesn't say anything about different wavelengths of the ocean wave itself; indeed, while a wavenumber spectrum makes some sense, a wavelength spectrum doesn't really, as the wave only repeats at the wavelength, not at submultiples of it. The source does not mention or suggest that there are wavelengths associated with the Fourier components, as that would not be a useful or very sensible notion in this context. One could argue that the wavenumbers correspond to wavelengths, but that source doesn't do that, for the reasons just stated, I think. Dicklyon (talk) 05:06, 16 June 2009 (UTC)
- I see. So Brews is assuming incorrectly that where there is a wavenumber, there must be a wavelength.--Srleffler (talk) 05:22, 16 June 2009 (UTC)
- I'm not saying that's necessarily incorrect, but it's certainly not appropriate here, and is not suggested by the source. Dicklyon (talk) 05:27, 16 June 2009 (UTC)
Reply to Srleffler
Statement by Srleffler: "Fourier theory doesn't work in nonlinear systems. You just can't take a wave propagating in a nonlinear medium, decompose it into Fourier components, and talk about the propagation velocity of those components."
- This is an incorrect view. Fourier analysis is simply a mathematical decomposition that replaces a function with a sum of simpler functions, sinusoids for example. Being a mathematical procedure it does not rely upon any dynamics or physical theory, and is not predicated upon linearity. Thus, at each moment in time the waveform can be expressed by a sum of the basis functions, and if the waveform propagates rigidly, the basis functions also can be taken to so propagate, in which case the coefficients of the decomposition will be time independent. If you insist upon a full mathematical development of these facts, I will provide one. But my position is that you are confusing math with physics or vice versa. Brews ohare (talk) 01:45, 17 June 2009 (UTC)
- I pretty much agree with that assessment, as I was explaining above, but providing a full development of Fourier theory won't do a thing to make the inclusion of your concepts in this article more acceptable. Dicklyon (talk) 02:36, 17 June 2009 (UTC)
- I admit the error in describing the math, however waves are a physical entity and I insist that an article on them deal with physics, not math when the two depart from one another. The decomposition of a wave in a nonlinear medium into sinusoidal components is unphysical, in that those components do not propagate the same as an isolated wave with the same frequency would. --Srleffler (talk) 04:35, 17 June 2009 (UTC)
- There is no need to arbitrarily insist on a particular description, or to forgo either physics or math on some general basis. If you would like to insist that a pure sinusoid would not propagate as a traveling wave, but would evolve into a sum of multiple sinusoids, that simple statement could be added with a suitable source for backup. Brews ohare (talk) 04:51, 17 June 2009 (UTC)
There is no need to develop Fourier series in the article; it was simply suggested FYI, in case there was disagreement. IMO acceptance of the above argument makes refusal to accept the reverted text a complete non sequitor. I will attempt to formulate that for you in an A -> B -> C fashion, if you like. Brews ohare (talk) 04:03, 17 June 2009 (UTC)
Reply to Dicklyon
Statement by Dicklyon: "the paragraph at the top of p.263 deduces that components in a wavenumber spectrum propagate at the same speed from the fact that the wave maintains its shape; in that context, the wavenumber spectral decomposition is part of the radar reflection calculation, and he doesn't say anything about different wavelengths of the ocean wave itself"
- The belief that the source "doesn't say anything about different wavelengths" is an incorrect interpretation of the source. If a fixed shape waveform traveling in time is Fourier analyzed into a sum of basis functions that propagate at the same rate as the traveling wave, the decomposition coefficients are time independent. There is no requirement that the underlying physics or the medium be linear. The mathematical requirement is only that the the wave be a traveling wave. If the wave is a traveling periodic wave, as envisioned by the source, and a Fourier series in sinsusoids is used, the basis functions all will be harmonics of the fundamental. Each harmonic corresponds to a wavenumber, which simply is the analog in space of frequency in time. Your objections to the reverted text are thus based upon a misconception that there is physics involved here, where it is only math. Brews ohare (talk) 02:17, 17 June 2009 (UTC)
- No, my objections to the reverted text are based on the fact that it is your own synthesis of concepts, not something you'll find in a source. Why are you pushing to include this strange text? Dicklyon (talk) 02:33, 17 June 2009 (UTC)
Dick: I am not synthesizing concepts; I am summarizing the source you provided. If you prefer, I will quote it at length directly, which will allow those who understand it to understand it, and those who don't to preserve their misconceptions. Brews ohare (talk) 04:03, 17 June 2009 (UTC)
My edit
The removal of "eigenfunction" was deliberate: Wavelength is a basic concept. This article should try to stay at a level where an intelligent 11 year old can understand it. Concepts like eigenfunctions should not be introduced so early in the article, if at all. I like the new wording, which gets the concept in there, but avoids the word.--Srleffler (talk) 04:51, 16 June 2009 (UTC)
- Right, but you removed the reason why sine waves are used, which is that they are the unique shapes, in linear time-invariant systems, that propagate unchanged. So I added that in plainer language. Dicklyon (talk) 04:57, 16 June 2009 (UTC)
I wonder if the reason for using sinusoids is correctly identified. In the media you describe, I suspect that all wave shapes propagate unchanged, inasmuch as they are composed of sinusoids that themselves so propagate, provided only that the speed of propagation is independent of wavelength. Brews ohare (talk) 05:14, 17 June 2009 (UTC)
- That would be true only in linear non-dispersive media; in water surface waves, waves of different wavelengths propagate at very different speeds, as one of the refs I pointed out shows. In nonlinear media, only certain shapes will propagate unchanged. Sinusoids are used in the case of linear media, dispersive or not, since the dispersion relation (between wavelength or wavenumber and velocity) completely determines how waves propagate. In nonlinear media, decomposing into sinusoids serves no such function; one might as well describe periodic waves in terms of Haar-function basis. To speak of sinusoidal components in nonlinear media really serves little purpose, in general. Dicklyon (talk) 06:08, 17 June 2009 (UTC)
- What did you think was a reason for using sinusoids? Why are you pushing sinusoidal decomposition? Dicklyon (talk) 06:10, 17 June 2009 (UTC)
I'm pushing sinusoidal decomposition because it is the principal justification for treating sinusoids at all, which seems to be your primary topic. Brews ohare (talk) 14:42, 17 June 2009 (UTC)
- That was a perfectly circular statement. And what are saying is my primary topic? Dicklyon (talk) 05:07, 18 June 2009 (UTC)
Language of discussion
Here is roughly how I'd approach the topic:
The simplest mathematical description of a traveling wave in one dimension is:
where y is the wave amplitude and f(x) is any function whatsoever. (Maybe some interpretation here, like: "a point xt = x-vt moves with speed v, causing the position of the corresponding amplitude y = f(xt) in the wave to travel with speed v"). If f(x + λ ) = f(x) for some λ, a wavelength may be defined and the traveling wave is called periodic. Under some rather general mathematical conditions, regardless of whether a wavelength can be defined:
where the set φn are referred to as basis functions. Using this decomposition, the traveling wave can be expressed as:
and each basis function φ travels at the wavespeed v. If the wave is periodic, the set φn can be chosen as sinusoidal waves, where the wavelength of each component wave is a multiple of that of the fundamental (longest wavelength) basis function and the basis functions often are called harmonics of the fundamental.
The application of this description to physical examples would follow. That might include a demo that the wave equation has such solutions, how that equation evolves in different contexts, and so forth. All depends upon how far one wishes to extend the simple idea of wavelength as expressed in f(x + λ) = f(x) into the subject of waves and wave equations (which is an extension, I'd say). Brews ohare (talk) 12:43, 17 June 2009 (UTC)
- Pardon me if you already understand this, but this treatment works only for waves in a linear, dispersionless medium. In most media, y also has direct dependence on t:
- This reflects the fact that the wave changes shape as it propagates. (Waves may also of course decay or have gain.) In a linear medium, you can describe how y evolves with time by considering a decomposition on a basis of sinusoidal waves with distinct velocities determined by the dispersion relation of the medium. Decomposing on a basis of sinusoidal waves that all propagate with the same velocity will not correctly describe the time evolution of the wave, in general.-Srleffler (talk) 03:56, 18 June 2009 (UTC)
- Pardon me in turn, but this formulation is entirely mathematical. As such it stands on purely logical ground, and like all mathematics, may or may not have application to any particular physical situation. It simply is misguided to believe that math only works when there exists a physical application.
- To stimulate some thought on your part, I challenge you to point out one place in the above math where linearity or lack of dispersion is invoked or is necessary without entering into a particular physical application. Brews ohare (talk) 09:29, 18 June 2009 (UTC)
- I'm not arguing your math is wrong. I am arguing that you have chosen the wrong mathematical model for the physical system you seek to describe. The equation does not accurately describe wave propagation in real, physical media. It is a naive model, a lie-to-children. There are exceptional cases in which waves propagate without changing shape or amplitude, but these are rare special cases.--Srleffler (talk) 17:30, 18 June 2009 (UTC)
- So simple cases are "lies to children"? We are talking wavelength here, and wave motion is mentioned mainly as illustration of wavelength. Simple cases are a good start. Brews ohare (talk) 19:09, 18 June 2009 (UTC)
As pointed out before, the decomposition into sinusoids is only useful for linear systems, such that the time evolution of the system can be described in terms of the time evolution of the components, added up; it works because sinusoids are special: they are the eigenfunctions of continuous-time linear systems (actually, complex exponentials are, but close enough). In the case of periodic non sinusoidal waves propagating, the linear system that will do that has to be pretty trivial, namely dispersionless, in which case the sinusoidal decomposition is hardly interesting or informative. And for real physical systems that will propagate waves without changing their shape, they tend to be nonlinear, in which case there is no longer any physical or mathematical motivation to consider sinusoidal analysis of the waves. Furthermore, even writing the general form of the traveling wave goes beyond what's needed to clearly explain the idea of wavelength, and no source that I've found couples that mathematical general form with the notion of wavelength. Without sources that couple these things, why would we put them into the article? Brews, you've even added "all the components travel at the same rate, which insures that the waveform remains unchanged as it moves," which is totally backward logic; you deduced the equal speeds of the components from the fact that the wave travels as a fixed shape, which is a trivial and useless result; you can turn it around and use it show that it "insures that the waveform remains unchanged as it moves"! Dicklyon (talk) 04:59, 18 June 2009 (UTC)
- There is no validity to the claim you are making about "sinusoids are special" and about "eigenfunctions of continuous-time linear systems". The whole subject is simply one of a complete set of basis functions, which transcends any concept of eigenfunctions, although eigenfunctions (e.g. of a Sturm-Liouville equation) are included as special cases. How these basis functions propagate is determined by the mathematical form (e.g. f(x - vt)) not by physics.
- Of course, in any particular application to a physical problem one has to ask whether the math is applicable.
- Again, your viewpoint is based upon a physical situation. The math stands independently, and makes no presumptions about linearity. This is not a superposition of forces or excitations: it is a superposition of functions pure and simple. From a physical standpoint there are a number of examples where the math works. You have named linear systems, but you also have named some special cases of nonlinear systems where it also works. Whether math applies to a physical problem is not about math, it is about the physics. This formulation is math, pure and simple. Brews ohare (talk) 09:29, 18 June 2009 (UTC)
- This is a physics article. If the math does not describe the physical systems under discussion, it is not suitable for inclusion in the article. --Srleffler (talk) 17:35, 18 June 2009 (UTC)
- Sorry, Srleffler. There is no requirement that this be strictly a physics article. In fact, most physics articles contain quite a bit of math. The math is quite general and applies to many physical systems. It is not clear to me why the article should be restricted to "physical systems under discussion" where the math doesn't apply. That would eliminate the wave equation, for example. Brews ohare (talk) 19:07, 18 June 2009 (UTC)
Brews, it would be helpful if you would not keep adding such irrelevant and misleading stuff, against the consensus of other knowledgeable editors. Instead, maybe you can explain the point of it. How does it help to clarify the concept of wavelength to discuss mathematical decompositions of things that have wavelengths? Do you know any sources that do anything resembling that? Dicklyon (talk) 05:05, 18 June 2009 (UTC)
- This "stuff" is neither misleading nor irrelevant; rather the shoe is on your foot. The logical exposition is to start with f(x-vt), move to a periodic traveling wave to define wavelength as f(x+λ-vt) = f(x-vt) = f(x-v(t+T)) -> λ = vT and then introduce a basis set and show the basis functions may have higher periodicity. Sine waves are introduced as a particular case. Your argument is that the general case is "irrelevant" because you can identify the phenomena in a special case (the sinusoid). That is like saying Shakespeare is irrelevant because all the elements are in Nancy Drew. Brews ohare (talk) 11:02, 18 June 2009 (UTC)
- Who does it this way? What is the logical role of the basis decomposition here? I don't understand what you're saying about sinusoids here; why would this particular case be interesting or relevant? Dicklyon (talk) 14:11, 18 June 2009 (UTC)
- I've broken the subject up into bite size pieces to make the relevance clear and added several references to the approach. I hope that it works now. Brews ohare (talk) 19:11, 18 June 2009 (UTC)
- One source of relevance is to make connection to the notion of dispersion: a general waveform propagates unchanged only if its component parts can so propagate. To understand that, one has to introduce the notion of component parts. Brews ohare (talk) 19:41, 18 June 2009 (UTC)
- The result remains nonsensical, since the idea of components propagating only makes sense in linear media. Your statement that "Such circumstances sometimes do occur in nonlinear media" is misleading, or downright wrong, if by "such circumstances" you mean "that the medium must be capable of propagating disturbances of different wavelengths at the same wave speed". Nonlinear media typically do NOT have that property, yet can propagate periodic non-sinusoidal signals (only of particular shapes, though -- they can't propagate sinusoids, for example, at non-trivial amplitude). Dicklyon (talk) 01:00, 19 June 2009 (UTC)

 here, instead of
here, instead of  ? Or perhaps mentioning that the formula can be used with other waves by replacing the speed of light with the speed of the individual wave?
--
? Or perhaps mentioning that the formula can be used with other waves by replacing the speed of light with the speed of the individual wave?
--


