| Revision as of 18:47, 21 September 2009 view sourceJimWae (talk | contribs)Extended confirmed users, Pending changes reviewers, Rollbackers37,709 edits Undid revision 314288932 by Matt Crypto-- only caption needed is "an hourglass" -- Try again - this part is still relevant to & introduces article← Previous edit | Revision as of 09:51, 22 September 2009 view source Nicholomothy (talk | contribs)11 edits →Time dilationNext edit → | ||
| Line 297: | Line 297: | ||
| {{main|Time dilation}} | {{main|Time dilation}} | ||
| "Time is nature's way of keeping everything from happening at once". This quote, attributed variously to ], ], and ], says that time is what separates ]. Einstein showed that people travelling at different speeds, while agreeing on cause and effect, will measure different time separations between events and can even observe different chronological orderings between non-causally related events. Though these effects are typically minute in the human experience, the effect becomes much more pronounced for objects moving at speeds approaching the speed of light. Many ]s exist for only a fixed fraction of a second in a lab relatively at rest, but some that travel close to the speed of light can be measured to travel further and survive much longer than expected (a ] is one example). According to the ], in the high-speed particle's ], it exists, on the average, for a standard amount of time known as its ], and the distance it travels in that time is zero, because its velocity is zero. Relative to a frame of reference at rest, time seems to "slow down" for the particle. Relative to the high-speed particle, distances seem to shorten. Even in Newtonian terms time may be considered the fourth dimension of motion; but Einstein showed how both temporal and spatial dimensions can be altered (or "warped") by high-speed motion. | "Time is nature's way of keeping everything from happening at once". This quote, attributed variously to ], ], and ], says that time is what separates ]. Einstein showed that people travelling at different speeds, while agreeing on cause and effect, will measure different time separations between events and can even observe different chronological orderings between non-causally related events. Though these effects are typically minute in the human experience, the effect becomes much more pronounced for objects moving at speeds approaching the speed of light. Many ]s exist for only a fixed fraction of a second in a lab relatively at rest, but some that travel close to the speed of light can be measured to travel further and survive much longer than expected (a ] is one example). According to the ], in the high-speed particle's ], it exists, on the average, for a standard amount of time known as its ], and the distance it travels in that time is zero, because its velocity is zero. Relative to a frame of reference at rest, time seems to "slow down" for the particle. Relative to the high-speed particle, distances seem to shorten. Even in Newtonian terms time may be considered the fourth dimension of motion; but Einstein showed how both temporal and spatial dimensions can be altered (or "warped") by high-speed motion. | ||
| (Time dilation-- | |||
| Time dilation | |||
| Jump to:navigation, search | |||
| This article needs additional citations for verification. | |||
| Please help improve this article by adding reliable references. Unsourced material may be challenged and removed. (May 2008) | |||
| Contents | |||
| 1 Overview of time dilation | |||
| 2 Simple inference of time dilation due to relative velocity | |||
| 3 Time dilation due to relative velocity symmetric between observers | |||
| 3.1 Temporal coordinate systems and clock synchronization | |||
| 4 Overview of time dilation formulae | |||
| 4.1 Time dilation due to relative velocity | |||
| 4.2 Time dilation due to gravitation and motion together | |||
| 5 Experimental confirmation | |||
| 5.1 Velocity time dilation tests | |||
| 5.2 Gravitational time dilation tests | |||
| 5.3 Velocity and gravitational time dilation combined-effect tests | |||
| 5.4 Muon lifetime | |||
| 6 Time dilation and space flight | |||
| 6.1 Time dilation at constant acceleration | |||
| 6.2 The spacetime geometry of velocity time dilation | |||
| 7 See also | |||
| 8 References | |||
| 9 External links | |||
| 9.1 tags | |||
| This article discusses a concept in physics. For the concept in sociology, see time displacement. | |||
| Time dilation is a phenomenon (or two phenomena, as mentioned below) described by the theory of relativity. It can be illustrated by supposing that two observers are in motion relative to each other, and/or differently situated with regard to nearby gravitational masses. They each carry a clock of identically similar construction and function. Then, the point of view of each observer will generally be, that the other observer's clock is in error (has changed its rate). | |||
| There are two kinds of time dilation, and both can operate together. | |||
| Overview of time dilation | |||
| Time dilation can arise from (1) relative velocity of motion between the observers, and (2) difference in their distance from gravitational mass. | |||
| (1) In the case that the observers are in relative uniform motion, and far away from any gravitational mass, the point of view of each will be that the other's (moving) clock is ticking at a slower rate than the local clock. The faster the relative velocity, the more is the rate of time dilation. This case is sometimes called special relativistic time dilation. It is often interpreted as time "slowing down" for the other (moving) clock. But that is only true from the physical point of view of the local observer, and of others at relative rest (i.e. in the local observer's frame of reference). The point of view of the other observer will be that again the local clock (this time the other clock) is correct, and it is the distant moving one that is slow. From a local perspective, time registered by clocks that are at rest with respect to the local frame of reference (and far from any gravitational mass) always appears to pass at the same rate. | |||
| (2) There is another case of time dilation, where both observers are differently situated in their distance from a significant gravitational mass, such as (for terrestrial observers) the Earth or the Sun. One may suppose for simplicity that the observers are at relative rest (which is not the case of two observers both rotating with the Earth -- an extra factor described below). In the simplified case, the general theory of relativity describes how, for both observers, the clock that is closer to the gravitational mass, i.e. deeper in its "gravity well", appears to go slower than the clock that is more distant from the mass (or higher in altitude away from the center of the gravitational mass). That does not mean that the two observers fully agree: each still makes the local clock to be correct; the observer more distant from the mass (higher in altitude) makes the other clock (closer to the mass, lower in altitude) to be slower than the local correct rate, and the observer situated closer to the mass (lower in altitude) makes the other clock (farther from the mass, higher in altitude) to be faster than the local correct rate. They agree at least that the clock nearer the mass is slower in rate, and on the ratio of the difference. This is gravitational time dilation. | |||
| In Albert Einstein's theories of relativity, time dilation in these two circumstances can be summarized: | |||
| In special relativity (or, hypothetically far from all gravitational mass), clocks that are moving with respect to an inertial system of observation are measured to be running slower. This effect is described precisely by the Lorentz transformation. | |||
| In general relativity, clocks at lower potentials in a gravitational field — such as in closer proximity to a planet — are found to be running slower. The articles gravitational time dilation and gravitational red shift give a more detailed discussion. Special and general relatistic effects can combine, for example in some time-scale applications mentioned below. | |||
| Thus, in special relativity, the time dilation effect is reciprocal: as observed from the point of view of either of two clocks which are in motion with respect to each other, it will be the other clock that is time dilated. (This presumes that the relative motion of both parties is uniform; that is, they do not accelerate with respect to one another during the course of the observations.) | |||
| In contrast, gravitational time dilation (as treated in general relativity) is not reciprocal: an observer at the top of a tower will observe that clocks at ground level tick slower, and observers on the ground will agree about that, i.e. about the direction and the ratio of the difference. There is not full agreement, all the observers make their own local clocks out to be correct, but the direction and ratio of gravitational time dilation is agreed by all observers, independent of their altitude. | |||
| Simple inference of time dilation due to relative velocity | |||
| Observer at rest sees time 2L/c | |||
| Observer moving parallel relative to setup, sees longer path, time > 2L/c, same speed cTime dilation can be inferred from the observed fact of the constancy of the speed of light in all reference frames. | |||
| This constancy of the speed of light means, counter to intuition, that speeds of material objects and light are not additive. It is not possible to make the speed of light appear faster by approaching at speed towards the material source that is emitting light. It is not possible to make the speed of light appear slower by receding from the source at speed. From one point of view, it is the implications of this unexpected constancy that take away from constancies expected elsewhere. | |||
| Consider a simple clock consisting of two mirrors A and B, between which a light pulse is bouncing. The separation of the mirrors is L, and the clock ticks once each time it hits a given mirror. | |||
| In the frame where the clock is at rest (diagram at right), the light pulse traces out a path of length 2L and the period of the clock is 2L divided by the speed of light: | |||
| From the frame of reference of a moving observer traveling at the speed v (diagram at lower right), the light pulse traces out a longer, angled path. The second postulate of special relativity states that the speed of light is constant in all frames, which implies a lengthening of the period of this clock from the moving observer's perspective. That is to say, in a frame moving relative to the clock, the clock appears to be running more slowly. Straightforward application of the Pythagorean theorem leads to the well-known prediction of special relativity: | |||
| The total time for the light pulse to trace its path is given by | |||
| The length of the half path can be calculated as a function of known quantities as | |||
| Substituting D from this equation into the previous, and solving for Δt' gives: | |||
| and thus, with the definition of Δt: | |||
| which expresses the fact that for the moving observer the period of the clock is longer than in the frame of the clock itself. | |||
| Time dilation due to relative velocity symmetric between observers | |||
| Common sense would dictate that if time passage has slowed for a moving object, the moving object would observe the external world to be correspondingly "sped up". Counterintuitively, special relativity predicts the opposite. | |||
| A similar oddity occurs in everyday life. If Sam sees Abigail at a distance she appears small to him and at the same time Sam appears small to Abigail. Experience has taught the human mind to accept the quirks of perspective but left it unprepared for relativity. | |||
| One is accustomed to the notion of relativity with respect to distance: the distance from Los Angeles to New York is by convention the same as the distance from New York to Los Angeles. On the other hand, when speeds are considered, one thinks of an object as "actually" moving, overlooking that its motion is always relative to something else — to the stars, the ground or to oneself. If one object is moving with respect to another, the latter is moving with respect to the former and with equal relative speed. | |||
| In the special theory of relativity, a moving clock is found to be ticking slowly with respect to the observer's clock. If Sam and Abigail are on different trains in near-lightspeed relative motion, Sam measures (by all methods of measurement) clocks on Abigail's train to be running slowly and, similarly, Abigail measures clocks on Sam's train to be running slowly. | |||
| Note that in all such attempts to establish "synchronization" within the reference system, the question of whether something happening at one location is in fact happening simultaneously with something happening elsewhere, is of key importance. Calculations are ultimately based on determining which events are simultaneous. Furthermore, establishing simultaneity of events separated in space necessarily requires transmission of information between locations, which by itself is an indication that the speed of light will enter the determination of simultaneity. | |||
| It is a natural and legitimate question to ask how, in detail, special relativity can be self-consistent if clock A is time-dilated with respect to clock B and clock B is also time-dilated with respect to clock A. It is by challenging the assumptions built into the common notion of simultaneity that logical consistency can be restored. Simultaneity is a relationship between an observer in a particular frame of reference and a set of events. By analogy, left and right are accepted to vary with the position of the observer, because they apply to a relationship. In a similar vein, Plato explained that up and down describe a relationship to the earth and one would not fall off at the antipodes. | |||
| Within the framework of the theory and its terminology there is a relativity of simultaneity that affects how the specified events are aligned with respect to each other by observers in relative motion. Because the pairs of putatively simultaneous moments are identified differently by different observers (as illustrated in the twin paradox article), each can treat the other clock as being the slow one without relativity being self-contradictory. This can be explained in many ways, some of which follow. | |||
| Temporal coordinate systems and clock synchronization | |||
| In Relativity, temporal coordinate systems are set up using a procedure for synchronizing clocks, discussed by Poincaré (1900) in relation to Lorentz's local time (see relativity of simultaneity). It is now usually called the Einstein synchronization procedure, since it appeared in his 1905 paper. | |||
| An observer with a clock sends a light signal out at time t1 according to his clock. At a distant event, that light signal is reflected back to, and arrives back to the observer at time t2 according to his clock. Since the light travels the same path at the same rate going both out and back for the observer in this scenario, the coordinate time of the event of the light signal being reflected for the observer tE is tE = (t1 + t2) / 2. In this way, a single observer's clock can be used to define temporal coordinates which are good anywhere in the universe. | |||
| Symmetric time dilation occurs with respect to temporal coordinate systems set up in this manner. It is an effect where another clock is being viewed as running slowly by an observer. Observers do not consider their own clock time to be time-dilated, but may find that it is observed to be time-dilated in another coordinate system. | |||
| Overview of time dilation formulae | |||
| Time dilation due to relative velocity | |||
| The formula for determining time dilation in special relativity is: | |||
| where | |||
| is the time interval between two co-local events (i.e. happening at the same place) for an observer in some inertial frame (e.g. ticks on his clock) – this is known as the proper time, | |||
| is the time interval between those same events, as measured by another observer, inertially moving with velocity v with respect to the former observer, | |||
| is the relative velocity between the observer and the moving clock, | |||
| is the speed of light, and | |||
| is the Lorentz factor. | |||
| Thus the duration of the clock cycle of a moving clock is found to be increased: it is measured to be "running slow". The range of such variances in ordinary life, where , even considering space travel, are not great enough to produce easily detectable time dilation effects, and such vanishingly small effects can be safely ignored. It is only when an object approaches speeds on the order of 30,000 km/s (1/10 the speed of light) that time dilation becomes important. | |||
| Time dilation by the Lorentz factor was predicted by Joseph Larmor (1897), at least for electrons orbiting a nucleus. Thus "... individual electrons describe corresponding parts of their orbits in times shorter for the system in the ratio :" (Larmor 1897). Time dilation of magnitude corresponding to this (Lorentz) factor has been experimentally confirmed, as described below. | |||
| Time dilation due to gravitation and motion together | |||
| Astronomical time scales and the GPS system represent significant practical applications, presenting problems that call for consideration of the combined effects of mass and motion in producing time dilation. | |||
| Relativistic time dilation effects, for the solar system and the Earth, have been evaluated from the starting point of an approximation to the Schwarzschild solution to the Einstein field equations. A timelike interval dtE in this metric can be approximated, when expressed in rectangular coordinates, and when truncated of higher powers in 1/c2, in the form:- | |||
| dtE2 = ( 1 - 2GMi/ric2 )dtc2 - ( dx2 + dy2 + dz2 )/c2 . . . . . (1), | |||
| in which: | |||
| dtE (expressed as a time-like interval) is a small increment forming part of an interval in the proper time tE (an interval that could be recorded on an atomic clock); | |||
| dtc is a small increment in the timelike coordinate tc ("coordinate time") of the clock's position in the chosen reference frame; | |||
| dx, dy and dz are small increments in three orthogonal space-like coordinates x, y, z of the clock's position in the chosen reference frame; and | |||
| GMi/ri represents a sum, to be designated U, of gravitational potentials due to the masses in the neighborhood, based on their distances ri from the clock. This sum of the GMi/ri is evaluated approximately, as a sum of Newtonian gravitational potentials (plus any tidal potentials considered), and is represented below as U (using the positive astronomical sign convention for gravitational potentials). The scope of the approximation may be extended to a case where U further includes effects of external masses other than the Mi, in the form of tidal gravitational potentials that prevail (due to the external masses) in a suitably small region of space around a point of the reference frame located somewhere in a gravity well due to those external masses, where the size of 'suitably small' remains to be investigated. | |||
| From this, after putting the velocity of the clock (in the coordinates of the chosen reference frame) as | |||
| v2 = (dx2 + dy2 + dz2)/dtc2 . . . . . (2) , | |||
| (then taking the square root, and truncating after binomial expansion, neglecting terms beyond the first power in 1/c2), a relation between the rate of the proper time and the rate of the coordinate time can be obtained as the differential equation | |||
| dtE/dtc = 1 - U/c2 - v2/2c2 . . . . . (3). | |||
| Equation (3) represents combined time dilations due to mass and motion, approximated to the first order in powers of 1/c2. The approximation can be applied to a number of the weak-field situations found around the Earth and in the solar-system. It can be thought of as relating the rate of proper time tE that can be measured by a clock, with the rate of a coordinate time tc. | |||
| In particular, for explanatory purposes, the time-dilation equation (3) provides a way of conceiving coordinate time, by showing that the rate of the clock would be exactly equal to the rate of the coordinate time if this "coordinate clock" could be situated | |||
| (a) hypothetically outside all relevant 'gravity wells', e.g. remote from all gravitational masses Mi, (so that U=0), and also | |||
| (b) at rest in relation to the chosen system of coordinates (so that v=0). | |||
| Equation (3) has been developed and integrated for the case where the reference frame is the solar system barycentric ('ssb') reference frame, to show the (time-varying) time dilation between the ssb coordinate time and local time at the Earth's surface: the main effects found included a mean time dilation of about 0.49 second per year (slower at the Earth's surface than for the ssb coordinate time), plus periodic modulation terms of which the largest has an annual period and an amplitude of about 1.66 millisecond. | |||
| Equation (3) has also been developed and integrated for the case of clocks at or near the Earth's surface. For clocks fixed to the rotating Earth's surface at mean sea level, regarded as a surface of the geoid, the sum ( U + v2/2 ) is a very nearly constant geopotential, and decreases with increasing height above sea level approximately as the product of the change in height and the gradient of the geopotential. This has been evaluated as a fractional increase in clock rate of about 1.1x10-13 per kilometer of height above sea level due to a decrease in combined rate of time dilation with increasing altitude. The value of dtE/dtc at height falls to be compared with the corresponding value at mean sea level. (Both values are slightly below 1, the value at height being a little larger (closer to 1) than the value at sea level.) | |||
| This gravitational time dilation relationship has been used in the synchronization or correlation of atomic clocks used to implement and maintain the atomic time scale TAI, where the different clocks are located at different heights above sea level, and since 1977 have had their frequencies steered to compensate for the differences of rate with height. | |||
| A fuller development of equation (3) for the near-Earth situation has been used to evaluate the combined time dilations relative to the Earth's surface experienced along the trajectories of satellites of the GPS global positioning system. The resulting values (in this case they are relativistic increases in the rate of the satellite-borne clocks, by about 38 microseconds per day) form the basis for adjustments essential for the functioning of the system. | |||
| Experimental confirmation | |||
| Time dilation has been tested a number of times. The routine work carried on in particle accelerators since the 1950s, such as those at CERN, is a continuously running test of the time dilation of special relativity. The specific experiments include: | |||
| Velocity time dilation tests | |||
| Ives and Stilwell (1938, 1941), “An experimental study of the rate of a moving clock”, in two parts. The stated purpose of these experiments was to verify the time dilation effect, predicted by Lamor-Lorentz ether theory, due to motion through the ether using Einstein's suggestion that Doppler effect in canal rays would provide a suitable experiment. These experiments measured the Doppler shift of the radiation emitted from cathode rays, when viewed from directly in front and from directly behind. The high and low frequencies detected were not the classical values predicted. | |||
| and = and | |||
| i.e. for sources with invariant frequencies The high and low frequencies of the radiation from the moving sources were measured as | |||
| and | |||
| as deduced by Einstein (1905) from the Lorentz transformation, when the source is running slow by the Lorentz factor. | |||
| Rossi and Hall (1941) compared the population of cosmic-ray-produced muons at the top of a mountain to that observed at sea level. Although the travel time for the muons from the top of the mountain to the base is several muon half-lives, the muon sample at the base was only moderately reduced. This is explained by the time dilation attributed to their high speed relative to the experimenters. That is to say, the muons were decaying about 10 times slower than if they were at rest with respect to the experimenters. | |||
| Hasselkamp, Mondry, and Scharmann (1979) measured the Doppler shift from a source moving at right angles to the line of sight (the transverse Doppler shift). The most general relationship between frequencies of the radiation from the moving sources is given by: | |||
| as deduced by Einstein (1905) . For () this reduces to fdetected = frestγ. Thus there is no transverse Doppler shift, and the lower frequency of the moving source can be attributed to the time dilation effect alone. | |||
| Gravitational time dilation tests | |||
| Pound, Rebka in 1959 measured the very slight gravitational red shift in the frequency of light emitted at a lower height, where Earth's gravitational field is relatively more intense. The results were within 10% of the predictions of general relativity. Later Pound and Snider (in 1964) derived an even closer result of 1%. This effect is as predicted by gravitational time dilation. | |||
| Velocity and gravitational time dilation combined-effect tests | |||
| Hafele and Keating, in 1971, flew caesium atomic clocks east and west around the Earth in commercial airliners, to compare the elapsed time against that of a clock that remained at the US Naval Observatory. Two opposite effects came into play. The clocks were expected to age more quickly (show a larger elapsed time) than the reference clock, since they were in a higher (weaker) gravitational potential for most of the trip (c.f. Pound, Rebka). But also, contrastingly, the moving clocks were expected to age more slowly because of the speed of their travel. The gravitational effect was the larger, and the clocks suffered a net gain in elapsed time. To within experimental error, the net gain was consistent with the difference between the predicted gravitational gain and the predicted velocity time loss. In 2005, the National Physical Laboratory in the United Kingdom reported their limited replication of this experiment. The NPL experiment differed from the original in that the caesium clocks were sent on a shorter trip (London–Washington D.C. return), but the clocks were more accurate. The reported results are within 4% of the predictions of relativity. | |||
| The Global Positioning System can be considered a continuously operating experiment in both special and general relativity. The in-orbit clocks are corrected for both special and general relativistic time dilation effects as described above, so that (as observed from the Earth's surface) they run at the same rate as clocks on the surface of the Earth. In addition, but not directly time dilation related, general relativistic correction terms are built into the model of motion that the satellites broadcast to receivers — uncorrected, these effects would result in an approximately 7-metre (23 ft) oscillation in the pseudo-ranges measured by a receiver over a cycle of 12 hours. | |||
| Muon lifetime | |||
| A comparison of muon lifetimes at different speeds is possible. In the laboratory, slow muons are produced, and in the atmosphere very fast moving muons are introduced by cosmic rays. Taking the muon lifetime at rest as the laboratory value of 2.22 μs, the lifetime of a cosmic ray produced muon traveling at 98% of the speed of light is about five times longer, in agreement with observations. In this experiment the "clock" is the time taken by processes leading to muon decay, and these processes take place in the moving muon at its own "clock rate", which is much slower than the laboratory clock. | |||
| Time dilation and space flight | |||
| Time dilation would make it possible for passengers in a fast-moving vehicle to travel further into the future while aging very little, in that their great speed slows down the rate of passage of on-board time. That is, the ship's clock (and according to relativity, any human travelling with it) shows less elapsed time than the clocks of observers on Earth. For sufficiently high speeds the effect is dramatic. For example, one year of travel might correspond to ten years at home. Indeed, a constant 1 g acceleration would permit humans to travel as far as light has been able to travel since the big bang (some 13.7 billion light years) in one human lifetime. The space travellers could return to Earth billions of years in the future. A scenario based on this idea was presented in the novel Planet of the Apes by Pierre Boulle. | |||
| A more likely use of this effect would be to enable humans to travel to nearby stars without spending their entire lives aboard the ship. However, any such application of time dilation during Interstellar travel would require the use of some new, advanced method of propulsion. | |||
| Current space flight technology has fundamental theoretical limits based on the practical problem that an increasing amount of energy is required for propulsion as a craft approaches the speed of light. The likelihood of collision with small space debris and other particulate material is another practical limitation. At the velocities presently attained, however, time dilation is not a factor in space travel. Travel to regions of space-time where gravitational time dilation is taking place, such as within the gravitational field of a black hole but outside the event horizon (perhaps on a hyperbolic trajectory exiting the field), could also yield results consistent with present theory. | |||
| Time dilation at constant acceleration | |||
| In special relativity, time dilation is most simply described in circumstances where relative velocity is unchanging. Nevertheless, the Lorentz equations allow one to calculate proper time and movement in space for the simple case of a spaceship whose acceleration, relative to some referent object in uniform (i.e. constant velocity) motion, equals g throughout the period of measurement. | |||
| Let t be the time in an inertial frame subsequently called the rest frame. Let x be a spatial coordinate, and let the direction of the constant acceleration as well as the spaceship's velocity (relative to the rest frame) be parallel to the x-axis. Assuming the spaceship's position at time t = 0 being x = 0 and the velocity being v0, the following formulas hold : | |||
| Position: | |||
| Velocity: | |||
| Proper time: | |||
| Time in the rest frame as a function of x: | |||
| The spacetime geometry of velocity time dilation | |||
| Time dilation in transverse motion.The green dots and red dots in the animation represent spaceships. The ships of the green fleet have no velocity relative to each other, so for the clocks onboard the individual ships the same amount of time elapses relative to each other, and they can set up a procedure to maintain a synchronized standard fleet time. The ships of the "red fleet" are moving with a velocity of 0.866 of the speed of light with respect to the green fleet. | |||
| The blue dots represent pulses of light. One cycle of light-pulses between two green ships takes two seconds of "green time", one second for each leg. | |||
| As seen from the perspective of the reds, the transit time of the light pulses they exchange among each other is one second of "red time" for each leg. As seen from the perspective of the greens, the red ships' cycle of exchanging light pulses travels a diagonal path that is two light-seconds long. (As seen from the green perspective the reds travel 1.73 () light-seconds of distance for every two seconds of green time.) | |||
| One of the red ships emits a light pulse towards the greens every second of red time. These pulses are received by ships of the green fleet with two-second intervals as measured in green time. Not shown in the animation is that all aspects of physics are proportionally involved. The lightpulses that are emitted by the reds at a particular frequency as measured in red time are received at a lower frequency as measured by the detectors of the green fleet that measure against green time, and vice versa. | |||
| The animation cycles between the green perspective and the red perspective, to emphasize the symmetry. As there is no such thing as absolute motion in relativity (as is also the case for Newtonian mechanics), both the green and the red fleet are entitled to consider themselves motionless in their own frame of reference. | |||
| Again, it is vital to understand that the results of these interactions and calculations reflect the real state of the ships as it emerges from their situation of relative motion. It is not a mere quirk of the method of measurement or communication. | |||
| See also | |||
| Four-vector | |||
| General relativity | |||
| Hafele-Keating experiment | |||
| Ives-Stilwell experiment | |||
| Trouton-Rankine experiment | |||
| Length contraction and Lorentz-Fitzgerald contraction | |||
| Lorentz transformation | |||
| Minkowski space | |||
| Pound-Rebka experiment | |||
| Relativistic Doppler effect and Transverse Doppler effect | |||
| Relativity of simultaneity | |||
| Special relativity | |||
| ......) | |||
| Infinity (∞) | |||
| Einstein (''The Meaning of Relativity''): "Two ]s taking place at the points A and B of a system K are simultaneous if they appear at the same instant when observed from the middle point, M, of the interval AB. Time is then defined as the ensemble of the indications of similar clocks, at rest relatively to K, which register the same simultaneously." | Einstein (''The Meaning of Relativity''): "Two ]s taking place at the points A and B of a system K are simultaneous if they appear at the same instant when observed from the middle point, M, of the interval AB. Time is then defined as the ensemble of the indications of similar clocks, at rest relatively to K, which register the same simultaneously." | ||
Revision as of 09:51, 22 September 2009
For other uses, see Time (disambiguation).
Time is a component of the measuring system used to sequence events, to compare the durations of events and the intervals between them, and to quantify the motions of objects. Time has been a major subject of religion, philosophy, and science, but defining it in a non-controversial manner applicable to all fields of study has consistently eluded the greatest scholars.
In physics as well as in other sciences, time is considered one of the few fundamental quantities. Time is used to define other quantities – such as velocity – so defining time in terms of such quantities would result in circularity of definition. An operational definition of time, wherein one says that observing a certain number of repetitions of one or another standard cyclical event (such as the passage of a free-swinging pendulum) constitutes one standard unit such as the second, is highly useful in the conduct of both advanced experiments and everyday affairs of life. The operational definition leaves aside the question whether there is something called time, apart from the counting activity just mentioned, that flows and that can be measured. Investigations of a single continuum called spacetime brings the nature of time into association with related questions into the nature of space, questions that have their roots in the works of early students of natural philosophy.
Among prominent philosophers, there are two distinct viewpoints on time. One view is that time is part of the fundamental structure of the universe, a dimension in which events occur in sequence. Time travel, in this view, becomes a possibility as other "times" persist like frames of a film strip, spread out across the time line. Sir Isaac Newton subscribed to this realist view, and hence it is sometimes referred to as Newtonian time. The opposing view is that time does not refer to any kind of "container" that events and objects "move through", nor to any entity that "flows", but that it is instead part of a fundamental intellectual structure (together with space and number) within which humans sequence and compare events. This second view, in the tradition of Gottfried Leibniz and Immanuel Kant, holds that time is neither an event nor a thing, and thus is not itself measurable nor can it be travelled.
Temporal measurement has occupied scientists and technologists, and was a prime motivation in navigation and astronomy. Periodic events and periodic motion have long served as standards for units of time. Examples include the apparent motion of the sun across the sky, the phases of the moon, the swing of a pendulum, and the beat of a heart. Currently, the international unit of time, the second, is defined in terms of radiation emitted by caesium atoms (see below). Time is also of significant social importance, having economic value ("time is money") as well as personal value, due to an awareness of the limited time in each day and in human life spans.
Temporal measurement
Temporal measurement, or chronometry, takes two distinct period forms: the calendar, a mathematical abstraction for calculating extensive periods of time, and the clock, a concrete mechanism that counts the ongoing passage of time. In day-to-day life, the clock is consulted for periods less than a day, the calendar, for periods longer than a day. Increasingly, personal electronic devices display both calendars and clocks simultaneously. The number (as on a clock dial or calendar) that marks the occurrence of a specified event as to hour or date is obtained by counting from a fiducial epoch—a central reference point.
History of the calendar
Main article: CalendarArtifacts from the Palaeolithic suggest that the moon was used to calculate time as early as 12,000, and possibly even 30,000 BP. Lunar calendars were among the first to appear, with all years having twelve lunar months (approximately 354 days). Without intercalation to add days or months to some years, seasons quickly drift in a calendar based solely on twelve lunar months. Lunisolar calendars have a thirteenth month added to some years to make up for the difference between a full year (now known to be about 365.24 days) and a year of just twelve lunar months. The numbers twelve and thirteen came to feature prominently in many cultures, at least partly due to this relationship of months to years.
The reforms of Julius Caesar in 45 BC put the Roman world on a solar calendar. This Julian calendar was faulty in that its intercalation still allowed the astronomical solstices and equinoxes to advance against it by about 11 minutes per year. Pope Gregory XIII introduced a correction in 1582; the Gregorian calendar was only slowly adopted by different nations over a period of centuries, but is today by far the one in most common use around the world.
History of time measurement devices

A large variety of devices have been invented to measure time. The study of these devices is called horology.
An Egyptian device dating to c.1500 BC, similar in shape to a bent T-square, measured the passage of time from the shadow cast by its crossbar on a non-linear rule. The T was oriented eastward in the mornings. At noon, the device was turned around so that it could cast its shadow in the evening direction.
A sundial uses a gnomon to cast a shadow on a set of markings which were calibrated to the hour. The position of the shadow marked the hour in local time.
The most precise timekeeping devices of the ancient world were the water clock or clepsydra, one of which was found in the tomb of Egyptian pharaoh Amenhotep I (1525–1504 BC). They could be used to measure the hours even at night, but required manual timekeeping to replenish the flow of water. The Greeks and Chaldeans regularly maintained timekeeping records as an essential part of their astronomical observations. Arab inventors and engineers in particular made improvements on the use of water clocks up to the Middle Ages. In the 11th century, the Chinese inventors and engineers invented the first mechanical clocks to be driven by an escapement mechanism.

The hourglass uses the flow of sand to measure the flow of time. They were used in navigation. Ferdinand Magellan used 18 glasses on each ship for his circumnavigation of the globe (1522). Incense sticks and candles were, and are, commonly used to measure time in temples and churches across the globe. Waterclocks, and later, mechanical clocks, were used to mark the events of the abbeys and monasteries of the Middle Ages. Richard of Wallingford (1292–1336), abbot of St. Alban's abbey, famously built a mechanical clock as an astronomical orrery about 1330. Great advances in accurate time-keeping were made by Galileo Galilei and especially Christiaan Huygens with the invention of pendulum driven clocks.
The English word clock probably comes from the Middle Dutch word "klocke" which is in turn derived from the mediaeval Latin word "clocca", which is ultimately derived from Celtic, and is cognate with French, Latin, and German words that mean bell. The passage of the hours at sea were marked by bells, and denoted the time (see ship's bells). The hours were marked by bells in the abbeys as well as at sea.

Clocks can range from watches, to more exotic varieties such as the Clock of the Long Now. They can be driven by a [variety of means, including gravity, springs, and various forms of electrical power, and regulated by a variety of means such as a pendulum.
A chronometer is a portable timekeeper that meets certain precision standards. Initially, the term was used to refer to the marine chronometer, a timepiece used to determine longitude by means of celestial navigation, a precision firstly achieved by John Harrison. More recently, the term has also been applied to the chronometer watch, a wristwatch that meets precision standards set by the Swiss agency COSC.
The most accurate timekeeping devices are atomic clocks, which are accurate to seconds in many millions of years, and are used to calibrate other clocks and timekeeping instruments. Atomic clocks use the spin property of atoms as their basis, and since 1967, the International System of Measurements bases its unit of time, the second, on the properties of caesium atoms. SI defines the second as 9,192,631,770 cycles of that radiation which corresponds to the transition between two electron spin energy levels of the ground state of the Cs atom.
Today, the Global Positioning System in coordination with the Network Time Protocol can be used to synchronize timekeeping systems across the globe.
In medieval philosophical writings, the atom was a unit of time referred to as the smallest possible division of time. The earliest known occurrence in English is in Byrhtferth's Enchiridion (a science text) of 1010–1012, where it was defined as 1/564 of a momentum (1½ minutes), and thus equal to 15/94 of a second. It was used in the computus, the process of calculating the date of Easter.
As of 2006, the smallest unit of time that has been directly measured is on the attosecond (10 s) time scale, or around 10 Planck times.
Definitions and standards
| Unit | Size | Notes |
|---|---|---|
| attosecond | 1/10 s | smallest measured time |
| femtosecond | 1/10 s | |
| picosecond | 1/10 s | |
| nanosecond | 1/10 s | |
| microsecond | 1/10 s | |
| millisecond | 0.001 s | |
| second | SI base unit | |
| minute | 60 s | |
| hour | 60 minutes | |
| day | 24 hours | |
| week | 7 days | Also called sennight |
| fortnight | 14 days | 2 weeks |
| lunar month | 27.2–29.5 days | Various definitions of lunar month exist. |
| month | 28–31 days | |
| quarter | 3 months | |
| year | 12 months | |
| common year | 365 days | 52 weeks + 1 day |
| leap year | 366 days | 52 weeks + 2 days |
| tropical year | 365.24219 days | average |
| Gregorian year | 365.2425 days | average |
| Olympiad | 4 year cycle | |
| lustrum | 5 years | Also called pentad |
| decade | 10 years | |
| Indiction | 15 year cycle | |
| generation | 17–25 years | approximate |
| jubilee (Biblical) | 50 years | |
| century | 100 years | |
| millennium | 1,000 years |
The SI base unit for time is the SI second. From the second, larger units such as the minute, hour and day are defined, though they are "non-SI" units because they do not use the decimal system, and also because of the occasional need for a leap second. They are, however, officially accepted for use with the International System. There are no fixed ratios between seconds and months or years as months and years have significant variations in length.
The official SI definition of the second is as follows:
The second is the duration of 9,192,631,770 periods of the radiation corresponding to the transition between the two hyperfine levels of the ground state of the caesium 133 atom.
At its 1997 meeting, the CIPM affirmed that this definition refers to a caesium atom in its ground state at a temperature of 0 K. Previous to 1967, the second was defined as:
the fraction 1/31,556,925.9747 of the tropical year for 1900 January 0 at 12 hours ephemeris time.
The current definition of the second, coupled with the current definition of the metre, is based on the special theory of relativity, which affirms our space-time to be a Minkowski space.
World time
Time keeping is so critical to the functioning of modern societies that it is coordinated at an international level. The basis for scientific time is a continuous count of seconds based on atomic clocks around the world, known as the International Atomic Time (TAI). Other scientific time standards include Terrestrial Time and Barycentric Dynamical Time.
Coordinated Universal Time (UTC) is the basis for modern civil time. Since January 1, 1972, it has been defined to follow TAI with an exact offset of an integer number of seconds, changing only when a leap second is added to keep clock time synchronized with the rotation of the Earth. In TAI and UTC systems, the duration of a second is constant, as it is defined by the unchanging transition period of the cesium atom.
Greenwich Mean Time (GMT) is an older standard, adopted starting with British railroads in 1847. Using telescopes instead of atomic clocks, GMT was calibrated to the mean solar time at the Royal Observatory, Greenwich in the UK. Universal Time (UT) is the modern term for the international telescope-based system, adopted to replace "Greenwich Mean Time" in 1928 by the International Astronomical Union. Observations at the Greenwich Observatory itself ceased in 1954, though the location is still used as the basis for the coordinate system. Because the rotational period of Earth is not perfectly constant, the duration of a second would vary if calibrated to a telescope-based standard like GMT or UT - in which a second was defined as a fraction of a day or year. The terms "GMT" and "Greenwich Mean Time" are sometimes used informally to refer to UT or UTC.
The Global Positioning System also broadcasts a very precise time signal worldwide, along with instructions for converting GPS time to UTC.
Earth is split up into a number of time zones. Most time zones are exactly one hour apart, and by convention compute their local time as an offset from UTC or GMT. In many locations these offsets vary twice yearly due to daylight saving time transitions.
Sidereal time
Sidereal time is the measurement of time relative to a distant star (instead of solar time that is relative to the sun). It is used in astronomy to predict when a star will be overhead. Due to the rotation of the earth around the sun a sidereal day is 1/366th of a day (4 minutes) less than a solar day.
Chronology
Main article: ChronologyAnother form of time measurement consists of studying the past. Events in the past can be ordered in a sequence (creating a chronology), and can be put into chronological groups (periodization). One of the most important systems of periodization is geologic time, which is a system of periodizing the events that shaped the Earth and its life. Chronology, periodization, and interpretation of the past are together known as the study of history.
Religion
Further information: Category:Time and fate deitiesIn the Old Testament book Ecclesiastes, traditionally ascribed to Solomon (970–928 BC), time (as the Hebrew word עדן, זמן `iddan(time) zĕman(season) is often translated) was traditionally regarded as a medium for the passage of predestined events. (Another word, זמן zman, was current as meaning time fit for an event, and is used as the modern Hebrew equivalent to the English word "time".)

There is an appointed time (zman) for everything. And there is a time (’êth) for every event under heaven–
A time (’êth) to give birth, and a time to die; A time to plant, and a time to uproot what is planted.
A time to kill, and a time to heal; A time to tear down, and a time to build up.
A time to weep, and a time to laugh; A time to mourn, and a time to dance.
A time to throw stones, and a time to gather stones; A time to embrace, and a time to shun embracing.
A time to search, and a time to give up as lost; A time to keep, and a time to throw away.
A time to tear apart, and a time to sew together; A time to be silent, and a time to speak.
A time to love, and a time to hate; A time for war, and a time for peace.
Linear and cyclical time
See also: Time Cycles and Wheel of timeIn general, the Judaeo-Christian concept, based on the Bible, is that time is linear, with a beginning, the act of creation by God. The Christian view assumes also an end, the eschaton, expected to happen when Jesus returns to earth in the Second Coming to judge the living and the dead. This will be the consummation of the world and time. St Augustine's City of God was the first developed application of this concept to world history. The Christian view is that God is uncreated and eternal so that He and the supernatural world are outside time and exist in eternity.
Ancient cultures such as Incan, Mayan, Hopi, and other Native American Tribes, plus the Babylonian, Ancient Greek, Hindu, Buddhist, Jainist, and others have a concept of a wheel of time, that regards time as cyclical and quantic consisting of repeating ages that happen to every being of the Universe between birth and extinction.
Numeric and Divine time
The Greek language denotes two distinct principles, Chronos and Kairos. The former refers to numeric, or chronological, time. The latter, literally "the right or opportune moment," relates specifically to metaphysical or Divine time. In theology, Kairos is qualitative, as opposed to quantitative.
Philosophy
Main articles: Philosophy of space and time and Temporal finitismThe Vedas, the earliest texts on Indian philosophy and Hindu philosophy dating back to the late 2nd millennium BC, describe ancient Hindu cosmology, in which the universe goes through repeated cycles of creation, destruction and rebirth, with each cycle lasting 4,320,000 years. Ancient Greek philosophers, including Parmenides and Heraclitus, wrote essays on the nature of time.
In Book 11 of St. Augustine's Confessions, he ruminates on the nature of time, asking, "What then is time? If no one asks me, I know: if I wish to explain it to one that asketh, I know not." He settles on time being defined more by what it is not than what it is, an approach similar to that taken in other negative definitions.
In contrast to ancient Greek philosophers who believed that the universe had an infinite past with no beginning, medieval philosophers and theologians developed the concept of the universe having a finite past with a beginning. This view is not shared by Abrahamic faiths as they believe time started by creation, therefore the only thing being infinite is God and everything else, including time, is finite.
Newton's belief in absolute space, and a precursor to Kantian time, Leibniz believed that time and space are relational. The differences between Leibniz's and Newton's interpretations came to a head in the famous Leibniz-Clarke Correspondence.
Immanuel Kant, in the Critique of Pure Reason, described time as an a priori intuition that allows us (together with the other a priori intuition, space) to comprehend sense experience. With Kant, neither space nor time are conceived as substances, but rather both are elements of a systematic mental framework that necessarily structures the experiences of any rational agent, or observing subject. Kant thought of time as a fundamental part of an abstract conceptual framework, together with space and number, within which we sequence events, quantify their duration, and compare the motions of objects. In this view, time does not refer to any kind of entity that "flows," that objects "move through," or that is a "container" for events. Spatial measurements are used to quantify the extent of and distances between objects, and temporal measurements are used to quantify the durations of and between events. (See Ontology).
Henri Bergson believed that time was neither a real homogeneous medium nor a mental construct, but possesses what he referred to as Duration. Duration, in Bergson's view, was creativity and memory as an essential component of reality.
Time as "unreal"
In 5th century BC Greece, Antiphon the Sophist, in a fragment preserved from his chief work On Truth held that: "Time is not a reality (hypostasis), but a concept (noêma) or a measure (metron)." Parmenides went further, maintaining that time, motion, and change were illusions, leading to the paradoxes of his follower Zeno. Time as illusion is also a common theme in Buddhist thought, and some modern philosophers have carried on with this theme. J. M. E. McTaggart's 1908 The Unreality of Time, for example, argues that time is unreal (see also The flow of time).
However, these arguments often center around what it means for something to be "real". Modern physicists generally consider time to be as "real" as space, though others such as Julian Barbour in his The End of Time argue that quantum equations of the universe take their true form when expressed in the timeless configuration spacerealm containing every possible "Now" or momentary configuration of the universe, which he terms 'platonia'. (See also: Eternalism (philosophy of time).)
Physical definition
Main article: Time in physicsFrom the age of Newton up until Einstein's profound reinterpretation of the physical concepts associated with time and space, time was considered to be "absolute" and to flow "equably" (to use the words of Newton) for all observers. The science of classical mechanics is based on this Newtonian idea of time.
Einstein, in his special theory of relativity, postulated the constancy and finiteness of the speed of light for all observers. He showed that this postulate, together with a reasonable definition for what it means for two events to be simultaneous, requires that distances appear compressed and time intervals appear lengthened for events associated with objects in motion relative to an inertial observer.
Einstein showed that if time and space is measured using electromagnetic phenomena (like light bouncing between mirrors) then due to the constancy of the speed of light, time and space become mathematically entangled together in a certain way (called Minkowski space) which in turn results in Lorentz transformation and in entanglement of all other important derivative physical quantities (like energy, momentum, mass, force, etc) in a certain 4-vectorial way (see special relativity for more details).
| Part of a series on |
| Classical mechanics |
|---|
| Second law of motion |
| Branches |
| Fundamentals |
| Formulations |
| Core topics |
| Rotation |
| Scientists |
Classical mechanics
In classical mechanics, Newton's concept of "relative, apparent, and common time" can be used in the formulation of a prescription for the synchronization of clocks. Events seen by two different observers in motion relative to each other produce a mathematical concept of time that works pretty well for describing the everyday phenomena of most people's experience.
Modern physics
In the late nineteenth century, physicists encountered problems with the classical understanding of time, in connection with the behavior of electricity and magnetism. Einstein resolved these problems by invoking a method of synchronizing clocks using the constant, finite speed of light as the maximum signal velocity. This led directly to the result that observers in motion relative to one another will measure different elapsed times for the same event.

Spacetime
Main article: SpacetimeTime has historically been closely related with space, the two together comprising spacetime in Einstein's special relativity and general relativity. According to these theories, the concept of time depends on the spatial reference frame of the observer, and the human perception as well as the measurement by instruments such as clocks are different for observers in relative motion. The past is the set of events that can send light signals to the observer, the future is the set of events to which the observer can send light signals.
Time dilation
"Time is nature's way of keeping everything from happening at once". This quote, attributed variously to Einstein, John Archibald Wheeler, and Woody Allen, says that time is what separates cause and effect. Einstein showed that people travelling at different speeds, while agreeing on cause and effect, will measure different time separations between events and can even observe different chronological orderings between non-causally related events. Though these effects are typically minute in the human experience, the effect becomes much more pronounced for objects moving at speeds approaching the speed of light. Many subatomic particles exist for only a fixed fraction of a second in a lab relatively at rest, but some that travel close to the speed of light can be measured to travel further and survive much longer than expected (a muon is one example). According to the special theory of relativity, in the high-speed particle's frame of reference, it exists, on the average, for a standard amount of time known as its mean lifetime, and the distance it travels in that time is zero, because its velocity is zero. Relative to a frame of reference at rest, time seems to "slow down" for the particle. Relative to the high-speed particle, distances seem to shorten. Even in Newtonian terms time may be considered the fourth dimension of motion; but Einstein showed how both temporal and spatial dimensions can be altered (or "warped") by high-speed motion.
(Time dilation--
Time dilation Jump to:navigation, search
This article needs additional citations for verification.
Please help improve this article by adding reliable references. Unsourced material may be challenged and removed. (May 2008) Contents 1 Overview of time dilation 2 Simple inference of time dilation due to relative velocity 3 Time dilation due to relative velocity symmetric between observers 3.1 Temporal coordinate systems and clock synchronization 4 Overview of time dilation formulae 4.1 Time dilation due to relative velocity 4.2 Time dilation due to gravitation and motion together 5 Experimental confirmation 5.1 Velocity time dilation tests 5.2 Gravitational time dilation tests 5.3 Velocity and gravitational time dilation combined-effect tests 5.4 Muon lifetime 6 Time dilation and space flight 6.1 Time dilation at constant acceleration 6.2 The spacetime geometry of velocity time dilation 7 See also 8 References 9 External links 9.1 tags
This article discusses a concept in physics. For the concept in sociology, see time displacement.
Time dilation is a phenomenon (or two phenomena, as mentioned below) described by the theory of relativity. It can be illustrated by supposing that two observers are in motion relative to each other, and/or differently situated with regard to nearby gravitational masses. They each carry a clock of identically similar construction and function. Then, the point of view of each observer will generally be, that the other observer's clock is in error (has changed its rate).
There are two kinds of time dilation, and both can operate together.
Overview of time dilation Time dilation can arise from (1) relative velocity of motion between the observers, and (2) difference in their distance from gravitational mass.
(1) In the case that the observers are in relative uniform motion, and far away from any gravitational mass, the point of view of each will be that the other's (moving) clock is ticking at a slower rate than the local clock. The faster the relative velocity, the more is the rate of time dilation. This case is sometimes called special relativistic time dilation. It is often interpreted as time "slowing down" for the other (moving) clock. But that is only true from the physical point of view of the local observer, and of others at relative rest (i.e. in the local observer's frame of reference). The point of view of the other observer will be that again the local clock (this time the other clock) is correct, and it is the distant moving one that is slow. From a local perspective, time registered by clocks that are at rest with respect to the local frame of reference (and far from any gravitational mass) always appears to pass at the same rate.
(2) There is another case of time dilation, where both observers are differently situated in their distance from a significant gravitational mass, such as (for terrestrial observers) the Earth or the Sun. One may suppose for simplicity that the observers are at relative rest (which is not the case of two observers both rotating with the Earth -- an extra factor described below). In the simplified case, the general theory of relativity describes how, for both observers, the clock that is closer to the gravitational mass, i.e. deeper in its "gravity well", appears to go slower than the clock that is more distant from the mass (or higher in altitude away from the center of the gravitational mass). That does not mean that the two observers fully agree: each still makes the local clock to be correct; the observer more distant from the mass (higher in altitude) makes the other clock (closer to the mass, lower in altitude) to be slower than the local correct rate, and the observer situated closer to the mass (lower in altitude) makes the other clock (farther from the mass, higher in altitude) to be faster than the local correct rate. They agree at least that the clock nearer the mass is slower in rate, and on the ratio of the difference. This is gravitational time dilation.
In Albert Einstein's theories of relativity, time dilation in these two circumstances can be summarized:
In special relativity (or, hypothetically far from all gravitational mass), clocks that are moving with respect to an inertial system of observation are measured to be running slower. This effect is described precisely by the Lorentz transformation. In general relativity, clocks at lower potentials in a gravitational field — such as in closer proximity to a planet — are found to be running slower. The articles gravitational time dilation and gravitational red shift give a more detailed discussion. Special and general relatistic effects can combine, for example in some time-scale applications mentioned below. Thus, in special relativity, the time dilation effect is reciprocal: as observed from the point of view of either of two clocks which are in motion with respect to each other, it will be the other clock that is time dilated. (This presumes that the relative motion of both parties is uniform; that is, they do not accelerate with respect to one another during the course of the observations.)
In contrast, gravitational time dilation (as treated in general relativity) is not reciprocal: an observer at the top of a tower will observe that clocks at ground level tick slower, and observers on the ground will agree about that, i.e. about the direction and the ratio of the difference. There is not full agreement, all the observers make their own local clocks out to be correct, but the direction and ratio of gravitational time dilation is agreed by all observers, independent of their altitude.
Simple inference of time dilation due to relative velocity
Observer at rest sees time 2L/c Observer moving parallel relative to setup, sees longer path, time > 2L/c, same speed cTime dilation can be inferred from the observed fact of the constancy of the speed of light in all reference frames.
This constancy of the speed of light means, counter to intuition, that speeds of material objects and light are not additive. It is not possible to make the speed of light appear faster by approaching at speed towards the material source that is emitting light. It is not possible to make the speed of light appear slower by receding from the source at speed. From one point of view, it is the implications of this unexpected constancy that take away from constancies expected elsewhere.
Consider a simple clock consisting of two mirrors A and B, between which a light pulse is bouncing. The separation of the mirrors is L, and the clock ticks once each time it hits a given mirror.
In the frame where the clock is at rest (diagram at right), the light pulse traces out a path of length 2L and the period of the clock is 2L divided by the speed of light:
From the frame of reference of a moving observer traveling at the speed v (diagram at lower right), the light pulse traces out a longer, angled path. The second postulate of special relativity states that the speed of light is constant in all frames, which implies a lengthening of the period of this clock from the moving observer's perspective. That is to say, in a frame moving relative to the clock, the clock appears to be running more slowly. Straightforward application of the Pythagorean theorem leads to the well-known prediction of special relativity:
The total time for the light pulse to trace its path is given by
The length of the half path can be calculated as a function of known quantities as
Substituting D from this equation into the previous, and solving for Δt' gives:
and thus, with the definition of Δt:
which expresses the fact that for the moving observer the period of the clock is longer than in the frame of the clock itself.
Time dilation due to relative velocity symmetric between observers Common sense would dictate that if time passage has slowed for a moving object, the moving object would observe the external world to be correspondingly "sped up". Counterintuitively, special relativity predicts the opposite.
A similar oddity occurs in everyday life. If Sam sees Abigail at a distance she appears small to him and at the same time Sam appears small to Abigail. Experience has taught the human mind to accept the quirks of perspective but left it unprepared for relativity.
One is accustomed to the notion of relativity with respect to distance: the distance from Los Angeles to New York is by convention the same as the distance from New York to Los Angeles. On the other hand, when speeds are considered, one thinks of an object as "actually" moving, overlooking that its motion is always relative to something else — to the stars, the ground or to oneself. If one object is moving with respect to another, the latter is moving with respect to the former and with equal relative speed.
In the special theory of relativity, a moving clock is found to be ticking slowly with respect to the observer's clock. If Sam and Abigail are on different trains in near-lightspeed relative motion, Sam measures (by all methods of measurement) clocks on Abigail's train to be running slowly and, similarly, Abigail measures clocks on Sam's train to be running slowly.
Note that in all such attempts to establish "synchronization" within the reference system, the question of whether something happening at one location is in fact happening simultaneously with something happening elsewhere, is of key importance. Calculations are ultimately based on determining which events are simultaneous. Furthermore, establishing simultaneity of events separated in space necessarily requires transmission of information between locations, which by itself is an indication that the speed of light will enter the determination of simultaneity.
It is a natural and legitimate question to ask how, in detail, special relativity can be self-consistent if clock A is time-dilated with respect to clock B and clock B is also time-dilated with respect to clock A. It is by challenging the assumptions built into the common notion of simultaneity that logical consistency can be restored. Simultaneity is a relationship between an observer in a particular frame of reference and a set of events. By analogy, left and right are accepted to vary with the position of the observer, because they apply to a relationship. In a similar vein, Plato explained that up and down describe a relationship to the earth and one would not fall off at the antipodes.
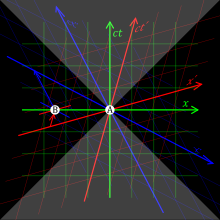
Within the framework of the theory and its terminology there is a relativity of simultaneity that affects how the specified events are aligned with respect to each other by observers in relative motion. Because the pairs of putatively simultaneous moments are identified differently by different observers (as illustrated in the twin paradox article), each can treat the other clock as being the slow one without relativity being self-contradictory. This can be explained in many ways, some of which follow.
Temporal coordinate systems and clock synchronization In Relativity, temporal coordinate systems are set up using a procedure for synchronizing clocks, discussed by Poincaré (1900) in relation to Lorentz's local time (see relativity of simultaneity). It is now usually called the Einstein synchronization procedure, since it appeared in his 1905 paper.
An observer with a clock sends a light signal out at time t1 according to his clock. At a distant event, that light signal is reflected back to, and arrives back to the observer at time t2 according to his clock. Since the light travels the same path at the same rate going both out and back for the observer in this scenario, the coordinate time of the event of the light signal being reflected for the observer tE is tE = (t1 + t2) / 2. In this way, a single observer's clock can be used to define temporal coordinates which are good anywhere in the universe.
Symmetric time dilation occurs with respect to temporal coordinate systems set up in this manner. It is an effect where another clock is being viewed as running slowly by an observer. Observers do not consider their own clock time to be time-dilated, but may find that it is observed to be time-dilated in another coordinate system.
Overview of time dilation formulae
Time dilation due to relative velocity
The formula for determining time dilation in special relativity is:
where
is the time interval between two co-local events (i.e. happening at the same place) for an observer in some inertial frame (e.g. ticks on his clock) – this is known as the proper time,
is the time interval between those same events, as measured by another observer, inertially moving with velocity v with respect to the former observer,
is the relative velocity between the observer and the moving clock, is the speed of light, and is the Lorentz factor.
Thus the duration of the clock cycle of a moving clock is found to be increased: it is measured to be "running slow". The range of such variances in ordinary life, where , even considering space travel, are not great enough to produce easily detectable time dilation effects, and such vanishingly small effects can be safely ignored. It is only when an object approaches speeds on the order of 30,000 km/s (1/10 the speed of light) that time dilation becomes important.
Time dilation by the Lorentz factor was predicted by Joseph Larmor (1897), at least for electrons orbiting a nucleus. Thus "... individual electrons describe corresponding parts of their orbits in times shorter for the system in the ratio :" (Larmor 1897). Time dilation of magnitude corresponding to this (Lorentz) factor has been experimentally confirmed, as described below.
Time dilation due to gravitation and motion together Astronomical time scales and the GPS system represent significant practical applications, presenting problems that call for consideration of the combined effects of mass and motion in producing time dilation.
Relativistic time dilation effects, for the solar system and the Earth, have been evaluated from the starting point of an approximation to the Schwarzschild solution to the Einstein field equations. A timelike interval dtE in this metric can be approximated, when expressed in rectangular coordinates, and when truncated of higher powers in 1/c2, in the form:-
dtE2 = ( 1 - 2GMi/ric2 )dtc2 - ( dx2 + dy2 + dz2 )/c2 . . . . . (1), in which:
dtE (expressed as a time-like interval) is a small increment forming part of an interval in the proper time tE (an interval that could be recorded on an atomic clock); dtc is a small increment in the timelike coordinate tc ("coordinate time") of the clock's position in the chosen reference frame; dx, dy and dz are small increments in three orthogonal space-like coordinates x, y, z of the clock's position in the chosen reference frame; and GMi/ri represents a sum, to be designated U, of gravitational potentials due to the masses in the neighborhood, based on their distances ri from the clock. This sum of the GMi/ri is evaluated approximately, as a sum of Newtonian gravitational potentials (plus any tidal potentials considered), and is represented below as U (using the positive astronomical sign convention for gravitational potentials). The scope of the approximation may be extended to a case where U further includes effects of external masses other than the Mi, in the form of tidal gravitational potentials that prevail (due to the external masses) in a suitably small region of space around a point of the reference frame located somewhere in a gravity well due to those external masses, where the size of 'suitably small' remains to be investigated. From this, after putting the velocity of the clock (in the coordinates of the chosen reference frame) as
v2 = (dx2 + dy2 + dz2)/dtc2 . . . . . (2) , (then taking the square root, and truncating after binomial expansion, neglecting terms beyond the first power in 1/c2), a relation between the rate of the proper time and the rate of the coordinate time can be obtained as the differential equation
dtE/dtc = 1 - U/c2 - v2/2c2 . . . . . (3). Equation (3) represents combined time dilations due to mass and motion, approximated to the first order in powers of 1/c2. The approximation can be applied to a number of the weak-field situations found around the Earth and in the solar-system. It can be thought of as relating the rate of proper time tE that can be measured by a clock, with the rate of a coordinate time tc.
In particular, for explanatory purposes, the time-dilation equation (3) provides a way of conceiving coordinate time, by showing that the rate of the clock would be exactly equal to the rate of the coordinate time if this "coordinate clock" could be situated
(a) hypothetically outside all relevant 'gravity wells', e.g. remote from all gravitational masses Mi, (so that U=0), and also (b) at rest in relation to the chosen system of coordinates (so that v=0). Equation (3) has been developed and integrated for the case where the reference frame is the solar system barycentric ('ssb') reference frame, to show the (time-varying) time dilation between the ssb coordinate time and local time at the Earth's surface: the main effects found included a mean time dilation of about 0.49 second per year (slower at the Earth's surface than for the ssb coordinate time), plus periodic modulation terms of which the largest has an annual period and an amplitude of about 1.66 millisecond.
Equation (3) has also been developed and integrated for the case of clocks at or near the Earth's surface. For clocks fixed to the rotating Earth's surface at mean sea level, regarded as a surface of the geoid, the sum ( U + v2/2 ) is a very nearly constant geopotential, and decreases with increasing height above sea level approximately as the product of the change in height and the gradient of the geopotential. This has been evaluated as a fractional increase in clock rate of about 1.1x10-13 per kilometer of height above sea level due to a decrease in combined rate of time dilation with increasing altitude. The value of dtE/dtc at height falls to be compared with the corresponding value at mean sea level. (Both values are slightly below 1, the value at height being a little larger (closer to 1) than the value at sea level.)
This gravitational time dilation relationship has been used in the synchronization or correlation of atomic clocks used to implement and maintain the atomic time scale TAI, where the different clocks are located at different heights above sea level, and since 1977 have had their frequencies steered to compensate for the differences of rate with height.
A fuller development of equation (3) for the near-Earth situation has been used to evaluate the combined time dilations relative to the Earth's surface experienced along the trajectories of satellites of the GPS global positioning system. The resulting values (in this case they are relativistic increases in the rate of the satellite-borne clocks, by about 38 microseconds per day) form the basis for adjustments essential for the functioning of the system.
Experimental confirmation Time dilation has been tested a number of times. The routine work carried on in particle accelerators since the 1950s, such as those at CERN, is a continuously running test of the time dilation of special relativity. The specific experiments include:
Velocity time dilation tests Ives and Stilwell (1938, 1941), “An experimental study of the rate of a moving clock”, in two parts. The stated purpose of these experiments was to verify the time dilation effect, predicted by Lamor-Lorentz ether theory, due to motion through the ether using Einstein's suggestion that Doppler effect in canal rays would provide a suitable experiment. These experiments measured the Doppler shift of the radiation emitted from cathode rays, when viewed from directly in front and from directly behind. The high and low frequencies detected were not the classical values predicted.
and = and
i.e. for sources with invariant frequencies The high and low frequencies of the radiation from the moving sources were measured as
and
as deduced by Einstein (1905) from the Lorentz transformation, when the source is running slow by the Lorentz factor. Rossi and Hall (1941) compared the population of cosmic-ray-produced muons at the top of a mountain to that observed at sea level. Although the travel time for the muons from the top of the mountain to the base is several muon half-lives, the muon sample at the base was only moderately reduced. This is explained by the time dilation attributed to their high speed relative to the experimenters. That is to say, the muons were decaying about 10 times slower than if they were at rest with respect to the experimenters. Hasselkamp, Mondry, and Scharmann (1979) measured the Doppler shift from a source moving at right angles to the line of sight (the transverse Doppler shift). The most general relationship between frequencies of the radiation from the moving sources is given by:
as deduced by Einstein (1905) . For () this reduces to fdetected = frestγ. Thus there is no transverse Doppler shift, and the lower frequency of the moving source can be attributed to the time dilation effect alone. Gravitational time dilation tests Pound, Rebka in 1959 measured the very slight gravitational red shift in the frequency of light emitted at a lower height, where Earth's gravitational field is relatively more intense. The results were within 10% of the predictions of general relativity. Later Pound and Snider (in 1964) derived an even closer result of 1%. This effect is as predicted by gravitational time dilation. Velocity and gravitational time dilation combined-effect tests Hafele and Keating, in 1971, flew caesium atomic clocks east and west around the Earth in commercial airliners, to compare the elapsed time against that of a clock that remained at the US Naval Observatory. Two opposite effects came into play. The clocks were expected to age more quickly (show a larger elapsed time) than the reference clock, since they were in a higher (weaker) gravitational potential for most of the trip (c.f. Pound, Rebka). But also, contrastingly, the moving clocks were expected to age more slowly because of the speed of their travel. The gravitational effect was the larger, and the clocks suffered a net gain in elapsed time. To within experimental error, the net gain was consistent with the difference between the predicted gravitational gain and the predicted velocity time loss. In 2005, the National Physical Laboratory in the United Kingdom reported their limited replication of this experiment. The NPL experiment differed from the original in that the caesium clocks were sent on a shorter trip (London–Washington D.C. return), but the clocks were more accurate. The reported results are within 4% of the predictions of relativity. The Global Positioning System can be considered a continuously operating experiment in both special and general relativity. The in-orbit clocks are corrected for both special and general relativistic time dilation effects as described above, so that (as observed from the Earth's surface) they run at the same rate as clocks on the surface of the Earth. In addition, but not directly time dilation related, general relativistic correction terms are built into the model of motion that the satellites broadcast to receivers — uncorrected, these effects would result in an approximately 7-metre (23 ft) oscillation in the pseudo-ranges measured by a receiver over a cycle of 12 hours. Muon lifetime A comparison of muon lifetimes at different speeds is possible. In the laboratory, slow muons are produced, and in the atmosphere very fast moving muons are introduced by cosmic rays. Taking the muon lifetime at rest as the laboratory value of 2.22 μs, the lifetime of a cosmic ray produced muon traveling at 98% of the speed of light is about five times longer, in agreement with observations. In this experiment the "clock" is the time taken by processes leading to muon decay, and these processes take place in the moving muon at its own "clock rate", which is much slower than the laboratory clock.
Time dilation and space flight Time dilation would make it possible for passengers in a fast-moving vehicle to travel further into the future while aging very little, in that their great speed slows down the rate of passage of on-board time. That is, the ship's clock (and according to relativity, any human travelling with it) shows less elapsed time than the clocks of observers on Earth. For sufficiently high speeds the effect is dramatic. For example, one year of travel might correspond to ten years at home. Indeed, a constant 1 g acceleration would permit humans to travel as far as light has been able to travel since the big bang (some 13.7 billion light years) in one human lifetime. The space travellers could return to Earth billions of years in the future. A scenario based on this idea was presented in the novel Planet of the Apes by Pierre Boulle.
A more likely use of this effect would be to enable humans to travel to nearby stars without spending their entire lives aboard the ship. However, any such application of time dilation during Interstellar travel would require the use of some new, advanced method of propulsion.
Current space flight technology has fundamental theoretical limits based on the practical problem that an increasing amount of energy is required for propulsion as a craft approaches the speed of light. The likelihood of collision with small space debris and other particulate material is another practical limitation. At the velocities presently attained, however, time dilation is not a factor in space travel. Travel to regions of space-time where gravitational time dilation is taking place, such as within the gravitational field of a black hole but outside the event horizon (perhaps on a hyperbolic trajectory exiting the field), could also yield results consistent with present theory.
Time dilation at constant acceleration In special relativity, time dilation is most simply described in circumstances where relative velocity is unchanging. Nevertheless, the Lorentz equations allow one to calculate proper time and movement in space for the simple case of a spaceship whose acceleration, relative to some referent object in uniform (i.e. constant velocity) motion, equals g throughout the period of measurement.
Let t be the time in an inertial frame subsequently called the rest frame. Let x be a spatial coordinate, and let the direction of the constant acceleration as well as the spaceship's velocity (relative to the rest frame) be parallel to the x-axis. Assuming the spaceship's position at time t = 0 being x = 0 and the velocity being v0, the following formulas hold :
Position:
Velocity:
Proper time:
Time in the rest frame as a function of x:
The spacetime geometry of velocity time dilation
Time dilation in transverse motion.The green dots and red dots in the animation represent spaceships. The ships of the green fleet have no velocity relative to each other, so for the clocks onboard the individual ships the same amount of time elapses relative to each other, and they can set up a procedure to maintain a synchronized standard fleet time. The ships of the "red fleet" are moving with a velocity of 0.866 of the speed of light with respect to the green fleet.
The blue dots represent pulses of light. One cycle of light-pulses between two green ships takes two seconds of "green time", one second for each leg.
As seen from the perspective of the reds, the transit time of the light pulses they exchange among each other is one second of "red time" for each leg. As seen from the perspective of the greens, the red ships' cycle of exchanging light pulses travels a diagonal path that is two light-seconds long. (As seen from the green perspective the reds travel 1.73 () light-seconds of distance for every two seconds of green time.)
One of the red ships emits a light pulse towards the greens every second of red time. These pulses are received by ships of the green fleet with two-second intervals as measured in green time. Not shown in the animation is that all aspects of physics are proportionally involved. The lightpulses that are emitted by the reds at a particular frequency as measured in red time are received at a lower frequency as measured by the detectors of the green fleet that measure against green time, and vice versa.
The animation cycles between the green perspective and the red perspective, to emphasize the symmetry. As there is no such thing as absolute motion in relativity (as is also the case for Newtonian mechanics), both the green and the red fleet are entitled to consider themselves motionless in their own frame of reference.
Again, it is vital to understand that the results of these interactions and calculations reflect the real state of the ships as it emerges from their situation of relative motion. It is not a mere quirk of the method of measurement or communication.
See also Four-vector General relativity Hafele-Keating experiment Ives-Stilwell experiment Trouton-Rankine experiment Length contraction and Lorentz-Fitzgerald contraction Lorentz transformation Minkowski space Pound-Rebka experiment Relativistic Doppler effect and Transverse Doppler effect Relativity of simultaneity Special relativity ......) Infinity (∞)
Einstein (The Meaning of Relativity): "Two events taking place at the points A and B of a system K are simultaneous if they appear at the same instant when observed from the middle point, M, of the interval AB. Time is then defined as the ensemble of the indications of similar clocks, at rest relatively to K, which register the same simultaneously."
Einstein wrote in his book, Relativity, that simultaneity is also relative, i.e., two events that appear simultaneous to an observer in a particular inertial reference frame need not be judged as simultaneous by a second observer in a different inertial frame of reference.
Relativistic time versus Newtonian time

The animations visualise the different treatments of time in the Newtonian and the relativistic descriptions. At heart of these differences are the Galilean and Lorentz transformations applicable in the Newtonian and relativistic theories, respectively.
In the figures, the vertical direction indicates time. The horizontal direction indicates distance (only one spatial dimension is taken into account), and the thick dashed curve is the spacetime trajectory ("world line") of the observer. The small dots indicate specific (past and future) events in spacetime.
The slope of the world line (deviation from being vertical) gives the relative velocity to the observer. Note how in both pictures the view of spacetime changes when the observer accelerates.
In the Newtonian description these changes are such that time is absolute: the movements of the observer do not influence whether an event occurs in the 'now' (i.e. whether an event passes the horizontal line through the observer).
However, in the relativistic description the observability of events is absolute: the movements of the observer do not influence whether an event passes the "light cone" of the observer. Notice that with the change from a Newtonian to a relativistic description, the concept of absolute time is no longer applicable: events move up-and-down in the figure depending on the acceleration of the observer.
Arrow of time
Main article: Arrow of timeTime appears to have a direction – the past lies behind, fixed and incommutable, while the future lies ahead and is not necessarily fixed. Yet the majority of the laws of physics don't provide this arrow of time. The exceptions include the Second law of thermodynamics, which states that entropy must increase over time (see Entropy); the cosmological arrow of time, which points away from the Big Bang, and the radiative arrow of time, caused by light only traveling forwards in time. In particle physics, there is also the weak arrow of time, from CPT symmetry, and also measurement in quantum mechanics (see Measurement in quantum mechanics).
Quantised time
See also: ChrononTime quantization is a hypothetical concept. In the modern established physical theories (the Standard Model of Particles and Interactions and General Relativity) time is not quantized.
Planck time (~ 5.4 × 10 seconds) is the unit of time in the system of natural units known as Planck units. Current established physical theories are believed to fail at this time scale, and many physicists expect that the Planck time might be the smallest unit of time that could ever be measured, even in principle. Tentative physical theories that describe this time scale exist; see for instance loop quantum gravity.
Time and the Big Bang
Stephen Hawking in particular has addressed a connection between time and the Big Bang. In A Brief History of Time and elsewhere, Hawking says that even if time did not begin with the Big Bang and there were another time frame before the Big Bang, no information from events then would be accessible to us, and nothing that happened then would have any effect upon the present time-frame. Upon occasion, Hawking has stated that time actually began with the Big Bang, and that questions about what happened before the Big Bang are meaningless. This less-nuanced, but commonly repeated formulation has received criticisms from philosophers such as Aristotelian philosopher Mortimer J. Adler.
Scientists have come to some agreement on descriptions of events that happened 10 seconds after the Big Bang, but generally agree that descriptions about what happened before one Planck time (5 × 10 seconds) after the Big Bang will likely remain pure speculation.
Speculative physics beyond the Big Bang

While the Big Bang model is well established in cosmology, it is likely to be refined in the future. Little is known about the earliest moments of the universe's history. The Penrose-Hawking singularity theorems require the existence of a singularity at the beginning of cosmic time. However, these theorems assume that general relativity is correct, but general relativity must break down before the universe reaches the Planck temperature, and a correct treatment of quantum gravity may avoid the singularity.
There may also be parts of the universe well beyond what can be observed in principle. If inflation occurred this is likely, for exponential expansion would push large regions of space beyond our observable horizon.
Some proposals, each of which entails untested hypotheses, are:
- models including the Hartle-Hawking boundary condition in which the whole of space-time is finite; the Big Bang does represent the limit of time, but without the need for a singularity.
- brane cosmology models in which inflation is due to the movement of branes in string theory; the pre-big bang model; the ekpyrotic model, in which the Big Bang is the result of a collision between branes; and the cyclic model, a variant of the ekpyrotic model in which collisions occur periodically.
- chaotic inflation, in which inflation events start here and there in a random quantum-gravity foam, each leading to a bubble universe expanding from its own big bang.
Proposals in the last two categories see the Big Bang as an event in a much larger and older universe, or multiverse, and not the literal beginning.
Time travel
Main article: Time travel See also: Time travel in fiction and Grandfather paradoxTime travel is the concept of moving backwards and/or forwards to different points in time, in a manner analogous to moving through space and different from the normal "flow" of time to an earthbound observer. Although time travel has been a plot device in fiction since the 19th century, and one-way travel into the future is arguably possible given the phenomenon of time dilation in the theory of relativity, it is currently unknown whether the laws of physics would allow time travel to the past. Any technological device, whether fictional or hypothetical, that is used to achieve time travel is known as a time machine.
A central problem with time travel to the past is the violation of causality; should an effect precede its cause, it would give rise to the possibility of temporal paradox. Some interpretations of time travel resolve this by accepting the possibility of travel between parallel realities or universes.
Theory would point toward there having to be a physical dimension in which one could travel to, where the present (i.e. the point that which you are leaving) would be present at a point fixed in either the past or future. Seeing as this theory would be dependent upon the theory of a multiverse, it is uncertain how or if it would be possible to just prove the possibility of time travel.
Perception of time
Psychology
See also: Mental chronometry and Sense of timeThis common experience was used to familiarize the general public to the ideas presented by Einstein's theory of relativity in a 1930 cartoon by Sidney "George" Strube:
Man: Well, it's like this,—supposing I were to sit next to a pretty girl for half an hour it would seem like half a minute,—
Einstein: Braffo! You haf zee ideah!
Man: But if I were to sit on a hot stove for two seconds then it would seem like two hours.
A form of temporal illusion verifiable by experiment is the kappa effect, whereby time intervals between visual events are perceived as relatively longer or shorter depending on the relative spatial positions of the events. In other words: the perception of temporal intervals appears to be directly affected, in these cases, by the perception of spatial intervals.
One hour to a six-month-old person would be approximately "1:4368", while one hour to a 40-year-old would be "1:349,440". Therefore an hour appears much longer to a young child than to an aged adult, even though the measure of time is the same.
Altered states of consciousness
Altered states of consciousness are sometimes characterized by a different estimation of time. Some psychoactive substances – such as entheogens – may also dramatically alter a person's temporal judgement. When viewed under the influence of such substances as LSD, psychedelic mushrooms, and peyote, a clock may appear to be a strange reference point and a useless tool for measuring the passage of events as it does not correlate with the user's experience. At higher doses, time may appear to slow down, stop, speed up, go backwards and even seem out of sequence.
Culture
Culture is another variable contributing to the perception of time. Anthropologist Benjamin Lee Whorf reported after studying the Hopi cultures that: "… the Hopi language is seen to contain no words, grammatical forms, construction or expressions or that refer directly to what we call “time”, or to past, present, or future…"
Use of time
See also: Time managementIn sociology and anthropology, time discipline is the general name given to social and economic rules, conventions, customs, and expectations governing the measurement of time, the social currency and awareness of time measurements, and people's expectations concerning the observance of these customs by others.
The use of time is an important issue in understanding human behaviour, education, and travel behaviour. Time use research is a developing field of study. The question concerns how time is allocated across a number of activities (such as time spent at home, at work, shopping, etc.). Time use changes with technology, as the television or the Internet created new opportunities to use time in different ways. However, some aspects of time use are relatively stable over long periods of time, such as the amount of time spent traveling to work, which despite major changes in transport, has been observed to be about 20-30 minutes one-way for a large number of cities over a long period of time. This has led to the disputed time budget hypothesis.
Time management is the organization of tasks or events by first estimating how much time a task will take to be completed, when it must be completed, and then adjusting events that would interfere with its completion so that completion is reached in the appropriate amount of time. Calendars and day planners are common examples of time management tools.
Arlie Russell Hochschild and Norbert Elias have written on the use of time from a sociological perspective.
See also
Template:Misplaced Pages-Books

- See the Time navigation templates below for an exhaustive list of related articles.
Books
Organizations
Leading scholarly organizations for researchers on the history and technology of time and timekeeping
- Antiquarian Horological Society - AHS (United Kingdom)
- Association Française des Amateurs d'Horlogerie Ancienne - AFAHA (France)
- Chronometrophilia (Switzerland)
- Deutsche Gesellschaft für Chronometrie - DGC (Germany)
- HORA Associazione Italiana Cultori di Orologeria Antica (Italy)
- National Association of Watch and Clock Collectors - NAWCC (United States of America)
Miscellaneous arts and sciences
|
Miscellaneous units of time
|
Category: Horology
Notes and references
| This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed. Find sources: "Time" – news · newspapers · books · scholar · JSTOR (July 2008) (Learn how and when to remove this message) |
- E. Fitgerald, The Rubaiyat of Omar Khayyam. (Penguin 1989), Stanza lxxi
- Duff, Michael J. (2002). "Trialogue on the number of fundamental constants" (PDF). Institute of Physics Publishing for SISSA/ISAS. Retrieved 2008-02-02.
{{cite journal}}: Cite journal requires|journal=(help); Unknown parameter|coauthors=ignored (|author=suggested) (help); Unknown parameter|month=ignored (help) p. 17. "I only add to this the observation that relativity and quantum mechanics provide, in string theory, units of length and time which look, at present, more fundamental than any other." - Duff, Okun, Veneziano, ibid. p. 3. "There is no well established terminology for the fundamental constants of Nature. … The absence of accurately defined terms or the uses (i.e. actually misuses) of ill-defined terms lead to confusion and proliferation of wrong statements."
-
Rynasiewicz, Robert : Johns Hopkins University (2004-08-12). "Newton's Views on Space, Time, and Motion". Stanford Encyclopedia of Philosophy. Stanford University. Retrieved 2008-01-10.
Newton did not regard space and time as genuine substances (as are, paradigmatically, bodies and minds), but rather as real entities with their own manner of existence as necessitated by God's existence... To paraphrase: Absolute, true, and mathematical time, from its own nature, passes equably without relation the anything external, and thus without reference to any change or way of measuring of time (e.g., the hour, day, month, or year).
{{cite web}}: Unknown parameter|copyright=ignored (help) -
Markosian, Ned. "Time". In Edward N. Zalta (ed.). The Stanford Encyclopedia of Philosophy (Winter 2002 Edition).
The opposing view, normally referred to either as "Platonism with Respect to Time" or as "Absolutism with Respect to Time," has been defended by Plato, Newton, and others. On this view, time is like an empty container into which events may be placed; but it is a container that exists independently of whether or not anything is placed in it.
{{cite encyclopedia}}: Unknown parameter|accessddate=ignored (help) - Burnham, Douglas : Staffordshire University (2006). "Gottfried Wilhelm Leibniz (1646-1716) Metaphysics - 7. Space, Time, and Indiscernibles". The Internet Encyclopedia of Philosophy. Retrieved 2008-01-10.
First of all, Leibniz finds the idea that space and time might be substances or substance-like absurd (see, for example, "Correspondence with Clarke," Leibniz's Fourth Paper, §8ff). In short, an empty space would be a substance with no properties; it will be a substance that even God cannot modify or destroy.... That is, space and time are internal or intrinsic features of the complete concepts of things, not extrinsic.... Leibniz's view has two major implications. First, there is no absolute location in either space or time; location is always the situation of an object or event relative to other objects and events. Second, space and time are not in themselves real (that is, not substances). Space and time are, rather, ideal. Space and time are just metaphysically illegitimate ways of perceiving certain virtual relations between substances. They are phenomena or, strictly speaking, illusions (although they are illusions that are well-founded upon the internal properties of substances).... It is sometimes convenient to think of space and time as something "out there," over and above the entities and their relations to each other, but this convenience must not be confused with reality. Space is nothing but the order of co-existent objects; time nothing but the order of successive events. This is usually called a relational theory of space and time.
-
Mattey, G. J. : UC Davis (1997-01-22). "Critique of Pure Reason, Lecture notes: Philosophy 175 UC Davis". Retrieved 2008-01-10.
What is correct in the Leibnizian view was its anti-metaphysical stance. Space and time do not exist in and of themselves, but in some sense are the product of the way we represent things. The are ideal, though not in the sense in which Leibniz thought they are ideal (figments of the imagination). The ideality of space is its mind-dependence: it is only a condition of sensibility.... Kant concluded "absolute space is not an object of outer sensation; it is rather a fundamental concept which first of all makes possible all such outer sensation."...Much of the argumentation pertaining to space is applicable, mutatis mutandis, to time, so I will not rehearse the arguments. As space is the form of outer intuition, so time is the form of inner intuition.... Kant claimed that time is real, it is "the real form of inner intuition."
-
McCormick, Matt : California State University, Sacramento (2006). "Immanuel Kant (1724-1804) Metaphysics : 4. Kant's Transcendental Idealism". The Internet Encyclopedia of Philosophy. Retrieved 2008-01-10.
Time, Kant argues, is also necessary as a form or condition of our intuitions of objects. The idea of time itself cannot be gathered from experience because succession and simultaneity of objects, the phenomena that would indicate the passage of time, would be impossible to represent if we did not already possess the capacity to represent objects in time.... Another way to put the point is to say that the fact that the mind of the knower makes the a priori contribution does not mean that space and time or the categories are mere figments of the imagination. Kant is an empirical realist about the world we experience; we can know objects as they appear to us. He gives a robust defense of science and the study of the natural world from his argument about the mind's role in making nature. All discursive, rational beings must conceive of the physical world as spatially and temporally unified, he argues.
{{cite web}}: CS1 maint: multiple names: authors list (link) - Richards, E. G. (1998). Mapping Time: The Calendar and its History. Oxford University Press. pp. 3–5.
- Rudgley, Richard (1999). The Lost Civilizations of the Stone Age. New York: Simon & Schuster. pp. 86–105.
- Barnett, Jo Ellen Time's Pendulum: The Quest to Capture Time—from Sundials to Atomic Clocks Plenum, 1998 ISBN 0-306-45787-3 p.28
- Barnett, ibid, p.37
- Laurence Bergreen, Over the Edge of the World: Magellan's Terrifying Circumnavigation of the Globe, HarperCollins Publishers, 2003, hardcover 480 pages, ISBN 0-06-621173-5
- North, J. (2004) God's Clockmaker: Richard of Wallingford and the Invention of Time. Oxbow Books. ISBN 1-85285-451-0
- Watson, E (1979) "The St Albans Clock of Richard of Wallingford". Antiquarian Horology 372-384.
- "New atomic clock can keep time for 200 million years: Super-precise instruments vital to deep space navigation". Vancouver Sun. 2008-02-16. Retrieved 2008-02-16.
- "Byrhtferth of Ramsey". (2008). In Encyclopædia Britannica. Retrieved September 15, 2008, from Encyclopædia Britannica Online: http://search.eb.com/eb/article-9438957
- "atom", Oxford English Dictionary, Draft Revision Sept. 2008 (contains relevant citations from Byrhtferth's Enchiridion)
- "Shortest time interval measured". BBC News. 2004-02-25.
- "Fastest view of molecular motion". BBC News. 2006-03-04.
- "New Scientist article". Retrieved 2008-11-27.
- ^ Organisation Intergouvernementale de la Convention du Métre (1998). The International System of Units (SI), 7th Edition (PDF). Retrieved 2006-06-13.
- "Base unit definitions: Second". NIST. Retrieved 2008-01-09.
- Dagobert Runes, Dictionary of Philosophy, p. 318
- St. Augustine, Confessions, Book 11. http://ccat.sas.upenn.edu/jod/augustine/Pusey/book11 (Accessed 5/26/07).
- Gottfried Martin, Kant's Metaphysics and Theory of Science
- Kant, Immanuel (1787). The Critique of Pure Reason, 2nd edition. translated by J. M. D. Meiklejohn, eBooks@Adelaide, 2004—http://ebooks.adelaide.edu.au/k/kant/immanuel/k16p/k16p15.html
- Bergson, Henri (1907) Creative Evolution. trans. by Arthur Mitchell. Mineola: Dover, 1998.
- Harry Foundalis. "You are about to disappear". Retrieved 2007-04-27.
- Tom Huston. "Buddhism and the illusion of time". Retrieved 2009-08-19.
- "Time is an illusion?". Retrieved 2007-04-27.
- Herman M. Schwartz, Introduction to Special Relativity, McGraw-Hill Book Company, 1968, hardcover 442 pages, see ISBN 0882754785 (1977 edition), pp. 10-13
- A. Einstein, H. A. Lorentz, H. Weyl, H. Minkowski, The Principle of Relativity, Dover Publications, Inc, 2000, softcover 216 pages, ISBN 0486600815, See pp. 37-65 for an English translation of Einstein's original 1905 paper.
- Hawking, Stephen. "The Beginning of Time". University of Cambridge. Retrieved 2008-01-10.
Since events before the Big Bang have no observational consequences, one may as well cut them out of the theory, and say that time began at the Big Bang. Events before the Big Bang, are simply not defined, because there's no way one could measure what happened at them. This kind of beginning to the universe, and of time itself, is very different to the beginnings that had been considered earlier.
- Hawking, Stephen. "The Beginning of Time". University of Cambridge. Retrieved 2008-01-10.
The conclusion of this lecture is that the universe has not existed forever. Rather, the universe, and time itself, had a beginning in the Big Bang, about 15 billion years ago.
- Hawking, Stephen (2006-02-27). "Professor Stephen Hawking lectures on the origin of the universe". University of Oxford. Retrieved 2008-01-10.
Suppose the beginning of the universe was like the South Pole of the earth, with degrees of latitude playing the role of time. The universe would start as a point at the South Pole. As one moves north, the circles of constant latitude, representing the size of the universe, would expand. To ask what happened before the beginning of the universe would become a meaningless question because there is nothing south of the South Pole.'
- Ghandchi, Sam : Editor/Publisher (2004-01-16). "Space and New Thinking". Retrieved 2008-01-10.
and as Stephen Hawking puts it, asking what was before Big Bang is like asking what is North of North Pole, a meaningless question.
{{cite web}}:|first=has generic name (help) - Adler, Mortimer J., Ph.D. "Natural Theology, Chance, and God". Retrieved 2008-01-10.
Hawking could have avoided the error of supposing that time had a beginning with the Big Bang if he had distinguished time as it is measured by physicists from time that is not measurable by physicists.... an error shared by many other great physicists in the twentieth century, the error of saying that what cannot be measured by physicists does not exist in reality.
{{cite web}}: CS1 maint: multiple names: authors list (link) "The Great Ideas Today". Encyclopaedia Britannica. 1992. -
Adler, Mortimer J., Ph.D. "Natural Theology, Chance, and God". Retrieved 2008-01-10.
Where Einstein had said that what is not measurable by physicists is of no interest to them, Hawking flatly asserts that what is not measurable by physicists does not exist — has no reality whatsoever.
With respect to time, that amounts to the denial of psychological time which is not measurable by physicists, and also to everlasting time — time before the Big Bang — which physics cannot measure. Hawking does not know that both Aquinas and Kant had shown that we cannot rationally establish that time is either finite or infinite.{{cite web}}: CS1 maint: multiple names: authors list (link) "The Great Ideas Today". Encyclopaedia Britannica. 1992. - Hawking, Stephen; and Ellis, G. F. R. (1973). The Large Scale Structure of Space-Time. Cambridge: Cambridge University Press. ISBN 0-521-09906-4.
{{cite book}}: CS1 maint: multiple names: authors list (link) - J. Hartle and S. W. Hawking (1983). "Wave function of the universe". Phys. Rev. D. 28: 2960. doi:10.1103/PhysRevD.28.2960.
- Langlois, David (2002). "Brane cosmology: an introduction". arXiv:hep-th/0209261.
{{cite journal}}: Cite journal requires|journal=(help) - Linde, Andre (2002). "Inflationary Theory versus Ekpyrotic/Cyclic Scenario". arXiv:hep-th/0205259.
{{cite journal}}: Cite journal requires|journal=(help) - "Recycled Universe: Theory Could Solve Cosmic Mystery". Space.com. 8 May 2006. Retrieved 2007-07-03.
{{cite news}}: Check date values in:|date=(help) - "What Happened Before the Big Bang?". Retrieved 2007-07-03.
- A. Linde (1986). "Eternal chaotic inflation". Mod. Phys. Lett. A1: 81.
A. Linde (1986). "Eternally existing self-reproducing chaotic inflationary universe". Phys. Lett. B175: 395–400. - Priestley, J. B. (1964). Man and Time. New York: Crescent Books. p. 96.
- Sunrise (2008). "Unified Field Theory: A new interpretation" (PDF). Chapter 2—The Development of the Unified Field Theory, pg. 31. Sunrise Information Services.
- Wada Y, Masuda T, Noguchi K, 2005, "Temporal illusion called 'kappa effect' in event perception" Perception 34 ECVP Abstract Supplement
- Carroll, John B. (ed.)(1956). Language Thought and Reality. Selected Writings of Benjamin Lee Whorf. MIT Press, Boston, Massachusetts. ISBN 0262730065 9780262730068
Further reading
- Barbour, Julian (1999). The End of Time: The Next Revolution in Physics. Oxford University Press. ISBN 0-19-514592-5.
- Landes, David (2000). Revolution in Time. Harvard University Press. ISBN 0-674-00282-2.
{{cite book}}: Cite has empty unknown parameter:|1=(help) - Das, Tushar Kanti (1990). The Time Dimension: An Interdisciplinary Guide. New York: Praeger. ISBN 0275926818.- Research bibliography
- Davies, Paul (1996). About Time: Einstein's Unfinished Revolution. New York: Simon & Schuster Paperbacks. ISBN 0-684-81822-1.
- Feynman, Richard (1994) . The Character of Physical Law. Cambridge (Mass): The MIT Press. pp. 108–126. ISBN 0-262-56003-8.
- Galison, Peter (1992). Einstein's Clocks and Poincaré's Maps: Empires of Time. New York: W. W. Norton. ISBN 0-393-02001-0.
- Highfield, Roger (1992). Arrow of Time: A Voyage through Science to Solve Time's Greatest Mystery. Random House. ISBN 0-449-90723-6.
- Mermin, N. David (2005). It's About Time: Understanding Einstein's Relativity. Princeton University Press. ISBN 0-691-12201-6.
- Penrose, Roger (1999) . The Emperor's New Mind: Concerning Computers, Minds, and the Laws of Physics. New York: Oxford University Press. pp. 391–417. ISBN 0-19-286198-0.
- Price, Huw (1996). Time's Arrow and Archimedes' Point. Oxford University Press. ISBN 0-19-511798-0.
- Reichenbach, Hans (1999) . The Direction of Time. New York: Dover. ISBN 0-486-40926-0.
- Stiegler, Bernard, Technics and Time, 1: The Fault of Epimetheus
- Whitrow, Gerald J. (1973). The Nature of Time. Holt, Rinehart and Wilson (New York).
- Whitrow, Gerald J. (1980). The Natural Philosophy of Time. Clarendon Press (Oxford).
- Whitrow, Gerald J. (1988). Time in History. The evolution of our general awareness of time and temporal perspective. Oxford University Press. ISBN 0-19-285211-6.
- Rovelli, Carlo (2006). What is time? What is space?. Rome: Di Renzo Editore. ISBN 8883231465.
- Charlie Gere, (2005) Art, Time and Technology: Histories of the Disappearing Body, Berg
External links
| This article's use of external links may not follow Misplaced Pages's policies or guidelines. Please improve this article by removing excessive or inappropriate external links, and converting useful links where appropriate into footnote references. (Learn how and when to remove this message) |
- Time at Open Directory
- Royal Observatory & history of astronomy: John Harrison and the Longitude problem
Perception of time
Physics
Philosophy
- Eastern Philosophy
- The Conceptual Scheme of Chinese Philosophical Thinking - Time
- Flaws in the Mind: The Invention of Time A site exploring J. Krishnamurti's views on psychological time.
- Western Philosophy
- Crouch, Will (2006–2008). "Is there a defensible argument for the non-existence of time?". On Philosophy. Retrieved 2008-01-24.
{{cite web}}: Text "Copyright James Nicholson" ignored (help)CS1 maint: date format (link) - Dowden, Bradley (California State University, Sacramento) (2007). "Time". In James Fieser, Ph.D., Bradley Dowden, Ph.D. (ed.). The Internet Encyclopedia of Philosophy. Retrieved 2008-01-31.
{{cite encyclopedia}}: CS1 maint: multiple names: authors list (link) - Le Poidevin, Robin (Winter 2004). "The Experience and Perception of Time". In Edward N. Zalta (ed.). The Stanford Encyclopedia of Philosophy. Retrieved 2008-01-17.
{{cite encyclopedia}}: CS1 maint: year (link) - Mcdonough, Jeff (Harvard University) (Winter 2007). "Leibniz's Philosophy of Physics". In Edward N. Zalta (ed.). The Stanford Encyclopedia of Philosophy. Stanford University. Retrieved 2008-01-31.
{{cite encyclopedia}}: Unknown parameter|copyright=ignored (help)CS1 maint: year (link) - Ross, Kelley L., Ph.D. (Los Angeles Valley College). "The Clarke-Leibniz Debate (1715-1716)". The Proceedings of the Friesian School, Fourth Series (1996, 1999, 2001). Retrieved 2008-01-17.
{{cite web}}: CS1 maint: multiple names: authors list (link) - Ross, Kelley L., Ph.D. (Los Angeles Valley College). "Three Points in Kant's Theory of Space and Time". The Proceedings of the Friesian School, Fourth Series (1996, 1999, 2001). Retrieved 2008-01-17.
{{cite web}}: CS1 maint: multiple names: authors list (link) - Savitt, Steven, Ph.D. (University of British Columbia) (Fall 2007). "Being and Becoming in Modern Physics". In Edward N. Zalta (ed.). The Stanford Encyclopedia of Philosophy. Retrieved 2008-01-17.
{{cite encyclopedia}}: CS1 maint: multiple names: authors list (link) CS1 maint: year (link) - Wilson, Catherine (City University of New York) (Summer 2004). "Kant and Leibniz". In Edward N. Zalta (ed.). The Stanford Encyclopedia of Philosophy. Stanford University. ISSN 1095-5054. Retrieved 2008-01-31.
{{cite encyclopedia}}: CS1 maint: year (link)
Timekeeping
- Different systems of measuring time
- UTC/TAI Timeserver
- BBC article on shortest time ever measured
- Federation of the Swiss Watch Industry FH
- American Watchmakers-Clockmakers Institute
- National Association of Watch & Clock Collectors
Miscellaneous
- Time-and-date.net : Accurate Time, Zones, perpetual calendar
- Exploring Time from Planck Time to the lifespan of the universe
- International Society for the Study of Time
Navigation templates
| Time | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| Key concepts | |||||||||
| Measurement and standards |
| ||||||||
| Philosophy of time | |||||||||
| Human experience and use of time | |||||||||
| Time in science |
| ||||||||
| Related | |||||||||


