| Demihepteract (7-demicube) | ||
|---|---|---|
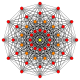
 Petrie polygon projection | ||
| Type | Uniform 7-polytope | |
| Family | demihypercube | |
| Coxeter symbol | 141 | |
| Schläfli symbol | {3,3} = h{4,3} s{2} | |
| Coxeter diagrams |
| |
| 6-faces | 78 | 14 {3} 64 {3} |
| 5-faces | 532 | 84 {3} 448 {3} |
| 4-faces | 1624 | 280 {3} 1344 {3} |
| Cells | 2800 | 560 {3} 2240 {3,3} |
| Faces | 2240 | {3} |
| Edges | 672 | |
| Vertices | 64 | |
| Vertex figure | Rectified 6-simplex | |
| Symmetry group | D7, = | |
| Dual | ? | |
| Properties | convex | |
In geometry, a demihepteract or 7-demicube is a uniform 7-polytope, constructed from the 7-hypercube (hepteract) with alternated vertices removed. It is part of a dimensionally infinite family of uniform polytopes called demihypercubes.
E. L. Elte identified it in 1912 as a semiregular polytope, labeling it as HM7 for a 7-dimensional half measure polytope.
Coxeter named this polytope as 141 from its Coxeter diagram, with a ring on one of the 1-length branches, ![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]() and Schläfli symbol or {3,3}.
and Schläfli symbol or {3,3}.
Cartesian coordinates
Cartesian coordinates for the vertices of a demihepteract centered at the origin are alternate halves of the hepteract:
- (±1,±1,±1,±1,±1,±1,±1)
with an odd number of plus signs.
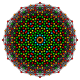
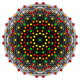
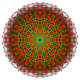
Images
| Coxeter plane |
B7 | D7 | D6 |
|---|---|---|---|
| Graph | 
|
|
|
| Dihedral symmetry |
|||
| Coxeter plane | D5 | D4 | D3 |
| Graph | 
|

|

|
| Dihedral symmetry |
|||
| Coxeter plane |
A5 | A3 | |
| Graph | 
|
| |
| Dihedral symmetry |
As a configuration
This configuration matrix represents the 7-demicube. The rows and columns correspond to vertices, edges, faces, cells, 4-faces, 5-faces and 6-faces. The diagonal numbers say how many of each element occur in the whole 7-demicube. The nondiagonal numbers say how many of the column's element occur in or at the row's element.
The diagonal f-vector numbers are derived through the Wythoff construction, dividing the full group order of a subgroup order by removing one mirror at a time.
| D7 | k-face | fk | f0 | f1 | f2 | f3 | f4 | f5 | f6 | k-figures | notes | |||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| A6 | ( ) | f0 | 64 | 21 | 105 | 35 | 140 | 35 | 105 | 21 | 42 | 7 | 7 | 041 | D7/A6 = 64*7!/7! = 64 | |
| A4A1A1 | { } | f1 | 2 | 672 | 10 | 5 | 20 | 10 | 20 | 10 | 10 | 5 | 2 | { }×{3,3,3} | D7/A4A1A1 = 64*7!/5!/2/2 = 672 | |
| A3A2 | 100 | f2 | 3 | 3 | 2240 | 1 | 4 | 4 | 6 | 6 | 4 | 4 | 1 | {3,3}v( ) | D7/A3A2 = 64*7!/4!/3! = 2240 | |
| A3A3 | 101 | f3 | 4 | 6 | 4 | 560 | * | 4 | 0 | 6 | 0 | 4 | 0 | {3,3} | D7/A3A3 = 64*7!/4!/4! = 560 | |
| A3A2 | 110 | 4 | 6 | 4 | * | 2240 | 1 | 3 | 3 | 3 | 3 | 1 | {3}v( ) | D7/A3A2 = 64*7!/4!/3! = 2240 | ||
| D4A2 | 111 | f4 | 8 | 24 | 32 | 8 | 8 | 280 | * | 3 | 0 | 3 | 0 | {3} | D7/D4A2 = 64*7!/8/4!/2 = 280 | |
| A4A1 | 120 | 5 | 10 | 10 | 0 | 5 | * | 1344 | 1 | 2 | 2 | 1 | { }v( ) | D7/A4A1 = 64*7!/5!/2 = 1344 | ||
| D5A1 | 121 | f5 | 16 | 80 | 160 | 40 | 80 | 10 | 16 | 84 | * | 2 | 0 | { } | D7/D5A1 = 64*7!/16/5!/2 = 84 | |
| A5 | 130 | 6 | 15 | 20 | 0 | 15 | 0 | 6 | * | 448 | 1 | 1 | D7/A5 = 64*7!/6! = 448 | |||
| D6 | 131 | f6 | 32 | 240 | 640 | 160 | 480 | 60 | 192 | 12 | 32 | 14 | * | ( ) | D7/D6 = 64*7!/32/6! = 14 | |
| A6 | 140 | 7 | 21 | 35 | 0 | 35 | 0 | 21 | 0 | 7 | * | 64 | D7/A6 = 64*7!/7! = 64 | |||
Related polytopes
There are 95 uniform polytopes with D6 symmetry, 63 are shared by the B6 symmetry, and 32 are unique:
References
- Coxeter, Regular Polytopes, sec 1.8 Configurations
- Coxeter, Complex Regular Polytopes, p.117
- Klitzing, Richard. "x3o3o *b3o3o3o - hax".
- H.S.M. Coxeter:
- Coxeter, Regular Polytopes, (3rd edition, 1973), Dover edition, ISBN 0-486-61480-8, p. 296, Table I (iii): Regular Polytopes, three regular polytopes in n-dimensions (n≥5)
- H.S.M. Coxeter, Regular Polytopes, 3rd Edition, Dover New York, 1973, p. 296, Table I (iii): Regular Polytopes, three regular polytopes in n-dimensions (n≥5)
- Kaleidoscopes: Selected Writings of H.S.M. Coxeter, edited by F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication, 1995, ISBN 978-0-471-01003-6
- (Paper 22) H.S.M. Coxeter, Regular and Semi Regular Polytopes I,
- (Paper 23) H.S.M. Coxeter, Regular and Semi-Regular Polytopes II,
- (Paper 24) H.S.M. Coxeter, Regular and Semi-Regular Polytopes III,
- John H. Conway, Heidi Burgiel, Chaim Goodman-Strauss, The Symmetries of Things 2008, ISBN 978-1-56881-220-5 (Chapter 26. pp. 409: Hemicubes: 1n1)
- Klitzing, Richard. "7D uniform polytopes (polyexa) x3o3o *b3o3o3o3o - hesa".
External links
- Olshevsky, George. "Demihepteract". Glossary for Hyperspace. Archived from the original on 4 February 2007.
- Multi-dimensional Glossary
| Fundamental convex regular and uniform polytopes in dimensions 2–10 | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Family | An | Bn | I2(p) / Dn | E6 / E7 / E8 / F4 / G2 | Hn | |||||||
| Regular polygon | Triangle | Square | p-gon | Hexagon | Pentagon | |||||||
| Uniform polyhedron | Tetrahedron | Octahedron • Cube | Demicube | Dodecahedron • Icosahedron | ||||||||
| Uniform polychoron | Pentachoron | 16-cell • Tesseract | Demitesseract | 24-cell | 120-cell • 600-cell | |||||||
| Uniform 5-polytope | 5-simplex | 5-orthoplex • 5-cube | 5-demicube | |||||||||
| Uniform 6-polytope | 6-simplex | 6-orthoplex • 6-cube | 6-demicube | 122 • 221 | ||||||||
| Uniform 7-polytope | 7-simplex | 7-orthoplex • 7-cube | 7-demicube | 132 • 231 • 321 | ||||||||
| Uniform 8-polytope | 8-simplex | 8-orthoplex • 8-cube | 8-demicube | 142 • 241 • 421 | ||||||||
| Uniform 9-polytope | 9-simplex | 9-orthoplex • 9-cube | 9-demicube | |||||||||
| Uniform 10-polytope | 10-simplex | 10-orthoplex • 10-cube | 10-demicube | |||||||||
| Uniform n-polytope | n-simplex | n-orthoplex • n-cube | n-demicube | 1k2 • 2k1 • k21 | n-pentagonal polytope | |||||||
| Topics: Polytope families • Regular polytope • List of regular polytopes and compounds | ||||||||||||
 or {3,3}.
or {3,3}.