This article has multiple issues. Please help improve it or discuss these issues on the talk page. (Learn how and when to remove these messages)
|
In mathematics, and particularly category theory, a coherence condition is a collection of conditions requiring that various compositions of elementary morphisms are equal. Typically the elementary morphisms are part of the data of the category. A coherence theorem states that, in order to be assured that all these equalities hold, it suffices to check a small number of identities.
An illustrative example: a monoidal category
Part of the data of a monoidal category is a chosen morphism , called the associator:
for each triple of objects in the category. Using compositions of these , one can construct a morphism
Actually, there are many ways to construct such a morphism as a composition of various . One coherence condition that is typically imposed is that these compositions are all equal.
Typically one proves a coherence condition using a coherence theorem, which states that one only needs to check a few equalities of compositions in order to show that the rest also hold. In the above example, one only needs to check that, for all quadruples of objects , the following diagram commutes.
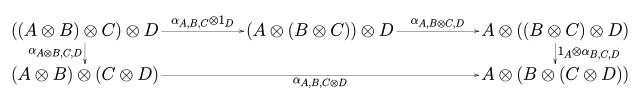
Any pair of morphisms from to constructed as compositions of various are equal.
Further examples
Two simple examples that illustrate the definition are as follows. Both are directly from the definition of a category.
Identity
Let f : A → B be a morphism of a category containing two objects A and B. Associated with these objects are the identity morphisms 1A : A → A and 1B : B → B. By composing these with f, we construct two morphisms:
- f o 1A : A → B, and
- 1B o f : A → B.
Both are morphisms between the same objects as f. We have, accordingly, the following coherence statement:
- f o 1A = f = 1B o f.
Associativity of composition
Let f : A → B, g : B → C and h : C → D be morphisms of a category containing objects A, B, C and D. By repeated composition, we can construct a morphism from A to D in two ways:
- (h o g) o f : A → D, and
- h o (g o f) : A → D.
We have now the following coherence statement:
- (h o g) o f = h o (g o f).
In these two particular examples, the coherence statements are theorems for the case of an abstract category, since they follow directly from the axioms; in fact, they are axioms. For the case of a concrete mathematical structure, they can be viewed as conditions, namely as requirements for the mathematical structure under consideration to be a concrete category, requirements that such a structure may meet or fail to meet.
See also
Notes
- (Kelly 1964, Introduction)
References
- Kelly, G.M (1964). "On MacLane's conditions for coherence of natural associativities, commutativities, etc". Journal of Algebra. 1 (4): 397–402. doi:10.1016/0021-8693(64)90018-3.
- Kelly, G. M.; Laplaza, M.; Lewis, G.; Mac Lane, Saunders (1972). Coherence in Categories. Lecture Notes in Mathematics. Vol. 281. doi:10.1007/BFb0059553. ISBN 978-3-540-05963-9.
- Im, Geun Bin; Kelly, G.M. (1986). "A universal property of the convolution monoidal structure". Journal of Pure and Applied Algebra. 43: 75–88. doi:10.1016/0022-4049(86)90005-8.
- Kassel, Christian (1995). "Tensor Categories". Quantum Groups. Graduate Texts in Mathematics. Vol. 155. pp. 275–293. doi:10.1007/978-1-4612-0783-2_11. ISBN 978-1-4612-6900-7.
- Laplaza, Miguel L. (1972). "Coherence for distributivity". Coherence in Categories. Lecture Notes in Mathematics. Vol. 281. pp. 29–65. doi:10.1007/BFb0059555. ISBN 978-3-540-05963-9.
- Lack, Stephen (2000). "A Coherent Approach to Pseudomonads". Advances in Mathematics. 152 (2): 179–202. doi:10.1006/aima.1999.1881.
- MacLane, Saunders (October 1963). "Natural Associativity and Commutativity". Rice Institute Pamphlet - Rice University Studies. hdl:1911/62865.
- Mac Lane, Saunders (1971). "7. Monoids §2 Coherence". Categories for the working mathematician. Graduate texts in mathematics. Vol. 4. Springer. pp. 161–165. doi:10.1007/978-1-4612-9839-7_8. ISBN 9781461298397.
- MacLane, Saunders; Paré, Robert (1985). "Coherence for bicategories and indexed categories". Journal of Pure and Applied Algebra. 37: 59–80. doi:10.1016/0022-4049(85)90087-8.
- Power, A.J. (1989). "A general coherence result". Journal of Pure and Applied Algebra. 57 (2): 165–173. doi:10.1016/0022-4049(89)90113-8.
- Yanofsky, Noson S. (2000). "The syntax of coherence". Cahiers de Topologie et Géométrie Différentielle Catégoriques. 41 (4): 255–304.
External links
- Malkiewich, Cary; Ponto, Kate (2021). "Coherence for bicategories, lax functors, and shadows". arXiv:2109.01249 .
 , called the associator:
, called the associator:

 in the category. Using compositions of these
in the category. Using compositions of these 
 , the following diagram commutes.
, the following diagram commutes.
 to
to  constructed as compositions of various
constructed as compositions of various