Inner automorphism - Misplaced Pages

(Redirected from Conjugation map) Automorphism of a group, ring, or algebra given by the conjugation action of one of its elements

In abstract algebra, an inner automorphism is an automorphism of a group, ring, or algebra given by the conjugation action of a fixed element, called the conjugating element. They can be realized via operations from within the group itself, hence the adjective "inner". These inner automorphisms form a subgroup of the automorphism group, and the quotient of the automorphism group by this subgroup is defined as the outer automorphism group.

Definition

If G is a group and g is an element of G (alternatively, if G is a ring, and g is a unit), then the function

{\begin{aligned}\varphi _{g}\colon G&\to G\\\varphi _{g}(x)&:=g^{-1}xg\end{aligned}}

is called (right) conjugation by g (see also conjugacy class). This function is an endomorphism of G: for all $x_{1},x_{2}\in G,$

\varphi _{g}(x_{1}x_{2})=g^{-1}x_{1}x_{2}g=g^{-1}x_{1}\left(gg^{-1}\right)x_{2}g=\left(g^{-1}x_{1}g\right)\left(g^{-1}x_{2}g\right)=\varphi _{g}(x_{1})\varphi _{g}(x_{2}),

where the second equality is given by the insertion of the identity between $x_{1}$ and $x_{2}.$ Furthermore, it has a left and right inverse, namely $\varphi _{g^{-1}}.$ Thus, $\varphi _{g}$ is both an monomorphism and epimorphism, and so an isomorphism of G with itself, i.e. an automorphism. An inner automorphism is any automorphism that arises from conjugation.

When discussing right conjugation, the expression $g^{-1}xg$ is often denoted exponentially by $x^{g}.$ This notation is used because composition of conjugations satisfies the identity: $\left(x^{g_{1}}\right)^{g_{2}}=x^{g_{1}g_{2}}$ for all $g_{1},g_{2}\in G.$ This shows that right conjugation gives a right action of G on itself.

A common example is as follows:

Describe a homomorphism $\Phi$ for which the image, ${\text{Im}}(\Phi )$ , is a normal subgroup of inner automorphisms of a group $G$ ; alternatively, describe a natural homomorphism of which the kernel of $\Phi$ is the center of $G$ (all $g\in G$ for which conjugating by them returns the trivial automorphism), in other words, ${\text{Ker}}(\Phi )={\text{Z}}(G)$ . There is always a natural homomorphism $\Phi :G\to {\text{Aut}}(G)$ , which associates to every $g\in G$ an (inner) automorphism $\varphi _{g}$ in ${\text{Aut}}(G)$ . Put identically, $\Phi :g\mapsto \varphi _{g}$ .

Let $\varphi _{g}(x):=gxg^{-1}$ as defined above. This requires demonstrating that (1) $\varphi _{g}$ is a homomorphism, (2) $\varphi _{g}$ is also a bijection, (3) $\Phi$ is a homomorphism.

$\varphi _{g}(xx')=gxx'g^{-1}=gx(g^{-1}g)x'g^{-1}=(gxg^{-1})(gx'g^{-1})=\varphi _{g}(x)\varphi _{g}(x')$
The condition for bijectivity may be verified by simply presenting an inverse such that we can return to $x$ from $gxg^{-1}$ . In this case it is conjugation by $g^{-1}$ denoted as $\varphi _{g^{-1}}$ .
$\Phi (gg')(x)=(gg')x(gg')^{-1}$ and $\Phi (g)\circ \Phi (g')(x)=\Phi (g)\circ (g'hg'^{-1})=gg'hg'^{-1}g^{-1}=(gg')h(gg')^{-1}$

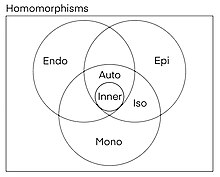
Inner and outer automorphism groups

The composition of two inner automorphisms is again an inner automorphism, and with this operation, the collection of all inner automorphisms of G is a group, the inner automorphism group of G denoted Inn(G).

Inn(G) is a normal subgroup of the full automorphism group Aut(G) of G. The outer automorphism group, Out(G) is the quotient group

\operatorname {Out} (G)=\operatorname {Aut} (G)/\operatorname {Inn} (G).

The outer automorphism group measures, in a sense, how many automorphisms of G are not inner. Every non-inner automorphism yields a non-trivial element of Out(G), but different non-inner automorphisms may yield the same element of Out(G).

Saying that conjugation of x by a leaves x unchanged is equivalent to saying that a and x commute:

a^{-1}xa=x\iff xa=ax.

Therefore the existence and number of inner automorphisms that are not the identity mapping is a kind of measure of the failure of the commutative law in the group (or ring).

An automorphism of a group G is inner if and only if it extends to every group containing G.

By associating the element a ∈ G with the inner automorphism f(x) = x in Inn(G) as above, one obtains an isomorphism between the quotient group G / Z(G) (where Z(G) is the center of G) and the inner automorphism group:

G\,/\,\mathrm {Z} (G)\cong \operatorname {Inn} (G).

This is a consequence of the first isomorphism theorem, because Z(G) is precisely the set of those elements of G that give the identity mapping as corresponding inner automorphism (conjugation changes nothing).

Non-inner automorphisms of finite p-groups

A result of Wolfgang Gaschütz says that if G is a finite non-abelian p-group, then G has an automorphism of p-power order which is not inner.

It is an open problem whether every non-abelian p-group G has an automorphism of order p. The latter question has positive answer whenever G has one of the following conditions:

G is nilpotent of class 2
G is a regular p-group
G / Z(G) is a powerful p-group
The centralizer in G, C_G, of the center, Z, of the Frattini subgroup, Φ, of G, C_G ∘ Z ∘ Φ(G), is not equal to Φ(G)

Types of groups

The inner automorphism group of a group G, Inn(G), is trivial (i.e., consists only of the identity element) if and only if G is abelian.

The group Inn(G) is cyclic only when it is trivial.

At the opposite end of the spectrum, the inner automorphisms may exhaust the entire automorphism group; a group whose automorphisms are all inner and whose center is trivial is called complete. This is the case for all of the symmetric groups on n elements when n is not 2 or 6. When n = 6, the symmetric group has a unique non-trivial class of non-inner automorphisms, and when n = 2, the symmetric group, despite having no non-inner automorphisms, is abelian, giving a non-trivial center, disqualifying it from being complete.

If the inner automorphism group of a perfect group G is simple, then G is called quasisimple.

Lie algebra case

An automorphism of a Lie algebra 𝔊 is called an inner automorphism if it is of the form Ad_g, where Ad is the adjoint map and g is an element of a Lie group whose Lie algebra is 𝔊. The notion of inner automorphism for Lie algebras is compatible with the notion for groups in the sense that an inner automorphism of a Lie group induces a unique inner automorphism of the corresponding Lie algebra.

Extension

If G is the group of units of a ring, A, then an inner automorphism on G can be extended to a mapping on the projective line over A by the group of units of the matrix ring, M₂(A). In particular, the inner automorphisms of the classical groups can be extended in that way.

References

Dummit, David S.; Foote, Richard M. (2004). Abstract algebra (3rd ed.). Hoboken, NJ: Wiley. p. 45. ISBN 978-0-4714-5234-8. OCLC 248917264.
Grillet, Pierre (2010). Abstract Algebra (2nd ed.). New York: Springer. p. 56. ISBN 978-1-4419-2450-6.
Lang, Serge (2002). Algebra (3rd ed.). New York: Springer-Verlag. p. 26. ISBN 978-0-387-95385-4.
Schupp, Paul E. (1987), "A characterization of inner automorphisms" (PDF), Proceedings of the American Mathematical Society, 101 (2), American Mathematical Society: 226–228, doi:10.2307/2045986, JSTOR 2045986, MR 0902532