| This article may be too technical for most readers to understand. Please help improve it to make it understandable to non-experts, without removing the technical details. (June 2022) (Learn how and when to remove this message) |
In mathematics, planar algebras first appeared in the work of Vaughan Jones on the standard invariant of a II1 subfactor. They also provide an appropriate algebraic framework for many knot invariants (in particular the Jones polynomial), and have been used in describing the properties of Khovanov homology with respect to tangle composition. Any subfactor planar algebra provides a family of unitary representations of Thompson groups. Any finite group (and quantum generalization) can be encoded as a planar algebra.
Definition
The idea of the planar algebra is to be a diagrammatic axiomatization of the standard invariant.
Planar tangle
A (shaded) planar tangle is the data of finitely many input disks, one output disk, non-intersecting strings giving an even number, say , intervals per disk and one -marked interval per disk.
Here, the mark is shown as a -shape. On each input disk it is placed between two adjacent outgoing strings, and on the output disk it is placed between two adjacent incoming strings. A planar tangle is defined up to isotopy.
Composition
To compose two planar tangles, put the output disk of one into an input of the other, having as many intervals, same shading of marked intervals and such that the -marked intervals coincide. Finally we remove the coinciding circles. Note that two planar tangles can have zero, one or several possible compositions.
Planar operad
The planar operad is the set of all the planar tangles (up to isomorphism) with such compositions.
Planar algebra
A planar algebra is a representation of the planar operad; more precisely, it is a family of vector spaces , called -box spaces, on which acts the planar operad, i.e. for any tangle (with one output disk and input disks with and intervals respectively) there is a multilinear map
with according to the shading of the -marked intervals, and these maps (also called partition functions) respect the composition of tangle in such a way that all the diagrams as below commute.
Examples
Planar tangles
The family of vector spaces generated by the planar tangles having intervals on their output disk and a white (or black) -marked interval, admits a planar algebra structure.
Temperley–Lieb
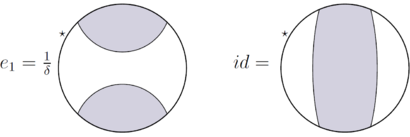
The Temperley-Lieb planar algebra is generated by the planar tangles without input disk; its -box space is generated by
Moreover, a closed string is replaced by a multiplication by .
Note that the dimension of is the Catalan number . This planar algebra encodes the notion of Temperley–Lieb algebra.
Hopf algebra
A semisimple and cosemisimple Hopf algebra over an algebraically closed field is encoded in a planar algebra defined by generators and relations, and "corresponds" (up to isomorphism) to a connected, irreducible, spherical, non degenerate planar algebra with non zero modulus and of depth two.
Note that connected means (as for evaluable below), irreducible means , spherical is defined below, and non-degenerate means that the traces (defined below) are non-degenerate.
Subfactor planar algebra
Definition
A subfactor planar algebra is a planar -algebra which is:
- (1) Finite-dimensional:
- (2) Evaluable:
- (3) Spherical:
- (4) Positive: defines an inner product.
Note that by (2) and (3), any closed string (shaded or not) counts for the same constant .
The tangle action deals with the adjoint by:
with the mirror image of and the adjoint of in .
Examples and results
No-ghost theorem: The planar algebra has no ghost (i.e. element with ) if and only if
For as above, let be the null ideal (generated by elements with ). Then the quotient is a subfactor planar algebra, called the Temperley–Lieb-Jones subfactor planar algebra . Any subfactor planar algebra with constant admits as planar subalgebra.
A planar algebra is a subfactor planar algebra if and only if it is the standard invariant of an extremal subfactor of index , with and . A finite depth or irreducible subfactor is extremal ( on ).
There is a subfactor planar algebra encoding any finite group (and more generally, any finite dimensional Hopf -algebra, called Kac algebra), defined by generators and relations. A (finite dimensional) Kac algebra "corresponds" (up to isomorphism) to an irreducible subfactor planar algebra of depth two.
The subfactor planar algebra associated to an inclusion of finite groups, does not always remember the (core-free) inclusion.
A Bisch-Jones subfactor planar algebra (sometimes called Fuss-Catalan) is defined as for but by allowing two colors of string with their own constant and , with as above. It is a planar subalgebra of any subfactor planar algebra with an intermediate such that and .
The first finite depth subfactor planar algebra of index is called the Haagerup subfactor planar algebra. It has index .
The subfactor planar algebras are completely classified for index at most and a bit beyond. This classification was initiated by Uffe Haagerup. It uses (among other things) a listing of possible principal graphs, together with the embedding theorem and the jellyfish algorithm.
A subfactor planar algebra remembers the subfactor (i.e. its standard invariant is complete) if it is amenable. A finite depth hyperfinite subfactor is amenable.
About the non-amenable case: there are unclassifiably many irreducible hyperfinite subfactors of index 6 that all have the same standard invariant.
Fourier transform and biprojections
Let be a finite index subfactor, and the corresponding subfactor planar algebra. Assume that is irreducible (i.e. ). Let be an intermediate subfactor. Let the Jones projection . Note that . Let and .
Note that and .
Let the bijective linear map be the Fourier transform, also called -click (of the outer star) or rotation; and let be the coproduct of and .
Note that the word coproduct is a diminutive of convolution product. It is a binary operation.
The coproduct satisfies the equality
For any positive operators , the coproduct is also positive; this can be seen diagrammatically:
Let be the contragredient (also called rotation). The map corresponds to four -clicks of the outer star, so it's the identity map, and then .
In the Kac algebra case, the contragredient is exactly the antipode, which, for a finite group, correspond to the inverse.
A biprojection is a projection with a multiple of a projection. Note that and are biprojections; this can be seen as follows:
A projection is a biprojection iff it is the Jones projection of an intermediate subfactor , iff .
Galois correspondence: in the Kac algebra case, the biprojections are 1-1 with the left coideal subalgebras, which, for a finite group, correspond to the subgroups.
For any irreducible subfactor planar algebra, the set of biprojections is a finite lattice, of the form , as for an interval of finite groups .
Using the biprojections, we can make the intermediate subfactor planar algebras.
The uncertainty principle extends to any irreducible subfactor planar algebra :
Let with the range projection of and the unnormalized trace (i.e. on ).
Noncommutative uncertainty principle: Let , nonzero. Then
Assuming and positive, the equality holds if and only if is a biprojection. More generally, the equality holds if and only if is the bi-shift of a biprojection.
References
- ^ Vaughan F. R. Jones (1999), "Planar algebras, I", arXiv:math/9909027
- "Dror Bar-Natan: Publications: Cobordisms". Math.toronto.edu. arXiv:math/0410495. doi:10.2140/gt.2005.9.1443. Retrieved 2016-11-20.
- Bar-Natan, Dror (2005). "Khovanov's homology for tangles and cobordisms". Geometry & Topology. 9 (3): 1443–1499. arXiv:math/0410495. doi:10.2140/gt.2005.9.1443. S2CID 1247623.
- Vaughan F. R. Jones (2017), "Some unitary representations of Thompson's groups F and T", J. Comb. Algebra, 1 (1): 1–44, arXiv:1412.7740, doi:10.4171/JCA/1-1-1, MR 3589908, S2CID 119631229
- Vijay Kodiyalam; V.S. Sunder (2004), "On Jones' planar algebras", J. Knot Theory Ramifications, 13 (2): 219–247, doi:10.1142/S021821650400310X, MR 2047470
- "Vijay Kodiyalam - Planar algebras - IMSc 2015". youtube.com. 2015-11-14.
- Vijay Kodiyalam; V.S. Sunder (2006), "The planar algebra of a semisimple and cosemisimple Hopf algebra", Proc. Indian Acad. Sci. Math. Sci., 116 (4): 1–16, arXiv:math/0506153, Bibcode:2005math......6153K
- Sorin Popa (1995), "An axiomatization of the lattice of higher relative commutants of a subfactor", Inventiones Mathematicae, 120 (3): 427–445, Bibcode:1995InMat.120..427P, doi:10.1007/BF01241137, MR 1334479, S2CID 1740471
- Alice Guionnet; Vaughan F. R. Jones; Dimitri Shlyakhtenko (2010), "Random matrices, free probability, planar algebras and subfactors", Clay Math. Proc., {11}: 201–239, MR 2732052
- Vijay Kodiyalam; V.S. Sunder (2009), "From subfactor planar algebras to subfactors", Internat. J. Math., 20 (10): 1207–1231, arXiv:0807.3704, doi:10.1142/S0129167X0900573X, MR 2574313, S2CID 115161031
- Paramita Das; Vijay Kodiyalam (2005), "Planar algebras and the Ocneanu-Szymanski theorem", Proc. Amer. Math. Soc., 133 (9): 2751–2759, doi:10.1090/S0002-9939-05-07789-0, ISSN 0002-9939, MR 2146224
- ^ Vijay Kodiyalam; Zeph Landau; V.S. Sunder (2003), "The planar algebra associated to a Kac algebra", Proc. Indian Acad. Sci. Math. Sci., 113 (1): 15–51, doi:10.1007/BF02829677, ISSN 0253-4142, MR 1971553, S2CID 56571515
- Ved Prakash Gupta (2008), "Planar algebra of the subgroup-subfactor", Proceedings Mathematical Sciences, 118 (4): 583–612, arXiv:0806.1791, Bibcode:2008arXiv0806.1791G, doi:10.1007/s12044-008-0046-0, S2CID 5589336
- Vijay Kodiyalam; V.S. Sunder (2000), "The subgroup-subfactor", Math. Scand., 86 (1): 45–74, doi:10.7146/math.scand.a-14281, ISSN 0025-5521, MR 1738515
- Masaki Izumi (2002), "Characterization of isomorphic group-subgroup subfactors", Int. Math. Res. Not., 2002 (34): 1791–1803, doi:10.1155/S107379280220402X, ISSN 1073-7928, MR 1920326
- Dietmar Bisch; Vaughan Jones (1997), "Algebras associated to intermediate subfactors", Inventiones Mathematicae, 128 (1): 89–157, Bibcode:1997InMat.128...89J, doi:10.1007/s002220050137, S2CID 119372640
- Pinhas Grossman; Vaughan Jones (2007), "Intermediate subfactors with no extra structure", J. Amer. Math. Soc., 20 (1): 219–265, Bibcode:2007JAMS...20..219G, doi:10.1090/S0894-0347-06-00531-5, MR 2257402
- Emily Peters (2010), "A planar algebra construction of the Haagerup subfactor", Internat. J. Math., 21 (8): 987–1045, arXiv:0902.1294, doi:10.1142/S0129167X10006380, MR 2679382, S2CID 951475
- Vaughan F. R. Jones; Scott Morrison; Noah Snyder (2014), "The classification of subfactors of index at most ", Bull. Amer. Math. Soc. (N.S.), 51 (2): 277–327, arXiv:1304.6141, doi:10.1090/S0273-0979-2013-01442-3, MR 3166042, S2CID 29962597
- Narjess Afzaly; Scott Morrison; David Penneys (2015), The classification of subfactors with index at most , pp. 70pp, arXiv:1509.00038, Bibcode:2015arXiv150900038A
- Uffe Haagerup (1994), "Principal graphs of subfactors in the index range ", Subfactors (Kyuzeso, 1993): 1–38, MR 1317352
- Vaughan Jones; David Penneys (2011), "The embedding theorem for finite depth subfactor planar algebras.", Quantum Topol., 2 (3): 301–337, arXiv:1007.3173, doi:10.4171/QT/23, MR 2812459, S2CID 59578009
- Stephen Bigelow; David Penneys (2014), "Principal graph stability and the jellyfish algorithm.", Math. Ann., 358 (1–2): 1–24, arXiv:1208.1564, doi:10.1007/s00208-013-0941-2, MR 3157990, S2CID 3549669
- Popa, Sorin (1994), "Classification of amenable subfactors of type II", Acta Mathematica, 172 (2): 163–255, doi:10.1007/BF02392646, MR 1278111
- Arnaud Brothier; Stefaan Vaes (2015), "Families of hyperfinite subfactors with the same standard invariant and prescribed fundamental group.", J. Noncommut. Geom., 9 (3): 775–796, arXiv:1309.5354, doi:10.4171/JNCG/207, MR 3420531, S2CID 117853753
- ^ Zhengwei Liu (2016), "Exchange relation planar algebras of small rank", Trans. Amer. Math. Soc., 368 (12): 8303–8348, arXiv:1308.5656, doi:10.1090/tran/6582, ISSN 0002-9947, MR 3551573, S2CID 117030298
- Dietmar Bisch (1994), "A note on intermediate subfactors", Pacific J. Math., 163 (2): 201–216, doi:10.2140/pjm.1994.163.201, ISSN 0030-8730, MR 1262294
- Zeph A. Landau (2002), "Exchange relation planar algebras", Geom. Dedicata, 95: 183–214, doi:10.1023/A:1021296230310, ISSN 0046-5755, MR 1950890, S2CID 119036175
- Masaki Izumi; Roberto Longo; Sorin Popa (1998), "A Galois correspondence for compact groups of automorphisms of von Neumann algebras with a generalization to Kac algebras", J. Funct. Anal., 155 (1): 25–63, arXiv:funct-an/9604004, doi:10.1006/jfan.1997.3228, ISSN 0022-1236, MR 1622812, S2CID 12990106
- Yasuo Watatani (1996), "Lattices of intermediate subfactors", J. Funct. Anal., 140 (2): 312–334, doi:10.1006/jfan.1996.0110, hdl:2115/68899, ISSN 0022-1236, MR 1409040
- Zeph A. Landau (1998), "Intermediate subfactors", Thesis - University of California at Berkeley: 132pp
- Keshab Chandra Bakshi (2016), "Intermediate planar algebra revisited", International Journal of Mathematics, 29 (12): 31pp, arXiv:1611.05811, Bibcode:2016arXiv161105811B, doi:10.1142/S0129167X18500775, S2CID 119305436
- Chunlan Jiang; Zhengwei Liu; Jinsong Wu (2016), "Noncommutative uncertainty principles", J. Funct. Anal., 270 (1): 264–311, arXiv:1408.1165, doi:10.1016/j.jfa.2015.08.007, S2CID 16295570
 , intervals per disk and one
, intervals per disk and one  -marked interval per disk.
-marked interval per disk.


 , called
, called  -box spaces, on which acts the planar operad, i.e. for any tangle
-box spaces, on which acts the planar operad, i.e. for any tangle  (with one output disk and
(with one output disk and  input disks with
input disks with  and
and  intervals respectively) there is a multilinear map
intervals respectively) there is a multilinear map

 according to the shading of the
according to the shading of the 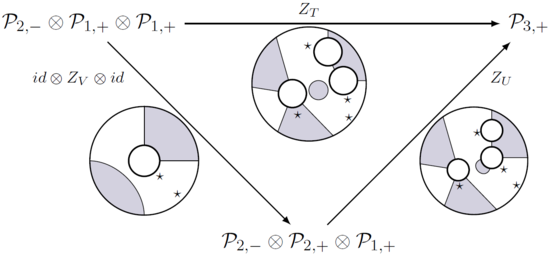
 generated by the planar tangles having
generated by the planar tangles having  is generated by the planar tangles without input disk; its
is generated by the planar tangles without input disk; its  -box space
-box space  is generated by
is generated by

 .
.

 is the
is the  .
This planar algebra encodes the notion of
.
This planar algebra encodes the notion of  (as for evaluable below), irreducible means
(as for evaluable below), irreducible means  , spherical is defined below, and non-degenerate means that the traces (defined below) are non-degenerate.
, spherical is defined below, and non-degenerate means that the traces (defined below) are non-degenerate.



 defines an inner product.
defines an inner product.

 the mirror image of
the mirror image of  the adjoint of
the adjoint of  in
in  .
.
 with
with  ) if and only if
) if and only if

 be the null ideal (generated by elements
be the null ideal (generated by elements  ). Then the quotient
). Then the quotient  is a subfactor planar algebra, called the Temperley–Lieb-Jones subfactor planar algebra
is a subfactor planar algebra, called the Temperley–Lieb-Jones subfactor planar algebra  . Any subfactor planar algebra with constant
. Any subfactor planar algebra with constant  is a subfactor planar algebra if and only if it is the
is a subfactor planar algebra if and only if it is the  of index
of index  , with
, with  and
and  .
A finite depth or irreducible
.
A finite depth or irreducible  on
on  ).
).
 -algebra
-algebra (sometimes called Fuss-Catalan) is defined as for
(sometimes called Fuss-Catalan) is defined as for  and
and  , with
, with  as above. It is a planar subalgebra of any subfactor planar algebra with an intermediate such that
as above. It is a planar subalgebra of any subfactor planar algebra with an intermediate such that  and
and  .
.
 is called the
is called the  .
.
 and a bit beyond.
This classification was initiated by
and a bit beyond.
This classification was initiated by  be a finite index subfactor, and
be a finite index subfactor, and  the corresponding subfactor planar algebra. Assume that
the corresponding subfactor planar algebra. Assume that  ). Let
). Let  be an intermediate subfactor. Let the Jones projection
be an intermediate subfactor. Let the Jones projection  . Note that
. Note that  . Let
. Let  and
and  .
.
 and
and  .
.
 be the Fourier transform, also called
be the Fourier transform, also called  -click (of the outer star) or
-click (of the outer star) or  rotation; and let
rotation; and let  be the coproduct of
be the coproduct of  .
.


 , the coproduct
, the coproduct 
 be the contragredient
be the contragredient  rotation). The map
rotation). The map  corresponds to four
corresponds to four  .
.
 with
with  a multiple of a projection.
Note that
a multiple of a projection.
Note that  and
and  are biprojections; this can be seen as follows:
are biprojections; this can be seen as follows:

 of an intermediate subfactor
of an intermediate subfactor  .
.
 , as for an interval of finite groups
, as for an interval of finite groups  .
.
 with
with  the range projection of
the range projection of  and
and  the unnormalized trace (i.e.
the unnormalized trace (i.e.  on
on  ).
).
 , nonzero. Then
, nonzero. Then

 positive, the equality holds if and only if
positive, the equality holds if and only if  , pp. 70pp,
, pp. 70pp,  ", Subfactors (Kyuzeso, 1993): 1–38,
", Subfactors (Kyuzeso, 1993): 1–38,