This article is about a topological function. For an arithmetic function, see Rounding . For a cipher component, see Round (cryptography) .
In topology and in calculus , a round function is a scalar function
M
→
R
{\displaystyle M\to {\mathbb {R} }}
manifold
M
{\displaystyle M}
critical points form one or several connected components , each homeomorphic to the circle
S
1
{\displaystyle S^{1}}
Morse-Bott functions .
The black circle in one of this critical loops. For instance
For example, let
M
{\displaystyle M}
torus . Let
K
=
(
0
,
2
π
)
×
(
0
,
2
π
)
.
{\displaystyle K=(0,2\pi )\times (0,2\pi ).\,}
Then we know that a map
X
:
K
→
R
3
{\displaystyle X\colon K\to {\mathbb {R} }^{3}\,}
given by
X
(
θ
,
ϕ
)
=
(
(
2
+
cos
θ
)
cos
ϕ
,
(
2
+
cos
θ
)
sin
ϕ
,
sin
θ
)
{\displaystyle X(\theta ,\phi )=((2+\cos \theta )\cos \phi ,(2+\cos \theta )\sin \phi ,\sin \theta )\,}
is a parametrization for almost all of
M
{\displaystyle M}
π
3
:
R
3
→
R
{\displaystyle \pi _{3}\colon {\mathbb {R} }^{3}\to {\mathbb {R} }}
G
=
π
3
|
M
:
M
→
R
,
(
θ
,
ϕ
)
↦
sin
θ
{\displaystyle G=\pi _{3}|_{M}\colon M\to {\mathbb {R} },(\theta ,\phi )\mapsto \sin \theta \,}
G
=
G
(
θ
,
ϕ
)
=
sin
θ
{\displaystyle G=G(\theta ,\phi )=\sin \theta }
g
r
a
d
G
(
θ
,
ϕ
)
=
(
∂
G
∂
θ
,
∂
G
∂
ϕ
)
(
θ
,
ϕ
)
=
(
0
,
0
)
,
{\displaystyle {\rm {grad}}\ G(\theta ,\phi )=\left({{\partial }G \over {\partial }\theta },{{\partial }G \over {\partial }\phi }\right)\!\left(\theta ,\phi \right)=(0,0),\,}
this is if and only if
θ
=
π
2
,
3
π
2
{\displaystyle \theta ={\pi \over 2},\ {3\pi \over 2}}
These two values for
θ
{\displaystyle \theta }
X
(
π
/
2
,
ϕ
)
=
(
2
cos
ϕ
,
2
sin
ϕ
,
1
)
{\displaystyle X({\pi /2},\phi )=(2\cos \phi ,2\sin \phi ,1)\,}
X
(
3
π
/
2
,
ϕ
)
=
(
2
cos
ϕ
,
2
sin
ϕ
,
−
1
)
{\displaystyle X({3\pi /2},\phi )=(2\cos \phi ,2\sin \phi ,-1)\,}
which represent two extremal circles over the torus
M
{\displaystyle M}
Observe that the Hessian for this function is
h
e
s
s
(
G
)
=
[
−
sin
θ
0
0
0
]
{\displaystyle {\rm {hess}}(G)={\begin{bmatrix}-\sin \theta &0\\0&0\end{bmatrix}}}
which clearly it reveals itself as rank of
h
e
s
s
(
G
)
{\displaystyle {\rm {hess}}(G)}
Round complexity
Mimicking the L–S category theory one can define the round complexity asking for whether or not exist round functions on manifolds and/or for the minimum number of critical loops.
References
Siersma and Khimshiasvili, On minimal round functions , Preprint 1118, Department of Mathematics, Utrecht University, 1999, pp. 18. Categories :
Text is available under the Creative Commons Attribution-ShareAlike License. Additional terms may apply.
**DISCLAIMER** We are not affiliated with Wikipedia, and Cloudflare.
The information presented on this site is for general informational purposes only and does not constitute medical advice.
You should always have a personal consultation with a healthcare professional before making changes to your diet, medication, or exercise routine.
AI helps with the correspondence in our chat.
We participate in an affiliate program. If you buy something through a link, we may earn a commission 💕
↑
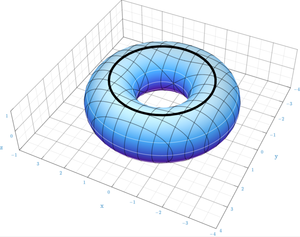
 ,
over a
,
over a  , whose
, whose  , also called critical loops. They are special cases of
, also called critical loops. They are special cases of 


 we get the restriction
we get the restriction

 is a function whose critical sets are determined by
is a function whose critical sets are determined by

 .
.
 give the critical sets
give the critical sets



 equal to one
at the tagged circles, making the critical point degenerate, that is, showing that the critical points are not isolated.
equal to one
at the tagged circles, making the critical point degenerate, that is, showing that the critical points are not isolated.