

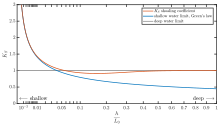
Quantities have been made dimensionless using the gravitational acceleration g and period T, with the deep-water wavelength given by L0 = gT/(2π) and the deep-water phase speed c0 = L0/T. The grey line corresponds with the shallow-water limit cp =cg = √(gh).
The phase speed – and thus also the wavelength L = cpT – decreases monotonically with decreasing depth. However, the group velocity first increases by 20% with respect to its deep-water value (of cg = 1/2c0 = gT/(4π)) before decreasing in shallower depths.
In fluid dynamics, wave shoaling is the effect by which surface waves, entering shallower water, change in wave height. It is caused by the fact that the group velocity, which is also the wave-energy transport velocity, decreases with water depth. Under stationary conditions, a decrease in transport speed must be compensated by an increase in energy density in order to maintain a constant energy flux. Shoaling waves will also exhibit a reduction in wavelength while the frequency remains constant.
In other words, as the waves approach the shore and the water gets shallower, the waves get taller, slow down, and get closer together.
In shallow water and parallel depth contours, non-breaking waves will increase in wave height as the wave packet enters shallower water. This is particularly evident for tsunamis as they wax in height when approaching a coastline, with devastating results.
Overview
Waves nearing the coast experience changes in wave height through different effects. Some of the important wave processes are refraction, diffraction, reflection, wave breaking, wave–current interaction, friction, wave growth due to the wind, and wave shoaling. In the absence of the other effects, wave shoaling is the change of wave height that occurs solely due to changes in mean water depth – without alterations in wave propagation direction or energy dissipation. Pure wave shoaling occurs for long-crested waves propagating perpendicular to the parallel depth contour lines of a mildly sloping sea-bed. Then the wave height at a certain location can be expressed as:
with the shoaling coefficient and the wave height in deep water. The shoaling coefficient depends on the local water depth and the wave frequency (or equivalently on and the wave period ). Deep water means that the waves are (hardly) affected by the sea bed, which occurs when the depth is larger than about half the deep-water wavelength
Physics


For non-breaking waves, the energy flux associated with the wave motion, which is the product of the wave energy density with the group velocity, between two wave rays is a conserved quantity (i.e. a constant when following the energy of a wave packet from one location to another). Under stationary conditions the total energy transport must be constant along the wave ray – as first shown by William Burnside in 1915. For waves affected by refraction and shoaling (i.e. within the geometric optics approximation), the rate of change of the wave energy transport is:
where is the co-ordinate along the wave ray and is the energy flux per unit crest length. A decrease in group speed and distance between the wave rays must be compensated by an increase in energy density . This can be formulated as a shoaling coefficient relative to the wave height in deep water.
For shallow water, when the wavelength is much larger than the water depth – in case of a constant ray distance (i.e. perpendicular wave incidence on a coast with parallel depth contours) – wave shoaling satisfies Green's law:
with the mean water depth, the wave height and the fourth root of
Water wave refraction
Following Phillips (1977) and Mei (1989), denote the phase of a wave ray as
- .
The local wave number vector is the gradient of the phase function,
- ,
and the angular frequency is proportional to its local rate of change,
- .
Simplifying to one dimension and cross-differentiating it is now easily seen that the above definitions indicate simply that the rate of change of wavenumber is balanced by the convergence of the frequency along a ray;
- .
Assuming stationary conditions (), this implies that wave crests are conserved and the frequency must remain constant along a wave ray as . As waves enter shallower waters, the decrease in group velocity caused by the reduction in water depth leads to a reduction in wave length because the nondispersive shallow water limit of the dispersion relation for the wave phase speed,
dictates that
- ,
i.e., a steady increase in k (decrease in ) as the phase speed decreases under constant .
See also
- Airy wave theory – Fluid dynamics theory on the propagation of gravity waves
- Breaking wave – Wave that becomes unstable as a consequence of excessive steepness
- Dispersion (water waves) – Dispersion of waves on a water surface
- Ocean surface waves – Surface waves generated by wind on open waterPages displaying short descriptions of redirect targets
- Shallow water equations – Set of partial differential equations that describe the flow below a pressure surface in a fluid
- Shoal – Natural submerged sandbank that rises from a body of water to near the surface
- Waves and shallow water – Effect of shallow water on a surface gravity wave
- Wave height – Difference between the elevations of a crest and a neighbouring trough
- Ursell number – Dimensionless number indicating the nonlinearity of long surface gravity waves on a fluid layer.
Notes
- Wiegel, R.L. (2013). Oceanographical Engineering. Dover Publications. p. 17, Figure 2.4. ISBN 978-0-486-16019-1.
- Longuet-Higgins, M.S.; Stewart, R.W. (1964). "Radiation stresses in water waves; a physical discussion, with applications" (PDF). Deep-Sea Research and Oceanographic Abstracts. 11 (4): 529–562. Bibcode:1964DSRA...11..529L. doi:10.1016/0011-7471(64)90001-4. Archived from the original (PDF) on 2010-06-12. Retrieved 2010-03-25.
- WMO (1998). Guide to Wave Analysis and Forecasting (PDF). Vol. 702 (2 ed.). World Meteorological Organization. ISBN 92-63-12702-6.
- ^ Goda, Y. (2010). Random Seas and Design of Maritime Structures. Advanced Series on Ocean Engineering. Vol. 33 (3 ed.). Singapore: World Scientific. pp. 10–13 & 99–102. ISBN 978-981-4282-39-0.
- ^ Dean, R.G.; Dalrymple, R.A. (1991). Water wave mechanics for engineers and scientists. Advanced Series on Ocean Engineering. Vol. 2. Singapore: World Scientific. ISBN 978-981-02-0420-4.
- Burnside, W. (1915). "On the modification of a train of waves as it advances into shallow water". Proceedings of the London Mathematical Society. Series 2. 14: 131–133. doi:10.1112/plms/s2_14.1.131.
- Phillips, Owen M. (1977). The dynamics of the upper ocean (2nd ed.). Cambridge University Press. ISBN 0-521-29801-6.
- Mei, Chiang C. (1989). The Applied Dynamics of Ocean Surface Waves. Singapore: World Scientific. ISBN 9971-5-0773-0.
 at a certain location can be expressed as:
at a certain location can be expressed as:

 the shoaling coefficient and
the shoaling coefficient and  the wave height in deep water. The shoaling coefficient
the wave height in deep water. The shoaling coefficient  and the wave
and the wave  (or equivalently on
(or equivalently on  ). Deep water means that the waves are (hardly) affected by the sea bed, which occurs when the depth
). Deep water means that the waves are (hardly) affected by the sea bed, which occurs when the depth 
 ) at
) at  describing the effect of wave shoaling on the
describing the effect of wave shoaling on the  is the wavelength in deep water:
is the wavelength in deep water:  with
with  the
the  the
the 

 is the co-ordinate along the wave ray and
is the co-ordinate along the wave ray and  is the energy flux per unit crest length. A decrease in group speed
is the energy flux per unit crest length. A decrease in group speed  and distance between the wave rays
and distance between the wave rays  . This can be formulated as a shoaling coefficient relative to the wave height in deep water.
. This can be formulated as a shoaling coefficient relative to the wave height in deep water.

 the
the 
 .
. ,
, .
. .
. ), this implies that wave crests are conserved and the
), this implies that wave crests are conserved and the  .
As waves enter shallower waters, the decrease in
.
As waves enter shallower waters, the decrease in  because the nondispersive
because the nondispersive 
 ,
, ) as the
) as the  .
.



