| Revision as of 03:44, 1 November 2001 view sourceBryan Derksen (talk | contribs)Extended confirmed users95,333 edits sometimes the simple stuff is quite useful← Previous edit | Latest revision as of 21:24, 23 August 2024 view source Seraphimblade (talk | contribs)Edit filter managers, Administrators46,272 edits →Constant acceleration: rm editorial | ||
| Line 1: | Line 1: | ||
| {{Short description|Speed and direction of a motion}} | |||
| {{About|velocity in physics}} | |||
| {{pp-semi-indef|small=yes}} | |||
| {{Infobox physical quantity | |||
| |name = Velocity | |||
| |image = US Navy 040501-N-1336S-037 The U.S. Navy sponsored Chevy Monte Carlo NASCAR leads a pack into turn four at California Speedway.jpg | |||
| |image_size = 260px | |||
| |caption = As a change of direction occurs while the racing cars turn on the curved track, their velocity is not constant even if their speed is. | |||
| |symbols = {{math|''v''}}, {{math|'''v'''}}, {{vec|{{math|''v''}}}}, {{math|'''''v'''''}} | |||
| |otherunits = ], ] | |||
| |baseunits = ]/] | |||
| |dimension = '''L''' '''T'''<sup>−1</sup> | |||
| }} | |||
| {{Classical mechanics|cTopic=Fundamental concepts}} | |||
| '''Velocity''' is the ] in combination with the direction of ] of an ]. Velocity is a fundamental concept in ], the branch of ] that describes the motion of bodies. | |||
| Velocity is a physical ] ]: both magnitude and direction are needed to define it. The ] ] (]) of velocity is called {{em|speed}}, being a coherent derived unit whose quantity is measured in the ] (]) as ] (m/s or m⋅s<sup>−1</sup>). For example, "5 metres per second" is a scalar, whereas "5 metres per second east" is a vector. If there is a change in speed, direction or both, then the object is said to be undergoing an '']''. | |||
| == Definition == | |||
| In ] (that is, when the velocities involved are significantly less than the ]) the average velocity of an object moving a distance ''d'' during a time interval ''t'' is described by the simple formula: | |||
| === Average velocity === | |||
| The '''average velocity''' of an object over a period of time is its ], <math>\Delta s</math>, divided by the duration of the period, <math>\Delta t</math>, given mathematically as<ref>{{Cite web |title=The Feynman Lectures on Physics Vol. I Ch. 8: Motion |url=https://www.feynmanlectures.caltech.edu/I_08.html |access-date=2024-01-05 |website=www.feynmanlectures.caltech.edu}}</ref><math display="block">\bar{v}=\frac{\Delta s}{\Delta t}.</math> | |||
| ===Instantaneous velocity=== | |||
| ] lines represent the values for acceleration at different points along the curve) and displacement '''''s''''' (the yellow ] under the curve.)]] | |||
| The '''instantaneous''' '''velocity''' of an object is the limit average velocity as the time interval approaches zero. At any particular time {{math|''t''}}, it can be calculated as the ] of the position with respect to time:<ref>{{cite book |author1=David Halliday |url=https://books.google.com/books?id=s5dDEAAAQBAJ |title=Fundamentals of Physics, Extended |author2=Robert Resnick |author3=Jearl Walker |publisher=John Wiley & Sons |year=2021 |isbn=978-1-119-77351-1 |edition=12th |page=71}} </ref> | |||
| <math display="block">\boldsymbol{v} = \lim_{{\Delta t}\to 0} \frac{\Delta \boldsymbol{s}}{\Delta t} = \frac{d\boldsymbol{s}}{dt} .</math> | |||
| From this derivative equation, in the one-dimensional case it can be seen that the area under a velocity vs. time ({{math|'''''v'''''}} vs. {{math|''t''}} graph) is the displacement, {{math|'''''s'''''}}. In calculus terms, the ] of the velocity function {{math|'''''v'''''(''t'')}} is the displacement function {{math|'''''s'''''(''t'')}}. In the figure, this corresponds to the yellow area under the curve. | |||
| v = d/t. | |||
| <math display="block" qid="Q190291"> \boldsymbol{s} = \int \boldsymbol{v} \ dt .</math> | |||
| Although the concept of an instantaneous velocity might at first seem counter-intuitive, it may be thought of as the velocity that the object would continue to travel at if it stopped accelerating at that moment. | |||
| === Difference between speed and velocity === | |||
| {{main|Speed}} | |||
| ] | |||
| While the terms ''speed'' and ''velocity'' are often colloquially used interchangeably to connote how fast an object is moving, in scientific terms they are different. Speed, the ] magnitude of a velocity vector, denotes only how fast an object is moving, while velocity indicates both an object's speed and direction.<ref>{{cite book |author1=Richard P. Olenick |url=https://books.google.com/books?id=xMWwTpn53KsC |title=The Mechanical Universe: Introduction to Mechanics and Heat |author2=Tom M. Apostol |author3=David L. Goodstein |publisher=Cambridge University Press |year=2008 |isbn=978-0-521-71592-8 |edition=illustrated, reprinted |page=84}} </ref><ref>{{cite book |author1=Michael J. Cardamone |url=https://books.google.com/books?id=LRsCK8nVueIC |title=Fundamental Concepts of Physics |publisher=Universal-Publishers |year=2007 |isbn=978-1-59942-433-0 |edition= |page=5}} </ref><ref>{{cite book |author1=Jerry D. Wilson |url=https://books.google.com/books?id=pJ9hEAAAQBAJ |title=College Physics Essentials, Eighth Edition (Two-Volume Set) |author2=Anthony J. Buffa |author3=Bo Lou |publisher=CRC Press |year=2022 |isbn=978-1-351-12991-6 |edition=illustrated |page=40}} </ref> | |||
| To have a ''constant velocity'', an object must have a constant speed in a constant direction. Constant direction constrains the object to motion in a straight path thus, a constant velocity means motion in a straight line at a constant speed. | |||
| ] is the change of an object's velocity over time. It is defined as: | |||
| <!-- Could discuss the basic equations of motion of a body moving at constant velocity here. --> | |||
| For example, a car moving at a constant 20 kilometres per hour in a circular path has a constant speed, but does not have a constant velocity because its direction changes. Hence, the car is considered to be undergoing an acceleration. | |||
| === Units === | |||
| Since the derivative of the position with respect to time gives the change in position (in ]s) divided by the change in time (in ]s), velocity is measured in ] (m/s). | |||
| ==Equation of motion== | |||
| a = (v<sub><i>f</i></sub> - v<sub><i>i</i></sub>)/t | |||
| {{main|Equation of motion}} | |||
| ===Average velocity=== | |||
| Velocity is defined as the rate of change of position with respect to time, which may also be referred to as the ''instantaneous velocity'' to emphasize the distinction from the average velocity. In some applications the average velocity of an object might be needed, that is to say, the constant velocity that would provide the same resultant displacement as a variable velocity in the same time interval, {{math|'''v'''(''t'')}}, over some time period {{math|Δ''t''}}. Average velocity can be calculated as:<ref>{{cite book |title=Fundamentals of Physics, Extended |author1=David Halliday |author2=Robert Resnick |author3=Jearl Walker |edition=12th |publisher=John Wiley & Sons |year=2021 |isbn=978-1-119-77351-1 |page=70 |url=https://books.google.com/books?id=s5dDEAAAQBAJ}} </ref><ref>{{cite book |title=The Calculus Lifesaver: All the Tools You Need to Excel at Calculus |author1=Adrian Banner |edition=illustrated |publisher=Princeton University Press |year=2007 |isbn=978-0-691-13088-0 |page=350 |url=https://books.google.com/books?id=OrumDwAAQBAJ}} </ref> | |||
| :<math display="block" qid="Q11465">\mathbf{\bar{v}} = \frac{\Delta\mathbf{x}}{\Delta t} = \frac{\int_{t_0}^{t_1}\mathbf{v}(t)dt}{t_1-t_0}. </math> | |||
| The average velocity is always less than or equal to the average speed of an object. This can be seen by realizing that while distance is always strictly increasing, displacement can increase or decrease in magnitude as well as change direction. | |||
| In terms of a displacement-time ({{math|''x''}} vs. {{math|''t''}}) graph, the instantaneous velocity (or, simply, velocity) can be thought of as the ], and the average velocity as the slope of the ] between two points with {{math|''t''}} coordinates equal to the boundaries of the time period for the average velocity. | |||
| The final velocity of an object after accelerating at acceleration ''a'' for a period of time ''t'' is: | |||
| ====Special cases==== | |||
| *When a particle moves with different uniform speeds ''v''<sub>1</sub>, ''v''<sub>2</sub>, ''v''<sub>3</sub>, ..., ''v''<sub>''n''</sub> in different time intervals ''t''<sub>1</sub>, ''t''<sub>2</sub>, ''t''<sub>3</sub>, ..., ''t''<sub>''n''</sub> respectively, then ] over the total time of journey is given as <math display="block"> \bar{v} = {v_1 t_1+ v_2 t_2+ v_3 t_3+ \dots+ v_n t_n\over t_1+ t_2+ t_3+ \dots + t_n} </math> | |||
| If {{math|1=''t''<sub>1</sub> = ''t''<sub>2</sub> = ''t''<sub>3</sub> = ... = ''t''}}, then average speed is given by the ] of the speeds <math display="block">\bar{v} ={v_1+v_2+v_3+\dots+v_n\over n} =\frac{1}{n}\sum_{i=1}^n{v_i}</math> | |||
| *When a particle moves different distances ''s''<sub>1</sub>, ''s''<sub>2</sub>, ''s''<sub>3</sub>,..., ''s''<sub>''n''</sub> with speeds ''v''<sub>1</sub>, ''v''<sub>2</sub>, ''v''<sub>3</sub>,..., ''v''<sub>''n''</sub> respectively, then the average speed of the particle over the total distance is given as<ref name="giba">{{cite book |title=Statistical Tools and Technique |author1=Giri & Bannerjee |edition= |publisher=Academic Publishers |year=2002 |isbn=978-81-87504-39-9 |page=4 |url=https://books.google.com/books?id=jmg_oXMGsugC}} </ref> | |||
| <math display="block">\bar{v} ={s_1+s_2+s_3+\dots+s_n\over t_1+t_2+t_3+\dots+t_n}={{s_1+s_2+s_3+\dots+s_n}\over {{s_1\over v_1}+{s_2\over v_2}+ {s_3\over v_3}+\dots+{s_n\over v_n}}}</math> | |||
| If {{math|1=''s''<sub>1</sub> = ''s''<sub>2</sub> = ''s''<sub>3</sub> = ... = ''s''}}, then average speed is given by the ] of the speeds<ref name="giba" /> | |||
| <math display="block">\bar{v} = n\left({1\over v_1} + {1\over v_2} + {1\over v_3} + \dots + {1\over v_n}\right)^{-1} =n\left(\sum_{i=1}^n\frac{1}{v_i}\right)^{-1}.</math> | |||
| ===Relationship to acceleration=== | |||
| Although velocity is defined as the rate of change of position, it is often common to start with an expression for an object's ]. As seen by the three green tangent lines in the figure, an object's instantaneous acceleration at a ] is the ] of the ] to the curve of a {{math|'''''v'''''(''t'')}} graph at that point. In other words, instantaneous acceleration is defined as the derivative of velocity with respect to time:<ref>{{cite book |title=Classical Physics: A Two-Semester Coursebook |author1=Bekir Karaoglu |edition= |publisher=Springer Nature |year=2020 |isbn=978-3-030-38456-2 |page=41 |url=https://books.google.com/books?id=1ZHTDwAAQBAJ}} </ref> | |||
| v<sub><i>f</i></sub> = v<sub><i>i</i></sub> + at | |||
| <math display="block" qid=Q11376> \boldsymbol{a} = \frac{d\boldsymbol{v}}{dt} .</math> | |||
| From there, velocity is expressed as the area under an {{math|'''''a'''''(''t'')}} acceleration vs. time graph. As above, this is done using the concept of the integral: | |||
| <math display="block">\boldsymbol{v} = \int \boldsymbol{a} \ dt .</math> | |||
| ====Constant acceleration==== | |||
| The average velocity of an accelerating object is (v<sub><i>f</i></sub> + v<sub><i>i</i></sub>)/2. To find the displacement of an accelerating objuect during a time interval, substitute this expression into the first formula to get: | |||
| In the special case of constant acceleration, velocity can be studied using the ]. By considering '''a''' as being equal to some arbitrary constant vector, this shows | |||
| <math display="block">\boldsymbol{v} = \boldsymbol{u} + \boldsymbol{a}t</math> | |||
| with {{math|'''''v'''''}} as the velocity at time {{math|''t''}} and {{math|'''''u'''''}} as the velocity at time {{math|1=''t'' = 0}}. By combining this equation with the suvat equation {{math|1='''''x''''' = '''''u'''t'' + '''''a'''t''<sup>2</sup>/2}}, it is possible to relate the displacement and the average velocity by | |||
| <math display="block">\boldsymbol{x} = \frac{(\boldsymbol{u} + \boldsymbol{v})}{2} t = \boldsymbol{\bar{v}}t.</math> | |||
| It is also possible to derive an expression for the velocity independent of time, known as the ], as follows: | |||
| <math display="block">v^{2} = \boldsymbol{v}\cdot\boldsymbol{v} = (\boldsymbol{u}+\boldsymbol{a}t) \cdot (\boldsymbol{u}+\boldsymbol{a}t) = u^{2} + 2t(\boldsymbol{a}\cdot\boldsymbol{u})+a^{2}t^{2}</math> | |||
| <math display="block">(2\boldsymbol{a})\cdot\boldsymbol{x} = (2\boldsymbol{a})\cdot(\boldsymbol{u}t + \tfrac{1}{2} \boldsymbol{a} t^2) = 2t (\boldsymbol{a} \cdot \boldsymbol{u}) + a^2 t^2 = v^{2} - u^{2}</math> | |||
| <math display="block">\therefore v^2 = u^2 + 2(\boldsymbol{a}\cdot\boldsymbol{x})</math> | |||
| where {{math|1=''v'' = {{abs|'''''v'''''}}}} etc. | |||
| The above equations are valid for both ] and ]. Where Newtonian mechanics and special relativity differ is in how different observers would describe the same situation. In particular, in Newtonian mechanics, all observers agree on the value of t and the transformation rules for position create a situation in which all non-accelerating observers would describe the acceleration of an object with the same values. Neither is true for special relativity. In other words, only relative velocity can be calculated. | |||
| == Quantities that are dependent on velocity == | |||
| === Momentum === | |||
| d = t(v<sub><i>f</i></sub> + v<sub><i>i</i></sub>)/2 | |||
| In classical mechanics, ] defines ], p, as a vector that is the product of an object's mass and velocity, given mathematically as<math display="block" qid="Q41273">\boldsymbol{p} = m\boldsymbol{v}</math>where ''m'' is the mass of the object. | |||
| === Kinetic energy === | |||
| The ] of a moving object is dependent on its velocity and is given by the equation<ref>{{cite book |title=Fundamentals of Physics, Chapters 33-37 |author1=David Halliday |author2=Robert Resnick |author3=Jearl Walker |edition= |publisher=John Wiley & Sons |year=2010 |isbn=978-0-470-54794-6 |page=1080 |url=https://books.google.com/books?id=aO-Xrlje7hMC}} </ref><math display="block" qid="Q46276"> E_{\text{k}} = \tfrac{1}{2} m v^2 </math>where ''E''<sub>k</sub> is the kinetic energy. Kinetic energy is a scalar quantity as it depends on the square of the velocity. | |||
| When only the object's initial velocity is known, the expression | |||
| === Drag (fluid resistance) === | |||
| In ], ] is a force acting opposite to the relative motion of any object moving with respect to a surrounding fluid. The drag force, <math>F_D</math>, is dependent on the square of velocity and is given as<math display="block" qid="Q9300786">F_D\, =\, \tfrac12\, \rho\, v^2\, C_D\, A</math>where | |||
| * <math>\rho</math> is the ] of the fluid,<ref>For ], the air density can be found using the ]. It is 1.293 kg/m<sup>3</sup> at 0 °C and 1 ].</ref> | |||
| * <math>v</math> is the speed of the object relative to the fluid, | |||
| * <math>A</math> is the ], and | |||
| * <math>C_D</math> is the ] – a ]. | |||
| === Escape velocity === | |||
| d = v<sub><i>i</i></sub>t + (at<sup>2</sup>)/2 | |||
| ] is the minimum speed a ballistic object needs to escape from a massive body such as Earth. It represents the kinetic energy that, when added to the object's ] (which is always negative), is equal to zero. The general formula for the escape velocity of an object at a distance ''r'' from the center of a planet with mass ''M'' is<ref>{{cite book |author1=Jim Breithaupt |url=https://books.google.com/books?id=r8I1gyNNKnoC |title=New Understanding Physics for Advanced Level |publisher=Nelson Thornes |year=2000 |isbn=978-0-7487-4314-8 |edition=illustrated |page=231}} </ref><math display="block">v_{\text{e}} = \sqrt{\frac{2GM}{r}} = \sqrt{2gr},</math>where ''G'' is the ] and ''g'' is the ]. The escape velocity from Earth's surface is about 11 200 m/s, and is irrespective of the direction of the object. This makes "escape velocity" somewhat of a misnomer, as the more correct term would be "escape speed": any object attaining a velocity of that magnitude, irrespective of atmosphere, will leave the vicinity of the base body as long as it does not intersect with something in its path. | |||
| === The Lorentz factor of special relativity === | |||
| In ], the dimensionless ] appears frequently, and is given by<ref>{{cite book |title=Modern Aspects Of Relativity |author1=Eckehard W Mielke |edition= |publisher=World Scientific |year=2022 |isbn=978-981-12-4406-3 |page=98 |url=https://books.google.com/books?id=HN5kEAAAQBAJ}} </ref><math display="block" qid=Q599404>\gamma = \frac{1}{\sqrt{1-\frac{v^2}{c^2}}}</math>where γ is the Lorentz factor and ''c'' is the speed of light. | |||
| ==Relative velocity== | |||
| can be used. The basic equations for final velocity and displacement can be combined to form an equation that is independent of time: | |||
| {{main|Relative velocity}} | |||
| ''Relative velocity'' is a measurement of velocity between two objects as determined in a single coordinate system. Relative velocity is fundamental in both classical and modern physics, since many systems in physics deal with the relative motion of two or more particles. | |||
| Consider an object A moving with velocity ] '''''v''''' and an object B with velocity vector '''''w'''''; these ''absolute velocities'' are typically expressed in the same ]. Then, the velocity of object A {{em|relative to}} object B is defined as the difference of the two velocity vectors: | |||
| <math display="block">\boldsymbol{v}_{A\text{ relative to }B} = \boldsymbol{v} - \boldsymbol{w}</math> | |||
| Similarly, the relative velocity of object B moving with velocity '''''w''''', relative to object A moving with velocity '''''v''''' is: | |||
| <math display="block">\boldsymbol{v}_{B\text{ relative to }A} = \boldsymbol{w} - \boldsymbol{v}</math> | |||
| Usually, the inertial frame chosen is that in which the latter of the two mentioned objects is in rest. | |||
| In Newtonian mechanics, the relative velocity is independent of the chosen inertial reference frame. This is not the case anymore with ] in which velocities depend on the choice of reference frame. | |||
| ===Scalar velocities=== | |||
| v<sub><i>f</i></sub><sup>2</sup> = v<sub><i>i</i></sub><sup>2</sup> + 2ad | |||
| In the one-dimensional case,<ref></ref> the velocities are scalars and the equation is either: | |||
| <math display="block"> v_\text{rel} = v - (-w),</math> if the two objects are moving in opposite directions, or: | |||
| <math display="block"> v_\text{rel} = v -(+w),</math> if the two objects are moving in the same direction. | |||
| ==Coordinate systems== | |||
| === Cartesian coordinates === | |||
| In multi-dimensional ], velocity is broken up into components that correspond with each dimensional axis of the coordinate system. In a two-dimensional system, where there is an x-axis and a y-axis, corresponding velocity components are defined as<ref>{{Cite web |title=The Feynman Lectures on Physics Vol. I Ch. 9: Newton's Laws of Dynamics |url=https://www.feynmanlectures.caltech.edu/I_09.html |access-date=2024-01-04 |website=www.feynmanlectures.caltech.edu}}</ref> | |||
| <math display="block">v_x=dx/dt,</math> | |||
| These simple equations become more complicated as velocities approach the speed of light, where the effects of ] starts to become significant. | |||
| <math display="block">v_y=dy/dt.</math> | |||
| The two-dimensional velocity vector is then defined as <math>\textbf{v}=<v_x, v_y></math>. The magnitude of this vector represents speed and is found by the ] as | |||
| <math display="block">|v|=\sqrt{v_x^2+v_y^2}.</math> | |||
| In three-dimensional systems where there is an additional z-axis, the corresponding velocity component is defined as | |||
| <math display="block">v_z=dz/dt.</math> | |||
| The three-dimensional velocity vector is defined as <math>\textbf{v}=<v_x, v_y, v_z></math> with its magnitude also representing speed and being determined by | |||
| <math display="block">|v|=\sqrt{v_x^2+v_y^2+v_z^2}.</math> | |||
| While some textbooks use subscript notation to define Cartesian components of velocity, others use <math>u</math>, <math>v</math>, and <math>w</math> for the <math>x</math>-, <math>y</math>-, and <math>z</math>-axes respectively.<ref>White, F. M. (2008). ''Fluid mechanics''. The McGraw Hill Companies,.</ref> | |||
| === Polar coordinates === | |||
| {{see also|Circular_motion#In_polar_coordinates|Radial, transverse, normal}} | |||
| <!-- This section is linked from ] --> | |||
| ], the tangential component causes visible changes of the position of the object.]] | |||
| In ], a two-dimensional velocity is described by a '']'', defined as the component of velocity away from or toward the origin, and a ''transverse velocity'', perpendicular to the radial one.<ref>{{cite book |title=Mechanics, Volume 6 |author1=E. Graham |author2=Aidan Burrows |author3=Brian Gaulter |edition=illustrated |publisher=Heinemann |year=2002 |isbn=978-0-435-51311-5 |page=77 |url=https://books.google.com/books?id=Zovge9bERh8C}} </ref><ref>{{cite book |title=Engineering Mechanics |author1=Anup Goel |author2=H. J. Sawant |edition= |publisher=Technical Publications |year=2021 |isbn=978-93-332-2190-0 |page=8 |url=https://books.google.com/books?id=UjcfEAAAQBAJ}} </ref> Both arise from ], which is the rate of rotation about the origin (with positive quantities representing counter-clockwise rotation and negative quantities representing clockwise rotation, in a right-handed coordinate system). | |||
| The radial and traverse velocities can be derived from the Cartesian velocity and displacement vectors by decomposing the velocity vector into radial and transverse components. The ] velocity is the component of velocity along a circle centered at the origin. | |||
| <math display="block">\boldsymbol{v}=\boldsymbol{v}_T+\boldsymbol{v}_R</math> | |||
| where | |||
| *<math>\boldsymbol{v}_T</math> is the transverse velocity | |||
| *<math>\boldsymbol{v}_R</math> is the radial velocity. | |||
| The ''radial speed'' (or magnitude of the radial velocity) is the ] of the velocity vector and the unit vector in the radial direction. | |||
| <math display="block">v_R = \frac{\boldsymbol{v} \cdot \boldsymbol{r}}{\left|\boldsymbol{r}\right|} = \boldsymbol{v} \cdot \hat{\boldsymbol{r}}</math> | |||
| where <math>\boldsymbol{r}</math> is position and <math>\hat{\boldsymbol{r}}</math> is the radial direction. | |||
| The transverse speed (or magnitude of the transverse velocity) is the magnitude of the ] of the unit vector in the radial direction and the velocity vector. It is also the dot product of velocity and transverse direction, or the product of the ] <math>\omega</math> and the radius (the magnitude of the position). | |||
| <math display="block">v_T=\frac{|\boldsymbol{r}\times\boldsymbol{v}|}{|\boldsymbol{r}|}= \boldsymbol{v} \cdot \hat{\boldsymbol{t}}=\omega|\boldsymbol{r}|</math> | |||
| such that | |||
| <math display="block">\omega=\frac{|\boldsymbol{r}\times\boldsymbol{v}|}{|\boldsymbol{r}|^2}.</math> | |||
| ] in scalar form is the mass times the distance to the origin times the transverse velocity, or equivalently, the mass times the distance squared times the angular speed. The sign convention for angular momentum is the same as that for angular velocity. | |||
| <math display="block">L = mrv_T = mr^2\omega</math> | |||
| where | |||
| *<math>m</math> is mass | |||
| *<math>r=|\boldsymbol{r}|.</math> | |||
| The expression <math>mr^2</math> is known as ]. | |||
| If forces are in the radial direction only with an inverse square dependence, as in the case of a gravitational ], angular momentum is constant, and transverse speed is inversely proportional to the distance, angular speed is inversely proportional to the distance squared, and the rate at which area is swept out is constant. These relations are known as ]. | |||
| ==See also== | |||
| {{cmn|colwidth=30em| | |||
| * ] (relativistic version of velocity for ]) | |||
| * ] | |||
| * ] | |||
| * ] | |||
| * ] (in relativity, using traveler time instead of observer time) | |||
| * ] (a version of velocity additive at relativistic speeds) | |||
| * ] | |||
| * ] | |||
| * ] | |||
| }} | |||
| ==Notes== | |||
| * Robert Resnick and Jearl Walker, ''Fundamentals of Physics'', Wiley; 7 Sub edition (June 16, 2004). {{isbn|0-471-23231-9}}. | |||
| ==References== | |||
| {{Reflist|30em}} | |||
| ==External links== | |||
| {{Commons category}} | |||
| * | |||
| * (]) | |||
| {{Kinematics}} | |||
| {{Classical mechanics derived SI units}} | |||
| {{Authority control}} | |||
| ] | |||
| ] | |||
| ] | |||
| ] | |||
Latest revision as of 21:24, 23 August 2024
Speed and direction of a motion This article is about velocity in physics. For other uses, see Velocity (disambiguation).
| Velocity | |
|---|---|
 As a change of direction occurs while the racing cars turn on the curved track, their velocity is not constant even if their speed is. As a change of direction occurs while the racing cars turn on the curved track, their velocity is not constant even if their speed is. | |
| Common symbols | v, v, v→, v |
| Other units | mph, ft/s |
| In SI base units | m/s |
| Dimension | L T |
| Part of a series on |
| Classical mechanics |
|---|
| Second law of motion |
| Branches |
| Fundamentals |
| Formulations |
| Core topics |
| Rotation |
| Scientists |
Velocity is the speed in combination with the direction of motion of an object. Velocity is a fundamental concept in kinematics, the branch of classical mechanics that describes the motion of bodies.
Velocity is a physical vector quantity: both magnitude and direction are needed to define it. The scalar absolute value (magnitude) of velocity is called speed, being a coherent derived unit whose quantity is measured in the SI (metric system) as metres per second (m/s or m⋅s). For example, "5 metres per second" is a scalar, whereas "5 metres per second east" is a vector. If there is a change in speed, direction or both, then the object is said to be undergoing an acceleration.
Definition
Average velocity
The average velocity of an object over a period of time is its change in position, , divided by the duration of the period, , given mathematically as
Instantaneous velocity
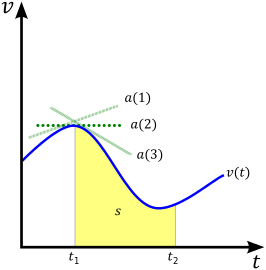
The instantaneous velocity of an object is the limit average velocity as the time interval approaches zero. At any particular time t, it can be calculated as the derivative of the position with respect to time:
From this derivative equation, in the one-dimensional case it can be seen that the area under a velocity vs. time (v vs. t graph) is the displacement, s. In calculus terms, the integral of the velocity function v(t) is the displacement function s(t). In the figure, this corresponds to the yellow area under the curve.
Although the concept of an instantaneous velocity might at first seem counter-intuitive, it may be thought of as the velocity that the object would continue to travel at if it stopped accelerating at that moment.
Difference between speed and velocity
Main article: Speed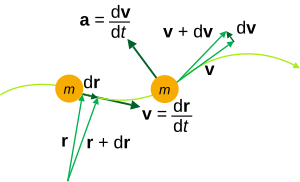
While the terms speed and velocity are often colloquially used interchangeably to connote how fast an object is moving, in scientific terms they are different. Speed, the scalar magnitude of a velocity vector, denotes only how fast an object is moving, while velocity indicates both an object's speed and direction.
To have a constant velocity, an object must have a constant speed in a constant direction. Constant direction constrains the object to motion in a straight path thus, a constant velocity means motion in a straight line at a constant speed.
For example, a car moving at a constant 20 kilometres per hour in a circular path has a constant speed, but does not have a constant velocity because its direction changes. Hence, the car is considered to be undergoing an acceleration.
Units
Since the derivative of the position with respect to time gives the change in position (in metres) divided by the change in time (in seconds), velocity is measured in metres per second (m/s).
Equation of motion
Main article: Equation of motionAverage velocity
Velocity is defined as the rate of change of position with respect to time, which may also be referred to as the instantaneous velocity to emphasize the distinction from the average velocity. In some applications the average velocity of an object might be needed, that is to say, the constant velocity that would provide the same resultant displacement as a variable velocity in the same time interval, v(t), over some time period Δt. Average velocity can be calculated as:
The average velocity is always less than or equal to the average speed of an object. This can be seen by realizing that while distance is always strictly increasing, displacement can increase or decrease in magnitude as well as change direction.
In terms of a displacement-time (x vs. t) graph, the instantaneous velocity (or, simply, velocity) can be thought of as the slope of the tangent line to the curve at any point, and the average velocity as the slope of the secant line between two points with t coordinates equal to the boundaries of the time period for the average velocity.
Special cases
- When a particle moves with different uniform speeds v1, v2, v3, ..., vn in different time intervals t1, t2, t3, ..., tn respectively, then average speed over the total time of journey is given as
If t1 = t2 = t3 = ... = t, then average speed is given by the arithmetic mean of the speeds
- When a particle moves different distances s1, s2, s3,..., sn with speeds v1, v2, v3,..., vn respectively, then the average speed of the particle over the total distance is given as
If s1 = s2 = s3 = ... = s, then average speed is given by the harmonic mean of the speeds
Relationship to acceleration
Although velocity is defined as the rate of change of position, it is often common to start with an expression for an object's acceleration. As seen by the three green tangent lines in the figure, an object's instantaneous acceleration at a point in time is the slope of the line tangent to the curve of a v(t) graph at that point. In other words, instantaneous acceleration is defined as the derivative of velocity with respect to time:
From there, velocity is expressed as the area under an a(t) acceleration vs. time graph. As above, this is done using the concept of the integral:
Constant acceleration
In the special case of constant acceleration, velocity can be studied using the suvat equations. By considering a as being equal to some arbitrary constant vector, this shows with v as the velocity at time t and u as the velocity at time t = 0. By combining this equation with the suvat equation x = ut + at/2, it is possible to relate the displacement and the average velocity by It is also possible to derive an expression for the velocity independent of time, known as the Torricelli equation, as follows: where v = |v| etc.
The above equations are valid for both Newtonian mechanics and special relativity. Where Newtonian mechanics and special relativity differ is in how different observers would describe the same situation. In particular, in Newtonian mechanics, all observers agree on the value of t and the transformation rules for position create a situation in which all non-accelerating observers would describe the acceleration of an object with the same values. Neither is true for special relativity. In other words, only relative velocity can be calculated.
Quantities that are dependent on velocity
Momentum
In classical mechanics, Newton's second law defines momentum, p, as a vector that is the product of an object's mass and velocity, given mathematically aswhere m is the mass of the object.
Kinetic energy
The kinetic energy of a moving object is dependent on its velocity and is given by the equationwhere Ek is the kinetic energy. Kinetic energy is a scalar quantity as it depends on the square of the velocity.
Drag (fluid resistance)
In fluid dynamics, drag is a force acting opposite to the relative motion of any object moving with respect to a surrounding fluid. The drag force, , is dependent on the square of velocity and is given aswhere
- is the density of the fluid,
- is the speed of the object relative to the fluid,
- is the cross sectional area, and
- is the drag coefficient – a dimensionless number.
Escape velocity
Escape velocity is the minimum speed a ballistic object needs to escape from a massive body such as Earth. It represents the kinetic energy that, when added to the object's gravitational potential energy (which is always negative), is equal to zero. The general formula for the escape velocity of an object at a distance r from the center of a planet with mass M iswhere G is the gravitational constant and g is the gravitational acceleration. The escape velocity from Earth's surface is about 11 200 m/s, and is irrespective of the direction of the object. This makes "escape velocity" somewhat of a misnomer, as the more correct term would be "escape speed": any object attaining a velocity of that magnitude, irrespective of atmosphere, will leave the vicinity of the base body as long as it does not intersect with something in its path.
The Lorentz factor of special relativity
In special relativity, the dimensionless Lorentz factor appears frequently, and is given bywhere γ is the Lorentz factor and c is the speed of light.
Relative velocity
Main article: Relative velocityRelative velocity is a measurement of velocity between two objects as determined in a single coordinate system. Relative velocity is fundamental in both classical and modern physics, since many systems in physics deal with the relative motion of two or more particles.
Consider an object A moving with velocity vector v and an object B with velocity vector w; these absolute velocities are typically expressed in the same inertial reference frame. Then, the velocity of object A relative to object B is defined as the difference of the two velocity vectors: Similarly, the relative velocity of object B moving with velocity w, relative to object A moving with velocity v is: Usually, the inertial frame chosen is that in which the latter of the two mentioned objects is in rest.
In Newtonian mechanics, the relative velocity is independent of the chosen inertial reference frame. This is not the case anymore with special relativity in which velocities depend on the choice of reference frame.
Scalar velocities
In the one-dimensional case, the velocities are scalars and the equation is either: if the two objects are moving in opposite directions, or: if the two objects are moving in the same direction.
Coordinate systems
Cartesian coordinates
In multi-dimensional Cartesian coordinate systems, velocity is broken up into components that correspond with each dimensional axis of the coordinate system. In a two-dimensional system, where there is an x-axis and a y-axis, corresponding velocity components are defined as
The two-dimensional velocity vector is then defined as . The magnitude of this vector represents speed and is found by the distance formula as
In three-dimensional systems where there is an additional z-axis, the corresponding velocity component is defined as
The three-dimensional velocity vector is defined as with its magnitude also representing speed and being determined by
While some textbooks use subscript notation to define Cartesian components of velocity, others use , , and for the -, -, and -axes respectively.
Polar coordinates
See also: Circular_motion § In_polar_coordinates; and Radial, transverse, normal
In polar coordinates, a two-dimensional velocity is described by a radial velocity, defined as the component of velocity away from or toward the origin, and a transverse velocity, perpendicular to the radial one. Both arise from angular velocity, which is the rate of rotation about the origin (with positive quantities representing counter-clockwise rotation and negative quantities representing clockwise rotation, in a right-handed coordinate system).
The radial and traverse velocities can be derived from the Cartesian velocity and displacement vectors by decomposing the velocity vector into radial and transverse components. The transverse velocity is the component of velocity along a circle centered at the origin. where
- is the transverse velocity
- is the radial velocity.
The radial speed (or magnitude of the radial velocity) is the dot product of the velocity vector and the unit vector in the radial direction. where is position and is the radial direction.
The transverse speed (or magnitude of the transverse velocity) is the magnitude of the cross product of the unit vector in the radial direction and the velocity vector. It is also the dot product of velocity and transverse direction, or the product of the angular speed and the radius (the magnitude of the position). such that
Angular momentum in scalar form is the mass times the distance to the origin times the transverse velocity, or equivalently, the mass times the distance squared times the angular speed. The sign convention for angular momentum is the same as that for angular velocity. where
- is mass
The expression is known as moment of inertia. If forces are in the radial direction only with an inverse square dependence, as in the case of a gravitational orbit, angular momentum is constant, and transverse speed is inversely proportional to the distance, angular speed is inversely proportional to the distance squared, and the rate at which area is swept out is constant. These relations are known as Kepler's laws of planetary motion.
See also
- Four-velocity (relativistic version of velocity for Minkowski spacetime)
- Group velocity
- Hypervelocity
- Phase velocity
- Proper velocity (in relativity, using traveler time instead of observer time)
- Rapidity (a version of velocity additive at relativistic speeds)
- Terminal velocity
- Velocity field
- Velocity vs. time graph
Notes
- Robert Resnick and Jearl Walker, Fundamentals of Physics, Wiley; 7 Sub edition (June 16, 2004). ISBN 0-471-23231-9.
References
- "The Feynman Lectures on Physics Vol. I Ch. 8: Motion". www.feynmanlectures.caltech.edu. Retrieved 2024-01-05.
- David Halliday; Robert Resnick; Jearl Walker (2021). Fundamentals of Physics, Extended (12th ed.). John Wiley & Sons. p. 71. ISBN 978-1-119-77351-1. Extract of page 71
- Richard P. Olenick; Tom M. Apostol; David L. Goodstein (2008). The Mechanical Universe: Introduction to Mechanics and Heat (illustrated, reprinted ed.). Cambridge University Press. p. 84. ISBN 978-0-521-71592-8. Extract of page 84
- Michael J. Cardamone (2007). Fundamental Concepts of Physics. Universal-Publishers. p. 5. ISBN 978-1-59942-433-0. Extract of page 5
- Jerry D. Wilson; Anthony J. Buffa; Bo Lou (2022). College Physics Essentials, Eighth Edition (Two-Volume Set) (illustrated ed.). CRC Press. p. 40. ISBN 978-1-351-12991-6. Extract of page 40
- David Halliday; Robert Resnick; Jearl Walker (2021). Fundamentals of Physics, Extended (12th ed.). John Wiley & Sons. p. 70. ISBN 978-1-119-77351-1. Extract of page 70
- Adrian Banner (2007). The Calculus Lifesaver: All the Tools You Need to Excel at Calculus (illustrated ed.). Princeton University Press. p. 350. ISBN 978-0-691-13088-0. Extract of page 350
- ^ Giri & Bannerjee (2002). Statistical Tools and Technique. Academic Publishers. p. 4. ISBN 978-81-87504-39-9. Extract of page 4
- Bekir Karaoglu (2020). Classical Physics: A Two-Semester Coursebook. Springer Nature. p. 41. ISBN 978-3-030-38456-2. Extract of page 41
- David Halliday; Robert Resnick; Jearl Walker (2010). Fundamentals of Physics, Chapters 33-37. John Wiley & Sons. p. 1080. ISBN 978-0-470-54794-6. Extract of page 1080
- For Earth's atmosphere, the air density can be found using the barometric formula. It is 1.293 kg/m at 0 °C and 1 atmosphere.
- Jim Breithaupt (2000). New Understanding Physics for Advanced Level (illustrated ed.). Nelson Thornes. p. 231. ISBN 978-0-7487-4314-8. Extract of page 231
- Eckehard W Mielke (2022). Modern Aspects Of Relativity. World Scientific. p. 98. ISBN 978-981-12-4406-3. Extract of page 98
- Basic principle
- "The Feynman Lectures on Physics Vol. I Ch. 9: Newton's Laws of Dynamics". www.feynmanlectures.caltech.edu. Retrieved 2024-01-04.
- White, F. M. (2008). Fluid mechanics. The McGraw Hill Companies,.
- E. Graham; Aidan Burrows; Brian Gaulter (2002). Mechanics, Volume 6 (illustrated ed.). Heinemann. p. 77. ISBN 978-0-435-51311-5. Extract of page 77
- Anup Goel; H. J. Sawant (2021). Engineering Mechanics. Technical Publications. p. 8. ISBN 978-93-332-2190-0. Extract of page 8
External links
| Kinematics | |
|---|---|
| |

 , divided by the duration of the period,
, divided by the duration of the period,  , given mathematically as
, given mathematically as





 If s1 = s2 = s3 = ... = s, then average speed is given by the
If s1 = s2 = s3 = ... = s, then average speed is given by the 


 with v as the velocity at time t and u as the velocity at time t = 0. By combining this equation with the suvat equation x = ut + at/2, it is possible to relate the displacement and the average velocity by
with v as the velocity at time t and u as the velocity at time t = 0. By combining this equation with the suvat equation x = ut + at/2, it is possible to relate the displacement and the average velocity by
 It is also possible to derive an expression for the velocity independent of time, known as the
It is also possible to derive an expression for the velocity independent of time, known as the 

 where v = |v| etc.
where v = |v| etc.


 , is dependent on the square of velocity and is given as
, is dependent on the square of velocity and is given as
 is the
is the  is the speed of the object relative to the fluid,
is the speed of the object relative to the fluid, is the
is the  is the
is the  where G is the
where G is the 
 Similarly, the relative velocity of object B moving with velocity w, relative to object A moving with velocity v is:
Similarly, the relative velocity of object B moving with velocity w, relative to object A moving with velocity v is:
 Usually, the inertial frame chosen is that in which the latter of the two mentioned objects is in rest.
Usually, the inertial frame chosen is that in which the latter of the two mentioned objects is in rest.
 if the two objects are moving in opposite directions, or:
if the two objects are moving in opposite directions, or:
 if the two objects are moving in the same direction.
if the two objects are moving in the same direction.


 . The magnitude of this vector represents speed and is found by the
. The magnitude of this vector represents speed and is found by the 

 with its magnitude also representing speed and being determined by
with its magnitude also representing speed and being determined by

 ,
,  for the
for the  -,
-,  -, and
-, and  -axes respectively.
-axes respectively.
 where
where
 is the transverse velocity
is the transverse velocity is the radial velocity.
is the radial velocity. where
where  is position and
is position and  is the radial direction.
is the radial direction.
 and the radius (the magnitude of the position).
and the radius (the magnitude of the position).
 such that
such that

 where
where
 is mass
is mass
 is known as
is known as 