Misplaced Pages

8-simplex	Rectified 8-simplex
Birectified 8-simplex	Trirectified 8-simplex
Orthogonal projections in A8 Coxeter plane

In eight-dimensional geometry, a rectified 8-simplex is a convex uniform 8-polytope, being a rectification of the regular 8-simplex.

There are unique 3 degrees of rectifications in regular 8-polytopes. Vertices of the rectified 8-simplex are located at the edge-centers of the 8-simplex. Vertices of the birectified 8-simplex are located in the triangular face centers of the 8-simplex. Vertices of the trirectified 8-simplex are located in the tetrahedral cell centers of the 8-simplex.

Rectified 8-simplex

Rectified 8-simplex
Type	uniform 8-polytope
Coxeter symbol	061
Schläfli symbol	t1{3} r{3} = {3} or ${\displaystyle \left\{{\begin{array}{l}3,3,3,3,3,3\\3\end{array}}\right\}}$
Coxeter-Dynkin diagrams	or
7-faces	18
6-faces	108
5-faces	336
4-faces	630
Cells	756
Faces	588
Edges	252
Vertices	36
Vertex figure	7-simplex prism, {}×{3,3,3,3,3}
Petrie polygon	enneagon
Coxeter group	A8, , order 362880
Properties	convex

E. L. Elte identified it in 1912 as a semiregular polytope, labeling it as S
₈. It is also called 0_6,1 for its branching Coxeter-Dynkin diagram, shown as .

Coordinates

The Cartesian coordinates of the vertices of the rectified 8-simplex can be most simply positioned in 9-space as permutations of (0,0,0,0,0,0,0,1,1). This construction is based on facets of the rectified 9-orthoplex.

Images

orthographic projections

Ak Coxeter plane	A8	A7	A6	A5
Graph
Dihedral symmetry
Ak Coxeter plane	A4	A3	A2
Graph
Dihedral symmetry

Birectified 8-simplex

Birectified 8-simplex
Type	uniform 8-polytope
Coxeter symbol	052
Schläfli symbol	t2{3} 2r{3} = {3} or ${\displaystyle \left\{{\begin{array}{l}3,3,3,3,3\\3,3\end{array}}\right\}}$
Coxeter-Dynkin diagrams	or
7-faces	18
6-faces	144
5-faces	588
4-faces	1386
Cells	2016
Faces	1764
Edges	756
Vertices	84
Vertex figure	{3}×{3,3,3,3}
Coxeter group	A8, , order 362880
Properties	convex

E. L. Elte identified it in 1912 as a semiregular polytope, labeling it as S
₈. It is also called 0_5,2 for its branching Coxeter-Dynkin diagram, shown as .

The birectified 8-simplex is the vertex figure of the 1₅₂ honeycomb.

Coordinates

The Cartesian coordinates of the vertices of the birectified 8-simplex can be most simply positioned in 9-space as permutations of (0,0,0,0,0,0,1,1,1). This construction is based on facets of the birectified 9-orthoplex.

Images

orthographic projections

Ak Coxeter plane	A8	A7	A6	A5
Graph
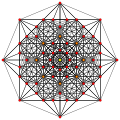
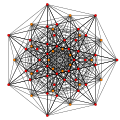
Dihedral symmetry
Ak Coxeter plane	A4	A3	A2
Graph
Dihedral symmetry

Trirectified 8-simplex

Trirectified 8-simplex
Type	uniform 8-polytope
Coxeter symbol	043
Schläfli symbol	t3{3} 3r{3} = {3} or ${\displaystyle \left\{{\begin{array}{l}3,3,3,3\\3,3,3\end{array}}\right\}}$
Coxeter-Dynkin diagrams	or
7-faces	9 + 9
6-faces	36 + 72 + 36
5-faces	84 + 252 + 252 + 84
4-faces	126 + 504 + 756 + 504
Cells	630 + 1260 + 1260
Faces	1260 + 1680
Edges	1260
Vertices	126
Vertex figure	{3,3}×{3,3,3}
Petrie polygon	enneagon
Coxeter group	A7, , order 362880
Properties	convex

E. L. Elte identified it in 1912 as a semiregular polytope, labeling it as S
₈. It is also called 0_4,3 for its branching Coxeter-Dynkin diagram, shown as .

Coordinates

The Cartesian coordinates of the vertices of the trirectified 8-simplex can be most simply positioned in 9-space as permutations of (0,0,0,0,0,1,1,1,1). This construction is based on facets of the trirectified 9-orthoplex.

Images

orthographic projections

Ak Coxeter plane	A8	A7	A6	A5
Graph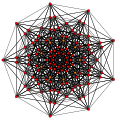
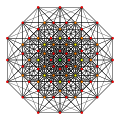
Dihedral symmetry
Ak Coxeter plane	A4	A3	A2
Graph
Dihedral symmetry

Related polytopes

This polytope is the vertex figure of the 9-demicube, and the edge figure of the uniform 2₆₁ honeycomb.

It is also one of 135 uniform 8-polytopes with A₈ symmetry.

A8 polytopes
t0	t1	t2	t3	t01	t02	t12	t03	t13	t23	t04	t14	t24	t34	t05
t15	t25	t06	t16	t07	t012	t013	t023	t123	t014	t024	t124	t034	t134	t234
t015	t025	t125	t035	t135	t235	t045	t145	t016	t026	t126	t036	t136	t046	t056
t017	t027	t037	t0123	t0124	t0134	t0234	t1234	t0125	t0135	t0235	t1235	t0145	t0245	t1245
t0345	t1345	t2345	t0126	t0136	t0236	t1236	t0146	t0246	t1246	t0346	t1346	t0156	t0256	t1256
t0356	t0456	t0127	t0137	t0237	t0147	t0247	t0347	t0157	t0257	t0167	t01234	t01235	t01245	t01345
t02345	t12345	t01236	t01246	t01346	t02346	t12346	t01256	t01356	t02356	t12356	t01456	t02456	t03456	t01237
t01247	t01347	t02347	t01257	t01357	t02357	t01457	t01267	t01367	t012345	t012346	t012356	t012456	t013456	t023456
t123456	t012347	t012357	t012457	t013457	t023457	t012367	t012467	t013467	t012567	t0123456	t0123457	t0123467	t0123567	t01234567

Notes

References

• H.S.M. Coxeter:
  • H.S.M. Coxeter, Regular Polytopes, 3rd Edition, Dover New York, 1973
  • Kaleidoscopes: Selected Writings of H.S.M. Coxeter, edited by F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication, 1995, ISBN 978-0-471-01003-6
    • (Paper 22) H.S.M. Coxeter, Regular and Semi Regular Polytopes I,
    • (Paper 23) H.S.M. Coxeter, Regular and Semi-Regular Polytopes II,
    • (Paper 24) H.S.M. Coxeter, Regular and Semi-Regular Polytopes III,
• Norman Johnson Uniform Polytopes, Manuscript (1991)
  • N.W. Johnson: The Theory of Uniform Polytopes and Honeycombs, Ph.D.
• Klitzing, Richard. "8D Uniform polytopes (polyzetta)". o3x3o3o3o3o3o3o - rene, o3o3x3o3o3o3o3o - brene, o3o3o3x3o3o3o3o - trene

External links

• Polytopes of Various Dimensions
• Multi-dimensional Glossary

• 8-polytopes