Misplaced Pages

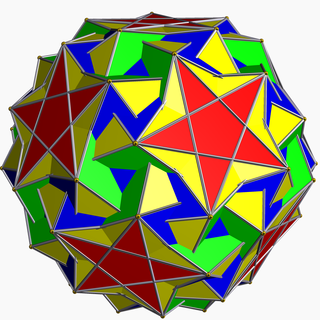
Snub icosidodecadodecahedron
Type	Uniform star polyhedron
Elements	F = 104, E = 180 V = 60 (χ = −16)
Faces by sides	(20+60){3}+12{5}+12{5/2}
Coxeter diagram
Wythoff symbol	| 5/3 3 5
Symmetry group	I, , 532
Index references	U46, C58, W112
Dual polyhedron	Medial hexagonal hexecontahedron
Vertex figure	3.3.3.5.3.5/3
Bowers acronym	Sided

In geometry, the snub icosidodecadodecahedron is a nonconvex uniform polyhedron, indexed as U₄₆. It has 104 faces (80 triangles, 12 pentagons, and 12 pentagrams), 180 edges, and 60 vertices. As the name indicates, it belongs to the family of snub polyhedra.

Cartesian coordinates

Let ${\displaystyle \rho \approx 1.3247179572447454}$ be the real zero of the polynomial ${\displaystyle x^{3}-x-1}$. The number ${\displaystyle \rho }$ is known as the plastic ratio. Denote by ${\displaystyle \phi }$ the golden ratio. Let the point ${\displaystyle p}$ be given by

${\displaystyle p={\begin{pmatrix}\rho \\\phi ^{2}\rho ^{2}-\phi ^{2}\rho -1\\-\phi \rho ^{2}+\phi ^{2}\end{pmatrix}}}$.

Let the matrix ${\displaystyle M}$ be given by

${\displaystyle M={\begin{pmatrix}1/2&-\phi /2&1/(2\phi )\\\phi /2&1/(2\phi )&-1/2\\1/(2\phi )&1/2&\phi /2\end{pmatrix}}}$.

${\displaystyle M}$ is the rotation around the axis ${\displaystyle (1,0,\phi )}$ by an angle of ${\displaystyle 2\pi /5}$, counterclockwise. Let the linear transformations ${\displaystyle T_{0},\ldots ,T_{11}}$ be the transformations which send a point ${\displaystyle (x,y,z)}$ to the even permutations of ${\displaystyle (\pm x,\pm y,\pm z)}$ with an even number of minus signs. The transformations ${\displaystyle T_{i}}$ constitute the group of rotational symmetries of a regular tetrahedron. The transformations ${\displaystyle T_{i}M^{j}}$ ${\displaystyle (i=0,\ldots ,11}$, ${\displaystyle j=0,\ldots ,4)}$ constitute the group of rotational symmetries of a regular icosahedron. Then the 60 points ${\displaystyle T_{i}M^{j}p}$ are the vertices of a snub icosidodecadodecahedron. The edge length equals ${\displaystyle 2{\sqrt {\phi ^{2}\rho ^{2}-2\phi -1}}}$, the circumradius equals ${\displaystyle {\sqrt {(\phi +2)\rho ^{2}+\rho -3\phi -1}}}$, and the midradius equals ${\displaystyle {\sqrt {\rho ^{2}+\rho -\phi }}}$.

For a snub icosidodecadodecahedron whose edge length is 1, the circumradius is

${\displaystyle R={\frac {1}{2}}{\sqrt {\rho ^{2}+\rho +2}}\approx 1.126897912799939}$

Its midradius is

${\displaystyle r={\frac {1}{2}}{\sqrt {\rho ^{2}+\rho +1}}\approx 1.0099004435452335}$

Related polyhedra

Medial hexagonal hexecontahedron

Medial hexagonal hexecontahedron
Type	Star polyhedron
Face
Elements	F = 60, E = 180 V = 104 (χ = −16)
Symmetry group	I, , 532
Index references	DU46
dual polyhedron	Snub icosidodecadodecahedron

The medial hexagonal hexecontahedron is a nonconvex isohedral polyhedron. It is the dual of the uniform snub icosidodecadodecahedron.

See also

• List of uniform polyhedra

References

1. Maeder, Roman. "46: snub icosidodecadodecahedron". MathConsult.

• Wenninger, Magnus (1983), Dual Models, Cambridge University Press, ISBN 978-0-521-54325-5, MR 0730208

External links

• Weisstein, Eric W. "Medial hexagonal hexecontahedron". MathWorld.

This polyhedron-related article is a stub. You can help Misplaced Pages by expanding it.

• v
• t
• e

• Uniform polyhedra
• Polyhedron stubs