| This article relies largely or entirely on a single source. Relevant discussion may be found on the talk page. Please help improve this article by introducing citations to additional sources. Find sources: "Span" engineering – news · newspapers · books · scholar · JSTOR (March 2023) |
In engineering, span is the distance between two adjacent structural supports (e.g., two piers) of a structural member (e.g., a beam). Span is measured in the horizontal direction either between the faces of the supports (clear span) or between the centers of the bearing surfaces (effective span):

A span can be closed by a solid beam or by a rope. The first kind is used for bridges, the second one for power lines, overhead telecommunication lines, some type of antennas or for aerial tramways.
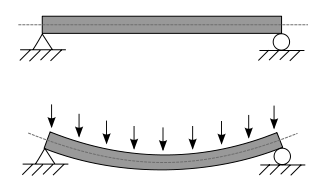
Span is a significant factor in finding the strength and size of a beam as it determines the maximum bending moment and deflection. The maximum bending moment and deflection in the pictured beam is found using:
where
- = Uniformly distributed load
- = Length of the beam between two supports (span)
- = Modulus of elasticity
- = Area moment of inertia
The maximum bending moment and deflection occur midway between the two supports. From this it follows that if the span is doubled, the maximum moment (and with it the stress) will quadruple, and deflection will increase by a factor of sixteen.
See also
- List of spans — longest spans of ropes used as power lines, antennas or aerial tramways.
- List of longest suspension bridge spans
- List of longest cantilever bridge spans
References
- Brett 2012, p. 137.
- Gere, James M.; Goodno, Barry J. (2001). Mechanics of Materials (Eighth ed.). Cengage Learning. p. 1086. ISBN 978-1-111-57773-5.
Sources
- Brett, P. (2012). Illustrated Dictionary of Building. CRC Press. ISBN 978-1-135-13857-8. Retrieved 2023-12-16.
This engineering-related article is a stub. You can help Misplaced Pages by expanding it. |
 and deflection
and deflection  in the pictured beam is found using:
in the pictured beam is found using:


 = Uniformly distributed load
= Uniformly distributed load = Length of the beam between two supports (span)
= Length of the beam between two supports (span) =
=  =
=