| Revision as of 21:58, 28 October 2007 view sourceDisavian (talk | contribs)Autopatrolled, Extended confirmed users, File movers, Pending changes reviewers, Rollbackers44,609 edits →Probability and statistics: +ref← Previous edit | Revision as of 21:58, 28 October 2007 view source Disavian (talk | contribs)Autopatrolled, Extended confirmed users, File movers, Pending changes reviewers, Rollbackers44,609 editsm →Probability and statistics: fix linkNext edit → | ||
| Line 286: | Line 286: | ||
| Note that since <math>\int_{-\infty}^{\infty} f(x)\,dx = 1</math>, for any pdf ''f''(''x''), the above formulæ can be used to produce other integral formulae for ''π''. | Note that since <math>\int_{-\infty}^{\infty} f(x)\,dx = 1</math>, for any pdf ''f''(''x''), the above formulæ can be used to produce other integral formulae for ''π''. | ||
| An empirical approximation of ''π'' is based on ] problem. Consider dropping a needle of length ''L'' repeatedly on a surface containing parallel lines drawn ''S'' units apart (with ''S'' > ''L''). If the needle is dropped ''n'' times and ''x'' of those times it comes to rest crossing a line (''x'' > 0), then one may approximate ''π'' using:<ref>{{cite web|first=Alex|last=Bogomolny|url=http://www.cut-the-knot.org/ctk/August2001.shtml|title=Math Surprises: An Example|work=]|date=2001-08|accessdate=2007-10-28}}</ref> | An empirical approximation of ''π'' is based on ] problem. Consider dropping a needle of length ''L'' repeatedly on a surface containing parallel lines drawn ''S'' units apart (with ''S'' > ''L''). If the needle is dropped ''n'' times and ''x'' of those times it comes to rest crossing a line (''x'' > 0), then one may approximate ''π'' using:<ref>{{cite web|first=Alex|last=Bogomolny|url=http://www.cut-the-knot.org/ctk/August2001.shtml|title=Math Surprises: An Example|work=]|date=2001-08|accessdate=2007-10-28}}</ref> | ||
| :<math>\pi \approx \frac{2nL}{xS}</math> | :<math>\pi \approx \frac{2nL}{xS}</math> | ||
| As a practical matter, this approximation is poor and ] very slowly. | As a practical matter, this approximation is poor and ] very slowly. | ||
Revision as of 21:58, 28 October 2007
| This article needs additional citations for verification. Please help improve this article by adding citations to reliable sources. Unsourced material may be challenged and removed. Find sources: "Pi" – news · newspapers · books · scholar · JSTOR (October 2007) (Learn how and when to remove this message) |

Pi or π is a mathematical constant, approximately equal to 3.14, that represents the ratio of any circle's circumference to its diameter in Euclidean geometry. The number also represents the ratio of a circle's area to the square of its radius, and appears in many other formulas from mathematics and geometry as well. Pi is an irrational number, which means that its decimal expansion 3.1415926535897932... never ends or repeats. Throughout the history of mathematics, much effort has been made to determine π more accurately and understand its nature; fascination with the number has even carried over into culture at large.
The Greek letter π, often spelled out pi in text, was adopted for the number from the Greek word for perimeter, probably by William Jones in 1706, and popularized by Leonhard Euler some years later. "π" is usually pronounced as pie when referring to the constant, although the letter is properly pronounced pee otherwise. The constant is occasionally also referred to as the circular constant, Archimedes' constant (not to be confused with an Archimedes number), or Ludolph's number.
| List of numbers – Irrational numbers ζ(3) – √2 – √3 – √5 – φ – α – e – π – δ | |
| Binary | 11.00100100001111110110… |
| Decimal | 3.14159265358979323846… |
| Hexadecimal | 3.243F6A8885A308D31319… |
| Continued fraction | Note that this continued fraction is not periodic. |
Fundamentals
The letter π

The name of the Greek letter π is pi, and this spelling is used in typographical contexts where the Greek letter is not available or where its usage could be problematic. When referring to this constant, the symbol π is always pronounced like "pie" in English, the conventional English pronunciation of the letter. In Greek, the name of this letter is pronounced slightly differently.
The constant is named "π" because it is the first letter of the Greek words περιφέρεια 'periphery' and περίμετρος 'perimeter', i.e. 'circumference'. π is Unicode character U+03C0 ("Greek small letter pi").
Definition

In Euclidean plane geometry, π is defined as the ratio of a circle's circumference to its diameter:
Note that the ratio /d does not depend on the size of the circle. For example, if a circle has twice the diameter d of another circle it will also have twice the circumference c, preserving the ratio /d. This fact is a consequence of the similarity of all circles.
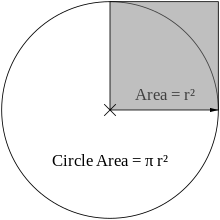
Alternatively π can be also defined as the ratio of a circle's area to the area of a square whose side is the radius:
The constant π may be defined in other ways that avoid the concepts of arc length and area, for example, as twice the smallest positive x for which cos(x) = 0. The formulæ below illustrate other (equivalent) definitions.
Irrationality and transcendence
Main article: Proof that π is irrationalThe constant π is an irrational number; that is, it cannot be written as the ratio of two integers. This was proven in 1761 by Johann Heinrich Lambert. In the 20th century, proofs were found that require no prerequisite knowledge beyond integral calculus. One of those, due to Ivan Niven, is widely known. A somewhat earlier similar proof is by Mary Cartwright. See proof that π is irrational.
Furthermore, π is also transcendental, as was proven by Ferdinand von Lindemann in 1882. This means that there is no polynomial with rational coefficients of which π is a root. An important consequence of the transcendence of π is the fact that it is not constructible. Because the coordinates of all points that can be constructed with compass and straightedge are constructible numbers, it is impossible to square the circle: that is, it is impossible to construct, using compass and straightedge alone, a square whose area is equal to the area of a given circle.
Numerical value
The numerical value of π truncated to 50 decimal places is:
- 3.14159 26535 89793 23846 26433 83279 50288 41971 69399 37510
- See the links below and those at sequence A000796 in OEIS for more digits.
While the value of pi has been computed to more than a trillion (10) digits, practical science and engineering will rarely require more than 10 decimal places. As an example, computing the circumference of the Earth's equator from its radius using only 10 decimal places of pi yields an error of less than 0.2 millimeters. A value truncated to 39 decimal places is sufficient to compute the circumference of the visible universe to a precision comparable to the size of a hydrogen atom. Most circular objects worthy of physical study, particularly on the scale of planetary radii, have imperfections and eccentricities which account for a greater error in calculation than would be yielded by calculations using approximations of pi.
The exact value of π has an infinite decimal expansion: its decimal expansion never ends and does not repeat, since π is an irrational number. This infinite sequence of digits has fascinated mathematicians and laymen alike, and much effort over the last few centuries has been put into computing more digits and investigating the number's properties. Despite much analytical work, and supercomputer calculations that have determined over 1 trillion digits of π, no simple pattern in the digits has ever been found. Digits of π are available on many web pages, and there is software for calculating π to billions of digits on any personal computer. See numerical approximations of π.
Calculating π
Main article: Computing ππ can be empirically measured by drawing a large circle, then measuring its diameter and circumference, since the circumference of a circle is always π times its diameter. π can also be calculated using purely mathematical methods. Most formulae used for calculating the value of π have desirable mathematical properties, but are difficult to understand without a background in trigonometry and calculus. However, some are quite simple, such as this form of the Gregory-Leibniz series:
While that series is easy to write and calculate, it is not immediately obvious why it yields π. In addition, this series converges so slowly that 300 terms are not sufficient to calculate π correctly to 2 decimal places. A more intuitive approach is to draw an imaginary circle of radius r centered at the origin. Then any point (x,y) whose distance d from the origin is less than r, as given by the pythagorean theorem, will be inside the circle:
Finding a collection of points inside the circle allows the circle's area A to be approximated. For example, by using integer coordinate points for a big r. Since the area A of a circle is π times the radius squared, π can be approximated by using:
History
See also: Computing π and Numerical approximations of πThe history of π parallels the development of mathematics as a whole. Some authors divide progress into three periods: the ancient period during which π was studied geometrically, the classical era following the development of calculus in Europe around the 17th century, and the age of digital computers.
Geometrical period
That the ratio of the circumference to the diameter of a circle is the same for all circles, and that it is slightly more than 3, was known to ancient Egyptian, Babylonian, Indian and Greek geometers. The earliest known approximations date from around 1900 BC; they are 25/8 (Babylonia) and 256/81 (Egypt), both within 1% of the true value. The Indian text Shatapatha Brahmana gives π as 339/108 ≈ 3.139. The Books of Kings (600 BC) appears to suggest π = 3, which is notably worse than other estimates available at the time, although the interpretation of the passage is disputed.
Archimedes (287-212 BC) was the first to estimate π rigorously. He realized that its magnitude can be bounded from below and above by inscribing circles in regular polygons and calculating the outer and inner polygons' respective perimeters:

By using the equivalent of 96-sided polygons, he proved that 223/71 < π < 22/7. Taking the average of these values yields π ≈ 3.1419. In the following centuries, most significant development took place in India and China. Around 480, the Chinese mathematician Zu Chongzhi gave the approximation π = 355/113, and showed that 3.1415926 < π < 3.1415927, which would stand as the most accurate value for π over the next 900 years.
Classical period
Until the second millennium, π was known to less than 10 decimal digits. The next major advancement in the study of π came with the development of calculus, and in particular the discovery of infinite series which in principle permit calculating π to any desired accuracy by adding sufficiently many terms. Around 1400, Madhava of Sangamagrama found the π first known such series,
now known as the Gregory-Leibniz series since it was rediscovered by James Gregory and Gottfried Leibniz in the 17th century. Unfortunately, the rate of convergence is too slow to calculate many digits in practice; about 4,000 terms must be summed to improve upon Archimedes' estimate. However, by transforming the series into
Madhava was able to calculate π as 3.14159265359, correct to 11 decimal places. The record was beaten in 1424 by the Persian astronomer Ghiyath al-Kashi, who determined 16 decimals of π.
The first major European contribution since Archimedes was made by the German mathematician Ludolph van Ceulen (1540–1610), who used a geometrical method to compute 32 decimals of π. He was so proud of the calculation, which required the most part of his life, that he had the digits engraved into his tombstone.
Around the same time, the methods of calculus and determination of infinite series and products for geometrical quantities began to emerge in Europe. The first such representation was the Viète formula,
found by François Viète in 1593. Another famous result is Wallis' product,
written down by John Wallis in 1655. Isaac Newton himself derived a series for π and calculated 15 digits, although he later confessed: "I am ashamed to tell you to how many figures I carried these computations, having no other business at the time."
John Machin was the first to compute 100 decimals of π, using the formula
with
Formulas of this type, now known as Machin-like formulas, were used to set several successive records and remained the best known method for calculating π well into the age of computers. A remarkable record was set by the calculating prodigy Johan Zacharias Dase, who in 1814 employed a Machin-like formula to calculate 200 decimals of π in his head. The best value at the end of the 19th century was due to William Shanks, who took 15 years to calculate π with 707 digits, although due to a mistake only the first 527 were correct. (To avoid such errors, modern record calculations of any kind are often performed twice, with two different formulas. If the results are the same, they are likely to be correct.)
Theoretical advances in the 18th century led to insights about π's nature that could not be achieved through numerical calculation alone. Johann Heinrich Lambert proved the irrationality of π in 1761, and Adrien-Marie Legendre proved in 1794 that also π² is irrational. When Leonhard Euler in 1735 solved the famous Basel problem – finding the exact value of
which is π²/6, he established a deep connection between π and the prime numbers. Both Legendre and Leonhard Euler speculated that π might be transcendental, a fact that was proved in 1882 by Ferdinand von Lindemann.
William Jones' book A New Introduction to Mathematics from 1706 is cited as the first text where the Greek letter π was used for this constant, but this notation became particularly popular after Leonhard Euler adopted it in 1737.
Age of computers
The advent of digital computers in the 20th century led to an increased rate of new π calculation records. John von Neumann used ENIAC to compute 2037 digits of π in 1949, a calculation that took 70 hours. Additional thousands of decimal places were obtained in the following decades, with the million-digit milestone passed in 1973. Progress was not only due to faster hardware, but also new algorithms. One of the most significant developments was the discovery of the fast Fourier transform (FFT) in the 1960s, which allows computers to perform arithmetic on extremely large numbers quickly.
In the beginning of the 20th century, the Indian mathematician Srinivasa Ramanujan found many new formulas for π, some remarkable for their elegance and mathematical depth. One of his most famous formulas is the series
which can be derived using the theory of modular functions. The series converges extraordinarily quickly, adding about eight decimals per term. Due to scarce distribution of Ramanujan's published work, it was however not widely known until around 1985 when William Gosper used it to calculate 17 million decimals of π. Based on Ramanujan's ideas, Gregory and David Chudnovsky also found the formula
which delivers 14 digits per term. The Chudnovsky brothers used this formula to set several π computing records in the end of the 1980s, including the first calculation of over one billion (1,011,196,691) decimals in 1989. It remains the formula of choice for π calculating software that runs on personal computers, as opposed to the supercomputers used to set modern records.
Whereas series typically increase the accuracy with a fixed amount for each added term, there exist iterative algorithms that multiply the number of correct digits at each step, with the downside that each step generally requires an expensive calculation. A breakthrough was made in 1975, when Richard Brent and Eugene Salamin independently discovered the Brent-Salamin algorithm, which uses only arithmetic to double the number of correct digits at each step. The algorithm consists of setting
and iterating
until an and bn are close enough. Then the estimate for π is given by
Using this scheme, 25 iterations suffice to reach 45 million correct decimals. A similar algorithm that quadruples the accuracy in each step has been found by Jonathan and Peter Borwein. The methods have been used by Yasumasa Kanada and team to set most of the π calculation records since 1980, up to a calculation of 206,158,430,000 decimals of π in 1999. The current record is 1,241,100,000,000 decimals, set by Kanada and team in 2002. Although most of Kanada's previous records were set using the Brent-Salamin algorithm, the 2002 calculation made use of two Machin-like formulas that were slower but crucially reduced memory consumption. The calculation was performed on a 64-node Hitachi supercomputer with 1 terabyte of main memory, capable of carrying out 2 trillion operations per seconds.
An important recent development was the BBP formula, found in 1995 by Simon Plouffe together with David H. Bailey and Peter Borwein. The formula,
is remarkable because it allows extracting any individual hexadecimal or binary digit of π without calculating all the preceding ones. Between 1998 and 2000, the distributed computing project PiHex used a modification of the BBP formula due to Fabrice Bellard to compute the quadrillionth (1,000,000,000,000,000:th) bit of π, which turned out to be 0.
Memorizing digits
Main article: Piphilology
Even long before computers have calculated π, memorizing a record number of digits became an obsession for some people. A Japanese man named Akira Haraguchi claims to have memorized 100,000 decimal places. This, however, has yet to be verified by Guinness World Records. The Guinness-recognized record for remembered digits of π is 67,890 digits, held by Lu Chao, a 24-year-old graduate student from China. It took him 24 hours and 4 minutes to recite to the 67,890th decimal place of π without an error.
There are many ways to memorize π, including the use of "piems", which are poems that represent π in a way such that the length of each word (in letters) represents a digit. Here is an example of a piem: How I need a drink, alcoholic in nature (or: of course), after the heavy lectures involving quantum mechanics. Notice how the first word has 3 letters, the second word has 1, the third has 4, the fourth has 1, the fifth has 5, and so on. Another example of a piem is: "May I have a large container of coffee today". The Cadaeic Cadenza contains the first 3834 digits of π in this manner. Piems are related to the entire field of humorous yet serious study that involves the use of mnemonic techniques to remember the digits of π, known as piphilology. See Pi mnemonics for examples. In other languages there are similar methods of memorization. However, this method proves inefficient for large memorizations of pi. Other methods include remembering patterns in the numbers (for instance, the year 1971 appears in the first fifty digits of pi).
Advanced properties
Numerical approximations
Main article: History of numerical approximations of πDue to the transcendental nature of π, there are no closed form expressions for the number in terms of algebraic numbers and functions. Formulae for calculating π using elementary arithmetic invariably include notation such as "...", which indicates that the formula is really a formula for an infinite sequence of approximations to π. The more terms included in a calculation, the closer to π the result will get, but none of the results will be π exactly.
Consequently, numerical calculations must use approximations of π. For many purposes, 3.14 or /7 is close enough, although engineers often use 3.1416 (5 significant figures) or 3.14159 (6 significant figures) for more precision. The approximations /7 and /113, with 3 and 7 significant figures respectively, are obtained from the simple continued fraction expansion of π. The approximation ⁄113 (3.1415929…) is the best one that may be expressed with a three-digit or four-digit numerator and denominator.
The earliest numerical approximation of π is almost certainly the value 3. In cases where little precision is required, it may be an acceptable substitute. That 3 is an underestimate follows from the fact that it is the ratio of the perimeter of an inscribed regular hexagon to the diameter of the circle.
Naturality
In non-Euclidean geometry the sum of the angles of a triangle may be more or less than π radians, and the ratio of a circle's circumference to its diameter may also differ from π. This does not change the definition of π, but it does affect many formulæ in which π appears. So, in particular, π is not affected by the shape of the universe; it is not a physical constant but a mathematical constant defined independently of any physical measurements. Nonetheless, it occurs often in physics.
For example, consider Coulomb's law (SI units)
Here, 4πr is just the surface area of sphere of radius r. In this form, it is a convenient way of describing the inverse square relationship of the force at a distance r from a point source. It would of course be possible to describe this law in other, but less convenient ways, or in some cases more convenient. If Planck charge is used, it can be written as
and thus eliminate the need for π.
Open questions
The most pressing open question about π is whether it is a normal number -- whether any digit block occurs in the expansion of π just as often as one would statistically expect if the digits had been produced completely "randomly", and that this is true in every base, not just base 10. Current knowledge on this point is very weak; e.g., it is not even known which of the digits 0,…,9 occur infinitely often in the decimal expansion of π.
Bailey and Crandall showed in 2000 that the existence of the above mentioned Bailey-Borwein-Plouffe formula and similar formulae imply that the normality in base 2 of π and various other constants can be reduced to a plausible conjecture of chaos theory. See Bailey's above mentioned web site for details.
It is also unknown whether π and e are algebraically independent. However it is known that at least one of πe and π + e is transcendental (see Lindemann–Weierstrass theorem).
Use in math and science
Main article: List of formulae involving ππ is ubiquitous in mathematics, appearing even in places that lack an obvious connection to the circles of Euclidean geometry.
Geometry and trigonometry
For any circle with radius r and diameter d = 2r, the circumference is πd and the area is πr. Further, π appears in formulas for areas and volumes of many other geometrical shapes based on circles, such as ellipses, spheres, cones, and tori. Accordingly, π appears in definite integrals that describe circumference, area or volume of shapes generated by circles. In the basic case, half the area of the unit disc is given by
and
gives half the circumference of the unit circle. More complicated shapes can be integrated as solids of revolution.
From the unit-circle definition of the trigonometric functions also follows that the sine and cosine have period 2π. That is, for all x and integers n, sin(x) = sin(x + 2πn) and cos(x) = cos(x + 2πn). Because sin(0) = 0, sin(2πn) = 0 for all integers n. Also, the angle measure of 180° is equal to π radians. In other words, 1° = 180/π.
In modern mathematics, π is often defined using trigonometric functions, for example as the smallest positive x for which sin x = 0, to avoid unnecessary dependence on the subtleties of Euclidean geometry and integration. Equivalently, π can be defined using the inverse trigonometric functions, for example as π = 2 arccos(0) or π = 4 arctan(1). Expanding inverse trigonometric functions as power series is the easiest way to derive infinite series for π.
Higher analysis and number theory
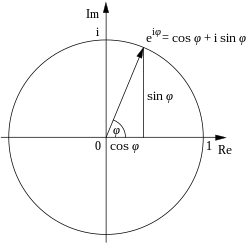
The frequent appearance of π in complex analysis can be related to the behavior of the exponential function of a complex variable, described by Euler's formula
where i is the imaginary unit satisfying i = −1 and e ≈ 2.71828 is Euler's number. This formula implies that imaginary powers of e describe rotations on the unit circle in the complex plane; these rotations have a period of 360° = 2π. In particular, the 180° rotation φ = π results in the remarkable Euler's identity
There are n different n-th roots of unity
A consequence is that the gamma function of a half-integer is a rational multiple of √π.
Physics
The number π appears routinely in equations describing fundamental principles of the Universe, due in no small part to its relationship to the nature of the circle and, correspondingly, spherical coordinate systems.
- Coulomb's law for the electric force:
Probability and statistics
In probability and statistics, there are many distributions whose formulæ contain π, including:
- probability density function (pdf) for the normal distribution with mean μ and standard deviation σ:
- pdf for the (standard) Cauchy distribution:
Note that since , for any pdf f(x), the above formulæ can be used to produce other integral formulae for π.
An empirical approximation of π is based on Buffon's needle problem. Consider dropping a needle of length L repeatedly on a surface containing parallel lines drawn S units apart (with S > L). If the needle is dropped n times and x of those times it comes to rest crossing a line (x > 0), then one may approximate π using:
As a practical matter, this approximation is poor and converges very slowly.
See also
- List of topics related to pi
- A simple proof that 22/7 exceeds π
- Feynman point comprising the 762nd through 767th decimal places of π, consisting of the digit 9 repeated six times.
- Indiana Pi Bill the attempt to legislate π = 3.2
- Pi Day
- Software for calculating π on personal computers
- Mathematical constants: e and φ
References
- OED: probably περιφέρεια or periphery
- "Characters Ordered by Unicode". W3C. Retrieved 2007-10-25.
- Rudin, Walter (1976) . Principles of mathematical analysis (3e ed.). McGraw-Hill. p. 183. ISBN 0-07-054235-X.
- "Current publisized world record of pi". Retrieved 2007-10-14.
- "Statistical estimation of pi using random vectors". Retrieved 2007-08-12.
- Beckmann, Petr (1976). A History of π. St. Martin's Griffin. ISBN 0-312-38185-9.
- "About: William Jones". Famous Welsh. Retrieved 2007-10-27.
- Borwein, Jonathan M.; Borwein, Peter; and Berggren, Lennart (2004). Pi: A Source Book, Springer. ISBN 0-387-20571-3
- Bailey, David H., Borwein, Peter B., and Plouffe, Simon (1997). "On the Rapid Computation of Various Polylogarithmic Constants" (PDF). Mathematics of Computation. 66 (218): 903–913.
{{cite journal}}: Unknown parameter|month=ignored (help)CS1 maint: multiple names: authors list (link) - Bellard, Fabrice. "A new formula to compute the n binary digit of pi". Retrieved 2007-10-27.
- Otake, Tomoko (2006-12-17). "How can anyone remember 100,000 numbers?". The Japan Times. Retrieved 2007-10-27.
{{cite news}}: Check date values in:|date=(help) - "Pi World Ranking List". Retrieved 2007-10-27.
- "Chinese student breaks Guiness record by reciting 67,890 digits of pi". News Guangdong. 2006-11-28. Retrieved 2007-10-27.
{{cite news}}: Check date values in:|date=(help) - The Life of Pi: From Archimedes to Eniac and Beyond
- Bogomolny, Alex (2001-08). "Math Surprises: An Example". cut-the-knot. Retrieved 2007-10-28.
{{cite web}}: Check date values in:|date=(help)
External links
- Digits
- First 4 Million Digits of π - Warning - Roughly 2 megabytes will be transferred.
- One million digits of pi at piday.org
- Project Gutenberg E-Text containing a million digits of π
- Search the first 200 million digits of π for arbitrary strings of numbers
- Source code for calculating the digits of π
- Pi World ranking list - List of many people who have memorized large numbers of digits of π (not up-to-date).
- General
- The Joy of Pi by David Blatner
- J J O'Connor and E F Robertson: A history of pi. Mac Tutor project
- A proof that π Is Irrational
- Lots of formulæ for π at MathWorld
- PlanetMath: Pi
- Finding the value of π
- Determination of π at cut-the-knot
- The Life of Pi by Jonathan Borwein
- BBC Radio Program about π
- Decimal expansions of Pi and related links at the On-Line Encyclopedia of Integer Sequences
- Statistical Distribution Information on PI based on 1.2 trillion digits of PI
- How to calculate Pi using the Monte Carlo method, explanation and source code in C++
Template:Link FA
Template:Link FA
Template:Link FA



































 , for any pdf f(x), the above formulæ can be used to produce other integral formulae for π.
, for any pdf f(x), the above formulæ can be used to produce other integral formulae for π.
