Pi - Misplaced Pages

This is an old revision of this page, as edited by 74.75.12.235 (talk) at 23:46, 8 October 2008 (→History). The present address (URL) is a permanent link to this revision, which may differ significantly from the current revision.

Revision as of 23:46, 8 October 2008 by 74.75.12.235 (talk) (→History)(diff) ← Previous revision | Latest revision (diff) | Newer revision → (diff)

Template:Two other uses

When a circle's diameter is 1, its circumference is π.

List of numbers – Irrational numbers ζ(3) – √2 – √3 – √5 – φ – α – e – π – δ
Binary	11.00100100001111110110…
Decimal	3.14159265358979323846…
Hexadecimal	3.243F6A8885A308D31319…
Continued fraction	$3+{\cfrac {1}{7+{\cfrac {1}{15+{\cfrac {1}{1+{\cfrac {1}{292+\ddots }}}}}}}}$ Note that this continued fraction is not periodic.

Pi or π is a mathematical constant which represents the ratio of any circle's circumference to its diameter in Euclidean geometry, which is the same as the ratio of a circle's area to the square of its radius. It is approximately equal to 3.14159. Pi is one of the most important mathematical constants: many formulae from mathematics, science, and engineering involve π.

Pi is an irrational number, which means that it cannot be expressed as a fraction m/n, where m and n are integers. Consequently its decimal representation never ends or repeats. Beyond being irrational, it is a transcendental number, which means that no finite sequence of algebraic operations on integers (powers, roots, sums, etc.) could ever produce it. Throughout the history of mathematics, much effort has been made to determine π more accurately and understand its nature; fascination with the number has even carried over into culture at large.

The Greek letter π, often spelled out pi in text, was adopted for the number from the Greek word for perimeter "περίμετρος", probably by William Jones in 1706, and popularized by Leonhard Euler some years later. The constant is occasionally also referred to as the circular constant, Archimedes' constant (not to be confused with an Archimedes number), or Ludolph's number.

pi is cool

Advanced properties

Numerical approximations

Main article: History of numerical approximations of π

Due to the transcendental nature of π, there are no closed form expressions for the number in terms of algebraic numbers and functions. Formulas for calculating π using elementary arithmetic typically include series or summation notation (such as "..."), which indicates that the formula is really a formula for an infinite sequence of approximations to π. The more terms included in a calculation, the closer to π the result will get.

Consequently, numerical calculations must use approximations of π. For many purposes, 3.14 or /₇ is close enough, although engineers often use 3.1416 (5 significant figures) or 3.14159 (6 significant figures) for more precision. The approximations /₇ and /₁₁₃, with 3 and 7 significant figures respectively, are obtained from the simple continued fraction expansion of π. The approximation ⁄₁₁₃ (3.1415929…) is the best one that may be expressed with a three-digit or four-digit numerator and denominator.

The earliest numerical approximation of π is almost certainly the value 3. In cases where little precision is required, it may be an acceptable substitute. That 3 is an underestimate follows from the fact that it is the ratio of the perimeter of an inscribed regular hexagon to the diameter of the circle.

Open questions

The most pressing open question about π is whether it is a normal number — whether any digit block occurs in the expansion of π just as often as one would statistically expect if the digits had been produced completely "randomly", and that this is true in every base, not just base 10. Current knowledge on this point is very weak; e.g., it is not even known which of the digits 0,…,9 occur infinitely often in the decimal expansion of π.

Bailey and Crandall showed in 2000 that the existence of the above mentioned Bailey-Borwein-Plouffe formula and similar formulas imply that the normality in base 2 of π and various other constants can be reduced to a plausible conjecture of chaos theory.

It is also unknown whether π and e are algebraically independent, although Yuri Nesterenko proved the algebraic independence of {π, e, Γ(1/4)} in 1996. However it is known that at least one of πe and π + e is transcendental (see Lindemann–Weierstrass theorem).

Use in mathematics and science

Main article: List of formulas involving π

π is ubiquitous in mathematics, appearing even in places that lack an obvious connection to the circles of Euclidean geometry.

Geometry and trigonometry

Higher analysis and number theory

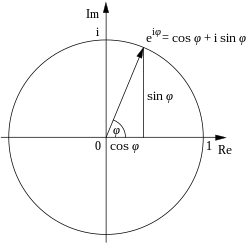
The frequent appearance of π in complex analysis can be related to the behavior of the exponential function of a complex variable, described by Euler's formula

e^{i\varphi }=\cos \varphi +i\sin \varphi \!

where i is the imaginary unit satisfying i = −1 and e ≈ 2.71828 is Euler's number. This formula implies that imaginary powers of e describe rotations on the unit circle in the complex plane; these rotations have a period of 360° = 2π. In particular, the 180° rotation φ = π results in the remarkable Euler's identity

e^{i\pi }=-1.\!

There are n different n-th roots of unity

e^{2\pi ik/n}\qquad (k=0,1,2,\dots ,n-1).

The Gaussian integral

\int _{-\infty }^{\infty }e^{-x^{2}}dx={\sqrt {\pi }}.

A consequence is that the gamma function of a half-integer is a rational multiple of √π.

Physics

Although not a physical constant, π appears routinely in equations describing fundamental principles of the Universe, due in no small part to its relationship to the nature of the circle and, correspondingly, spherical coordinate systems. Using units such as Planck units can sometimes eliminate π from formulae.

The cosmological constant:

\Lambda ={{8\pi G} \over {3c^{2}}}\rho

Heisenberg's uncertainty principle, which shows that the uncertainty in the measurement of a particle's position (Δx) and momentum (Δp) can not both be arbitrarily small at the same time:

\Delta x\,\Delta p\geq {\frac {h}{4\pi }}

Einstein's field equation of general relativity:

R_{ik}-{g_{ik}R \over 2}+\Lambda g_{ik}={8\pi G \over c^{4}}T_{ik}

Coulomb's law for the electric force, describing the force between two electric charges (q₁ and q₂) separated by distance r:

F={\frac {\left|q_{1}q_{2}\right|}{4\pi \varepsilon _{0}r^{2}}}

Magnetic permeability of free space:

\mu _{0}=4\pi \cdot 10^{-7}\,\mathrm {N/A^{2}} \,

Kepler's third law constant, relating the orbital period (P) and the semimajor axis (a) to the masses (M and m) of two co-orbiting bodies:

{\frac {P^{2}}{a^{3}}}={(2\pi )^{2} \over G(M+m)}

Probability and statistics

In probability and statistics, there are many distributions whose formulas contain π, including:

the probability density function for the normal distribution with mean μ and standard deviation σ, due to the Gaussian integral:

f(x)={1 \over \sigma {\sqrt {2\pi }}}\,e^{-(x-\mu )^{2}/(2\sigma ^{2})}

the probability density function for the (standard) Cauchy distribution:

f(x)={\frac {1}{\pi (1+x^{2})}}.

Note that since $\int _{-\infty }^{\infty }f(x)\,dx=1$ for any probability density function f(x), the above formulas can be used to produce other integral formulas for π.

Buffon's needle problem is sometimes quoted as a empirical approximation of π in "popular mathematics" works. Consider dropping a needle of length L repeatedly on a surface containing parallel lines drawn S units apart (with S > L). If the needle is dropped n times and x of those times it comes to rest crossing a line (x > 0), then one may approximate π using the Monte Carlo method:

\pi \approx {\frac {2nL}{xS}}.

Though this result is mathematically impeccable, it cannot be used to determine more than very few digits of π by experiment. Reliably getting just three digits (including the initial "3") right requires millions of throws, and the number of throws grows exponentially with the number of digits desired. Furthermore, any error in the measurement of the lengths L and S will transfer directly to an error in the approximated π. For example, a difference of a single atom in the length of a 10-centimeter needle would show up around the 9th digit of the result. In practice, uncertainties in determining whether the needle actually crosses a line when it appears to exactly touch it will limit the attainable accuracy to much less than 9 digits.