| Triangular tiling | Tetrahedral-octahedral honeycomb |
|---|---|
 With red and yellow equilateral triangles |
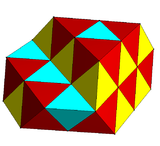 With cyan and yellow tetrahedra, and red rectified tetrahedra (octahedra) |
In geometry, the simplicial honeycomb (or n-simplex honeycomb) is a dimensional infinite series of honeycombs, based on the affine Coxeter group symmetry. It is represented by a Coxeter-Dynkin diagram as a cyclic graph of n + 1 nodes with one node ringed. It is composed of n-simplex facets, along with all rectified n-simplices. It can be thought of as an n-dimensional hypercubic honeycomb that has been subdivided along all hyperplanes , then stretched along its main diagonal until the simplices on the ends of the hypercubes become regular. The vertex figure of an n-simplex honeycomb is an expanded n-simplex.
In 2 dimensions, the honeycomb represents the triangular tiling, with Coxeter graph ![]()
![]()
![]() filling the plane with alternately colored triangles. In 3 dimensions it represents the tetrahedral-octahedral honeycomb, with Coxeter graph
filling the plane with alternately colored triangles. In 3 dimensions it represents the tetrahedral-octahedral honeycomb, with Coxeter graph ![]()
![]()
![]()
![]()
![]() filling space with alternately tetrahedral and octahedral cells. In 4 dimensions it is called the 5-cell honeycomb, with Coxeter graph
filling space with alternately tetrahedral and octahedral cells. In 4 dimensions it is called the 5-cell honeycomb, with Coxeter graph ![]()
![]()
![]()
![]()
![]() , with 5-cell and rectified 5-cell facets. In 5 dimensions it is called the 5-simplex honeycomb, with Coxeter graph
, with 5-cell and rectified 5-cell facets. In 5 dimensions it is called the 5-simplex honeycomb, with Coxeter graph ![]()
![]()
![]()
![]()
![]()
![]()
![]() , filling space by 5-simplex, rectified 5-simplex, and birectified 5-simplex facets. In 6 dimensions it is called the 6-simplex honeycomb, with Coxeter graph
, filling space by 5-simplex, rectified 5-simplex, and birectified 5-simplex facets. In 6 dimensions it is called the 6-simplex honeycomb, with Coxeter graph ![]()
![]()
![]()
![]()
![]()
![]()
![]() , filling space by 6-simplex, rectified 6-simplex, and birectified 6-simplex facets.
, filling space by 6-simplex, rectified 6-simplex, and birectified 6-simplex facets.
By dimension
Projection by folding
The (2n-1)-simplex honeycombs and 2n-simplex honeycombs can be projected into the n-dimensional hypercubic honeycomb by a geometric folding operation that maps two pairs of mirrors into each other, sharing the same vertex arrangement:
| ... | ||||||||||
| ... | ||||||||||
| ... |
Kissing number
These honeycombs, seen as tangent n-spheres located at the center of each honeycomb vertex have a fixed number of contacting spheres and correspond to the number of vertices in the vertex figure. This represents the highest kissing number for 2 and 3 dimensions, but falls short on higher dimensions. In 2-dimensions, the triangular tiling defines a circle packing of 6 tangent spheres arranged in a regular hexagon, and for 3 dimensions there are 12 tangent spheres arranged in a cuboctahedral configuration. For 4 to 8 dimensions, the kissing numbers are 20, 30, 42, 56, and 72 spheres, while the greatest solutions are 24, 40, 72, 126, and 240 spheres respectively.
See also
- Hypercubic honeycomb
- Alternated hypercubic honeycomb
- Quarter hypercubic honeycomb
- Truncated simplicial honeycomb
- Omnitruncated simplicial honeycomb
References
- George Olshevsky, Uniform Panoploid Tetracombs, Manuscript (2006) (Complete list of 11 convex uniform tilings, 28 convex uniform honeycombs, and 143 convex uniform tetracombs)
- Branko Grünbaum, Uniform tilings of 3-space. Geombinatorics 4(1994), 49 - 56.
- Norman Johnson Uniform Polytopes, Manuscript (1991)
- Coxeter, H.S.M. Regular Polytopes, (3rd edition, 1973), Dover edition, ISBN 0-486-61480-8
- Kaleidoscopes: Selected Writings of H. S. M. Coxeter, edited by F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication, 1995, ISBN 978-0-471-01003-6
- (Paper 22) H.S.M. Coxeter, Regular and Semi Regular Polytopes I, (1.9 Uniform space-fillings)
- (Paper 24) H.S.M. Coxeter, Regular and Semi-Regular Polytopes III,
| Fundamental convex regular and uniform honeycombs in dimensions 2–9 | ||||||
|---|---|---|---|---|---|---|
| Space | Family | / / | ||||
| E | Uniform tiling | 0 | δ3 | hδ3 | qδ3 | Hexagonal |
| E | Uniform convex honeycomb | 0 | δ4 | hδ4 | qδ4 | |
| E | Uniform 4-honeycomb | 0 | δ5 | hδ5 | qδ5 | 24-cell honeycomb |
| E | Uniform 5-honeycomb | 0 | δ6 | hδ6 | qδ6 | |
| E | Uniform 6-honeycomb | 0 | δ7 | hδ7 | qδ7 | 222 |
| E | Uniform 7-honeycomb | 0 | δ8 | hδ8 | qδ8 | 133 • 331 |
| E | Uniform 8-honeycomb | 0 | δ9 | hδ9 | qδ9 | 152 • 251 • 521 |
| E | Uniform 9-honeycomb | 0 | δ10 | hδ10 | qδ10 | |
| E | Uniform 10-honeycomb | 0 | δ11 | hδ11 | qδ11 | |
| E | Uniform (n-1)-honeycomb | 0 | δn | hδn | qδn | 1k2 • 2k1 • k21 |


 affine
affine  , then stretched along its main diagonal until the simplices on the ends of the hypercubes become regular. The
, then stretched along its main diagonal until the simplices on the ends of the hypercubes become regular. The 






























 /
/  /
/ 